Stability Analysis of Fractured Rock Slopes with Vertical Cracks Subjected to the Hydraulic Effect Based on the Hoek–Brown Failure Criterion

Abstract

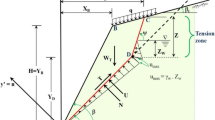
In this paper, the stability of fractured rock slopes subjected to the hydraulic effect is studied using the upper bound limit analysis. The Hoek–Brown failure criterion and the strength reduction technique are introduced to obtain the expression of the safety factor of slopes considering hydraulic effect. Based on the nonlinear sequential quadratic programming, the safety factor (Fs) and critical failure surface for fractured rock slopes are investigated with different groundwater levels and crack depths. Besides, the detailed parametric analyses of Hoek–Brown failure criterion (i.e., Geological Strength Index (GSI), the disturbance coefficient (D) and the material constant (m_i) obtained by compression tests) on fractured rock slopes are carried out. The results show that with an increase in the groundwater level and crack depth, the Fs of the fractured rock slope gradually decreases. The nonlinear strength parameters also have significant effect on the slope stability: As GSI increases, the values of Fs increase significantly; with an increase in D, the Fs gradually decreases; and with an increase in m_i, the Fs gradually decreases when the groundwater level is low but gradually increases when the groundwater level is high.

Appendix

$$ \begin{aligned} f_{1} & = \left[ {\text{e}^{{3\left( {\theta_{\text{h}} - \theta_{0} } \right)\tan \varphi_{\text{t}} }} \left( {3\tan \varphi_{\text{t}} \cos \theta_{\text{h}} + \sin \theta_{\text{h}} } \right)} \right. \\ & \quad - {{\left. {3\tan \varphi_{\text{t}} \cos \theta_{0} - \sin \theta_{0} } \right]} \mathord{\left/ {\vphantom {{\left. {3\tan \varphi_{\text{t}} \cos \theta_{0} - \sin \theta_{0} } \right]} {3\left( {1 + 9\tan^{2} \varphi_{\text{t}} } \right)}}} \right. \kern-0pt} {3\left( {1 + 9\tan^{2} \varphi_{\text{t}} } \right)}} \\ \end{aligned} $$

(27)

$$ f_{2} = \frac{1}{6}\sin \theta_{0} \frac{{L_{1} }}{{r_{0} }}\left( {2\cos \theta_{0} - \frac{{L_{1} }}{{r_{0} }}} \right) $$

(28)

$$ f_{3} = \frac{1}{6}\text{e}^{{\left( {\theta_{\text{h}} - \theta_{0} } \right)\tan \varphi_{\text{t}} }} \left[ {\sin \left( {\theta_{\text{h}} - \theta_{0} } \right) - \frac{{L_{1} }}{{r_{0} }}\sin \theta_{\text{h}} } \right]\left( {\cos \theta_{0} - \frac{{L_{1} }}{{r_{0} }} + \text{e}^{{\left( {\theta_{\text{h}} - \theta_{0} } \right)\tan \varphi_{\text{t}} }} \cos \theta_{\text{h}} } \right) $$

(29)

$$ f_{4} = \frac{1}{2}\left( {\frac{H}{{r_{0} }}} \right)^{2} \left( {\cot \beta^{{\prime }} - \cot \beta } \right)\left[ {\cos \theta_{0} - \frac{{L_{1} }}{{r_{0} }} - \frac{1}{3}\frac{H}{{r_{0} }}(\cot \beta^{{\prime }} + \cot \beta )} \right] $$

(30)

$$ p_{1} = \left[ {\text{e}^{{3\tan \varphi_{\text{t}} \left( {\theta_{\text{c}} - \theta_{0} } \right)}} \left( {3\tan \varphi_{\text{t}} \cos \theta_{\text{c}} + \sin \theta_{\text{c}} } \right) - } \right.{{\left. {3\tan \varphi_{\text{t}} \cos \theta_{0} - \sin \theta_{0} } \right]} \mathord{\left/ {\vphantom {{\left. {3\tan \varphi_{\text{t}} \cos \theta_{0} - \sin \theta_{0} } \right]} {3\left( {1 + 9\tan^{2} \varphi_{\text{t}} } \right)}}} \right. \kern-0pt} {3\left( {1 + 9\tan^{2} \varphi_{\text{t}} } \right)}} $$

(31)

$$ p_{2} = \frac{1}{6}\sin \theta_{0} \frac{{L_{2} }}{{r_{0} }}\left( {2\cos \theta_{0} - \frac{{L_{2} }}{{r_{0} }}} \right) $$

(32)

$$ p_{3} = \frac{1}{3}\text{e}^{{2\tan \varphi_{\text{t}} (\theta_{\text{c}} - \theta_{0} )}} \left( {\cos \theta_{\text{c}} } \right)^{2} \left\{ {\text{e}^{{\tan \varphi_{\text{t}} (\theta_{c} - \theta_{0} )}} \sin \theta_{\text{c}} - \sin \theta_{0} } \right\} $$

(33)

$$ \begin{aligned} f^{1} & = \left[ {\text{e}^{{3\tan \varphi_{\text{t}} \left( {\theta_{2} - \theta_{0} } \right)}} \left( {3\tan \varphi_{\text{t}} \sin \theta_{2} - \cos \theta_{2} } \right) + \text{e}^{{3\tan \varphi_{\text{t}} \left( {\theta_{\text{c}} - \theta_{0} } \right)}} } \right. \\ & \quad {{\left. {(\cos \theta_{\text{c}} - 3\tan \varphi_{\text{t}} \sin \theta_{\text{c}} )} \right]} \mathord{\left/ {\vphantom {{\left. {(\cos \theta_{\text{c}} - 3\tan \varphi_{\text{t}} \sin \theta_{\text{c}} )} \right]} {\left( {1 + 9\tan^{2} \varphi_{\text{t}} } \right)}}} \right. \kern-0pt} {\left( {1 + 9\tan^{2} \varphi_{\text{t}} } \right)}} \\ & \quad - \frac{{\sin \theta_{1} }}{{2\tan \varphi_{\text{t}} }}\left( {{\text{e}}^{{(2\theta_{2} - 3\theta_{0} + \theta_{1} )\tan \varphi_{\text{t}} }} - {\text{e}}^{{(2\theta_{\text{c}} - 3\theta_{0} + \theta_{1} )\tan \varphi_{\text{t}} }} } \right) \\ \end{aligned} $$

(34)

$$ \begin{aligned} f^{2} & = \left[ {\left( {3\tan \varphi_{\text{t}} \sin \theta_{3} - \cos \theta_{3} } \right)} \right.{\text{e}}^{{3(\theta_{3} - \theta_{0} )\tan \varphi_{\text{t}} }} \\ & \quad + {\text{e}}^{{3(\theta_{2} - \theta_{0} )\tan \varphi_{\text{t}} }} \left. {(\cos \theta_{2} - 3\tan \varphi_{\text{t}} \sin \theta_{2} )} \right]/\left( {1 + 9\tan^{2} \varphi_{\text{t}} } \right) \\ & \quad - \frac{{\sin \theta_{\text{h}} }}{{2\tan \varphi_{\text{t}} }}\left[ {{\text{e}}^{{(2\theta_{3} + \theta_{\text{h}} - 3\theta_{0} )\tan \varphi_{\text{t}} }} - {\text{e}}^{{(2\theta_{2} + \theta_{\text{h}} - 3\theta_{0} )\tan \varphi_{\text{t}} }} } \right] \\ & \quad + \tan \beta \left[ {\left( {3\tan \varphi_{\text{t}} \cos \theta_{3} + \sin \theta_{3} } \right)} \right.{\text{e}}^{{3(\theta_{3} - \theta_{0} )\tan \varphi_{\text{t}} }} \\ & \quad - {\text{e}}^{{3(\theta_{2} - \theta_{0} )\tan \varphi_{\text{t}} }} \left. {(\sin \theta_{2} + 3\tan \varphi_{\text{t}} \cos \theta_{2} )} \right]/\left( {1 + 9\tan^{2} \varphi_{\text{t}} } \right) \\ & \quad - \frac{{\cos \theta_{3} \tan \beta }}{{2\tan \varphi_{\text{t}} }}\left[ {{\text{e}}^{{3(\theta_{3} - \theta_{0} )\tan \varphi_{\text{t}} }} - {\text{e}}^{{(2\theta_{2} + \theta_{3} - 3\theta_{0} )\tan \varphi_{\text{t}} }} } \right] \\ \end{aligned} $$

(35)

$$ \begin{aligned} f^{3} & = \left[ {\left( {3\tan \varphi_{\text{t}} \sin \theta_{\text{h}} - \cos \theta_{h} } \right)} \right.{\text{e}}^{{3(\theta_{\text{h}} - \theta_{0} )\tan \varphi_{\text{t}} }} \\ & \quad + {\text{e}}^{{3(\theta_{3} - \theta_{0} )\tan \varphi_{\text{t}} }} {\kern 1pt} \left. {({ \cos }\theta_{3} - 3\tan \varphi_{\text{t}} \sin \theta_{3} )} \right]/\left( {1 + 9\tan^{2} \varphi_{\text{t}} } \right) \\ & \quad - \frac{{\sin \theta_{\text{h}} }}{{2\tan \varphi_{\text{t}} }}\left[ {{\text{e}}^{{3(\theta_{\text{h}} - \theta_{0} )\tan \varphi_{\text{t}} }} - {\text{e}}^{{(2\theta_{3} + \theta_{\text{h}} - 3\theta_{0} )\tan \varphi_{\text{t}} }} } \right] \\ \end{aligned} $$

(36)

$$ \begin{aligned} f^{1*} & = \frac{{\left[ {\left( {3\tan \varphi_{\text{t}} \sin \theta_{2} - \cos \theta_{2} } \right)} \right.{\text{e}}^{{3(\theta_{2} - \theta_{0} )\tan \varphi_{\text{t}} }} + {\text{e}}^{{3(\theta_{1} - \theta_{0} )\tan \varphi_{\text{t}} }} \left. {{\kern 1pt} (\cos \theta_{1} - 3\tan \varphi_{\text{t}} \sin \theta_{1} )} \right]}}{{1 + 9\tan^{2} \varphi_{\text{t}} }} \\ & \quad - \frac{{\sin \theta_{1} \left[ {{\text{e}}^{{(2\theta_{2} - 3\theta_{0} + \theta_{1} )\tan \varphi_{\text{t}} }} - {\text{e}}^{{3(\theta_{1} - \theta_{0} )\tan \varphi_{\text{t}} }} } \right]}}{{2\tan \varphi_{\text{t}} }} \\ \end{aligned} $$

(37)