Skip to main content
SpringerLink
Log in

TIME DELAYS IN EPIDEMIC MODELS

Modeling and Numerical Considerations

  • Conference paper
Delay Differential Equations and Applications

Part of the book series: NATO Science Series ((NAII,volume 205))

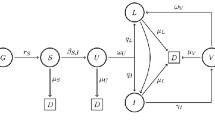
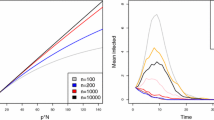
Abstract

Continuous time deterministic epidemic models are traditionally formulated as systems of ordinary differential equations for the numbers of individuals in various disease states, with the sojourn time in a state being exponentially distributed. Time delays are introduced to model constant sojourn times in a state, for example, the infective or immune state. Models then become delay-differential and/or integral equations. For a review of some epidemic models with delay see van den Driessche [228]. More generally, an arbitrarily distributed sojourn time in a state, for example, the infective or immune state, is used by some authors (see [69] and the references therein).

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution
Chapter
USD 29.95
Price excludes VAT (USA)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever
eBook
USD 349.00
Price excludes VAT (USA)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever
Softcover Book
USD 449.99
Price excludes VAT (USA)
  • Compact, lightweight edition
  • Dispatched in 3 to 5 business days
  • Free shipping worldwide - see info
Hardcover Book
USD 449.99
Price excludes VAT (USA)
  • Durable hardcover edition
  • Dispatched in 3 to 5 business days
  • Free shipping worldwide - see info

Tax calculation will be finalised at checkout

Purchases are for personal use only

Institutional subscriptions

Preview

Unable to display preview. Download preview PDF.

Unable to display preview. Download preview PDF.

Similar content being viewed by others

References

  1. M. Adimy and K. Ezzinbi, Spectral decomposition for some functional differential equations, Submitted.

    Google Scholar 

  2. E. Ait Dads, Contribution à l’existence de solutions pseudo presque périodiques d’une classe d’équations fonctionnelles, Doctorat d’Etat Unversité Cadi Ayyad, Marrakech (1994).

    Google Scholar 

  3. E. Ait Dads, K. Ezzinbi, O. Arino, Pseudo almost periodic solutions for some differential equations in a Banach space, Nonlinear Analysis, Theory, Methods & Applications 28 (1997), 1141–1155.

    Article  MathSciNet  MATH  Google Scholar 

  4. E. Ait Dads and K. Ezzinbi, Boundedness and almost periodicity for some state-dependent delay differential equations, Electron. J. Differential Equations 2002 (2002), No. 67, 1–13.

    MathSciNet  Google Scholar 

  5. W.C. Allee, Animal Aggregations: A Study in General Sociology, Chicago University Press, Chicago (1931).

    Google Scholar 

  6. H. Amann, Linear and Quasilinear Parabolic Problems, Birkhäuser (1995).

    Google Scholar 

  7. L. Amerio, and G. Prouse, Almost Periodic Functions and Functional Analysis, Van Nostrand (1971).

    Google Scholar 

  8. B. Amir and L. Maniar, Composition of pseudo almost periodic functions and semilinear Cauchy problems with non dense domain, Ann. Math. Pascal 6 (1999), 1–11.

    MathSciNet  MATH  Google Scholar 

  9. B. Amir and L. Maniar, Asymptotic behavior of hyperbolic Cauchy problems for Hille-Yosida operators with an application to retarded differential equations, Questiones Mathematicae 23 (2000), 343–357.

    MathSciNet  MATH  Google Scholar 

  10. B. Amir and L. Maniar, Existence and asymptotic behavior of solutions of semilinear Cauchy problems with non dense domain via extrapolation spaces, Rend. Circ. Mat. Palermo XLIX (2000), 481–496.

    MathSciNet  Google Scholar 

  11. Y. Ammar, Eine dreidimensionale invariante Mannigfaltigkeit für autonome Differentialgleichungen mit Verzögerung, Doctoral dissertation, Munchen (1993).

    Google Scholar 

  12. R.M. Anderson and R.M. May, Infectious Diseases of Humans, Oxford University Press (1991).

    Google Scholar 

  13. J. Arino, K.L. Cooke, P. van den Driessche, and J. Velasco- Hernández, An epidemiology model that includes vaccine efficacy and waning, Discr. Contin. Dyn. Systems B 4 (2003), 479–495.

    Google Scholar 

  14. O. Arino and M. Kimmel, Stability analysis of models of cell production systems, Math. Modelling 7 (1986), 1269–1300.

    Article  MathSciNet  MATH  Google Scholar 

  15. O. Arino, E. Sánchez and A. Fathallah, State-dependent delay differential equations in population dynamics: modeling and analysis, in “Topics in Functional Differential and Difference Equations”, ed. T. Faria and P. Freitas, Fields Institute Commun. 29 (2001), Amer. Math. Soc., Providence, pp. 19–36.

    Google Scholar 

  16. O. Arino and E. Sanchez, A saddle point theorem for functional state-dependent delay equations, preprint (2002).

    Google Scholar 

  17. A. Bátkai, L. Maniar and A. Rhandi, Regularity properties of perturbed Hille-Yosida operators and retarded differential equations, Semigroup Forum 64 (2002), 55–70.

    MathSciNet  MATH  Google Scholar 

  18. M. Bardi, An equation of growth of a single species with realistic dependence on crowding and seasonal factors, J. Math. Biol. 17 (1983), 33–43.

    Article  MathSciNet  MATH  Google Scholar 

  19. M. Bardi and A. Schiaffino, Asymptotic behavior of positive solutions of periodic delay logistic equations, J. Math. Biol. 14 (1982), 95–100.

    Article  MathSciNet  Google Scholar 

  20. M. Bartha, Convergence of solutions for an equation with statedependent delay, J. Math. Anal. Appl. 254 (2001), 410–432.

    Article  MathSciNet  MATH  Google Scholar 

  21. M. Bartha, Periodic solutions for differential equations with statedependent delay and positive feedback, Nonlinear Analysis - TMA 53 (2003), 839–857.

    Article  MathSciNet  MATH  Google Scholar 

  22. C.J.K. Batty, and R. Chill, Bounded convolutions and solutions of inhomogeneous Cauchy problems, Forum Math. 11 (1999), 253–277.

    Article  MathSciNet  MATH  Google Scholar 

  23. J.R. Beddington and R.M. May, Time delays are not necessarily destabilizing, Math. Biosci. 27 (1975), 109–117.

    Article  MATH  Google Scholar 

  24. J. Bélair, Population modles with state-dependent delays, in Mathematical Population Dynamics, ed. O. Arino, D. E. Axelrod and M. Kimmel, Marcel Dekker, New York (1991), pp. 165–176.

    Google Scholar 

  25. J. Bélair and S. A. Campbell, Stability and bifurcations of equilibrium in a multiple-delayed differential equation, SIAM J. Appl. Math. 54 (1994), 1402–1424.

    Article  MathSciNet  MATH  Google Scholar 

  26. J. Bélair, M.C. Mackey and J.M. Mahaffy, Age-structured and two delay models for erythropoiesis, Math. Biosci. 128 (1995), 317–346.

    Article  MATH  Google Scholar 

  27. E. Beretta and Y. Kuang, Geometric stability switch criteria in delay differential systems with delay dependent parameters, SIAM J. Math. Anal. 33 (2002), 1144–1165.

    Article  MathSciNet  MATH  Google Scholar 

  28. A. Beuter, J. Bélair and C. Labrie, Feedback and delay in neurological diseases: a modeling study using dynamical systems, Bull. Math. Biol. 55 (1993), 525–541.

    MATH  Google Scholar 

  29. R.H. Bing, The Geometric Topology of 3-Manifolds, A.M.S. Colloquium Publ., Vol. 40, Providence (1983).

    Google Scholar 

  30. S.P. Blythe, R.M. Nisbet and W.S.C. Gurney, Instability and complex dynamic behaviour in population models with long time delays, Theor. Pop. Biol. 22 (1982), 147–176.

    Article  MathSciNet  MATH  Google Scholar 

  31. L.L. Bonilla and A. Liñán, Relaxation oscillations, pulses, and traveling waves in the diffusive Volterra delay-differential equation, SIAM J. Appl. Math. 44 (1984), 369–391.

    Article  MathSciNet  MATH  Google Scholar 

  32. R.D. Braddock and P. van den Driessche, On a two lag differential delay equation, J. Austral. Math. Soc. Ser. B 24 (1983), 292–317.

    MATH  Google Scholar 

  33. F. Brauer. Time lag in disease models with recruitment. Mathematics and Computer Modeling 31 (2000), 11–15.

    Article  Google Scholar 

  34. F. Brauer and C. Castillo-Chávez, Mathematical Models in Population Biology and Epidemiology, Springer (2001).

    Google Scholar 

  35. N.F. Britton, Reaction-Diffusion Equations and their Applications to Biology, Academic Press, London (1986).

    MATH  Google Scholar 

  36. N.F. Britton, Spatial structures and periodic traveling waves in an integro-differential reaction-diffusion population model, SIAM J. Appl. Math. 50 (1990), 1663–1688.

    Article  MathSciNet  MATH  Google Scholar 

  37. S. Busenberg and K.L. Cooke, Periodic solutions of a periodic nonlinear delay differential equation, SIAM J. Appl. Math. 35 (1978), 704–721.

    Article  MathSciNet  MATH  Google Scholar 

  38. S. Busenberg and K.L. Cooke. Vertically Transmitted Diseases. Springer-Verlag (1993).

    Google Scholar 

  39. S. Busenberg and W. Huang, Stability and Hopf bifurcation for a population delay model with diffusion effects, J. Differential Equations 124 (1996), 80–107.

    Article  MathSciNet  MATH  Google Scholar 

  40. Y. Cao and T.C. Gard, Ultimate bounds and global asymptotic stability for differential delay equations, Rocky Mountain J. Math. 25 (1995), 119–131.

    MathSciNet  MATH  Google Scholar 

  41. M.-P. Chen, J.S. Yu, X.Z. Qian and Z.C. Wang, On the stability of a delay differential population model, Nonlinear Analysis - TMA, 25 (1995), 187–195.

    Article  MathSciNet  MATH  Google Scholar 

  42. Y. Chen, Global attractivity of a population model with statedependent delay, in “Dynamical Systems and Their Applications in Biology”, ed. S. Ruan, G. Wolkowicz and J. Wu, Fields Institute Communications 36 (2003), Amer. Math. Soc., Providence, pp. 113–118.

    Google Scholar 

  43. S.N. Chow and H.O. Walther, Characteristic multipliers and stability of symmetric periodic solutions of ̇ x(t) = g(x(t−1)). Transactions of the A.M.S. 307 (1988), 127–142.

    Article  MathSciNet  MATH  Google Scholar 

  44. Ph. Clément, O. Diekmann, M. Gyllenberg, H.J.A.M. Heijmans and H.R. Thieme, Perturbation theory for dual semigroups. I. The sun-reflexive case, Math. Ann. 277 (1987), 709–725.

    Article  MathSciNet  MATH  Google Scholar 

  45. D.S. Cohen and S. Rosenblat, A delay logistic equation with growth rate, SIAM J. Appl. Math. 42 (1982), 608–624.

    Article  MathSciNet  MATH  Google Scholar 

  46. K.L. Cooke, Stability analysis for a vector disease model, Rocky Mountain J. Math. 9 (1979), 31–42.

    Article  MathSciNet  MATH  Google Scholar 

  47. K.L. Cooke, P. van den Driessche, and X. Zou. Interaction of maturation delay and nonlinear birth in population and epidemic models. J. Math. Biol. 39 (1999) 332–352. Erratum: J. Math. Biol., 45 (2002), 470.

    Article  MathSciNet  MATH  Google Scholar 

  48. K.L. Cooke and W. Huang, On the problem of linearization for state-dependent delay differential equations. Proceedings of the A.M.S. 124 (1996), 1417–1426.

    Article  MathSciNet  MATH  Google Scholar 

  49. K.L. Cooke and J.A. Yorke, Some equations modelling growth processes and gonorrhea epidemics, Math. Biosci. 16 (1973), 75–101.

    Article  MathSciNet  MATH  Google Scholar 

  50. C. Corduneanu, Almost-Periodic Functions, 2nd edition, Chelsea, New York (1989).

    MATH  Google Scholar 

  51. C. Corduneanu, Integral Equations and Stability of Feedback Systems, Academic Press New York and London (1973).

    MATH  Google Scholar 

  52. J.M. Cushing (1977a), Integrodifferential Equations and Delay Models in Population Dynamics, Lecture Notes in Biomathematics 20 (1977), Springer-Verlag, Heidelberg.

    MATH  Google Scholar 

  53. J.M. Cushing (1977b), Time delays in single growth models, J. Math. Biol. 4 (1977), 257–264.

    Article  MathSciNet  MATH  Google Scholar 

  54. F.A. Davidson and S.A. Gourley, The effects of temporal delays in a model for a food-limited diffusion population, J. Math. Anal. Appl. 261 (2001), 633–648

    Article  MathSciNet  MATH  Google Scholar 

  55. W. Desch, G. Gühring and I. Györi, Stability of nonautonomous delay equations with a positive fundamental solution, Tübingerberichte zur Funktionalanalysis 9 (2000).

    Google Scholar 

  56. O. Diekmann, S.A. van Gils, S.M. Verduyn Lunel and H.O. Walter, Delay Equations Functional, complex and nonlinear Analysis, Springer Verlag (1991).

    Google Scholar 

  57. O. Diekmann, S.A. van Gils, S.M. Verduyn Lunel and H.O. Walther, Delay Equations: Functional-, Complex- and Nonlinear Analysis. Springer, New York (1995).

    MATH  Google Scholar 

  58. O. Diekmann and J.A.P. Heesterbeek, Mathematical Epidemiology of Infectious Diseases: Model Building, Analysis and Interpretation, Wiley (2000).

    Google Scholar 

  59. P. Dormayer, An attractivity region for characteristic multipliers of special symmetric periodic solutions of ̇ (t) = αƒ(x(t−1)) near critical amplitudes, J. Math. Anal. Appl. 169 (1992), 70–91.

    Article  MathSciNet  MATH  Google Scholar 

  60. P. Dormayer, Floquet multipliers and secondary bifurcation of periodic solutions of functional differential equations, Habilitation thesis, Gießen, 1996.

    Google Scholar 

  61. R.D. Driver, Existence theory for a delay-differential system, Contributions to Differential Equations 1 (1963), 317–336.

    MathSciNet  Google Scholar 

  62. Eichmann, M., A local Hopf bifurcation theorem for differential equations with state-dependent delays, Doctoral dissertation, Justus-Liebig-Universität, Gießen (2006).

    Google Scholar 

  63. K.J. Engel and R. Nagel, One-Parameter Semigroups for Linear Evolution Equations, Graduate Texts in Mathematics 194 Springer-Verlag (2000).

    Google Scholar 

  64. K. Ezzinbi, Contribution à l’étude des équations différentielles à retard en dimension finie et infinie : existence et aspects qualitatifs, application à des problèmes de dynamique de populations, Thesis, Marrakesh (1997).

    Google Scholar 

  65. M. D. Fargue, Reducibilité des systèmes héréditaires à des systèmes dynamiques, C. R. Acad. Sci. Paris. Sér. B 277 (1973), 471–473.

    MathSciNet  Google Scholar 

  66. T. Faria and Huang, Stability of periodic solutions arising from Hopf bifurcation for a reaction-diffusion equation with time delay, in “Differential Equations ad Dynamical Systems”, ed. A. Galves, J. K. Hale and C. Rocha, Fields Institute Communications 31 (2002), Amer. Math. Soc., Providence, pp. 125–141.

    Google Scholar 

  67. W. Feng and X. Lu, Asymptotic periodicity in diffusive logistic equations with discrete delays, Nonlinear Analysis - TMA 26 (1996), 171–178.

    Article  MathSciNet  MATH  Google Scholar 

  68. W. Feng and X. Lu, Global periodicity in a class of reactiondiffusion systems with time delays, Discrete Contin. Dynam. Systems - Ser. B 3 (2003), 69–78.

    MathSciNet  MATH  Google Scholar 

  69. Z. Feng and H.R. Thieme, Endemic models with arbitrarily distributed periods of infection I: Fundamental properties of the model, SIAM J. Appl. Math. 61 (2000), 803–833.

    Article  MathSciNet  MATH  Google Scholar 

  70. P. Fife, Mathematical Aspects of Reacting and Diffusing Systems, Lecture Notes in Biomathematics 28, Springer-Verlag, Berlin (1979).

    Google Scholar 

  71. R.A. Fisher (1937), The wave of advance of advantageous genes, Ann. Eugenics 7, 353–369.

    Google Scholar 

  72. A.C. Flower and M.C. Mackey, Relaxation oscillations in a class of delay differential equations, SIAM J. Appl. Math. 63 (2002), 299–323.

    Article  MathSciNet  Google Scholar 

  73. H.I. Freedman and K. Gopalsamy, Global stability in time-delayed single-species dynamics, Bull. Math. Biol. 48 (1986), 485–492.

    MathSciNet  MATH  Google Scholar 

  74. H.I. Freedman and J.Wu, Periodic solutions of single-species models with periodic delay, SIAM J. Math. Anal. 23 (1992), 689–701.

    Article  MathSciNet  MATH  Google Scholar 

  75. H.I. Freedman and H. Xia, Periodic solutions of single species models with delay, in “Differential Equations, Dynamical Systems, and Control Science”, ed. K. D. Elworthy, W. N. Everitt and E. B. Lee, Marcel Dekker, New York, pp. 55–74 (1994).

    Google Scholar 

  76. H.I. Freedman and X. Zhao, Global asymptotics in some quasimonotone reaction-diffusion systems with delays, J. Differential Equations 137 (1997), 340–362.

    Article  MathSciNet  MATH  Google Scholar 

  77. R.E. Gaines and J. L. Mawhin, Coincidence Degree and Nonlinear Differential Equations, Springer-Verlay, Berlin (1977).

    MATH  Google Scholar 

  78. K. Gopalsamy, Global stability in the delay-logistic equation with discrete delays, Houston J. Math. 16 (1990), 347–356.

    MathSciNet  MATH  Google Scholar 

  79. K. Gopalsamy, Stability and Oscillations in Delay Differential Equations of Population Dynamics, Mathematics and its Applications 74, Kluwer Academic Pub., Dordrecht (1992).

    MATH  Google Scholar 

  80. K. Gopalsamy and B.D. Aggarwala, The logistic equation with a diffusionally coupled delay, Bull. Math. Biol. 43 (1981), 125–140.

    MathSciNet  MATH  Google Scholar 

  81. K. Gopalsamy, M.R.S. Kulenović and G. Ladas, Time lags in a “food-limited” population model, Appl. Anal. 31 (1988), 225–237.

    MathSciNet  MATH  Google Scholar 

  82. K. Gopalsamy, M.R.S. Kulenović and G. Ladas (1990a), Enrivonmental periodicity and time delays in a “food-limited” population model, J. Math. Anal. Appl. 147 (1990), 545–555.

    Article  MATH  Google Scholar 

  83. K. Gopalsamy, M.R.S. Kulenović and G. Ladas (1990b), Oscillations and global attractivity in models of hematopoiesis, J. Dynamics Differential Equations 2 (1990), 117–132.

    Article  MATH  Google Scholar 

  84. K. Gopalsamy and G. Ladas, On the oscillation and asymptotic behavior of N(t) = N(t)[a+bN(t−τ)−cN2(t−τ)], Quart. Appl. Math. 48 (1990), 433–440.

    MathSciNet  MATH  Google Scholar 

  85. S.A. Gourley and N.F. Britton, On a modified Volterra population equation with diffusion, Nonlinear Analysis - TMA 21 (1993), 389–395.

    Article  MathSciNet  MATH  Google Scholar 

  86. S.A. Gourley and M.A.J. Chaplain, Travelling fronts in a foodlimited population model with time delay, Proc. Royal Soc. Edinburgh 132A (2002), 75–89.

    MathSciNet  Google Scholar 

  87. S.A. Gourley and S. Ruan, Dynamics of the diffusive Nicholson’s blowflies equation with distributed delay, Proc. Royal Soc. Edinburgh 130A (2000), 1275–1291.

    MathSciNet  Google Scholar 

  88. S.A. Gourley and J.W.-H. So, Dynamics of a food-limited population model incorporating nonlocal delays on a finite domain, J. Math. Biol. 44 (2002), 49–78.

    Article  MathSciNet  MATH  Google Scholar 

  89. J.R. Greaf, C. Qian and P.W. Spikes, Oscillation and global attractivity in a periodic delay equation, Canad. Math. Bull. 38 (1996), 275–283.

    Google Scholar 

  90. D. Green and H.W. Stech, Diffusion and hereditary effects in a class of population models, in “Differential Equations and Applications in Ecology, Epidemics, and Population Problems,” ed. S. Busenberg and K. Cooke, Academic Press, New York, pp. 19–28 (1981).

    Google Scholar 

  91. E.A. Grove, G. Ladas and C. Qian, Global attractivity in a “foodlimited” population model, Dynamic. Systems Appl. 2 (1993), 243–249.

    MathSciNet  MATH  Google Scholar 

  92. G. Gühring and F. Räbiger, Asymptotic properties of mild solutions of evolution equations with applications to retarded differential equations, Abstr. Apl. Anal. 4 (1999), 169–194.

    Article  MATH  Google Scholar 

  93. G. Gühring, F. Räbiger and W. Ruess, Linearized stability for semilinear non-autonomous equations with applications to retarded differential equations, to appear in Diff. Integ. Equat.

    Google Scholar 

  94. G. Gühring, F. Räbiger and R. Schnaubelt, A characteristic equation for non-autonomous partial functional differential equations, J. Differential Equations, to appear.

    Google Scholar 

  95. W.S.C. Gurney, S.P. Blythe and R.M. Nisbet, Nicholson’s blowflies revisited, Nature 287 (1980), 17–21.

    Article  Google Scholar 

  96. I. Györi and S. I. Trofimchuk, Global attractivity in x'(t) = −δx(t)+pf(x(t−τ)), Dynamic. Systems Appl. 8 (1999), 197–210.

    MATH  Google Scholar 

  97. I. Györi and S. I. Trofimchuk, On existence of rapidly oscillatory solutions in the Nicholson blowflies equation, Nonlinear Analysis - TMA 48 (2002), 1033–1042.

    Article  MATH  Google Scholar 

  98. K.P. Hadeler, On the stability of the stationary state of a population growth equation with time-lag, J. Math. Biol. 3 (1976), 197–201.

    Article  MathSciNet  MATH  Google Scholar 

  99. K.P. Hadeler and J. Tomiuk, Periodic solutions of difference differential equations, Arch. Rational Mech. Anal. 65 (1977), 82–95.

    Article  MathSciNet  Google Scholar 

  100. J.K. Hale Ordinary Differential Equations, Wiley-Inter Science, A Division of John Wiley & Sons, New York, London, Sydney, Toronto (1969).

    MATH  Google Scholar 

  101. J.K. Hale, Theory of Functional Differential Equations, Springer Verlag (1977).

    Google Scholar 

  102. J. K. Hale and W. Huang, Global geometry of the stable regions for two delay differential equations, J. Math. Anal. Appl. 178 (1993), 344–362.

    Article  MathSciNet  MATH  Google Scholar 

  103. J.K. Hale and X.B. Lin, Symbolic dynamics and nonlinear semi-flows. Annali di Matematica Pura ed Applicata 144 (1986), 229–259.

    Article  MathSciNet  MATH  Google Scholar 

  104. J.K. Hale and S. Verduyn Lunel, Introduction to Functional Differential Equations, Springer Verlag (1991).

    Google Scholar 

  105. J.K. Hale and S. Verduyn Lunel, Introduction to Functional Differential Equations. Springer, New York (1993).

    MATH  Google Scholar 

  106. T.G. Hallam and J.T. DeJuna, Effects of toxicants on populations: A qualitative approach III. Environmental and food chain pathways, J. Theor. Biol. 109 (1984), 411–429.

    Google Scholar 

  107. F. Hartung, T. Krisztin, H.O. Walther and J. Wu, Functional differential equations with state-dependent delay: Theory and applications, To appear in Handbook of Differential Equations - Ordinary Differential Equations, A. Canada, P. Drabek. and A. Fonda eds., Elsevier Science B. V., North Holland.

    Google Scholar 

  108. B.D. Hassard, N.D. Kazarinoff and Y.H. Wan (1981), Theory and Applications of Hopf Bifurcation, London Mathematical Society Lecture Note Series 41, Cambridge University Press, Cambridge.

    MATH  Google Scholar 

  109. E. Hille and R.S. Phillips, Functional Analysis and Semigroups, Amer. Math. Soc. Providence (1975).

    Google Scholar 

  110. Y. Hino, S. Murakami and T. Naito, Functional Differential Equations with Infinite Delay, Lecture Notes in Mathematics 1473, Springer Verlag (1991).

    Google Scholar 

  111. W. Huang, Global dynamics for a reaction-diffusion equation with time delay, J. Differential Equations 143 (1998), 293–326.

    Article  MathSciNet  MATH  Google Scholar 

  112. H.W. Hethcote and P. van den Driessche. Two SIS epidemiologic models with delays. J. Math. Biol., 40 (2000), 3–26.

    Article  MathSciNet  MATH  Google Scholar 

  113. G.E. Hutchinson, Circular cause systems in ecology, Ann. N. Y. Acad. Sci. 50 (1948), 221–246.

    Article  Google Scholar 

  114. G.E. Hutchinson, An Introduction to Population Ecology, Yale University Press, New Haven (1978).

    MATH  Google Scholar 

  115. A.F. Ivanov, B. Lani-Wayda and H.O. Walther, Unstable hyperbolic periodic solutions of differential delay equations. In Recent Trends in Differential Equations, R.P. Agarwal ed., 301–316, WSSIAA vol. 1, World Scientific, Singapore (1992).

    Google Scholar 

  116. A.F. Ivanov and J. Losson, Stable rapidly oscillating solutions in delay differential equations with negative feedback. Differential and Integral Equations 12 (1999), 811–832.

    MathSciNet  MATH  Google Scholar 

  117. G.S. Jones (1962a), On the nonlinear differential-difference equation ƒ'(t) = −αƒ(x − 1)[1 + ƒ(x)], J. Math. Anal. Appl. 4 (1962), 440–469.

    Article  MathSciNet  MATH  Google Scholar 

  118. G.S. Jones (1962b), The existence of periodic solutions of ƒ'(t) = −αƒ(x − 1)[1 + ƒ(x)], J. Math. Anal. Appl. 5 (1962), 435–450.

    Article  MathSciNet  Google Scholar 

  119. S. Kakutani and L. Markus, On the non-linear diffierencedi fferential equation y'(t) = [A−By(t−τ)]y(t), in “Contributions to the Theory of Nonlinear Oscillations”, Vol. 4, ed. S. Lefschetz, Princeton University Press, New Jersey, 1–18 (1958).

    Google Scholar 

  120. J.L. Kaplan and J.A. Yorke, Ordinary differential equations which yield periodic solutions of differential delay equations. J. Math. Anal. Appl. 48 (1974), 317–324.

    Article  MathSciNet  MATH  Google Scholar 

  121. J.L. Kaplan and J.A. Yorke, On the stability of a periodic solution of a differential delay equation, SIAM J. Math. Anal. 6 (1975), 268–282.

    Article  MathSciNet  MATH  Google Scholar 

  122. F. Kappel, Linear Autonomous Functional Differential Equations, Summer School on Delay Differential Equations Marrakesh June 7–16 (1995).

    Google Scholar 

  123. G. Karakostas, The effect of seasonal variations to the delay population equation, Nonlinear Analysis -TMA 6 (1982), 1143–1154.

    Article  MathSciNet  MATH  Google Scholar 

  124. G. Karakostas, Ch.G. Philos and Y.G. Sficas, Stable steady state of some population models, J. Dynamics Differential Equations 4 (1992), 161–190.

    Article  MathSciNet  MATH  Google Scholar 

  125. J. Kirk, J.S. Orr and J. Forrest, The role of chalone in the control of the bone marrow stem cell population, Math. Biosci. 6 (1970), 129–143.

    Article  Google Scholar 

  126. R.L. Kitching, Time, resources and population dynamics in insects, Austral. J. Ecol. 2 (1977), 31–42.

    Article  Google Scholar 

  127. A.N. Kolmogorov, I.G. Petrovskii and N.S. Piskunov, Étude de l’équation de le diffusion avec croissance de la quantité de mateère et son application à un problème biologique, Moscow Univ. Bull. 1 (1937), 1–25.

    Google Scholar 

  128. V.A. Kostitzin, Sur les équations intégrodifférentielles de la théorie de l’action toxique du milieu, C. R. Acad. Sci. 208 (1939), 1545–1547.

    MATH  Google Scholar 

  129. J. Kreulich, Eberlein weak almost periodicity and differential equations in Banach spaces, Ph.D. Thesis, Essen (1992).

    Google Scholar 

  130. C. Kribs-Zaleta and J. Velasco-Hernández. A simple vaccination model with multiple endemic states. Math. Biosci. 164 (2000), 183–201.

    Article  MATH  Google Scholar 

  131. H.P. Krishnan, An analysis of singularly perturbed delay-differential equations and equations with state-dependent delays, Ph.D. thesis, Brown University, Providence (R.I.) (1998).

    Google Scholar 

  132. T. Krisztin, A local unstable manifold for differential equations with state-dependent delay, Discrete and Continuous Dynamical Systems 9 (2003), 993–1028.

    MathSciNet  MATH  Google Scholar 

  133. T. Krisztin, Invariance and noninvariance of center manifolds of time-t maps with respect to the semiflow, SIAM J. Math. Analysis 36 (2004), 717–739.

    Article  MathSciNet  MATH  Google Scholar 

  134. T. Krisztin, C1-smoothness of center manifolds for differential equations with state-dependent delay, Preprint, to appear in Nonlinear Dynamics and Evolution Equations, Fields Institute Communications.

    Google Scholar 

  135. T. Krisztin and O. Arino, The 2-dimensional attractor of a differential equation with state-dependent delay, J. Dynamics Differential Equations 13 (2001), 453–522.

    Article  MathSciNet  MATH  Google Scholar 

  136. T. Krisztin and H.O. Walther, Unique periodic orbits for delayed positive feedback and the global attractor, J. Dynamics and Differential Equations 13 (2001), 1–57.

    Article  MathSciNet  MATH  Google Scholar 

  137. T. Krisztin, H.O. Walther and J. Wu, Shape, Smoothness, and Invariant Stratification of an Attracting Set for Delayed Monotone Positive Feedback. Fields Institute Monograph series vol. 11, A.M.S., Providence (1999).

    MATH  Google Scholar 

  138. T. Krisztin, H.O. Walther and J. Wu, The structure of an attracting set defined by delayed and monotone positive feedback, CWI Quarterly 12 (1999), 315–327.

    MATH  Google Scholar 

  139. T. Krisztin and J. Wu, Monotone semiflows generated by neutral equations with different delays in neutral and retarded parts, Acta Mathematicae Universitatis Comenianae 63 (1994), 207–220.

    MathSciNet  MATH  Google Scholar 

  140. Y. Kuang, Global attractivity in delay differential equations related to models of physiology and population biology, Japan. J. Indust. Appl. Math. 9 (1992), 205–238.

    MathSciNet  MATH  Google Scholar 

  141. Y. Kuang, Delay Differential Equations with Applications in Population Dynamics, Academic Press, New York (1993).

    MATH  Google Scholar 

  142. Y. Kuang and H.L. Smith (1992a), Slowly oscillating periodic solutions of autonomous state-dependent delay equations, Nonlinear Analysis - TMA 19 (1992), 855–872.

    Article  MathSciNet  MATH  Google Scholar 

  143. Y. Kuang and H.L. Smith (1992b), Periodic solutions of differential delay equations with threshold-type delays, Contemporay Mathematics 129 (1992), 153–175.

    MathSciNet  Google Scholar 

  144. M.R.S. Kulenović, G. Ladas and Y.G. Sficas, Global attractivity in Nicholson’s blowflies, Appl. Anal. 43 (1992), 109–124.

    MathSciNet  MATH  Google Scholar 

  145. G. Ladas and C. Qian, Oscillation and global stability in a delay logistic equation, Dynamics and Stability of Systems 9 (1994), 153–162.

    MathSciNet  MATH  Google Scholar 

  146. V. Lakshmikantham and S. Leela, Differential and integral inequalities, theory and applications, Volume 1, Academic Press, (1969).

    Google Scholar 

  147. B.S. Lalli and B.G. Zhang, On a periodic delay population model, Quart. Appl. Math. 52 (1994), 35–42.

    MathSciNet  MATH  Google Scholar 

  148. K.A. Landman, Bifurcation and stability theory of periodic solutions for integrodifferential equations, Stud. Appl. Math. 62 (1980), 217–248.

    MathSciNet  MATH  Google Scholar 

  149. B. Lani-Wayda, Hyperbolic Sets, Shadowing and Persistence for Noninvertible Mappings in Banach Spaces, Pitman Research Notes in Math., Vol. 334, Longman, Essex (1995).

    MATH  Google Scholar 

  150. B. Lani-Wayda, Erratic solutions of simple delay equations, Transactions of the A.M.S. 351 (1999), 901–945.

    Article  MathSciNet  MATH  Google Scholar 

  151. B. Lani-Wayda, Wandering solutions of equations with sine-like feedback. Memoirs of the A.M.S. Vol. 151, No. 718, 2001.

    Google Scholar 

  152. B. Lani-Wayda and R. Srzednicki, The Lefschetz fixed point theorem and symbolic dynamics in delay equations, Ergodic Theory and Dynamical Systems 22 (2002), 1215–1232.

    Article  MathSciNet  MATH  Google Scholar 

  153. B. Lani-Wayda and H.O. Walther, Chaotic motion generated by delayed negative feedback. Part I: A transversality criterion, Differential and Integral Equations 8 (1995), 1407–1452.

    MathSciNet  MATH  Google Scholar 

  154. B. Lani-Wayda and H.O. Walther, Chaotic motion generated by delayed negative feedback. Part II: Construction of nonlinearities, Mathematische Nachrichten 180 (1996), 141–211.

    Article  MathSciNet  MATH  Google Scholar 

  155. Y. Latushkin, T. Randolph and R. Schnaubelt, Exponential dichotomy and mild solutions of nonautonomous equations in Banach spaces, preprint.

    Google Scholar 

  156. Y. Latushkin and R. Schnaubelt, The spectral mapping theorem for evolution semigroups on Lp associated with strongly continuous cocycles, preprint.

    Google Scholar 

  157. S.M. Lenhart and C.C. Travis, Global stability of a biological model with time delay, Proc. Amer. Math. Soc. 96 (1986), 75–78.

    Article  MathSciNet  MATH  Google Scholar 

  158. X. Li, S. Ruan and J. Wei, Stability and bifurcation in delay-differential equations with two delays, J. Math. Anal. Appl. 236 (1999), 254–280.

    Article  MathSciNet  MATH  Google Scholar 

  159. Y. Li, Existence and global attractivity of a positive periodic solution of a class of delay differential equation, Sci. China Ser. A 41 (1998), 273–284.

    MathSciNet  MATH  Google Scholar 

  160. Y. Li and Y. Kuang, Periodic solutions in periodic state-dependent delay equations and population models, Proc. Amer. Math. Soc. 130 (2001), 1345–1353.

    Article  MathSciNet  Google Scholar 

  161. J. Lin and P.B. Kahn, Phase and amplitude instability in delay-diffusion population models, J. Math. Biol. 13 (1982), 383–393.

    Article  MathSciNet  MATH  Google Scholar 

  162. E. Liz, C. Martínez and S. Trofimchuk, Attractivity properties of infinite delay Mackey-Glass type equations, Differential Integral Equations 15 (2002), 875–896.

    MathSciNet  MATH  Google Scholar 

  163. E. Liz, M. Pinto, G. Robledo, S. Trofimchuk and V. Tkachenko, Wright type delay differential equations with negative Schwarzian, Discrete Contin. Dynam. Systems 9 (2003), 309–321.

    MathSciNet  MATH  Google Scholar 

  164. M. Louihi, M.L. Hbid and O. Arino, Semigroup properties and the Crandall-Liggett approximation for a class of differential equations with state-dependent delays, J. Differential Equations 181 (2002), 1–30.

    Article  MathSciNet  MATH  Google Scholar 

  165. S. Luckhaus, Global boundedness for a delay differential equation, Trans. Amer. Math. Soc. 294 (1986), 767–774.

    Article  MathSciNet  MATH  Google Scholar 

  166. N. MacDonald, Time Lags in Biological Models, Lecture Notes in Biomathematics 27 (1978), Springer-Verlag, Heidelberg.

    MATH  Google Scholar 

  167. M.C. Mackey and L. Glass, Oscillation and chaos in physiological control systems, Science 197 (1977), 287–289.

    Article  Google Scholar 

  168. M.C. Mackey and J.G. Milton, Dynamics disease, Ann. N. Y. Acad. Sci. 504 (1988), 16–32.

    Article  Google Scholar 

  169. M.C. Mackey and J.G. Milton, Feedback delays and the origins of blood cell dynamics, Comm. Theor. Biol. 1 (1990), 299–327.

    Google Scholar 

  170. J.M. Mahaffy, P.J. Zak and K.M. Joiner, A geometric analysis of the stability regions for a linear differential equation with two delays, Internat. J. Bifur. Chaos 5 (1995), 779–796.

    Article  MathSciNet  MATH  Google Scholar 

  171. J. Mallet-Paret, Morse decompositions for differential delay equations, J. Differential Equations 72 (1988), 270–315.

    Article  MathSciNet  MATH  Google Scholar 

  172. J. Mallet-Paret and R. Nussbaum, Boundary layer phenomena for differential-delay equations with state-dependent time lags, I, Arch. Rational Mech. Anal. 120 (1992), 99–146.

    Article  MathSciNet  MATH  Google Scholar 

  173. J. Mallet-Paret and R. Nussbaum, Boundary layer phenomena for differential-delay equations with state-dependent time lags, II, J. reine angew. Math. 477 (1996), 129–197.

    MathSciNet  MATH  Google Scholar 

  174. J. Mallet-Paret and R. Nussbaum, Boundary layer phenomena for differential-delay equations with state-dependent time lags, III, J. Differential Equations 189 (2003), 640–692.

    Article  MathSciNet  MATH  Google Scholar 

  175. J. Mallet-Paret, R. Nussbaum and P. Paraskevopoulos, Periodic solutions for functional differential equations with multiple statedependent time lags, Topol. Methods Nonlinear Anal. 3 (1994), 101–162.

    MathSciNet  MATH  Google Scholar 

  176. J. Mallet-Paret and G. Sell, Systems of differential delay equations: Floquet multipliers and discrete Lyapunov functions, J. Differential Equations 125 (1996), 385–440.

    Article  MathSciNet  MATH  Google Scholar 

  177. J. Mallet-Paret and H.O. Walther, Rapidly oscillating soutions are rare in scalar systems governed by monotone negative feedback with a time lag. Preprint, 1994.

    Google Scholar 

  178. L. Maniar and A. Rhandi, Extrapolation and inhomogeneous retarded differential equations in infinite dimensional Banach space via extrapolation spaces, Rend. Circ. Mat. Palermo 47 (1998), 331–346.

    Article  MathSciNet  MATH  Google Scholar 

  179. J. E. Marsden and M. McCracken, The Hopf Bifurcation and Its Applications, Applied Mathematical Sciences 19, Springer-Verlag, New York (1976).

    Google Scholar 

  180. R.M. May, Stability and Complexity in Model Ecosystems, 2nd ed., Princeton University Press, Princeton (1974).

    Google Scholar 

  181. H. McCallum, N. Barlow, and J. Hone, How should pathogen transmission be modelled? Trends Ecol. Evol., 16 (2001), 295–300.

    Article  Google Scholar 

  182. R.K. Miller, On Volterra’s population equation, SIAM J. Appl. Math. 14 (1966), 446–452.

    Article  MathSciNet  MATH  Google Scholar 

  183. R.K. Miller, Nonlinear Volterra Integral Equations, Benjamin Press, Menlo Park, California (1971).

    MATH  Google Scholar 

  184. Y. Morita, Destabilization of periodic solutions arising in delay-diffusion systems in several space dimensions, Japan J. Appl. Math. 1 (1984), 39–65.

    Article  MathSciNet  MATH  Google Scholar 

  185. J.D. Murray, Mathematical Biology, Biomathematics 19, Springer-Verlag, Berlin (1989).

    Google Scholar 

  186. R. Nagel and E. Sinestrari, Inhomogeneous Volterra integrodifferential equations for Hille-Yosida operators, in Functional Analysis (ed. K.D. Bierstedt, A. Pietsch, W.M. Ruess and D. Vogt), Lecture Notes Pure Appl. Math. 150, Marcel Dekker (1994), 51–70.

    MathSciNet  Google Scholar 

  187. J. van Neerven, The adjoint of a Semigroup of Linear Operators, Lecture Notes in Mathematics 1529, Springer-Verlag (1992).

    Google Scholar 

  188. A.J. Nicholson, The balance of animal population, J. Animal Ecol. 2 (1933), 132–178.

    Article  Google Scholar 

  189. A.J. Nicholson, An outline of the dynamics of animal populations, Austral. J. Zoo. 2 (1954), 9–65.

    Article  Google Scholar 

  190. G. Nickel and A. Rhandi, On the essential spectral radius of semigroups generated by perturbations of Hille-Yosida operators, J. Diff. Integ. Equat., to appear.

    Google Scholar 

  191. R.M. Nisbet and W.S.C. Gurney, Population dynamics in a periodically varying environment, J. Theor. Biol. 56 (1976), 459–475.

    Article  Google Scholar 

  192. R.M. Nisbet and W.S.C. Gurney, Modelling Fluctuating Populations, John Wiley and Sons (1982).

    Google Scholar 

  193. R. Nussbaum, Periodic solutions of some nonlinear autonomous functional differential equations, Ann. Mat. Pura Appl. 101 (1974), 263–306.

    Article  MathSciNet  MATH  Google Scholar 

  194. C.V. Pao, Systems of parabolic equations with continuous and discrete delays, J. Math. Anal. Appl. 205 (1997), 157–185.

    Article  MathSciNet  MATH  Google Scholar 

  195. A. Pazy, Semigroups of linear operators and applications to partial differential equations, Springer Verlag (1983).

    Google Scholar 

  196. E.R. Pianka, Evolutionary Ecology, Harper and Row, New York (1974).

    Google Scholar 

  197. C. Qian, Global attractivity in nonlinear delay differential equations, J. Math. Anal. Appl. 197 (1996), 529–547.

    Article  MathSciNet  MATH  Google Scholar 

  198. R. Redlinger, On Volterra’s population equation with diffusion, SIAM J. Math. Anal. 16 (1985), 135–142.

    Article  MathSciNet  MATH  Google Scholar 

  199. A. Rhandi, Extrapolation methods to solve non-autonomous retarded differential equations, Studia Math. 126 (1997), 219–233.

    MathSciNet  Google Scholar 

  200. A. Rhandi and R. Schnaubelt, Asymptotic behaviour of a non-autonomous population equation with diffusion in L 1, Disc. Cont. Dyn. Syst. 5 (1999), 663–683.

    MathSciNet  MATH  Google Scholar 

  201. A. Rhandi, Positivity and Stability for a population equation with diffusion on L1, Positivity 2 (1998), 101–113.

    Article  MATH  MathSciNet  Google Scholar 

  202. G. Rosen, Time delays produced by essential nonlinearity in population growth models, Bull. Math. Biol. 28 (1987), 253–256.

    Google Scholar 

  203. S. Ruan and D. Xiao, Stability of steady states and existence of traveling waves in a vector disease model, Proceedings of the royal Society of Edinburgh: Sect. A - Mathematics 134 (2004), 991–1011.

    MathSciNet  MATH  Google Scholar 

  204. S.H. Saker and S. Agarwal, Oscillation and global attractivity in a periodic nicholson’s blowflies model, Math. Comput. Modelling 35 (2002), 719–731.

    Article  MathSciNet  MATH  Google Scholar 

  205. L.F. Shampine and S. Thompson, Solving DDEs in MATLAB, Appl. Numer. Math. 37 (2001), 441–458.

    Article  MathSciNet  MATH  Google Scholar 

  206. H.C. Simpson, Stability of periodic solutions of integrodifferential equations, SIAM J. Appl. Math. 38 (1980), 341–363.

    Article  MathSciNet  MATH  Google Scholar 

  207. A. Schiaffino, On a diffusion Volterra equation, Nonlinear Analysis - TMA 3 (1979), 595–600.

    Article  MathSciNet  MATH  Google Scholar 

  208. A. Schiaffino and A. Tesei, Monotone methods and attractivity for Volterra integro-partial differential equations, Proc. Royal Soc. Edinburgh 89A (1981), 135–142.

    MathSciNet  Google Scholar 

  209. D. Schley and S.A. Gourley, Linear stability criteria for population models with periodic perturbed delays, J. Math. Biol. 40 (2000), 500–524.

    Article  MathSciNet  MATH  Google Scholar 

  210. A. Schoenfliess, Die Entwicklung der Lehre von den Punktmannigfaltigkeiten. Bericht erstattet der Deutschen Mathematiker- Vereinigung, Teil II, Jahres-Berichte der DMV, Ergänzungsband II (1908).

    Google Scholar 

  211. A.L. Skubachevsky and H.O. Walther, On Floquet multipliers for slowly oscillating periodic solutions of nonlinear functional differential equations, Trudy Moskov. Mat. Obshch.64 (2002), 3–54; English translation in: Transactions of the Moscow Mathematical Society (2003), 1–44.

    Google Scholar 

  212. H.L. Smith, Monotone Dynamical Systems, An Introduction to the Theory of Competitive and Cooperative Systems, Mathematical Surveys and Monographs 41, Amer. Math. Soc., Providence (1995).

    Google Scholar 

  213. J.W.-H. So, J. Wu and Y. Yang, Numerical steady state and Hopf bifurcation analysis on the diffusive Nicholson’s blowflies equation, Appl. Math. Comput. 111 (2000), 33–51.

    Article  MathSciNet  MATH  Google Scholar 

  214. J.W.-H. So and Y. Yang, Dirichlet problem for the diffusive Nicholson’s blowflies equation, J. Differential Equations 150 (1998), 317–348.

    Article  MathSciNet  MATH  Google Scholar 

  215. J.W.-H. So and J.S. Yu, Global attractivity and uniform persistence in Nicholson’s blowfles, Differential Equations Dynamical Systems 2 (1994), 11–18.

    MathSciNet  MATH  Google Scholar 

  216. J.W.-H. So and J.S. Yu, On the uniform stability for a “foodlimited” population model with time delay, Proc. Royal Soc. Edinburgh 125A (1995), 991–1002.

    MathSciNet  Google Scholar 

  217. J.W.-H. So and X. Zou, Traveling waves for the diffusive Nicholson’s blowflies equation, Appl. Math. Comput. 122 (2001), 385–392.

    Article  MathSciNet  MATH  Google Scholar 

  218. H. Stech, The effect of time lags on the stability of the equilibrium state of a population growth equation, J. Math. Biol. 5 (1978), 115–120.

    MathSciNet  MATH  Google Scholar 

  219. H. Steinlein and H.O. Walther, Hyperbolic sets and shadowing for noninvertible maps. In Advanced Topics in the Theory of Dynamical Systems, Fusco, G., Iannelli, M., and L. Salvadori eds., 219–234, Academic Press, New York (1989).

    Google Scholar 

  220. H. Steinlein and H.O. Walther, Hyperbolic sets, transversal homoclinic trajectories, and symbolic dynamics for C1-maps in Banach spaces, J. Dynamical Systems and Differential Equations 2 (1990), 325–365.

    Article  MathSciNet  MATH  Google Scholar 

  221. J. Sugie, On the stability for a population growth equation with time delay, Proc. Royal Soc. Edinburgh 120A (1992), 179–184.

    MathSciNet  Google Scholar 

  222. C.E. Taylor and R.R. Sokal, Oscillations in housefly population sizes due to time lags, Ecology 57 (1976), 1060–1067.

    Article  Google Scholar 

  223. A. Tesei, Stability properties for partial Volterra integrodifferential equations, Ann. Mat. Pura Appl. 126 (1980), 103–115.

    Article  MathSciNet  MATH  Google Scholar 

  224. H.R. Thieme, Semiflows generated by Lipschitz perturbations of non-densely defined linear operators, Diff. Integ. Equat. 3 (1990), 1035–1066.

    MathSciNet  MATH  Google Scholar 

  225. H.R. Thieme, The transition through stages with arbitrary length distributions, and applications in epidemics, In C. Castillo-Chavez, with S. Blower, P. van den Driessche, D. Kirschner, and A.- A. Yakubu, editors, Mathematical Approaches for Emerging and Reemerging Infectious Diseases: Models, Methods, and Theory, volume 126 of IMA Volumes in Mathematics and its Applications, pages 45–84 (2002).

    Google Scholar 

  226. C.C. Travis and G.F. Webb, Existence and stability, for partial functional differential equations, Tran. Amer. Math. Soc. 200 (1974), 395–418.

    Article  MathSciNet  MATH  Google Scholar 

  227. C.C. Travis and G.F.Webb, Existence, stability, and compacteness in the α-norm for partial functional differential equations, Tran. Amer. Math. Soc. 240 (1978), 129–143.

    Article  MathSciNet  MATH  Google Scholar 

  228. P. van den Driessche, Some epidemiological models with delays, In Differential Equations and Applications to Biology and to Industry (Claremont, CA, 1994), pages 507–520. World Sci. Publishing, River Edge, NJ (1996).

    Google Scholar 

  229. P. van den Driessche, Time delay in epidemic models, in C. Castillo-Chavez, with S. Blower, P. van den Driessche, D. Kirschner, and A.-A. Yakubu, editors, Mathematical Approaches for Emerging and Reemerging Infectious Diseases: An Introduction, volume 125 of IMA Volumes in Mathematics and its Applications, pages 119–128 (2002).

    Google Scholar 

  230. P. van den Driessche and J. Watmough, A simple SIS epidemic model with a backward bifurcation, J. Math. Biol. 40 (2000), 525–540.

    Article  MathSciNet  MATH  Google Scholar 

  231. Vanderbauwhede, A., and S.A. van Gils, Center manifolds and contractions on a scale of Banach spaces, J. Functional Analysis 71 (1987), 209–224.

    Article  Google Scholar 

  232. F.F. Verhulst, Notice sur la loi que la population suit dans son acroissement, Corr. Math. Phys. 10 (1838), 113–121.

    Google Scholar 

  233. V. Volterra, Remarques sur la note de M. Régnier et Mlle. Lambin (Étude d’un cas d’antagonisme microbien), C. R. Acad. Sci. 199 (1934), 1684–1686.

    MATH  Google Scholar 

  234. H.O. Walther, Existence of a non-constant periodic solution of a nonlinear autonomous functional differential equation representing the growth of a single species population, J. Math. Biol. 1 (1975), 227–240.

    Article  MathSciNet  MATH  Google Scholar 

  235. H.O. Walther, Stable periodic motion of a system with state dependent delay, Differential Integral Equations 15 (2002), 923–944.

    MathSciNet  MATH  Google Scholar 

  236. H.O.Walther, Über Ejektivität und periodische Lösungen bei Funktionaldifferentialgleichungen mit verteilter Verzögerung, Habilitation thesis, München (1977).

    Google Scholar 

  237. H.O. Walther, On instability, ω-limit sets and periodic solutions of nonlinear autonomous differential delay equations, in Functional Differential Equations and Approximation of Fixed Points, H.O. Peitgen and H.O. Walther eds., Lecture Notes in Math. 730, 489–503, Springer, Heidelberg (1979).

    Chapter  Google Scholar 

  238. H.O. Walther, Bifurcation from periodic solutions in functional differential equations, Mathematische Zeitschrift 182 (1983), 269–289.

    Article  MathSciNet  MATH  Google Scholar 

  239. H.O. Walther, A differential delay equation with a planar attractor, Accepted for the Proceedings of the Int. Conf. on Differential Equations Marrakech 1991.

    Google Scholar 

  240. H.O. Walther, The two-dimensional attractor of x′(t) = −μx(t)+ ƒ(x(t − 1)), Memoirs of the A.M.S. Vol. 113, No. 544, 1995.

    Google Scholar 

  241. H.O. Walther, Stable sets of periodic solutions of a delay differential equation. To appear in Proceedings of the Internat. Conf. on Differential Equations Marrakesh 1995.

    Google Scholar 

  242. H.O. Walther, The singularities of an attractor of a delay differential equation, Functional Differential Equations 5 (1998), 513–548.

    MathSciNet  MATH  Google Scholar 

  243. H.O. Walther, Contracting return maps for some delay differential equations, In Topics in Functional Differential and Difference Equations, T. Faria and P. Freitas eds., Fields Institute Communications series 29, A.M.S., Providence (2001), 349–360.

    Google Scholar 

  244. H.O. Walther, Contracting return maps for monotone delayed feedback, Discrete and Continuous Dynamical Systems 7 (2001), 259–274.

    Article  MathSciNet  MATH  Google Scholar 

  245. H.O. Walther, Stable periodic motion of a system with statedependent delay, Differential and Integral Equations 15 (2002), 923–944.

    MathSciNet  MATH  Google Scholar 

  246. H.O. Walther, Stable periodic motion of a delayed spring, Topological Methods in Nonlinear Analysis 21 (2003), 1–28.

    MathSciNet  MATH  Google Scholar 

  247. H.O. Walther, The solution manifold and C 1-smoothness of solution operators for differential equations with state dependent delay, J. Differential Eqs. 195 (2003), 46–65.

    Article  MathSciNet  MATH  Google Scholar 

  248. H.O. Walther, Stable periodic motion of a system using echo for position control, J. Dynamics and Differential Eqs. 15 (2003), 143–223.

    Article  MathSciNet  MATH  Google Scholar 

  249. H.O. Walther, Smoothness properties of semiflows for differential equations with state dependent delay, Russian, in Proceedings of the International Conference on Differential and Functional Differential Equations, Moscow, 2002 1, pp. 40–55, Moscow State Aviation Institute (MAI), Moscow (2003). English version: Journal of the Mathematical Sciences 124 (2004), 5193–5207.

    Google Scholar 

  250. H.O. Walther and M. Yebdri, Smoothness of the attractor of almost all solutions of a delay differential equation, DISSERTATIONES MATHEMATICAE CCCLXVIII (1997).

    Google Scholar 

  251. M. Wazewska-Czyzewska and A. Lasota, Mathematical problems of the dynamics of the red-blood cells system, Ann. Polish Math. Soc. III Appl. Math. 6 (1976), 23–40.

    MathSciNet  MATH  Google Scholar 

  252. E. M. Wright, A non-linear difference-differential equation, J. reine angew. Math. 194 (1955), 66–87.

    MathSciNet  MATH  Google Scholar 

  253. J. Wu, Theory and Applications of Partial Functional Differential Equations, Applied Mathematical Sciences 119, Springer-Verlag, New York (1996).

    Google Scholar 

  254. Wu, J., Stable phase-locked periodic solutions in a delay differential system, J. Differential Eqs. 194 (2003), 237–286.

    Article  MATH  Google Scholar 

  255. X. Xie, Uniqueness and stability of slowly oscillating periodic solutions of delay differential equations with bounded nonlinearity, J. Dynamics and Differential Equations 3 (1991), 515–540.

    Article  MATH  Google Scholar 

  256. J. Yan and Q. Feng, Global attractivity and oscillation in a nonlinear delay equation, Nonlinear Analysis - TMA 43 (2001), 101–108.

    Article  MathSciNet  MATH  Google Scholar 

  257. Y. Yamada, Asymptotic behavior of solutions for semilinear Volterra diffusion equations, Nonlinear Analysis - TMA 21 (1993), 227–239.

    Article  MATH  Google Scholar 

  258. K. Yoshida, The Hopf bifurcation and its stability for semilinear differential equations with time delay arising in ecology, Hiroshima Math. J. 12 (1982), 321–348.

    MathSciNet  MATH  Google Scholar 

  259. J.S. Yu (1996), Global attractivity of the zero solution of a class of functional-differential equations and its applications, Sci. China Ser. A 39 225–237.

    MathSciNet  MATH  Google Scholar 

  260. S.D. Zaidman, Abstract differential equations, Research notes in Mathematics (1994).

    Google Scholar 

  261. C. Zhang, Pseudo almost periodic functions and their applications, Ph.D thesis, University of Western Ontario (1992).

    Google Scholar 

  262. C. Zhang, Pseudo almost-periodic solutions of some differential equations, J. Math. Anal. Appl. 181 (1994), 62–76.

    Article  MathSciNet  MATH  Google Scholar 

  263. B. G. Zhang and K. Gopalsamy, Global attractivity and oscillations in a periodic delay-logistic equation, J. Math. Anal. Appl. 150 (1990), 274–283.

    Article  MathSciNet  MATH  Google Scholar 

  264. Lin Zhensheng, Theory of linear systems, Ann. of Diff. Equ. 6 (1990).

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, University of Manitoba, Winnipeg, Manitoba, Canada

    J. Arino

  2. Department of Mathematics and Statistics, University of Victoria BC, Canada

    P. van den Driessche

Authors
  1. J. Arino
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. P. van den Driessche
    View author publications

    You can also search for this author in PubMed Google Scholar

Editor information

Editors and Affiliations

  1. University of Pau, France

    O. Arino

  2. University Cadi Ayyad, Marrakech, Morocco

    M.L. Hbid

  3. University Cadi Ayyad, Marrakech, Morocco

    E. Ait Dads

Rights and permissions

Reprints and permissions

Copyright information

© 2006 Springer

About this paper

Cite this paper

Arino, J., van den Driessche, P. (2006). TIME DELAYS IN EPIDEMIC MODELS. In: Arino, O., Hbid, M., Dads, E.A. (eds) Delay Differential Equations and Applications. NATO Science Series, vol 205. Springer, Dordrecht. https://doi.org/10.1007/1-4020-3647-7_13

Download citation

Publish with us

Policies and ethics

Search

Navigation