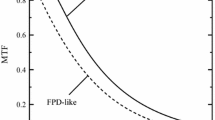
Abstract
Accurate determination of the line spread function (LSF) on the basis of the edge processing algorithm in X-ray imaging systems is one of the most basic procedures for evaluating the performance of such systems. Extensive research has been focused on algorithms for the precise or fast measurement of the LSF in digital X-ray systems. Most of the standard methods for evaluating the performance of an imaging system are based on a fully digitalized radiographic system or a film-based system. However, images obtained by computed radiography (CR), which converts a captured analog signal into a digital image through an analog-to-digital converting scanner, show the combined characteristics of analog and digital imaging systems. Fundamentally, the characteristics of digital imaging systems differ substantially from those of film imaging systems because of their different methods of acquiring and displaying image data. In addition, a system with both analog and digital component has characteristics that differ from those of both digital and analog systems. In this research, we present a new modulation transfer function (MTF) that mimics the existing MTF in terms of measurement but satisfies existing standard protocols through modification of the hypothesis contents. In the case of the LSF and the point spread function measured with a CR system, the developed edge algorithm shows better performance than the conventional methods. We also demonstrate the usefulness of this method in an actual measurement with a CR digital X-ray imaging system.
Similar content being viewed by others
References
K. Rossmann, Radiology 93, 257 (1969).
K. Rossmann, Radiology 90, 863 (1963).
J. Bushberg, J. Seibert, M. Leidholdt, Jr. and J. Boone, The Essential Physics of Medical Imaging, Second ed. (Lippincott Williams & Wilkins, Philadelphia, Pennsylvania, 2002).
H. H. Barret and W. Swindell, Radiological imaging: the theory of image formation, detection and processing (Academic Press, New York, 1981).
N. Ulrich, Radiat. Prot. Dosimetry 114, 32 (2005).
E. Samei, Advances in Digital Radiography: RSNA categorical course in Diagnostic Radiology Physics (2003), p 37.
E. Samei, M. J. Flynn and D. D. Reimann, Med. Phys. 25, 102 (1998).
J. T. Dobbins, Med. Phys. 22, 171 (1995).
P. R. Granfors and R. Aufrichtig, Med. Phys. 27, 1324 (2000).
A. F. Greene, C. W. Hartley, P. N. D. Dupuy and M. Chinader. J. Archaeol. Sci. 78, 120 (2017).
International Electrotechnical Commission, International standard IEC62220-1: Medical electrical equipment -Characteristics of digital imaging devices-Part 1: Determination of the detective quantum efficiency. (Geneva, Switzerland, 2003).
R. Bracesell, The Fourier transform and its applications (McGraw-Hill, New York, 1986).
Y. C. Ryu, Using Morphological Filter for Accurate Elimination of Scattering Effects in Line Spread Function and Modulation Transfer Function, J. Koream Phys. Soc. (submitted 2017).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Kim, Y.G., Ryu, Y. Accurate evaluation of modulation transfer function using the Fourier shift theorem. Journal of the Korean Physical Society 71, 1064–1068 (2017). https://doi.org/10.3938/jkps.71.1064
Received:
Published:
Issue Date:
DOI: https://doi.org/10.3938/jkps.71.1064