Skip to main content
SpringerLink
Log in

Optimal control in a mathematical model for leukemia therapy with phase constraints

  • Published:
Moscow University Computational Mathematics and Cybernetics Aims and scope Submit manuscript

Abstract

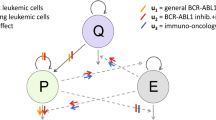
We examine the optimal control problem that arises in the mathematical modeling of leukemia therapy, to solve which the Pontryagin maximum principle and the penalty function method are employed. It is assumed that the drug is capable of killing not only diseased cells, but healthy cells as well. The character of the drug’s interaction with cells is described by appropriate therapy functions.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. E. K. Afenya and C. P. Calderon, “A Brief Look at Normal Cells Decline and Inhibition in Acute Leukemia,” J. Can. Det. Prev. 20(3), 171–191 (1996).

    Google Scholar 

  2. C. L. Frenzen and J. D. Murray, “A Cell Kinetics Justification for Gompertz Equation,” SIAM J. Appl. Math. 46, 614–624 (1986).

    Article  MathSciNet  MATH  Google Scholar 

  3. M. Gyllenberg and G. F. Webb, “Quiescence As An Explanation of Gompertzian Tumor Growth,” Growth Dev. Aging 53(1), 25–33 (1989).

    Google Scholar 

  4. W. S. Kendal, “Gompertzian Growth As a Consequence of Tumor Heterogeneity,” Math. Biosci. 73, 103–107 (1985).

    Article  MathSciNet  MATH  Google Scholar 

  5. A. K. Laird, “Dynamics of Tumor Growth: Comparison of Growth and Cell Population Dynamics,” Math. Biosci. 185, 153–167 (2003).

    Article  MathSciNet  Google Scholar 

  6. L. S. Pontryagin, V. G. Boltyanskii, R. V. Gamkrelidze, and E. F. Mishchenko, The Mathematical Theory of Optimal Processes (Nauka, Moscow, 1983; Gordon and Breach, New York, 1986).

    MATH  Google Scholar 

  7. F. P. Vasil’ev, Optimization Methods (MTsNMO, Moscow, 2011) [in Russian].

    Google Scholar 

  8. N. N. Moiseev, Elements of the Theory of Optimal Systems (Nauka, Moscow, 1975) [in Russian].

    MATH  Google Scholar 

  9. A. S. Bratus, E. Fimmel, F. Nurnberg, and Y. Todorov, “On Strategies on a Mathematical Model for Leukemia Therapy,” Nonlinear Analysis: Real World Applications 13, 1044–1059 (2011).

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Faculty of Computational Mathematics and Cybernetics, Moscow State University, Moscow, 119992, Russia

    A. S. Bratus & A. S. Goncharov

  2. Mannheim University of Applied Sciences, Mannheim, 68163, Germany

    I. T. Todorov

Authors
  1. A. S. Bratus
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. A. S. Goncharov
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. I. T. Todorov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to A. S. Bratus.

Additional information

Original Russian Text © A.S. Bratus, A.S. Goncharov, I.T. Todorov, 2012, published in Vestnik Moskovskogo Universiteta. Vychislitel’naya Matematika i Kibernetika, 2012, No. 4, pp. 25–28.

About this article

Cite this article

Bratus, A.S., Goncharov, A.S. & Todorov, I.T. Optimal control in a mathematical model for leukemia therapy with phase constraints. MoscowUniv.Comput.Math.Cybern. 36, 178–182 (2012). https://doi.org/10.3103/S0278641912040024

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.3103/S0278641912040024

Keywords

Search

Navigation