Abstract
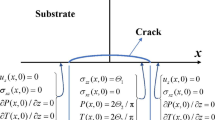
On the basis of a nonlinear model of deformation of a crystalline medium with a complex lattice, the problem of the stationary propagation of a Griffith crack under the action of homogeneous expanding stresses is posed and solved. It is shown that the stressed and deformed states of the medium are determined both by external influences on the medium and by the gradients of the optical mode (mutual displacement of atoms). The contributions from these factors are separated. Finding the components of the stress tensor and macro-displacement vector is reduced to solving Riemann–Hilbert boundary value problems. Their exact analytical solutions are obtained.
Similar content being viewed by others
REFERENCES
E. L. Aero, “Microscale deformations in a two-dimensional lattice: Structural transitions and bifurcations at critical shear,” Phys. Solid State 42, 1147–1153 (2000). https://doi.org/10.1134/1.1131331
E. L. Aero, “Micromechanics of a double continuum in a model of a medium with variable periodic structure,” J. Eng. Math. 55, 81–95 (2006). https://doi.org/10.1007/s10665-005-9012-3
A. N. Bulygin and Y. V. Pavlov, “Solution of dynamic equations of plane deformation for nonlinear model of complex crystal lattice,” in Advanced Structured Materials, Vol. 164: Mechanics and Control of Solids and Structures (Springer, Cham, 2022), pp. 115–136. https://doi.org/10.1007/978-3-030-93076-9_6
Fracture an Advanced Treatise, Ed. by H. Liebowitz, Vol. II: Mathematical Fundamentals (Academic Press, New York, 1968).
J. F. Knott, Fundamentals of Fracture Mechanics (Butterworths, London, 1973).
D. Broek, Elementary Engineering Fracture Mechanics (Martinus Nijhoff Publishers, Dordrecht, 1984).
E. L. Aero, A. N. Bulygin, and Yu. V. Pavlov, “Nonlinear deformation model of crystal media allowing martensite transformations: solution of static equations,” Mech. Solids 53, 623–632 (2018). https://doi.org/10.3103/S0025654418060043
E. L. Aero, A. N. Bulygin, and Yu. V. Pavlov, “Nonlinear model of deformation of crystalline media allowing for martensitic transformations: plane deformation,” Mech. Solids 54, 797–806 (2019). https://doi.org/10.3103/S0025654419050029
J. Frenkel and T. Kontorova, “On the theory of plastic deformation and twinning,” Acad. Sci. USSR J. Phys. 1, 137–149 (1939).
O. M. Braun and Y. S. Kivshar, The Frenkel-Kontorova Model. Concepts, Methods, and Applications (Springer, Berlin, 2004).
W. Voigt, Lehrbuch der Kristallphysik (Teubner, Leipzig, 1910).
G. Leibfried, Gittertheorie der Mechanischen und Thermissechen Eigenschaften der Kristalle. Handbuch Der Physik, Band 7, Teil 2 (Springer-Verlag, Berlin, 1955).
C. Kittel, Introduction to Solid State Physics (Wiley, New York, 1956).
N. I. Muskhelishvili, Some Basic Problems of the Mathematical Theory of Elasticity: Fundamental Equations, Plane Theory of Elasticity, Torsion, and Bending (Nauka, Moscow, 1966; Springer, 1977).
M. V. Keldysh and L. I. Sedov, “Effective solution of some boundary-value problems for harmonic functions,” Dokl. Akad. Nauk SSSR 16 (1), 7–10 (1937).
E. H. Yoffe, “The moving Griffith crack,” Phil. Mag. Ser. 7 42 (330), 739–750 (1951). https://doi.org/10.1080/14786445108561302
C. E. Inglis, “Stresses in a plate due to the presence of cracks and sharp corners,” Trans. Instn. Nav. Archit. Lond. 55, 219–230 (1913).
E. L. Aero, A. N. Bulygin, and Yu. V. Pavlov, “Solutions of the sine-Gordon equation with a variable amplitude,” Theor. Math. Phys. 184, 961–972 (2015). https://doi.org/10.1007/s11232-015-0309-8
E. L. Aero, A. N. Bulygin, and Yu. V. Pavlov, “Exact analytical solutions for nonautonomic nonlinear Klein-Fock-Gordon equation,” in Advanced Structured Materials, Vol. 87: Advances in Mechanics of Microstructured Media and Structures (Springer, Cham, 2018), pp. 21–33. https://doi.org/10.1007/978-3-319-73694-5_2
E. L. Aero, A. N. Bulygin, and Yu. V. Pavlov, “Some solutions of dynamic and static nonlinear nonautonomous Klein-Fock-Gordon equation,” in Advanced Structured Materials, Vol. 122: Nonlinear Wave Dynamics of Materials and Structures (Springer, Cham, 2020), pp. 107–120. https://doi.org/10.1007/978-3-030-38708-2_7
Author information
Authors and Affiliations
Corresponding author
Additional information
Translated by A.Borimova
About this article
Cite this article
Bulygin, A.N., Pavlov, Y.V. The Problem Solution on the Propagation of a Griffith Crack Based on the Equations of a Nonlinear Model. Mech. Solids 58, 1437–1446 (2023). https://doi.org/10.3103/S0025654422601483
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.3103/S0025654422601483