Abstract
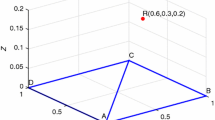
We considered the two-dimensional stress aspect of a fault from the viewpoint of differential geometry. For this analysis, we concentrated on the curvatures of the Airy stress function surface. We found the following: (i) Because the principal stresses are the principal curvatures of the stress function surface, the first and the second invariant quantities in the elasticity correspond to invariant quantities in differential geometry; specifically, the mean and Gaussian curvatures, respectively; (ii) Coulomb’s failure criterion shows that the coefficient of friction is the physical expression of the geometric energy of the stress function surface; (iii) The differential geometric expression of the Goursat formula shows that the fault (dislocation) type (strike-slip or dip-slip) corresponds to the stress function surface type (elliptic or hyperbolic). Finally, we discuss the need to use non-biharmonic stress tensor theory to describe the stress aspect of multi-faults or an earthquake source zone.
Similar content being viewed by others
References
Mandl, G. (1988), Mechanics of Tectonic Faulting: Models and Basic Concepts, Elsevier, Amsterdam.
Mogi, K. (1967), Effect of the intermediate principal stress on rock failure, J. Geophys. Res. 72,20, 5117–5131, DOI: 10.1029/JZ072i020p05117.
Nagahama, H., and R. Teisseyre (2008), Continuum theory of defects: Advanced approaches. In: R. Teisseyre, H. Nagahama, and E. Majewski (eds.), Physics of Assymetric Continuum: Extreme and Fracture Processes, Springer-Verlag, Berlin, 220–248.
Park, R.G. (1997), Foundation of Structural Geology, Chapman and Hall, New York.
Parry, R.H.G. (2004), Mohr Circles, Stress Paths and Geotechnics, Taylor and Francis, London, DOI: 10.4324/9780203428283.
Pottmann, H., P. Grohs, and N.J. Mitra (2009), Laguerre minimal surfaces, isotropic geometry and linear elasticity, Adv. Comput. Math. 31,4, 391–419, DOI: 10.1007/s10444-008-9076-5.
Teisseyre, R. (1973), Earthquake processes in a micromorphic continuum, Pure Appl. Geophys. 102,1, 15–28, DOI: 10.1007/BF00876588.
Teisseyre, R. (2009), Tutorial on new developments in the physics of rotational motions, Bull. Seismol. Soc. Am. 99,2B, 1028–1039, DOI: 10.1785/0120080089.
Teisseyre, R. (2010), Fluid theory with asymmetric molecular stresses: Difference between vorticity and spin equations, Acta Geophys. 58,6, 1056–1071, DOI: 10.2478/s11600-010-0029-2.
Teisseyre, R., and M. Górski (2009), Transport in fracture processes: Fragmentation and slip, Acta Geophys. 57,3, 583–599, DOI: 10.2478/s11600-009-0020-y.
Teisseyre, R., and M. Górski (2011), Earthquake fragmentation and slip processes: spin and shear-twist wave mosaic, Acta Geophys. 59,3, 453–469, DOI: 10.2478/s11600-011-0001-9.
Teisseyre, R., and M. Shimbo (1995), Differential geometry methods in deformation problems. In: R. Teisseyre (ed.), Theory of Earthquake Premonitory and Fracture Processes, Polish Scientific Publisher PWN, 503–544.
Twiss, R.J., and E.M. Moores (1992), Structural Geology, W.H. Freeman, New York.
Willmore, T.J. (1996), Riemannian Geometry, Oxford University Press, New York.
Yamasaki, K. (2005), Tensor analysis of dislocation-stress relationship based on the extended deformation gradient, Acta Geophys. Pol. 53,1, 1–12.
Yamasaki, K. (2009), A quantum particle motion and thermodynamics in faultsdefects field: Path integral formulation based on extended deformation gradient tensor, Acta Geophys. 57,3, 567–582, DOI: 10.2478/s11600-009-0016-7.
Yamasaki, K., and H. Nagahama (1999), Hodge duality and continuum theory of defects, J. Phys. A Math. Gen. 32,44, L475–L481, DOI: 10.1088/0305-4470/32/44/103.
Yamasaki, K., and H. Nagahama (2002), A deformed medium including a defect field and differential forms, J. Phys. A Math. Gen. 35,16, 3767–3778, DOI: 10.1088/0305-4470/35/16/315.
Yamasaki, K., and H. Nagahama (2008), Energy integral in fracture mechanics (J-integral) and Gauss-Bonnet theorem, J. Appl. Math. Mech. 88,6, 515–520, DOI: 10.1002/zamm.200700140.
Yamasaki, K., T. Yajima, and T. Iwayama (2011), Differential geometric structures of stream functions: incompressible two-dimensional flow and curvatures, J. Phys. A Math. Theor. 44,15, 155501–155521, DOI: 10.1088/1751-8113/44/15/155501.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Yamasaki, K., Yajima, T. Differential geometric approach to the stress aspect of a fault: Airy stress function surface and curvatures. Acta Geophys. 60, 4–23 (2012). https://doi.org/10.2478/s11600-011-0055-8
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.2478/s11600-011-0055-8