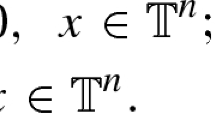Abstract
For a positive integer n and R>0, we set\(B_R^n = \left\{ {x \in \mathbb{R}^n |\left\| x \right\|_\infty< R} \right\}\). Given R>1 and n≥4 we construct a sequence of analytic perturbations (H j ) of the completely integrable Hamiltonian\(h\left( r \right) = \tfrac{1}{2}r_1^2 + ...\tfrac{1}{2}r_{n - 1}^2 + r_n \) on\(\mathbb{T}^n \times B_R^n \), with unstable orbits for which we can estimate the time of drift in the action space. These functions H j are analytic on a fixed complex neighborhood V of\(\mathbb{T}^n \times B_R^n \), and setting\(\varepsilon _j : = \left\| {h - H_j } \right\|_{C^0 (V)} \) the time of drift of these orbits is smaller than (C(1/ɛ j )1/2(n-3)) for a fixed constant c>0. Our unstable orbits stay close to a doubly resonant surface, the result is therefore almost optimal since the stability exponent for such orbits is 1/2(n−2). An analogous result for Hamiltonian diffeomorphisms is also proved. Two main ingredients are used in order to deal with the analytic setting: a version of Sternberg's conjugacy theorem in a neighborhood of a normally hyperbolic manifold in a symplectic system, for which we give a complete (and seemingly new) proof; and Easton windowing method that allow us to approximately localize the wandering orbits and estimate their speed of drift.
Similar content being viewed by others
References
V.I. Arnold: “Instability of dynamical systems with several degrees of freedom”, Dokl. Akad. Nauk SSSR, Vol. 156, (1964), pp. 9–12; Soviet Math. Dokl., Vol. 5, (1964), pp. 581–585.
A. Banyaga, R. de la Llave and C.E. Wayne: “Cohomology equations near hyperbolic points and geometric versions of Sternberg linearization theorem”, J. Geom. Anal., Vol. 6, (1996), pp. 613–649.
A. Banyaga, R. de la Llave and C.E. Wayne: “Cohomology equations and commutators of germs of contact diffeomorphisms”, Trans. AMS, Vol. 312, (1989), pp. 755–778.
G. Benettin and G. Gallavotti: “Stability of motions near resonances in quasiintegrable Hamiltonian systems”, J. Phys. Stat., Vol. 44, (1986), pp. 293–338.
G. Benettin, L. Galgani and A. Giorgilli: “A proof of Nekhoroshev's theorem for the stability times in nearly integrable Hamiltonian systems”, Celestial Mech., Vol. 37, (1985), pp. 1–25.
U. Bessi: “An approach to Arnold's diffusion through the calculus of variations”, Nonlinear Anal. TMA, Vol. 26, (1996), pp. 1115–1135.
U. Bessi: “Arnold's example with three rotators”, Nonlinearity, Vol. 10, (1997), pp. 763–781.
J. Bourgain: “Diffusion for Hamiltonian perturbations of integrable systems in high dimensions”, (2003), preprint.
J. Bourgain and V. Kaloshin: “Diffusion for Hamiltonian perturbations of integrable systems in high dimensions”, (2004), preprint.
M. Chaperon: “Géométrie différentielle et singularités de systèmes dynamiques”, Astérisque, Vol. 138–139, (1986).
M. Chaperon: “Invariant manifolds revisited”, Tr. mat. inst. Steklova, Vol. 236, (2002), Differ. Uravn. i. Din. Sist., pp. 428–446.
M. Chaperon: “Stable manifolds and the Perron-Irwin method”, Erg. Th. and Dyn. Syst., Vol. 24, (2004), pp. 1359–1394.
M. Chaperon and F. Coudray: “Invariant manifolds, conjugacies and blow-up”, Erg. Th. and Dyn. Syst., Vol. 17, (1997), pp. 783–791.
B.V. Chirikov: “A universal instability of many-dimensional oscillator systems”, Phys. Reports, Vol. 52, (1979), pp. 263–379.
R. Douady: “Stabilité ou instabilité des points fixes elliptiques”, Ann. Sc. Éc. Norm. Sup., Vol. 21, (1988), pp. 1–46.
R. Easton and R. McGehee: “Homoclinic phenomena for orbits doubly asymptotic to an invariant three-sphere”, Ind. Univ. Math. Journ., Vol. 28(2), (1979).
E. Fontich and P. Mart′ in: “Arnold diffusion in perturbations of analytic integrable Hamiltonian systems”, Discrete and Continuous Dyn. Syst., Vol. 7(1), (2001), pp. 61–84.
G. Gallavotti: “Quasi-integrable mechanical systems”, In: K. Osterwalder and R. Stora (Eds.): Phénomènes criliques, systèmes aléatoires, théories de jauge, part II (Les Houches 1984), North-Holland, Amsterdam New York, 1986, pp. 539–624.
M. Herman: Notes de travail, December, 1999, manuscript.
M.W. Hirsch, C.C. Pugh and M. Shub: Invariant Manifolds, Lecture Notes in Mathematics, Vol. 583, Springer Verlag, 1977.
S. Kuksin and J. Pöschel: “Nekhoroshev estimates for quasi-convex Hamiltonian systems”, Math. Z., Vol. 213, (1993), pp. 187–216.
P. Lochak: “Canonical perturbation theory via simultaneous approximation”, Usp. Mat. Nauk., Vol. 47 (1992), pp. 59–140; Russian Math. Surveys, Vol. 47, (1992), pp. 57–133.
P. Lochak, J.-P. Marco and D. Sauzin: “On the splitting of invariant manifolds in multi-dimensional near-integrable Hamiltonian systems” Memoirs of the Amer. Math. Soc., Vol. 163, (2003).
P. Lochak and A.I. Neishtadt: “Estimates in the theorem of N. N. Nekhorocheff for systems with a quasi-convex Hamiltonian”, Chaos, Vol. 2, (1992), pp. 495–499.
P. Lochak, A.I. Neishtadt and L. Niederman: “Stability of nearly integrable convex Hamiltonian systems over exponentially long times”, In: Proc. 1991 Euler Institute Conf. on Dynamical Systems, Birkhaüser, Boston, 1993.
J.-P. Marco: “Transition le long des chaines de tores invariants pour les systèmes hamiltoniens analytiques”, Ann. Inst. H. Poincaré, Vol. 64(2), (1996), pp. 205–252.
J.-P. Marco: “Uniform lower bounds of the splitting for analytic symplectic systems”, Ann. Inst. Fourier, submitted to.
J.-P. Marco, and D. Sauzin: “Stability and instability for Gevrey quasi-convex nearintegrable Hamiltonian systems”, Publ. Math. I.H.E.S., Vol. 96, (2003), pp. 77.
N.N. Nekhoroshev: “An exponential estimate of the time of stability of nearly integrable Hamiltonian systems”, Usp. Mal. Nauk., Vol. 32, (1977), pp. 5–66; Russian Math. Surveys, Vol. 32, (1977), pp. 1–65.
J. Pöschel: “Nekhoroshev estimates for quasi-convex Hamiltonian systems”, Math. Z., Vol. 213, (1993), pp. 187–216.
S. Sternberg: “Local contractions and a theorem of Poincaré”, Amer. J. Math., Vol. 79, (1957), pp. 809–824.
S. Sternberg: “On the structure of local homeomorphisms II”, Amer. J. Math., Vol. 80, (1958), pp. 623–631.
S. Sternberg: “On the structure of local homeomorphisms III”, Amer. J. Math., Vol. 81, (1959), pp. 578–604.
S. Sternberg: “Infinite Lie groups and the formal aspects of dynamical systems”, J. Math. Mech., Vol. 10, (1961), pp. 451–474.
D. V. Treshchev: “Evolution of slow variables in near-integrable Hamiltonian systems”, Progress in nonlinear science, Vol. 1, (2001), pp. 166–169.
Author information
Authors and Affiliations
About this article
Cite this article
Lochak, P., Marco, JP. Diffusion times and stability exponents for nearly integrable analytic systems. centr.eur.j.math. 3, 342–397 (2005). https://doi.org/10.2478/BF02475913
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.2478/BF02475913