Abstract
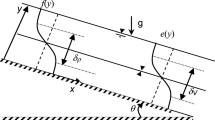
The parabolized stability equation (PSE) was derived to study the linear stability of particle-laden flow in growing Blasius boundary layer. The stability characteristics for various Stokes numbers and particle concentrations were analyzed after solving the equation numerically using the perturbation method and finite difference. The inclusion of the nonparallel terms produces a reduction in the values of the critical Reynolds number compared with the parallel flow. There is a critical value for the effect of Stokes number, and the critical Stokes number being about unit, and the most efficient instability suppression takes place when Stokes number is of order 10. But the presence of the nonparallel terms does not affect the role of the particles in gas. That is, the addition of fine particles (Stokes number is much smaller than 1) reduces the critical Reynolds number while the addition of coarse particles (Stokes number is much larger than 1) enhances it. Qualitatively the effect of nonparallel mean flow is the same as that for the case of plane parallel flows.
Similar content being viewed by others
References
Alizard, F., Robinet, J.C., 2006. Two-dimensional Temporal Modes in Nonparallel Flows. Int. Conf. on Boundary and Interior Layers, Bail, Germany, 78:387–390.
Asmolov, E.S., Manuilovich, S.V., 1998. Stability of a dusty-gas laminar boundary layer on a flat plate. J. Fluid Mech., 365:137–170. [doi:10.1017/S0022112098001256]
Barry, M.D.J., Ross, M.A.S., 1970. The flat boundary layer. Part 2. The effect of increasing thickness on stability. J. Fluid Mech., 43(4):813–818. [doi:10.1017/S0022112070002768]
Bertolotti, F.P., Herbert, T., Spalart, P.R., 1992. Linear and nonlinear stability of the Blasius boundary layer. J. Fluid Mech., 242(1):441–471. [doi:10.1017/S0022112092002453]
Bhaganagar, K., Rempfer, D., Lumley, J., 2002. Direct numerical simulation of spatial transition to turbulence using fourth order vertical velocity second order vertical vorticity formulation. J. Comput. Phys., 180(1):200–228. [doi:10.1006/jcph.2002.7088]
Dimas, A.A., Kiger, K.T., 1998. Linear instability of a particle-laden mixing layer with a dynamic dispersed phase. Phys. Fluids, 10(10):2539–2557. [doi:10.1063/1.869769]
Ehrenstein, U., Gaillaire, F., 2005. On 2D temporal modes in spatially evolving open flows: the flat-plate boundary layer. J. Fluid Mech., 536:209–218. [doi:10.1017/S0022112005005112]
Fasel, H., Konzelmann, U., 1990. Non-parallel stability of a flat-plate boundary layer using the complete Navier-Stokes equations. J. Fluid Mech., 221(1):311–347. [doi:10.1017/S0022112090003585]
Gaster, M., 1974. On the effects of boundary-layer growth on flow stability. J. Fluid Mech., 66(3):465–480. [doi:10.1017/S0022112074000310]
Govindarajan, R., Narasimha, R., 1999. Low-order parabolic theory for 2D boundary-layer stability. Phys. Fluids, 11(6):1449–1458. [doi:10.1063/1.870008]
Govindarajan, R., Narasimha, R., 2005. Accurate estimate of disturbance amplitude variation from solution of minimal composite stability theory. Theoretical and Computational Fluid Dynamics, 19(4):229–235. [doi:10.1007/s00162-005-0162-8]
Grosch, C.E., Orszag, S.A., 1977. Numerical solution of problems in unbounded regions: coordinate transforms. J. Comput. Phys., 25(3):273–296 [doi:10.1016/0021-9991(77)90102-4]
Isakov, E.B., Rudnyak, Y.V., 1995. Stability of rarefied dusty gas and suspension flows in plane channel. Fluid Dynamics, 30(5):708–712. [doi:10.1007/BF02079390]
Jordinson, R., 1970. The flat plate boundary layer. Part 1. Numerical integration of the Orr-Sommerfeld equation. J. Fluid Mech., 43(4):801–811. [doi:10.1017/S0022112070002756]
Kurtz, E.F., Crandall, S.H., 1962. Computer-aided analysis of hydrodynamic stability. J. Math. Phys., 41:264–279.
Lin, R.S., Malik, M.R., 1997. On the stability of attachment-line boundary layers. J. Fluid Mech., 333:125–137. [doi:10.1017/S0022112096004260]
Michael, D.H., 1965. Kelvin-Helmholtz instability of a dusty gas. Proc. Camb. Phil. Soc., 61:569–571.
Rempfer, D., 2003. Low dimensional modeling and numerical simulation of transition in simple shear flows. Annu. Rev. Fluid Mech., 35(1):229–265. [doi:10.1146/annurev.fluid.35.030602.113908]
Saffman, P.G., 1962. On the stability of laminar flow of dusty gas. J. Fluid Mech., 13(1):120–128. [doi:10.1017/S0022112062000555]
Schlichting, H., 1954. Boundary Layer Theory. McGraw Hill Book Company, Inc., New York, p.431.
Sproull, W.T., 1961. Viscosity of dusty gases. Nature, 190(4780):976–978. [doi:10.1038/190976a0]
Squires, K.D., Eaton, J.K., 1990. Particle response and turbulence modification in isotropic turbulence. Phys. Fluids A, 2(7):1191–1203. [doi:10.1063/1.857620]
Theofilis, V., 2003. Advances in global linear instability of nonparallel and three-dimensional flows. Prog. in Aerospace Sciences, 39(4):249–315. [doi:10.1016/S03760421(02)00030-1]
Tong, X.L., Wang, L.P., 1999. Two-way coupled particleladen mixing layer. Part 1: linear instability. Int. J. Multiphase Flow, 25(4):575–598. [doi:10.1016/S0301-9322(98)00059-7]
Torobin, L.B., Gauvin, W.H., 1961. Fundamental aspects of solids-gas flow, Part 6. Multi-particle behavior in turbulent fluids. Can. J. Chem. Engng., 39:113–120.
Wan, Z.H., Lin, J.Z., You, Z.J., 2005. Non-axisymmetric instability in the Taylor-Couette flow of fiber suspension. Journal of Zhejiang University SCIENCE, 6A(Suppl. I):1–7. [doi:10.1631/jzus.2005.AS0001]
Zebib, A., 1984. A Chebyshev method for the solution of boundary value problems. J. Comput. Phys., 53(3):443–455. [doi:10.1016/0021-9991(84)90070-6]
Author information
Authors and Affiliations
Additional information
Project supported by the National Natural Science Foundation of China (No. 10372090) and the Doctoral Program of Higher Education of China (No. 20030335001)
Rights and permissions
About this article
Cite this article
Xie, Ml., Lin, Jz. & Xing, Ft. On the hydrodynamic stability of a particle-laden flow in growing flat plate boundary layer. J. Zhejiang Univ. - Sci. A 8, 275–284 (2007). https://doi.org/10.1631/jzus.2007.A0275
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1631/jzus.2007.A0275