Skip to main content
SpringerLink
Log in

Mechanical and failure behaviors of lattice-plate hybrid structures

  • Prospective Article
  • Published:
MRS Communications Aims and scope Submit manuscript

Abstract

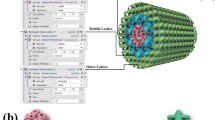
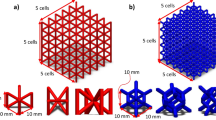
The authors design six alumina hybrid structures consisting of stretching-dominated plates and different space-filling lattices comprised of hollow tubes and perform finite element simulations to study mechanical and failure behaviors of such hybrid structures. The authors investigate the effects of three geometrical parameters on the stiffness and failure of these hybrid structures and further compare their advantages and disadvantages. The authors find that the failure modes of these hybrid structures can be tuned by altering cell unit type and geometrical parameters. Among these hybrid structures, the ones with effective support from the lattice unit cells in the stretching direction exhibit better specific stiffness and strength. By varying the lattice and plate thickness, the authors find that the relations between stiffness/failure strength and density follow a power law. When intrinsic material failure occurs, the power law exponent is 1; when buckling failure arises, the power law exponent is 3. However, by varying tube thickness, their relations follow unusual power relations with the exponent changing from nearly 0 to nearly infinity. In addition, the hybrid structures also exhibit defect insensitivity. This study shows that such hybrid structures are able to greatly expand the design space of architectured cellular materials for engineering applications.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Figure 1
Figure 2
Figure 3
Figure 4
Figure 5
Figure 6
Figure 7
Figure 8
Figure 9
Figure 10

Similar content being viewed by others

References

  1. T.A. Schaedler, A.J. Jacobsen, and W.B. Carter: Toward lighter, stiffer materials. Science 341, 1181–1182 (2013).

    Article  CAS  Google Scholar 

  2. L.J. Gibson and M.F. Ashby: Cellular Solids: Structure and Properties (Cambridge University Press, Cambridge, UK, 1997).

    Book  Google Scholar 

  3. A.G. Evans, J.W. Hutchinson, and M.F. Ashby: Multifunctionality of cellular metal systems. Prog. Mater. Sci. 43, 171–221 (1999).

    Article  Google Scholar 

  4. H.B.G. Wadley, N.A. Fleck, and A.G. Evans: Fabrication and structural performance of periodic cellular metal sandwich structures. Compos. Sci. Technol. 63, 2331–2343 (2003).

    Article  CAS  Google Scholar 

  5. V.S. Deshpande, N.A. Fleck, and M.F. Ashby: Effective properties of the octet truss lattice material. J. Mech. Phys. Solids 49, 1747–1769 (2001).

    Article  CAS  Google Scholar 

  6. H.N.G. Wadley: Multifunctional periodic cellular metals. Philos. Trans. R. Soc. A 364, 31–68 (2006).

    Article  CAS  Google Scholar 

  7. V.S. Deshpande, M.F. Ashby, and N.A. Fleck: Foam topology: bending versus stretching dominated architectures. Acta Mater. 49, 1035–1040 (2001).

    Article  CAS  Google Scholar 

  8. T.A. Schaedler, A.J. Jacobsen, A. Torrents, A.E. Sorensen, J. Lian, J.R. Greer, L. Valdevit, and W.B. Carter: Ultralight metallic microlattices. Science 334, 962–965 (2011).

    Article  CAS  Google Scholar 

  9. J. Bauer, S. Hengsbach, I. Tesari, R. Schwaiger, and O. Kraft: High-strength cellular ceramic composites with 3D microarchitecture. Proc. Natl. Acad. Sci. USA 111, 2453–2458 (2014).

    Article  CAS  Google Scholar 

  10. Z.C. Eckel, C. Zhou, J.H. Martin, A.J. Jacobsen, W.B. Carter, and T.A. Schaedler: Additive manufacturing of polymer derived ceramics. Science 351, 58–62 (2016).

    Article  CAS  Google Scholar 

  11. T. George, V.S. Deshpande, and H.N.G. Wadley: Mechanical response of carbon fiber composite sandwich panels with pyramidal truss cores. Composites Part A 47, 31–40 (2013).

    Article  CAS  Google Scholar 

  12. K.C. Cheung, and N. Gershenfeld: Reversibly assembled cellular composite materials. Science 341, 1219–2121 (2013).

    Article  CAS  Google Scholar 

  13. X. Zheng, H. Lee, T.H. Weisgraber, M. Shusteff, J. DeOtte, E.B. Duoss, J.D. Kuntz, M.M. Biener, Q. Ge, J.A. Jackson, S.O. Kucheyev, N.X. Fang, and C.M. Spadaccini: Ultralight, ultrastiff mechanical metamaterials. Science 344, 1373–1377 (2014).

    Article  CAS  Google Scholar 

  14. R.B. Dinwiddie, R.R. Dehoff, P.D. Lloyd, L.E. Lowe, and J.B. Ulrich: Thermographic in-situ process monitoring of the electron-beam melting technology used in additive manufacturing. Proc. SPIE 8705, 87050K (2013).

    Article  Google Scholar 

  15. N. Wicks, and J.W. Hutchinson: Optimal truss plates. Int. J. Solids Struct. 38, 5165–5183 (2001).

    Article  Google Scholar 

  16. L. Valdevit, J.W. Hutchinson, and A.G. Evans: Structurally optimized sandwich panels with prismatic cores. Int. J. Solids Struct. 41, 5105–5124 (2004).

    Article  Google Scholar 

  17. L. Valdevit, A. Pantano, H.A. Stone, and A.G. Evans: Optimal active cooling performance of metallic sandwich panels with prismatic cores. Int. J. Heat Mass Transf. 49, 3819–3830 (2006).

    Article  Google Scholar 

  18. M.P. Bendsoe, and O. Sigmund: Topology Optimization (Springer, Berlin, 2002).

    Google Scholar 

  19. P.W. Christensen, and A. Klabering: An Introduction to Structural Optimization (Springer, Berlin, 2008).

    Google Scholar 

  20. Altair Engineering Inc. Altair OptiStruct. http://www.altairhyperworks.com/Product (2013).

  21. Autodesk Inc. Autodesk Within. http://www.withinlab.com/software/new_index.php (2015).

  22. Z.G. Liu, P. Liu, W. Huang, W.H. Wong, A.L. Commillus, and Y.W. Zhang: A nanolattice-plate hybrid structure to achieve a nearly linear relation between stiffness/strength and density. Mater. Design 160, 496–502 (2018).

    Article  Google Scholar 

  23. N.A. Fleck, V.S. Deshpande, and M.F. Ashby: Micro-architectured materials: past, present and future. Proc. R. Soc. A 466, 2495–2516 (2010).

    Article  CAS  Google Scholar 

  24. O. Rehme: Cellular design for laser freeform fabrication, Ph.D. Thesis, Hamburg-Harburg, Technical University, 2009.

    Google Scholar 

  25. E.W. Andrews, G. Gioux, P.R. Onck, and L.J. Gibson: Size effects in ductile cellular solids. Part II: experimental results. Int. J. Mech. Sci. 43, 701–713 (2001).

    Article  Google Scholar 

  26. O. Kesler and L.J. Gibson: Size effects in metallic foam core sandwich beams. Mater. Sci. Eng., A 326, 228–234 (2002).

    Article  Google Scholar 

  27. G. Dai and W. Zhang: Size effects of effective Young’s modulus for periodic cellular materials. Sci. China, Ser. G: Phys., Mech. Astron. 52, 1262–1270 (2009).

    Article  Google Scholar 

  28. P.R. Onck, E.W. Andrews, and L.J. Gibson: Size effects in ductile cellular solids. Part I: modeling. Int. J. Mech. Sci. 43, 681–699 (2001).

    Article  Google Scholar 

  29. C. Tekoglu and P.R. Onck: Size effects in the mechanical behavior of cellular materials. J. Mater. Sci. 40, 5911–5917 (2005).

    Article  CAS  Google Scholar 

  30. ABAQUS Version 6.14. User’s Manual Version 6.14 (Providence, RI, USA, 2014).

    Google Scholar 

  31. L.J. Gibson: The mechanical behavior of cancellous bone. J. Biomech. 18, 317–328 (1985).

    Article  CAS  Google Scholar 

  32. L.J. Gibson and M.F. Ashby: The mechanics of two-dimensional cellular materials. Proc. R. Soc. A 382, 25–42 (1982).

    Google Scholar 

  33. L.J. Gibson and M.F. Ashby: The mechanics of three-dimensional cellular materials. Proc. R. Soc. A 382, 43–59 (1982).

    CAS  Google Scholar 

  34. L.J. Gibson, M.F. Ashby, and K.E. Easterling: Structure and mechanics of the iris leaf. J. Mater. Sci. 23, 3041–3048 (1988).

    Article  Google Scholar 

  35. L.J. Gibson, M.F. Ashby, G.N. Karam, U. Wegst, and H.R. Shercliff: The mechanical properties of natural materials II: microstructures for mechanical efficiency. Proc. R. Soc. A 450, 141–162 (1995).

    Google Scholar 

  36. L.J. Gibson: Biomechanics of cellular solids. J. Biomech. 38, 377–399 (2005).

    Article  Google Scholar 

  37. L.C. Montemayor, W.H. Wong, Y.W. Zhang, and J.R. Greer: Insensitivity to flaws leads to damage tolerance in brittle architected meta-materials. Sci. Rep. 6, 20570 (2016).

    Article  CAS  Google Scholar 

  38. A.J. Mateos, W. Huang, Y.W. Zhang, and J.R. Greer: Discrete-continuum duality of architected materials: failure, flaws, and fracture. Adv. Funct. Mater. 29, 1806772 (2019).

    Google Scholar 

  39. A.M. Abazari, S.M. Safavi, G. Rezazadeh, and L.G. Villanueva: Modelling the size effects on the mechanical properties of micro/nano structures. Sensors 15, 28543–28562 (2015).

    Article  Google Scholar 

  40. J.R. Greer, and J.T.M. Hosson: Plasticity in small-sized metallic systems: intrinsic versus extrinsic size effect. Prog. Mater. Sci. 56, 654–724 (2011).

    Article  CAS  Google Scholar 

Download references

Acknowledgments

The support of this work by the Institute of High Performance Computing, A*STAR and use of computing resources at the A*STAR Computational Resource Centre and National Supercomputer Centre, Singapore, are gratefully acknowledged.

Author information

Authors and Affiliations

  1. Institute of High Performance Computing, A*STAR, Singapore, 138632, Singapore

    Zhigang Liu & Ping Liu

  2. School of Aeronautics, Northwestern Polytechnical University, Xi’an, Shaanxi, 710072, People’s Republic of China

    Wei Huang

  3. Institute of High Performance Computing, A*STAR, Singapore, 138632, Singapore

    Wei Hin Wong, Athanasius Louis Commillus & Yong-Wei Zhang

Authors
  1. Zhigang Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ping Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Wei Huang
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Wei Hin Wong
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Athanasius Louis Commillus
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Yong-Wei Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Ping Liu.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Liu, Z., Liu, P., Huang, W. et al. Mechanical and failure behaviors of lattice-plate hybrid structures. MRS Communications 10, 42–54 (2020). https://doi.org/10.1557/mrc.2019.153

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1557/mrc.2019.153

Search

Navigation