Soft topological soft groups

Abstract

Purpose

The purpose of this paper is to introduce several notions, such as soft topological soft groups, soft topological soft normal subgroups, and soft topological soft factor groups, and to study their properties.

Methods

We have adopted the analytical method.

Results

We have studied properties of soft topological soft groups, soft subgroups, soft normal subgroups, soft factor groups, and soft homomorphisms.

Conclusions

The fundamental homomorphism theorem is extended in a soft topological soft group setting.

Introduction

In 1999, Molodtsov[1] proposed a new approach, viz. soft set theory for modeling vagueness and uncertainties inherent in the problems of physical science, biological science, engineering, economics, social science, medical science, etc. After that, in 2001 to 2003, Maji et al.[2, 3] worked on some mathematical aspects of soft sets and fuzzy soft sets. On the other hand, Biswas and Nanda[4], and Rosenfeld[5] worked on rough groups and fuzzy groups, respectively. In 2007, Aktas and Cagman[6] introduced a basic version of soft group theory which we further extended to fuzzy soft group[7] in 2011. Recently, in 2011, Shabir and Naz[8] introduced a notion of soft topological spaces. As a continuation of this, it is natural to investigate the behavior of topological structure or a combination of algebraic and topological structures in soft set theoretic form. In view of this and also considering the importance of topological group structure in developing Haar measure and Haar integral, we have introduced in this paper a notion of soft topological soft groups. In this connection, it is worth mentioning that in a fuzzy setting, some significant works have been done on fuzzy topological group structure by Foster[9], and Liang and Hai[10] and, in a soft setting, we have worked on soft topological groups[11]. In this paper, our aim is to introduce a notion of soft topological soft groups and its subsystems and morphisms, and to study their properties.

Preliminary

Following the works of Molodtsov[1], Maji et al.[2], and Aktas and Cagman[6], some definitions and preliminary results are presented in this section in our form. Unless otherwise stated, U will be assumed to be an initial universal set and A will be taken to be a set of parameters. Let P(U) denote the power set of U, and S(U A) denote the set of all soft sets over U. In particular, if U is a group, then P(U) will denote the set of all subgroups of U.

Soft sets

Definition 1

A pair (F,A), where F is a mapping from A to P(U), is called a soft set over U.

Let (F₁,A) and (F₂,A) be two soft sets over a common universe U, then (F₁,A) is said to be a soft subset of (F₂,A) if F₁(x) ⊆ F₂(x), for all x ∈ A. This relation is denoted by$(F_1,A)\tilde{\subseteq }(F_2,A).(F_1,A)$ is said to be soft equal to (F₂,A) if F₁(x) = F₂(x), for all x ∈ A. It is denoted by (F₁,A) = (F₂,A).

The complement of a soft set (F,A) is defined as (F,A)^c= (F^c,A), where F^c(x) = (F(x))^c= U−F(x), for all x ∈ A.

A soft set (F,A) over U is said to be a null soft set (an absolute soft set) if F(x) = ϕ (F(x) = U), for all x ∈ A. This is denoted by$\tilde{\Phi }\left(\tilde{A}\right)$.

Definition 2

Let {(F _i ,A);i ∈ I} be a nonempty family of soft sets over a common universe U, and then the following are defined:

• (a) Their intersection, denoted by ${\tilde{\cap }}_{i\in I},$ is defined by ${\tilde{\cap }}_{i\in I}(F_i,A)=({\tilde{\cap }}_{i\in I}{F}_i,A),$ where $\left({\tilde{\cap }}_{i\in I}{F}_i\right)\left(x\right)={\cap }_{i\in I}\left(F_i\right(x\left)\right),\mathit{\text{for}}\mathit{\text{all}}x\in A.$

• (b) Their union, denoted by ${\tilde{\cup }}_{i\in I},$ is defined by ${\tilde{\cup }}_{i\in I}(F_i,A)=({\tilde{\cup }}_{i\in I}{F}_i,A),$ where $\left({\tilde{\cup }}_{i\in I}{F}_i\right)\left(x\right)={\cup }_{i\in I}\left(F_i\right(x\left)\right),\mathit{\text{for}}\mathit{\text{all}}x\in A.$

Definition 3

Let X and Y be two nonempty sets and f : X → Y be a mapping, and then the following are defined:

• (i) The image of a soft set (F,A) ∈ S(X,A) under the mapping f is defined by f(F,A) = (f(F),A), where [f(F)](x) = f[F(x)], for all x ∈ A.

• (ii) The inverse image of a soft set (G,A) ∈ S(Y,A) under the mapping f is defined by f ⁻¹(G,A) = (f ⁻¹(G),A), where [f ⁻¹(G)](x) = f ⁻¹[G(x)], for all x ∈ A.

Proposition 1

Let X and Y be two nonempty sets and f : X → Y be a mapping. If (F₁,A), (F₂,A) ∈ S(X,A), then

(i)$(F_1,A)\tilde{\subset }(F_2,A)\Rightarrow f\left[\right(F_1,A\left)\right]\tilde{\subset }f\left[\right(F_2,A\left)\right]$.

(ii)$f\left[\right(F_1,A\left)\tilde{\cup }\right(F_2,A\left)\right]=f\left[\right(F_1,A\left)\right]\tilde{\cup }f\left[\right(F_2,A\left)\right]$.

(iii)$f\left[\right(F_1,A\left)\tilde{\cap }\right(F_2,A\left)\right]\tilde{\subset }f\left[\right(F_1,A\left)\right]\tilde{\cap }f\left[\right(F_2,A\left)\right]$.

(iv)$f\left[\right(F_1,A\left)\tilde{\cap }\right(F_2,A\left)\right]=f\left[\right(F_1,A\left)\right]\tilde{\cap }f\left[\right(F_2,A\left)\right]$, if f is injective.

Proof

We give the proof for (ii). Proofs of other results are similar.

• (ii)
  $$\left[f\right(F_1\tilde{\cup }{F}_2\left)\right]\left(x\right)=f\left[F_1\right(x)\cup F_2(x\left)\right]=f\left[F_1\right(x\left)\right]\cup f\left[F_2\right(x\left)\right]$$

  (as F ₁(x) and F ₂(x) are ordinary sets) $=\left[f\right(F_1\left)\right]\left(x\right)\cup \left[f\right(F_2\left)\right(x\left)\right]=\left[f\right(F_1\left)\tilde{\cup }f\right(F_2\left)\right]\left(x\right),\mathit{\text{for}}\mathit{\text{all}}x\in A.$Hence, $f\left[\right(F_1,A\left)\tilde{\cup }\right(F_2,A\left)\right]=f\left[\right(F_1,A\left)\right]\tilde{\cup }f\left[\right(F_2,A\left)\right]$. □

Proposition 2

Let X and Y be two nonempty sets and f : X → Y be a onto mapping. If (G₁,A), (G₂,A) ∈ S(Y,A), then

(i)$(G_1,A)\tilde{\subset }(G_2,A)\Rightarrow f^{-1}\left[\right(G_1,A\left)\right]\tilde{\subset }{f}^{-1}\left[\right(G_2,A\left)\right]$.

(ii)$f^{-1}\left[\right(G_1,A\left)\tilde{\cup }\right(G_2,A\left)\right]=f^{-1}\left[\right(G_1,A\left)\right]\tilde{\cup }{f}^{-1}\left[\right(G_2,A\left)\right]$.

(iii)$f^{-1}\left[\right(G_1,A\left)\tilde{\cap }\right(G_2,A\left)\right]=f^{-1}\left[\right(G_1,A\left)\right]\tilde{\cap }{f}^{-1}\left[\right(G_2,A\left)\right]$.

Proof

We give the proof for (iii). Proofs of other results are similar.

• (iii)
  $$\left[f^{-1}\right(F_1\tilde{\cap }{F}_2\left)\right]\left(x\right)$$

= f⁻¹ [F₁ (x) ∩ F₂ (x)]

= f⁻¹ [F₁ (x)] ∩ f⁻¹ [F₂ (x)] (as F₁ (x) and F₂ (x) are ordinary sets)

= [f⁻¹ (F₁)](x) ∩ [f⁻¹ (F₂)(x)]

$$=\left[f^{-1}\right(F_1\left)\tilde{\cap }{f}^{-1}\right(F_2\left)\right]\left(x\right),\mathit{\text{for}}\mathit{\text{all}}x\in A$$

.

Hence,$f^{-1}\left[\right(F_1,A\left)\tilde{\cap }\right(F_2,A\left)\right]=f^{-1}\left[\right(F_1,A\left)\right]\tilde{\cap }{f}^{-1}\left[\right(F_2,A\left)\right]$. □

Proposition 3

Let X and Y be two nonempty sets and f : X → Y be a mapping. If (G,A) ∈ S(Y,A), then

(i) $f\left[f^{-1}\right(G,A\left)\right]\tilde{\subset }(G,A).$

(ii) f[f⁻¹(G,A)] = (G,A), if f is surjective.

Proof

We give the proof for (ii). Proof of part (i) is similar.

• (ii) f[f ⁻¹ (G)](x)

= f[f⁻¹ (G(x))]

= G(x) if f is surjective (as G(x) is a ordinary set), for all x ∈ A.

Hence, f[f⁻¹ (G,A)] = (G,A), if f is surjective. □

Proposition 4

Let X and Y be two nonempty sets and f:X → Y be a mapping. If (F,A) ∈ S(X,A), then

(i)$(G,A)\tilde{\subset }{f}^{-1}\left[f\right(G,A\left)\right]$.

(ii) f⁻¹ [f(G,A)] = (G,A), if f is injective.

Proof

We give the proof for (ii). Proof of part (i) is similar.

• (ii) f ⁻¹ [f(G)](x)

= f⁻¹ [f(G(x))]

= G(x) if f is injective (as G(x) is a ordinary set), for all x ∈ A.

Hence, f⁻¹ [f(G,A)] = (G,A), if f is injective. □

Soft groups

Let G, G₁, G₂, and K be groups and A be any nonempty set.

Definition 4

Let (F,A) be a soft set over G, and then (F,A) is said to be a soft group over G if F(x) is a subgroup of G, for all x ∈ A, i.e., F(x) ≤ G, for all x ∈ A[6].

Theorem 1

Let {(F _i ,A); i ∈ I} be a nonempty family of soft groups of G where I is an index set, and then${\bigcap ^{~}}_{i\in I}(F_i,A)$ is a soft group over G[6].

Definition 5

Let (F,A) be a soft group over G, and then[6]

• (i) (F,A) is said to be an identity soft group over G if F(x) = {e}, for all x ∈ A, where e is the identity element of G.

• (ii) (F,A) is said to be an absolute soft group if F(x) = G, for all x ∈ A.

Theorem 2

Let (F,A) be a soft group over G and f : G → K be a group homomorphism[6]:

(i) If F(x) = Kerf, the kernel of f, for all x ∈ A, then (f(F),A) is an identity soft group over K.

(ii) If (F,A) be an absolute soft group over G and f be onto, then (f(F),A) is an absolute soft group over K.

Definition 6

Let (F₁,A) and (F₂,A) be two soft groups over G, and then (F₁,A) is said to be a soft subgroup (soft normal subgroup) of (F₂,A), denoted by (F₁,A)$\tilde{\le }$(F₂,A) ((F₂,A)$\widetilde{⊲}$(F₁,A)) if F₁ (x) ≤ F₂ (x) (F₁ (x) ⊲ F₂ (x)), for all x ∈ A[6].

Theorem 3

Let (F,A) be a soft group over G and {(H _i A); i ∈ I} is a nonempty family of soft subgroups (soft normal subgroups) of (F A), where I is an index set, and then${\bigcap ^{~}}_{i\in I}(H_i,A)$ is a soft subgroup (normal soft subgroup) of (F,A)[6].

Theorem 4

Let (F₁,A) and (F₂,A) be two soft groups over G and (F₁,A) be a soft subgroup of (F₂,A). If f : G → K be a homomorphism, then (f(F₁),A) and (f(F₂),A) are both soft subgroups over K, and (f(F₁),A) is a soft subgroup of (f(F₂),A)[6].

Definition 7

Let (F,A) be a soft group over G₁ and f : G₁ → G₂ be a homomorphism. Define the function K _f : A → P(G₁) such that$K_f\left(x\right)={\left[\mathit{\text{Ker}}\right(f\left)\right]}_{F\left(x\right)}=\left(\mathit{\text{Kerf}}\right)\cap F\left(x\right)=\{g\in F(x);f(g)=e_{G_2}\}$, for all x ∈ A. Therefore, (K _f ,A) is a soft group over G₁. It is clear that (K _f ,A) is a normal soft subgroup of (F,A)[7].

Definition 8

Let$\mathcal{G}=\left\{\right(G_i):i\in \Delta \}$ be a nonempty collection of groups and A be a nonempty set. Let$F:A\to \mathcal{G}$ be a mapping, and then (F A) will be called a generalized soft group. In fact, the direct product π_i∈ΔG _i is a group, and as each$G_i(\in \mathcal{G})$ is embedded in π_i∈ΔG _i , the generalized soft group can be interpreted as a soft group$(\widehat{F},A)$ over π_i∈ΔG _i such that$\widehat{F}\left(a\right)=\widetilde{F\left(a\right)}$, where$\widetilde{F\left(a\right)}$ is the embedded subgroup of π_i∈ΔG _i corresponding to the group F(a)[7].

Definition 9

Let (N,A) and (F,A) be two soft groups over G such that (N,A) is a normal soft subgroup of (F,A). Define a mapping$\frac{F}{N}$ over A by$\frac{F}{N}\left(x\right)=$ the factor group$\frac{F\left(x\right)}{N\left(x\right)},\mathit{\text{for}}\mathit{\text{all}}x\in A$, then the factor group$\frac{F\left(x\right)}{N\left(x\right)}$ is a group, for each x ∈ A. Thus, for each x ∈ A, we get a factor group$\frac{F\left(x\right)}{N\left(x\right)}$, and thus, it induces a generalized soft group which we call soft factor group and denote it by$(\frac{F}{N},A)$[7].

Definition 10

Let (F₁,A) and (F₂,A) be two soft groups over G₁ and G₂, respectively, and then (F₁,A) is said to be soft homomorphic to (F₂,A), denoted by (F₁,A) ∼ (F₂,A), if for each x ∈ A, there exists a homomorphism α _x : F₁(x) → F₂(x) such that α _x (F₁(x)) = F₂(x)[7].

In this definition, if α _x : F₁ (x) → F₂ (x) is an isomorphism for each x ∈ A, then (F₁,A) is said to be soft isomorphic to (F₂,A). This is denoted by (F₁,A)≃(F₂,A).

Definition 11

Let (F₁,A) and (F₂,A) be two soft groups over G₁ and G₂, respectively. Also, let (F₁,A) be soft homomorphic to (F₂,A)[7]. Define α F₁ : A → P (G₂) by (α F₁)(x) = (α _x (F₁(x))), for all x ∈ A and α⁻¹F₂ : A → P(G₁) by$\left({\alpha }^{-1}{F}_2\right)\left(x\right)=\left({\alpha }_x^{-1}\right(F_2\left(x\right)),\mathit{\text{for}}\mathit{\text{all}}x\in A$ where α _x be the corresponding homomorphism of the Definition 10.

Theorem 5

Let (F₁,A) and (F₂,A) be two soft groups over G₁ and G₂, respectively. Also, let (F₁,A) be soft homomorphic to (F₂,A)[7]:

(i) If α _x : F₁(x) → F₂(x) be the corresponding homomorphism for each x ∈ A, then (α F₁,A) and (α⁻¹F₂,A) are soft groups over G₂ and G₁, respectively.

(ii) If (F₃,A) be a soft normal subgroup of (F₁,A), then (α F₃,A) is a soft normal subgroup of (α F₁,A).

(iii) If (F₄,A) be a soft normal subgroup of (F₂,A), then (α⁻¹F₄,A) is a soft normal subgroup of (α⁻¹F₂,A).

Proof

• (i) Since α _x : F ₍ x) → F ₂(x) is a homomorphism, it follows that (α F ₁)(x) = (α _x (F ₁(x))) is a subgroup of F ₂(x) and hence subgroup of G ₂ for each x ∈ A. Therefore, (α F ₁,A) is a soft group over G ₂. Again, $\left({\alpha }^{-1}{F}_2\right)\left(x\right)=\left({\alpha }_x^{-1}\right(F_2\left(x\right))$ is a subgroup of F ₁(x) and hence subgroup of G ₁, for each x ∈ A, where $\left({\alpha }_x^{-1}\right(F_2\left(x\right))$ is the inverse image of F ₂(x) under the mapping α _x . Thus, (α ⁻¹ F ₂,A) is a soft group G ₁.

• (ii) Since α _x is a homomorphism from F ₁(x) onto F ₂(x), it follows that α _x (F ₁(x)) and α _x (F ₃(x)) are subgroups of F ₂(x), for all x ∈ A. Again, F ₃(x) is a normal subgroup of F ₁(x), and α _x is a homomorphism; α _x (F ₃(x)) is a subgroup of α _x (F ₁(x)). Let y ∈ α _x (F ₁(x)), then their exists z ∈ F ₁(x) such that y = α _x (z). Now, y α _x (F ₃(x)) = α _x (z) α _x (F ₃(x)) = α _x (z F ₃(x)) = α _x (F ₃(x)z) = α _x (F ₃(x)) α _x (z) = α _x (F ₃(x)) y, for all x ∈ A. Thus, α _x (F ₃(x)) is normal subgroup of α _x (F ₁(x)),for all x ∈ A. Therefore, (α,F ₃,A) is soft normal subgroup of (α,F ₁,A).

• (iii) Proof is similar to that of part (ii).

□

Theorem 6

Let (N,A) be a soft normal subgroup of (F,A), and then for each x ∈ A, the canonical mapping ϕ _x : F(x) → F(x)/N(x), given by ϕ _x (ξ) = ξ N(x), ξ ∈ F(x), is an onto homomorphism[7].

Definition 12

Let (F₁,A) and (F₂,A) be two soft groups over G₁ and G₂, respectively, such that (F₁,A) is soft homomorphic to (F₂,A). Also, let for each x ∈ A, α _x : F₁(x) → F₂(x) be the corresponding homomorphism and K _x be the kernel of α _x . Define a mapping K : A → P(G₁), such that K(x) = K _x . Clearly, (K,A) is a soft set over G₁ and is called soft kernel corresponding to {α _x ;x ∈ A}. Also, (K,A) is a soft normal subgroup of (F₁,A)[7].

Theorem 7

Let (F₁,A) and (F₂,A) be two soft groups over G₁ and G₂, respectively, such that (F₁,A) is soft homomorphic to (F₂,A). Also, let that for each x ∈ A, α _x : F₁(x) → F₂(x) be the corresponding homomorphism and (K A) be the soft kernel corresponding to the family of homomorphisms {α _x , x ∈ A}, then the soft group$(\frac{F_1}{K},A)$ is isomorphic to the soft group (F₂,A)[7].

Topological groups

In this section, the well-known definition of topological groups is taken, and some established results on topological groups are cited, which will be used in this paper.

Definition 13

Let G be a group and τ be a topology on G, and then (G,τ) is called topological group if the onto mappings are as follows[12]:

• (i) f : (G,τ) × (G,τ)→(G,τ) defined by f(x,y) = xy, for all x,y ∈ G and

• (ii) g :(G,τ) → (G,τ) defined by g(x) = x ⁻¹, for all x ∈ G are continuous.

Theorem 8

Let (G,τ) be a topological group[12]:

(a) If H be an algebraic subgroup of G, then (H,τ _H ) is a topological group, where τ _H is the relativized topology on H induced from τ.

(b) Let H be a normal subgroup of G and ϕ :G → G/H be the canonical mapping. If τ^′= {A ⊂ G/H:ϕ⁻¹(A) ∈ τ}, then τ^′is a quotient topology and (G/H,τ^′) is a topological group.

(c) If H be a normal subgroup of G, then the canonical mapping$\varphi :(G,\tau )\to (\frac{G}{H},{\tau }^{\prime })$ defined by ϕ(x) = xH, x ∈ G is an open homomorphism.

Theorem 9

Let α be an algebraic homomorphism from a topological group (G,τ) into a topological group (G₁,τ₁). Let H be the kernel of α and$\varphi :(G,\tau )\to (\frac{G}{H},{\tau }^{\prime })$ be the canonical mapping. Let α = α₀ϕ for some${\alpha }_0:(\frac{G}{H},{\tau }^{\prime })\to (G_1,{\tau }_1)$, and then α : (G τ) → (G₁,τ₁) is continuous (open) if${\alpha }_0:(\frac{G}{H},{\tau }^{\prime })\to (G_1,{\tau }_1)$ is continuous (open)[12].

Theorem 10

Let (G _α ,τ _α ) be a topological group, for all α ∈ A, and then G = π_α∈AG _α , endowed with the product topology π_α∈Aτ _α , is a topological group[12].

Soft topological spaces

In this section, some properties of soft topology are studied using the definition of soft topology by Shabir and Naz[8]. Unless otherwise stated, X is an initial universal set. A is the nonempty set of parameters, and S(X A) denotes the collection of all soft sets over X under the parameter set A.

Definition 14

Let τ be the collection of soft sets over X, and then τ is said to be a soft topology on X if the following conditions are met[8]:

• (i)
  $$(\tilde{\varphi },A),(\tilde{X},A)\in \tau$$

  where $\tilde{\varphi }\left(\alpha \right)=\varphi$ and $\tilde{X}\left(\alpha \right)=X,\mathit{\text{for}}\mathit{\text{all}}\alpha \in A.$

• (ii) The intersection of any two soft sets in τ belongs to τ.

• (iii) The union of any number of soft sets in τ belongs to τ.

The triplet (X,A,τ) is called a soft topological space over X.

Proposition 5

Let (X,A,τ) be a soft topological space over X, and then the collection τ^α= {F(α): (F,A) ∈ τ} for each α ∈ A defines a topology on X[8].

Proposition 6

Let (X,A,τ₁) and (X,A,τ₂) be two soft topological spaces over X, and then$(X,A,{\tau }_1\tilde{\cap }{\tau }_2)$ where${\tau }_1\tilde{\cap }{\tau }_2=\left\{\right(F,A):(F,A)\in {\tau }_1\text{\&}(F,A)\in {\tau }_2\}$ is a soft topological space over X. However, the union of two soft topological spaces over X may not be a soft topological space over X[8].

Definition 15

Let τ₁ and τ₂ be two soft topologies over X. If${\tau }_1\tilde{\subseteq }{\tau }_2,$ then τ₂ is said to be soft finer than τ₁.

Definition 16

Let τ and ν be two soft topologies on two nonempty sets X and Y , respectively, and f : X → Y be a mapping. The image of τ and the pre-image of ν under f are denoted by f(τ) and f⁻¹(ν), respectively, defined by the following:

• (i) f(τ) = {(G,A) ∈ S(Y,A): f ⁻¹(G,A) = (f ⁻¹(G),A) ∈ τ} and

• (ii) f ⁻¹(ν) = {f ⁻¹(G,A) = (f ⁻¹(G),A): (G,A) ∈ ν}.

Theorem 11

Let τ and ν be two soft topologies on two nonempty sets X and Y, respectively, and f : X → Y be a mapping, and then

(i) f⁻¹(ν) is a soft topology on X, and

(ii) f(τ) is a soft topology on Y.

Proof

Proof follows from Propositions 1, 2, and 3. □

Theorem 12

Let τ₁ and τ₂ be two soft topologies over X. Let f : X → Y be a onto mapping. If${\tau }_1\tilde{\subseteq }{\tau }_2$, then$f\left({\tau }_1\right)\tilde{\subseteq }f\left({\tau }_2\right).$

Theorem 13

Let ν₁ and ν₂ be two soft topologies over Y. Let f : X → Y be a mapping. If${\nu }_1\tilde{\subseteq }{\nu }_2$, then$f^{-1}\left({\nu }_1\right)\tilde{\subseteq }{f}^{-1}\left({\nu }_2\right).$

Soft topological soft groups

In this section, the definition of a soft topological soft group is introduced, and some of its properties are studied. Also, the fundamental homomorphism theorem in the soft topological soft group setting is established. Throughout this section, X and Y are assumed to be groups.

Definition 17

Let (F,A) be a soft group over X and τ be a soft topology on X, and then (F,A,τ) is called a soft topological soft group over X if for each$\alpha \in A,\left[F\right(\alpha ),{\tau }_{F\left(\alpha \right)}^{\alpha }]$ is a topological group on F(α) where${\tau }_{F\left(\alpha \right)}^{\alpha }$ is the relativized topology on F(α) induced from τ^α.

Example 1

Let X = S₃ = {e,(12),(13),(23),(123),(132)}, A = {α₁, α₂}, (F,A) be a soft set defined by F(α₁) = {e, (12)}, F(α₂) = {e, (13)}, and$\tau =\left\{\right(\tilde{\varphi },A),(\tilde{X},A),(F_1,A),(F_2,A),(F_3,A\left)\right\}$ where F₁(α₁) = {e}, F₂(α₁) = {(12)}, F₃(α₁) = {e, (12)}, F₁(α₂) = {e}, F₂(α₂) = {(13)}, F₃(α₂) = {e, (13)}, and then clearly, (F,A) is a soft group over X. Now,${\tau }^{{\alpha }_1}=\{\varphi ,X,\{e\},\{\left(12\right)\left\}\right\{e,\left(12\right)\left\}\right\}$ and${\tau }_{F\left({\alpha }_1\right)}^{{\alpha }_1}=\{\varphi ,\{e\},\{(12)\}\{e,(12)\}\}$.

It can be easily shown that${\tau }_{F\left({\alpha }_1\right)}^{{\alpha }_1}$ is a group topology on F(α₁), and similarly,${\tau }_{F\left({\alpha }_2\right)}^{{\alpha }_2}$ is a group topology on F(α₂). Therefore, (F,A,τ) is a soft topological soft group over X.

Definition 18

Let (F₁,A,τ₁) and (F₂,A,τ₂) be two soft topological soft groups over X, and then their intersection defined by$(F_1,A,{\tau }_1)\tilde{\cap }(F_2,A,{\tau }_2)=(F_1\tilde{\cap }{F}_2,A,{\tau }_1\tilde{\cap }{\tau }_2)$, where$\left(F_1\tilde{\cap }{F}_2\right)\left(\alpha \right)=F_1\left(\alpha \right)\cap F_2\left(\alpha \right),\mathit{\text{for}}\mathit{\text{all}}\alpha \in A,$ and${\tau }_1\tilde{\cap }{\tau }_2$ is defined in as Proposition 6.

Remark 1

If (F₁,A,τ₁) and (F₂,A,τ₂) be two soft topological soft groups on X, then$(F_1\tilde{\cap }{F}_2,A)$ is a soft set, and${\tau }_1\tilde{\cap }{\tau }_2$ is a soft topology on X, but in general,$(F_1\tilde{\cap }{F}_2,A,{\tau }_1\tilde{\cap }{\tau }_2)$ is not necessarily a soft topological soft group, as shown by the following example. However, if τ₁ = τ₂ = τ(say) then by Theorem 16, the intersection of such soft topological soft groups is a soft topological soft group.

Example 2

Let X = S₃ = {e,(12),(13),(23),(123),(132)}, A = {α}, (F,A) be a soft set defined by F(α) = {e, (123), (132)}. Therefore, (F,A) is a soft group over X. Also, let (F₁,A) = {α/{e}}, (F₂,A) = {α/{(123)}}, (F₃,A) = {α/{(132)}}, (F₄,A) = {α/{e, (123)}}, (F₅,A) = {α/{e, (132)}}, (F₆,A) = {α/{(123), (132)}}, (F₇,A) = {α/{e,(123), (132)}}, (F₈,A) = {α/{(12), (132)}}, (F₉,A) = {α/{(12), (123), (132)}},(F₁₀,A) = {α/{e, (12), (132)}}, and (F₁₁,A) = {α/{e, (12), (123), (132)}} are the soft sets over X. If$\tau =\left\{\right(\tilde{\varphi },A),(\tilde{X},A),(F_1,A),(F_2,A),(F_3,A),(F_4,A),(F_5,A),(F_6,A),(F_7,A\left)\right\},$ then τ is a soft topology on X. Thus, τ^α= {ϕ, X, {e}, {(123)}, {(132)}, {e,(123)}, {e,(132)}, {(123), (132)}, {e, (123), (132)}} and${\tau }_{F\left(\alpha \right)}^{\alpha }=\{\varphi ,\{e\},\{(123)\},\{(132)\},\{e,(123)\},\{e,(132)\},\{(123),(132)\},\{e,(123),(132)\}\}$ is a group topology on F(α). Again, if$\nu =\left\{\right(\tilde{\varphi },A),(\tilde{X},A),(F_1,A),(F_2,A),(F_4,A),(F_8,A),(F_9,A),(F_{1}0,A),(F_{1}1,A\left)\right\},$ then ν is a soft topology on X, and ν^α= {ϕ, X, {e}, {(123)}, {e, (123)}, {(12), (132)}, {(12), (123), (132)}, {e, (12), (132)}, {e,(12), (123), (132)}} and${\nu }_{F\left(\alpha \right)}^{\alpha }=\{\varphi ,\{e\},\{\left(123\right)\},\{\left(132\right)\},\{e,\left(123\right)\},\{e,\left(132\right)\},\{\left(123\right),\left(132\right)\},\{e,\left(123\right),\left(132\right)\left\}\right\}$ is a group topology on F(α). Therefore, (F,A,τ) and (F,A,ν) are two soft topological soft groups over X. Now,$\tau \tilde{\cap }\nu =\left\{\right(\tilde{\varphi },A),(\tilde{X},A),(F_1,A),(F_2,A),(F_4,A\left)\right\}$. Hence,${\left(\tau \tilde{\cap }\nu \right)}^{\alpha }=\{\varphi ,X,\{e\},\{\left(123\right)\},\{e,\left(123\right)\left\}\right\}$ and${\left(\tau \tilde{\cap }\nu \right)}_{F\left(\alpha \right)}^{\alpha }=\{\varphi ,\{e\},\{\left(123\right)\},\{e,\left(123\right)\},\{e,\left(123\right),\left(132\right)\left\}\right\}.$ Here, (123),(132) ∈ F(α) and (123)(132) = (e) ∈ {e}, but the only one open set containing (132) is {e, (123), (132)} and (123){e, (123), (132)} = {e, (123), (132)} ⊈ {e}. Hence,${\left(\tau \tilde{\cap }\nu \right)}_{F\left(\alpha \right)}^{\alpha }$ is not a group topology on F(α). Therefore,$(F,A,\tau \tilde{\cap }\nu )$ is not a soft topological soft group over X.

Theorem 14

Let H and G be two subgroups of X and τ be a soft topology on X. If$u\in {\tau }_H^{\alpha }$ and$v\in {\tau }_G^{\alpha }$, then$u\cap v\in {\tau }_{H\cap G}^{\alpha }$.

Proof

Since$u\in {\tau }_H^{\alpha }$, it follows that there exists u₁ ∈ τ^αsuch that u = u₁ ∩H. Now, u₁ ∈ τ^αimplies that ∃ (F₁,A) ∈ τ such that F₁(α) = u₁. Again, since$v\in {\tau }_G^{\alpha }$, it follows that there exists v₁ ∈ τ^αsuch that v = v₁ ∩ G. Now, v₁ ∈ τ^αimplies that ∃ (F₂,A) ∈ τ such that F₂(α) = v₁. Hence,$(F_1\tilde{\cap }{F}_2,A)\in \tau$ and$\left(F_1\tilde{\cap }{F}_2\right)\left(\alpha \right)=F_1\left(\alpha \right)\cap F_2\left(\alpha \right)=u_1\cap v_1.$ Thus,$u_1\cap v_1\in {\tau }^{\alpha }\Rightarrow H\cap G\cap u_1\cap v_1\in {\tau }_{H\cap G}^{\alpha }\Rightarrow (H\cap u_1)\cap (G\cap v_1)=u\cap v\in {\tau }_{H\cap G}^{\alpha }$. □

Theorem 15

Let H and G be two subgroups of X and τ be a soft topology on X. If$(H,{\tau }_H^{\alpha }),(G,{\tau }_G^{\alpha })$ be two topological groups on H,G, respectively, then$[H\cap G,{\tau }_{H\cap G}^{\alpha }]$ is a topological group on H∩G.

Proof

Let x,y ∈ H ∩ G and$W\in {\tau }_{H\cap G}^{\alpha }$ such that x y⁻¹ ∈ W. ⇒ ∃ w ∈ τ^αsuch that W = w ∩ H∩G.⇒ ∃ (F,A) ∈ τ such that F(α) = w ∈ τ^α. Now,$F\left(\alpha \right)\cap H\in {\tau }_H^{\alpha }$ and x y⁻¹ ∈ F(α)∩H. Since${\tau }_H^{\alpha }$ is a topological group,$\exists u_1,v_1\in {\tau }_H^{\alpha }$ and x ∈ u₁, y ∈ v₁ such that$u_1{v}_1^{-1}\subseteq F\left(\alpha \right)\cap H$. Similarly$\exists u_2,v_2\in {\tau }_G^{\alpha }$ and x ∈ u₂, y ∈ v₂ such that$u_2{v}_2^{-1}\subseteq F\left(\alpha \right)\cap G$. Hence, by Theorem 14, we have$u_1\cap u_2\in {\tau }_{H\cap G}^{\alpha }$ and$v_1\cap v_2\in {\tau }_{H\cap G}^{\alpha }$. Also, x ∈ u₁ ∩u₂, y ∈ v₁ ∩ v₂ such that$(u_1\cap u_2){(v_1\cap v_2)}^{-1}\subseteq u_1{v}_1^{-1}\cap u_2{v}_2^{-1}\subseteq F\left(\alpha \right)\cap G\cap H=W$. Therefore,$[H\cap G,{\tau }_{H\cap G}^{\alpha }]$ is a topological group on H∩G. □

Theorem 16

Let (F,A,τ) and (G,A,τ) be two soft topological soft groups over X, and then$(F,A,\tau )\tilde{\cap }(G,A,\tau )=(F\tilde{\cap }G,A,\tau )$ is a soft topological soft group over X.

Proof

(F,A,τ) and (G,A,τ) be two soft topological soft groups over X. ⇒ (F,A) and (G,A) be two soft groups over X, and hence,$(F\tilde{\cap }G,A)$ is a soft group over X.Also, for each$\alpha \in A,\left[F\right(\alpha ),{\tau }_{F\left(\alpha \right)}^{\alpha }]$ and$\left[G\right(\alpha ),{\tau }_{G\left(\alpha \right)}^{\alpha }]$ are two topological groups on F(α) and G(α), respectively. Therefore, by Theorem 15,$\left[F\right(\alpha )\cap G(\alpha ),{\tau }_{F\left(\alpha \right)\cap G\left(\alpha \right)}^{\alpha }]=\left[\right(F\tilde{\cap }G\left)\right(\alpha ),{\tau }_{\left(F\tilde{\cap }G\right)\left(\alpha \right)}^{\alpha }]$ is a topological group on F(α) ∩ G(α), for all α ∈ A. Thus,$(F,A,\tau )\tilde{\cap }(G,A,\tau )$ is a soft topological soft group over X.□

Note 1

Let {(H _i ,A,τ); i ∈ I} be a nonempty family of soft topological soft subgroups over X where I is an index set, and then${\bigcap ^{~}}_{i\in I}(H_i,A,\tau )(=({\tilde{\cap }}_{i\in I}{H}_i,A,\tau \left)\right)$ is a soft topological soft subgroup over X.

Theorem 17

Let (F,A,τ) be a soft topological soft group over X and f : X → Y be an open homomorphism, and then (f(F),A,f(τ)) is a soft topological soft group over Y.

Proof

It is clear that (f(F),A) is a soft group over Y and f(τ) is a soft topology on Y. We show that${\left[f\right(\tau \left)\right]}_{f\left(F\right(\alpha \left)\right)}^{\alpha }$ is a group topology on f(F(α)), for all α ∈ A. Let y₁, y₂ ∈ f(F(α)) and$W\in {\left[f\right(\tau \left)\right]}_{f\left(F\right(\alpha \left)\right)}^{\alpha }$ be such that$y_1{y}_2^{-1}\in W$. Now, y₁,y₂ ∈ f(F(α))⇒∃ x₁,x₂ ∈ F(α) such that f(x₁) = y₁ and f(x₂) = y₂. Again,$W\in {\left[f\right(\tau \left)\right]}_{f\left(F\right(\alpha \left)\right)}^{\alpha }\Rightarrow \exists (G,A)\in f\left(\tau \right)$ such that W = G(α) ∩ f(F(α)). Therefore, (f⁻¹(G),A) ∈ τ. Hence,$\Rightarrow f^{-1}\left(G\right(\alpha \left)\right)\cap F\left(\alpha \right)\in {\left[\tau \right]}_{F\left(\alpha \right)}^{\alpha },\mathit{\text{for}}\mathit{\text{all}}\alpha \in A$. Again,$f\left(x_1{x}_2^{-1}\right)=f\left(x_1\right){\left(f\right(x_2\left)\right)}^{-1}=y_1{y}_2^{-1}\in W=G\left(\alpha \right)\cap f\left(F\right(\alpha \left)\right)\subset G\left(\alpha \right)$. Therefore,$x_1{x}_2^{-1}\in f^{-1}\left(G\right(\alpha \left)\right)$. Also, since x₁,x₂ ∈ F(α) and F(α) is a group,$x_1{x}_2^{-1}\in F\left(\alpha \right)$. Hence,$x_1{x}_2^{-1}\in f^{-1}\left(G\right(\alpha \left)\right)\cap F\left(\alpha \right)$. Since${\left[\tau \right]}_{F\left(\alpha \right)}^{\alpha }$ is a group topology on F(α) and$x_1{x}_2^{-1}\in f^{-1}\left(G\right(\alpha \left)\right)\cap F\left(\alpha \right)\in {\left[\tau \right]}_{F\left(\alpha \right)}^{\alpha }$, there exists$u,v\in {\left[\tau \right]}_{F\left(\alpha \right)}^{\alpha }$ such that x₁ ∈ u,x₂ ∈ v and u v⁻¹ ⊆ f⁻¹(G(α))∩F(α). Hence, ∃ (H,A) ∈ τ such that u = H(α) ∩ F(α). Since f is open, we have [f(H),A] ∈ f(τ) and$y_1\in f\left(u\right)=f\left[H\right(\alpha )\cap F(\alpha \left)\right]\subseteq f\left(H\right(\alpha \left)\right)\cap f\left(F\right(\alpha \left)\right)\in {\left[f\right(\tau \left)\right]}_{f\left(F\right(\alpha \left)\right)}^{\alpha }$. Similarly,$y_2\in f\left(v\right)\in {\left[f\right(\tau \left)\right]}_{f\left(F\right(\alpha \left)\right)}^{\alpha }$. Thus, f(u)[f(v)]⁻¹ = f(u v⁻¹) ⊆ f[f⁻¹(G(α))∩F(α)] ⊆ f f⁻¹(G(α))∩f(F(α)) ⊆ G(α)∩f(F(α)) = W. Therefore, (f(F),A,f(τ)) is a soft topological soft group over Y. □

Theorem 18

Let (G,A,ν) be a soft topological soft group over Y and f : X → Y be an onto homomorphism, and then (f⁻¹(G),A,f⁻¹(ν)) is a soft topological soft group over X.

Proof

It is clear that (f⁻¹ (G),A) is a soft group over X and f⁻¹(ν) is a soft topology on X. We show that${\left[f^{-1}\right(\nu \left)\right]}_{f^{-1}\left(G\right(\alpha \left)\right)}^{\alpha }$ is a group topology on f⁻¹(G(α)), for all α ∈ A. Let x₁, x₂ ∈ f⁻¹(G(α)) and$W\in {\left[f^{-1}\right(\nu \left)\right]}_{f^{-1}\left(G\right(\alpha \left)\right)}^{\alpha }$ be such that$x_1{x}_2^{-1}\in W$. Let f(x₁) = y₁ and f(x₂) = y₂. Thus, y₁,y₂ ∈ G(α). Again,$W\in {\left[f^{-1}\right(\nu \left)\right]}_{f^{-1}\left(G\right(\alpha \left)\right)}^{\alpha }\Rightarrow \exists (F,A)\in f^{-1}\left(\nu \right)$ such that W = F(α) ∩ f⁻¹(G(α)) Now, (F,A) ∈ f⁻¹(ν)n⇒∃ (H₁,A) ∈ ν such that f⁻¹(H₁,A) = (F,A). Hence, W = f⁻¹H₁(α) ∩ f⁻¹(G(α)) = f⁻¹[G(α)∩H₁(α)]. Thus,$y_1{y}_2^{-1}=f\left(x_1\right){\left[f\right(x_2\left)\right]}^{-1}=f\left(x_1{x}_2^{-1}\right)\in f\left(W\right)$ and f(W) = [H₁(α) ∩ G(α)] = H₁(α) ∩ G(α)∈${\left[\nu \right]}_{G\left(\alpha \right)}^{\alpha }$ (since f is onto). Since${\left[\nu \right]}_{G\left(\alpha \right)}^{\alpha }$ is a group topology on G(α), it follows that there exists$u,v\in {\left[\nu \right]}_{G\left(\alpha \right)}^{\alpha }$ with y₁ ∈ u and y₂ ∈ v such that u v⁻¹ ⊆ H₁(α) ∩ G(α). Also,$u\in {\left[\nu \right]}_{G\left(\alpha \right)}^{\alpha }\Rightarrow \exists (H_2,A)\in \nu$ such that u = H₂(α) ∩ G(α). Therefore, [f⁻¹(H₂),A] ∈ f⁻¹(ν) and$x_1\in f^{-1}\left(u\right)=f^{-1}\left[H_2\right(\alpha )\cap G(\alpha \left)\right]=f^{-1}\left(H_2\right(\alpha \left)\right)\cap f^{-1}\left(G\right(\alpha \left)\right)\in {\left[f^{-1}\right(\nu \left)\right]}_{f^{-1}\left(G\right(\alpha \left)\right)}^{\alpha }$. Similarly,$x_2\stackrel{-1}{inf}\left(v\right)\in {\left[f^{-1}\right(\nu \left)\right]}_{f^{-1}\left(G\right(\alpha \left)\right)}^{\alpha }$. Thus,$f^{-1}\left(u\right){\left[f^{-1}\right(v\left)\right]}^{-1}=f^{-1}\left(u{v}^{-1}\right)\subseteq f^{-1}\left[H_1\right(\alpha )\cap G(\alpha \left)\right]=W$. Therefore, (f⁻¹(G),A,f⁻¹(ν)) is a soft topological soft group over X. □

Definition 19

Let (F,A,τ) be a soft topological soft group over X, and then

• (i) (F,A,τ) is said to be an identity soft topological soft group if F(α) = {e}, for all α ∈ A, where e is the identity element of X.

• (ii) (F,A,τ) is said to be an absolute soft topological soft group if F(α) = X, for all α ∈ A.

Theorem 19

Let τ be a soft topology on X and f : X → Y be an open homomorphism:

(i) If (F,A,τ) be an identity soft topological soft group over X, then (f(F),A,f(τ)) is an identity soft topological soft group over Y.

(ii) If (F,A,τ) be a soft topological soft group over X and F(α) = Kerf, for all α ∈ A, then (f(F),A,f(τ)) is an identity soft topological soft group over Y.

(iii) If f is onto and (F,A,τ) be an absolute soft topological soft group over X, then (f(F),A,f(τ)) is an absolute soft topological soft group over Y.

Proof

We give the proof for (i). Proofs of other results are similar:

• (i) From Theorem 17, (f(F),A,f(τ)) is a soft topological soft group over Y. Again, (F,A,τ) is an identity soft topological soft group. Therefore, F(α) = {e}, for all α ∈ A, and hence, [f(F)](α) = f[F(α)] = {e ^′}, for all α ∈ A, where e ^′is the identity element of Y. Therefore, (f(F),A,f(τ)) is an identity soft topological soft group over Y.□

Theorem 20

Let ν be a soft topology on Y and f : X → Y be a homomorphism:

(i) If (G,A,ν) be an identity soft topological soft group over Y and Kerf = {e}, then (f⁻¹(G),A,f⁻¹(ν)) is an identity soft topological soft group over X.

(ii) If (G,A,ν) be an absolute soft topological soft group over Y, then (f⁻¹(G),A,f⁻¹(ν)) is an absolute soft topological soft group over X.

Proof

It follows from Theorem 18 and Theorem 19. □

Definition 20

Let (F₁,A,τ₁) and (F₂,A,τ₂) be two soft topological soft groups over X, and then (F₁,A,τ₁) is said to be a soft topological soft subgroup (soft topological soft normal subgroup) of (F₂,A,τ₂) if the following conditions are met:

• (i) (F ₁,A) is a soft subgroup (soft normal subgroup) of (F ₂,A), and

• (ii)
  $${\left[{\tau }_1^{\alpha }\right]}_{F_1\left(\alpha \right)}={\left[{\left[{\tau }_2^{\alpha }\right]}_{F_2\left(\alpha \right)}\right]}_{F_1\left(\alpha \right)}={\left[{\tau }_2^{\alpha }\right]}_{F_2\left(\alpha \right)/F_1\left(\alpha \right)},\mathit{\text{for}}\mathit{\text{all}}\alpha \in A.$$

This is denoted by$(F_1,A,{\tau }_1)\tilde{\le }(F_2,A,{\tau }_2)\left(\right(F_1,A,{\tau }_1\left)\widetilde{⊲}\right(F_2,A,{\tau }_2\left)\right)$.

Example 3

Let X = S₃ = {e,(12),(13),(23),(123),(132)} and A = {α₁, α₂}. Also, let (F₁,A) = {{e}, {e, (12)}}, (F₂,A) = {{e, (123), (132)}, {e, (12)}},(H₁,A) = {{e}, {e}}, (H₂,A) = {{(123)}, {(12)}}, (H₃,A) = {{(132)}, {(13)}},(H₄,A) = {{e, (123)}, {e, (12)}}, (H₅,A) = {{e, (132)}, {e, (13)}}, (H₆,A) = {{(123), (132)}, {(12), (13)}}, (H₇,A) = {{e, (123),x (132)}, {e, (12) (13)}} and${\tau }_1=\left\{\right(\tilde{\varphi },A),(\tilde{X},A),(H_1,A),(H_2,A),(H_4,A\left)\right\},{\tau }_2=\left\{\right(\tilde{\varphi },A),(\tilde{X},A),(H_1,A),(H_2,A),(H_3,A),(H_4,A),(H_5,A),(H_6,A),(H_7,A\left)\right\}$, then clearly, (F₁,A) is a soft subgroup (soft normal subgroup) of (F₂,A). Now,${\tau }_1^{{\alpha }_1}=\{\varphi ,X,\{e\},\{(123)\},\{e,(123)\}\}$. Hence,${\left[{\tau }_1^{{\alpha }_1}\right]}_{F_1\left({\alpha }_1\right)}=\{\varphi ,\{e\left\}\right\}$ is a discrete topology on F₁(α₁), and hence,$\left(F_1\right({\alpha }_1),{\left[{\tau }_1^{{\alpha }_1}\right]}_{F_1\left({\alpha }_1\right)})$ is a topological group on F₁(α₁). Similarly,${\left[{\tau }_1^{{\alpha }_2}\right]}_{F_1\left({\alpha }_2\right)}=\{\varphi ,\{e\},\{\left(12\right)\},\{e,\left(12\right)\left\}\right\},{\left[{\tau }_2^{{\alpha }_1}\right]}_{F_2\left({\alpha }_1\right)}=\{\varphi ,\{e\},\{\left(123\right)\},\{\left(132\right)\},\{e,\left(123\right)\},\{e,\left(132\right)\},\{\left(123\right),\left(132\right)\},\{e,\left(123\right),\left(132\right)\left\}\right\}$ and${\left[{\tau }_2^{{\alpha }_2}\right]}_{F_2\left({\alpha }_2\right)}=\{\varphi ,\{e\},\{\left(12\right)\},\{e,\left(12\right)\left\}\right\}$ are discrete topologies on F₁(α₂), F₂(α₁) and F₂(α₂), respectively, and hence,$\left(F_1\right({\alpha }_2),{\left[{\tau }_1^{{\alpha }_2}\right]}_{F_1\left({\alpha }_2\right)}),\left(F_2\right({\alpha }_1),{\left[{\tau }_2^{{\alpha }_1}\right]}_{F_2\left({\alpha }_1\right)})$ and$\left(F_2\right({\alpha }_2),{\left[{\tau }_2^{{\alpha }_2}\right]}_{F_2\left({\alpha }_2\right)})$ are topological groups on F₁(α₂), F₂(α₁) and F₂(α₂), respectively. Thus, (F₁,A,τ₁) and (F₂,A,τ₂) are two soft topological soft groups over X. Again,${\left[{\tau }_2^{{\alpha }_1}\right]}_{F_2\left({\alpha }_1\right)/F_1\left({\alpha }_1\right)}=\{\varphi ,\{e\left\}\right\}={\left[{\tau }_1^{{\alpha }_1}\right]}_{F_1\left({\alpha }_1\right)}$ and${\left[{\tau }_2^{{\alpha }_2}\right]}_{F_2\left({\alpha }_2\right)/F_1\left({\alpha }_2\right)}=\{\varphi ,\{e\},\{\left(12\right)\},\{e,\left(12\right)\left\}\right\}={\left[{\tau }_1^{{\alpha }_2}\right]}_{F_1\left({\alpha }_2\right)}$. Therefore, (F₁,A,τ₁) is a soft topological soft subgroup (soft topological soft normal subgroup) of (F₂,A,τ₂).

Theorem 21

Let (F,A,τ) and (G,A,τ) be two soft topological soft groups over X. If F(α)⊆G(α), for all α ∈ A, then$(F,A,\tau )\tilde{\le }(G,A,\tau )$.

Theorem 22

Let (F,A,τ) be a soft topological soft group over X and {(H _i ,A,τ); i ∈ I} be a nonempty family of soft topological soft subgroups (soft topological soft normal subgroups) of (F,A,τ) where I is an index set, and then${\bigcap ^{~}}_{i\in I}(H_i,A,\tau )(=({\tilde{\cap }}_{i\in I}{H}_i,A,\tau \left)\right)$ is a soft topological soft subgroup (soft topological soft normal subgroup) of (F,A,τ).

Proof

From Theorem 14, we get${\bigcap ^{~}}_{i\in I}(H_i,A,\tau )$ is a soft topological soft groups over X. Also, (∩_i∈IH _i )(α) is a subgroup (normal subgroup) of F(α), for all α ∈ A. Hence, (∩_i∈IH _i ,A) is a soft subgroup (soft normal subgroup) of (F,A). Again,${\left[{\tau }^{\alpha }\right]}_{\left({\cap }_{i\in I}{H}_i\right)\left(\alpha \right)}={\left[{\tau }^{\alpha }\right]}_{F\left(\alpha \right)/\left({\cap }_{i\in I}{H}_i\right)\left(\alpha \right)}.$ Therefore,${\bigcap ^{~}}_{i\in I}(H_i,A,\tau )$ is a soft topological soft subgroup (soft topological soft normal subgroup) of (F,A,τ). □

Theorem 23

Let (F,A,τ) be a soft topological soft group over X. If (N,A) be a soft subgroup of (F,A), then (N,A,τ) is a soft topological soft group over X and (N,A,τ) is a soft topological soft subgroup of (F,A,τ).

Proof

Since (F,A,τ) be a soft topological soft group over X, then for each$\alpha \in A,\left[F\right(\alpha ),{\tau }_{F\left(\alpha \right)}^{\alpha }]$ is a topological group on F(α). Also, N(α) is a subgroup of F(α), for all α ∈ A. Thus,${\left[{\tau }_{F\left(\alpha \right)}^{\alpha }\right]}_{N\left(\alpha \right)}=\left[{\tau }_{N\left(\alpha \right)}^{\alpha }\right]$ is a group topology on N(α), for all α ∈ A. Therefore, (N,A,τ) is a soft topological soft group over X. Also, from Definition 20, (N,A,τ) is a soft topological soft subgroup of (F,A,τ). □

Theorem 24

Let τ be a soft topology over X. Let (F₁,A,τ) and (F₂,A,τ) be two soft topological soft groups over X. If f : X → Y be a soft open homomorphism, then (f(F₁),A,f(τ)) and (f(F₂),A,f(τ)) are both soft topological soft groups over Y. Also, if$(F_1,A,\tau )\tilde{\le }\left(\widetilde{⊲}\right)(F_2,A,\tau )$, then$\left(f\right(F_1),A,f(\tau \left)\right)\tilde{\le }\left(\widetilde{⊲}\right)\left(f\right(F_2),A,f(\tau \left)\right).$

Proof

From Theorem 17, we get (f(F₁),A,f(τ)) and (f(F₂),A,f(τ)) are both soft topological soft groups over Y. Also, since$(F_1,A,\tau )\tilde{\le }\left(\widetilde{⊲}\right)(F_2,A,\tau )$, then$(F_1,A)\tilde{\le }\left(\widetilde{⊲}\right)(F_2,A)$ and${\tau }_{F_1\left(\alpha \right)}^{\alpha }={\tau }_{F_2\left(\alpha \right)/F_1\left(\alpha \right)}^{\alpha }$. Thus,$f\left(F_1\right),A\left)\tilde{\le }\left(\widetilde{⊲}\right)\right(f\left(F_2\right),A)$ and${\left[f\right(\tau \left)\right]}_{F_1\left(\alpha \right)}^{\alpha }={\left[f\right(\tau \left)\right]}_{f\left(F_1\right(\alpha \left)\right)/f\left(F_2\right(\alpha \left)\right)}^{\alpha },\mathit{\text{for}}\mathit{\text{all}}\alpha \in A$. Therefore,$\left(f\right(F_1),A,f(\tau \left)\right)\tilde{\le }\left(\widetilde{⊲}\right)\left(f\right(F_2),A,f(\tau \left)\right)$. □

Theorem 25

Let ν be a soft topology over Y. Let (G₁,A,ν) and (G₂,A,ν) be two soft topological soft groups over Y. If f : X → Y be an onto homomorphism, then (f⁻¹(G₁),A,f⁻¹(ν)) and (f⁻¹(G₂),A,f⁻¹(ν)) are both soft topological soft groups over X. Also, if$(G_1,A,\nu )\tilde{\le }\left(\widetilde{⊲}\right)(G_2,A,\nu )$, then$\left(f^{-1}\right(G_1),A,f^{-1}(\nu \left)\right)\tilde{\le }\left(\widetilde{⊲}\right)\left(f^{-1}\right(G_2),A,f^{-1}(\nu \left)\right)$.

Proof

From Theorem 18, we get (f⁻¹(G₁),A,f⁻¹(ν)) and (f⁻¹(G₂),A,f⁻¹(ν)) are both soft topological soft groups over X. Also, since$(G_1,A,\nu )\tilde{\le }\left(\widetilde{⊲}\right)(G_2,A,\nu )$, then$(G_1,A)\tilde{\le }\left(\widetilde{⊲}\right)(G_2,A)$ and${\nu }_{G_1\left(\alpha \right)}^{\alpha }={\nu }_{G_2\left(\alpha \right)/G_1\left(\alpha \right)}^{\alpha }$.$\Rightarrow \left(f^{-1}\right(G_1),A)\tilde{\le }\left(\widetilde{⊲}\right)\left(f^{-1}\right(G_2),A)$ and${\left[f^{-1}\right(\nu \left)\right]}_{G_1\left(\alpha \right)}^{\alpha }={\left[f^{-1}\right(\nu \left)\right]}_{f^{-1}\left(G_1\right(\alpha \left)\right)/f^{-1}\left(G_2\right(\alpha \left)\right)}^{\alpha },\mathit{\text{for}}\mathit{\text{all}}\alpha \in A.$ Therefore,$\left(f^{-1}\right(G_1),A,f^{-1}(\nu \left)\right)\tilde{\le }\left(\widetilde{⊲}\right)\left(f^{-1}\right(G_2),A,f^{-1}(\nu \left)\right)$. □

Theorem 26

Let (F,A,τ) be a soft topological soft group over X and f : X → Y be a homomorphism. Define the set K _f (α) by K _f (α) = [Ker(f)]_F(α)= (Kerf)∩F(α) = {g∈F(α);f(g) = e _Y },for all α ∈ A, then

(i) (K _f ,A,τ) is a soft topological soft group over X.

(ii) (K _f ,A,τ) is a soft topological normal soft subgroup of (F,A,τ).

Proof

It follows from Definition 7 and Theorem 23. □

Remark 2

Let (N,A,τ) and (F,A,τ) be two soft topological soft groups over X such that (N,A,τ) is a soft topological soft normal subgroup of (F,A,τ). Define a mapping$\frac{F}{N}$ over A by$\frac{F}{N}\left(\alpha \right)=$ the factor group$\frac{F\left(\alpha \right)}{N\left(\alpha \right)},\mathit{\text{for}}\mathit{\text{all}}\alpha \in A.$ Therefore,$(\frac{F}{N},A)$ is a factor soft group. Again,$\mathit{\text{for}}\mathit{\text{all}}\alpha \in A,{\tau }_{F\left(\alpha \right)}^{\alpha }$ is a group topology on F(α). If${\psi }_{\alpha }:F\left(\alpha \right)\to \frac{F\left(\alpha \right)}{N\left(\alpha \right)}$ be a canonical mapping and define${\tau }_{\frac{F\left(\alpha \right)}{N\left(\alpha \right)}}^{\alpha }=\{U\subseteq \frac{F\left(\alpha \right)}{N\left(\alpha \right)}:{\psi }_{\alpha }^{-1}(U)\in {\tau }_{F\left(\alpha \right)}^{\alpha }\}$. Therefore, for each$\alpha \in A,(\frac{F\left(\alpha \right)}{N\left(\alpha \right)},{\tau }_{\frac{F\left(\alpha \right)}{N\left(\alpha \right)}}^{\alpha })$ is a topological group on$\frac{F\left(\alpha \right)}{N\left(\alpha \right)}$ (from part b Theorem 8).

Definition 21

Let (F₁,A,τ) and (F₂,A,ν) be two soft topological soft groups over X and Y , respectively, and then (F₁,A,τ) is said to be soft topological soft homomorphic to (onto) (F₂,A,ν), denoted by (F₁,A,τ)∼(F₂,A,ν), if for each$\alpha \in A,\exists {\varphi }_{\alpha }:\left(F_1\right(\alpha ),{\tau }_{F_1\left(\alpha \right)}^{\alpha })\to \left(F_2\right(\alpha ),{\nu }_{F_2\left(\alpha \right)}^{\alpha })$ such that

• (i) ϕ _α : F ₁(α) → F ₂(α) is a homomorphism (onto homomorphism).

• (ii)
  $${\varphi }_{\alpha }:\left(F_1\right(\alpha ),{\tau }_{F_1\left(\alpha \right)}^{\alpha })\to \left(F_2\right(\alpha ),{\nu }_{F_2\left(\alpha \right)}^{\alpha })$$

  is continuous.

Definition 22

Let (F₁,A,τ₁) and (F₂,A,τ₂) be two soft topological soft groups over X and Y , respectively. Also, let (F₁,A,τ₁) be soft topological soft homomorphic to (F₂,A,τ₂). Define ϕ F₁ : A → P(Y) by (ϕ F₁)(α) = (ϕ _α (F₁(α))), for all α ∈ A where ϕ _α satisfies relations (i) and (ii) of the Definition 21.

Definition 23

Let (F₁,A,τ₁) and (F₂,A,τ₂) be two soft topological soft groups over X and Y , respectively. Also, let (F₁,A,τ₁) be soft topological soft homomorphic to (F₂,A,τ₂). Define ϕ⁻¹F₂ : A → P(X) by$\left({\varphi }^{-1}{F}_2\right)\left(\alpha \right)=\left({\varphi }_{\alpha }^{-1}\right(F_2\left(\alpha \right)\left)\right),\mathit{\text{for}}\mathit{\text{all}}\alpha \in A$ where ϕ _α satisfies relations (i) and (ii) of the Definition 21.

Theorem 27

Let (F₁,A,τ) and (F₂,A,ν) be two soft topological soft groups over X and Y, respectively. Also, let (F₁,A,τ) be soft topological soft homomorphic to (F₂,A,ν). If${\varphi }_{\alpha }:\left(F_1\right(\alpha ),{\tau }_{F_1\left(\alpha \right)}^{\alpha })\to \left(F_2\right(\alpha ),{\nu }_{F_2\left(\alpha \right)}^{\alpha })$ be the corresponding homomorphism for each α ∈ A, then

(i) (ϕ F₁,A,ν) is a soft topological soft group over Y and$(\varphi F_1,A,\nu )\tilde{\le }(F_2,A,\nu )$.

(ii) (ϕ⁻¹F₂,A,τ) is a soft topological soft group over X and$({\varphi }^{-1}{F}_2,A,\tau )\tilde{\le }(F_1,A,\tau )$.

Proof

• (i) For each α ∈ A, (ϕ F ₁)(α) = ϕ _α (F ₁(α)) is a subgroup of F ₂(α). Hence, (ϕ F ₁,A) is a soft subgroup of (F ₂,A). By Theorem 22, (ϕ F ₁,A,ν) is a soft topological soft group over Y and $(\varphi F_1,A,\nu )\tilde{\le }(F_2,A,\nu )$.

• (ii) For each $\alpha \in A,\left({\varphi }^{-1}{F}_2\right)\left(\alpha \right)={\varphi }_{\alpha }^{-1}\left(F_2\right(\alpha \left)\right)$ is a subgroup of F ₁(α). Hence, (ϕ ⁻¹ F ₂,A) is a soft subgroup of (F ₁,A), and then by Theorem 22, (ϕ ⁻¹ F ₂,A,τ) is a soft topological soft group over X and $({\varphi }^{-1}{F}_2,A,\tau )\tilde{\le }(F_1,A,\tau )$.

□

Theorem 28

Let (F₁,A,τ₁) and (F₂,A,τ₂) be two soft topological soft groups over X and Y, respectively. Also, let (F₁,A,τ₁) be soft topological soft homomorphic onto (F₂,A,τ₂).

(i) If (F₃,A,τ₁) be a soft topological soft normal subgroup of (F₁,A,τ₁), then (ϕ F₃,A,τ₂) is a soft topological soft normal subgroup of (ϕ F₁,A,τ₂), where ϕF is as defined in Definition 22.

(ii) If (F₄,A,τ₂) be a soft topological soft normal subgroup of (F₂,A,τ₂), then (ϕ⁻¹F₄,A,τ₁) is a soft topological soft normal subgroup of (ϕ⁻¹F₂,A,τ₁), where ϕ⁻¹F is as in Definition 23.

Proof

• (i) Since (F ₁,A,τ ₁) be soft topological soft homomorphic onto (F ₂,A,τ ₂), then it is clear that (ϕ F ₃,A,τ ₂) and (ϕ F ₁,A,τ ₂) are soft topological soft subgroups over Y. Also, for all α ∈ A, ϕ _α (F ₁(α)) and ϕ _α (F ₃(α)) are both subgroups of F ₂(α) and ϕ _α (F ₃(α)) is a normal subgroup of ϕ _α (F ₁(α)). Thus, for all α ∈ A, ϕ _α (F ₃(α)) is a normal subgroup of ϕ _α (F ₁(α)). Thus, (ϕ F ₃,A) is a soft normal subgroup of (ϕ F ₁,A). Therefore, (ϕ F ₃,A,τ ₂) is a soft topological soft normal subgroup of (ϕ F ₁,A,τ ₂).

• (ii) Since (F ₁,A,τ ₁) be soft topological soft homomorphic onto (F ₂,A,τ ₂), then it is clear that (ϕ ⁻¹ F ₃,A,τ ₁) and (ϕ ⁻¹ F ₁,A,τ ₁) are soft topological soft subgroups over X. Also, $\mathit{\text{for all}}\alpha \in A,{\varphi }_{\alpha }^{-1}\left(F_4\right(\alpha \left)\right)$ and ${\varphi }_{\alpha }^{-1}\left(F_2\right(\alpha \left)\right)$ are both subgroups of F ₁(α) and ${\varphi }_{\alpha }^{-1}\left(F_4\right(\alpha \left)\right)$ is a normal subgroup of ${\varphi }_{\alpha }^{-1}\left(F_2\right(\alpha \left)\right)$. Hence, $\mathit{\text{for}}\mathit{\text{all}}\alpha \in A,{\varphi }_{\alpha }^{-1}\left(F_3\right(\alpha \left)\right)$ is a normal subgroup of ${\varphi }_{\alpha }^{-1}\left(F_2\right(\alpha \left)\right)$. Thus, (ϕ ⁻¹ F ₃,A) is a soft normal subgroup of (ϕ ⁻¹ F ₂,A). Therefore, (ϕ ⁻¹ F ₃,A,τ ₁) is a soft topological soft normal subgroup of (ϕ ⁻¹ F ₂,A,τ ₁).

□

Definition 24

Let (F₁,A,τ) and (F₂,A,ν) be two soft topological soft groups over X and Y , respectively, and then (F₁,A,τ) is said to be soft topological soft isomorphic to (F₂,A,ν), denoted by (F₁,A,τ) ≃ (F₂,A,ν), if for each$\alpha \in A,\exists {\varphi }_{\alpha }:\left(F_1\right(\alpha ),{\tau }_{F_1\left(\alpha \right)}^{\alpha })\to \left(F_2\right(\alpha ),{\nu }_{F_2\left(\alpha \right)}^{\alpha })$ such that

• (i) ϕ _α : F ₁(α) → F ₂(α) is an isomorphism.

• (ii)
  $${\varphi }_{\alpha }:\left(F_1\right(\alpha ),{\tau }_{F_1\left(\alpha \right)}^{\alpha })\to \left(F_2\right(\alpha ),{\nu }_{F_2\left(\alpha \right)}^{\alpha })$$

  is homeomorphism.

Theorem 29

Let (N,A,τ) be a soft topological soft normal subgroup of (F,A,τ), and then for each α ∈ A, the canonical mapping${\psi }_{\alpha }:\left(F\right(\alpha ),{\tau }_{F\left(\alpha \right)}^{\alpha })\to \left(\frac{F\left(\alpha \right)}{N\left(\alpha \right)},{\tau }_{\frac{F\left(\alpha \right)}{N\left(\alpha \right)}}^{\alpha }\right),$ given by ψ _α (ξ) = ξN(α), ξ ∈ F(α), is an open homomorphism.

Proof

(N,A,τ) is a soft topological soft normal subgroup of (F,A,τ).⇒ N(α) is normal subgroup of F(α), for all α ∈ A.$\Rightarrow \left(N\right(\alpha ),{\tau }_{N\left(\alpha \right)}^{\alpha })$ is a topological normal subgroup of$\left(F\right(\alpha ),{\tau }_{F\left(\alpha \right)}^{\alpha })$. Therefore, from part c of Theorem 8, the canonical mapping${\psi }_{\alpha }:\left(F\right(\alpha ),{\tau }_{F\left(\alpha \right)}^{\alpha })\to \left(\frac{F\left(\alpha \right)}{N\left(\alpha \right)},{\tau }_{\frac{F\left(\alpha \right)}{N\left(\alpha \right)}}^{\alpha }\right)$ is an open homomorphism. □

Theorem 30

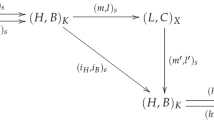
Let (F₁,A,τ) and (F₂,A,ν) be two soft topological groups over X and Y, respectively. Also, let (F₁,A,τ) be soft topological soft homomorphic to (F₂,A,ν). If${\varphi }_{\alpha }:\left(F_1\right(\alpha ),{\tau }_{F_1\left(\alpha \right)}^{\alpha })\to \left(F_2\right(\alpha ),{\nu }_{F_2\left(\alpha \right)}^{\alpha })$ be the corresponding homomorphism for each α ∈ A and K(α) be the kernel of ϕ _α , then${\varphi }_{\alpha }:\left(F_1\right(\alpha ),{\tau }_{F_1\left(\alpha \right)}^{\alpha })\to \left(F_2\right(\alpha ),{\nu }_{F_2\left(\alpha \right)}^{\alpha })$ is continuous (open) if${\varphi }_{\alpha }^0:\left(\frac{F_1\left(\alpha \right)}{K\left(\alpha \right)},{\tau }_{\frac{F_1\left(\alpha \right)}{K\left(\alpha \right)}}^{\alpha }\right)\to \left(F_2\left(\alpha \right),{\nu }_{F_2\left(\alpha \right)}^{\alpha }\right)$ is continuous (open), where${\varphi }_{\alpha }^0\left(\xi K\right(\alpha \left)\right)={\varphi }_{\alpha }\left(\xi \right)$.

Proof

Since${\varphi }_{\alpha }:\left(F_1\right(\alpha ),{\tau }_{F_1\left(\alpha \right)}^{\alpha })\to \left(F_2\right(\alpha ),{\nu }_{F_2\left(\alpha \right)}^{\alpha })$ is the corresponding homomorphism and K(α) be the kernel of ϕ _α . Let${\psi }_{\alpha }:F_1\left(\alpha \right)\to \frac{F_1\left(\alpha \right)}{K\left(\alpha \right)}$ be the canonical mapping defined by ψ _α (ξ) = ξK(α),ξ ∈ F₁(α). Again, define${\varphi }_{\alpha }^0:\left(\frac{F_1\left(\alpha \right)}{K\left(\alpha \right)},{\tau }_{\frac{F_1\left(\alpha \right)}{K\left(\alpha \right)}}^{\alpha }\right)\to \left(F_2\left(\alpha \right),{\nu }_{F_2\left(\alpha \right)}^{\alpha }\right)$ by${\varphi }_{\alpha }^0\left[\xi K\right(\alpha \left)\right]={\varphi }_{\alpha }\left(\xi \right),\xi \in F_1\left(\alpha \right),$ and then${\varphi }_{\alpha }\left(\xi \right)={\varphi }_{\alpha }^0\left[\xi K\right(\alpha \left)\right]={\varphi }_{\alpha }^0\left({\psi }_{\alpha }\right(\xi \left)\right)=\left({\varphi }_{\alpha }^0{\psi }_{\alpha }\right)\left(\xi \right),\mathit{\text{for}}\mathit{\text{all}}\xi \in F_1\left(\alpha \right)$. Therefore,${\varphi }_{\alpha }={\varphi }_{\alpha }^0{\psi }_{\alpha }$, and hence, by Theorem 9,${\varphi }_{\alpha }:\left(F_1\right(\alpha ),{\tau }_{F_1\left(\alpha \right)}^{\alpha })\to \left(F_2\right(\alpha ),{\nu }_{F_2\left(\alpha \right)}^{\alpha })$ is continuous (open) if${\varphi }_{\alpha }^0:\left(\frac{F_1\left(\alpha \right)}{K\left(\alpha \right)},{\tau }_{\frac{F_1\left(\alpha \right)}{K\left(\alpha \right)}}^{\alpha }\right)\to \left(F_2\left(\alpha \right),{\nu }_{F_2\left(\alpha \right)}^{\alpha }\right)$ is continuous (open). □

Theorem 31

(Fundamental homomorphismtheorem) Let (F₁,A,τ) and (F₂,A,ν) be two soft topological soft groups over X and Y, respectively, and (F₁,A,τ) ∼ (F₂,A,ν). Also, let${\varphi }_{\alpha }:\left(F_1\right(\alpha ),{\tau }_{F_1\left(\alpha \right)}^{\alpha })\to \left(F_2\right(\alpha ),{\nu }_{F_2\left(\alpha \right)}^{\alpha })$ be the corresponding homomorphism and K(α) be the kernel of ϕ _α ,for all α ∈ A. If ϕ _α is open and${\psi }_{\alpha }:F_1\left(\alpha \right)\to \frac{F_1\left(\alpha \right)}{K\left(\alpha \right)}$ be the canonical mapping, then (F₁/K,A) ≃ (F₂,A) such that$\mathit{\text{for}}\mathit{\text{all}}\alpha \in A,{\varphi }_{\alpha }^0:\left(\frac{F_1\left(\alpha \right)}{K\left(\alpha \right)},{\tau }_{\frac{F_1\left(\alpha \right)}{K\left(\alpha \right)}}^{\alpha }\right)\to \left(F_2\left(\alpha \right),{\nu }_{F_2\left(\alpha \right)}^{\alpha }\right)$ is a homeomorphism.

Proof

Since (F₁,A,τ) ∼ (F₂,A,ν) and${\varphi }_{\alpha }:\left(F_1\right(\alpha ),{\tau }_{F_1\left(\alpha \right)}^{\alpha })\to \left(F_2\right(\alpha ),{\nu }_{F_2\left(\alpha \right)}^{\alpha })$ be the corresponding homomorphism, then the mapping ϕ _α : F₁(α) → F₂(α) is an algebraic homomorphism, and${\varphi }_{\alpha }:\left(F_1\right(\alpha ),{\tau }_{F_1\left(\alpha \right)}^{\alpha })\to \left(F_2\right(\alpha ),{\nu }_{F_2\left(\alpha \right)}^{\alpha })$ is continuous. Also, K(α) be the kernel of ϕ _α , then by Theorem 7, (F₁/K,A) ≃ (F₂,A). Again, the mapping${\varphi }_{\alpha }^0:\left(\frac{F_1\left(\alpha \right)}{K\left(\alpha \right)},{\tau }_{\frac{F_1\left(\alpha \right)}{K\left(\alpha \right)}}^{\alpha }\right)\to \left(F_2\left(\alpha \right),{\nu }_{F_2\left(\alpha \right)}^{\alpha }\right)$, defined by${\varphi }_{\alpha }^0\left[\xi K\right(\alpha \left)\right]={\varphi }_{\alpha }\left(\xi \right),\mathit{\text{for}}\mathit{\text{all}}\xi \in F_1\left(\alpha \right),$ is an algebraic isomorphism from the group$\frac{F_1\left(\alpha \right)}{K\left(\alpha \right)}$ onto the group F₂(α) and${\varphi }_{\alpha }={\varphi }_{\alpha }^0{\psi }_{\alpha },\mathit{\text{for}}\mathit{\text{all}}\alpha \in A.$ Since ϕ _α is continuous and open, then by Theorem 30,${\varphi }_{\alpha }^0:\left(\frac{F_1\left(\alpha \right)}{K\left(\alpha \right)},{\tau }_{\frac{F_1\left(\alpha \right)}{K\left(\alpha \right)}}^{\alpha }\right)\to \left(F_2\left(\alpha \right),{\nu }_{F_2\left(\alpha \right)}^{\alpha }\right),\mathit{\text{for}}\mathit{\text{all}}\alpha \in A$ is also continuous and open. Since${\varphi }_{\alpha }^0$ is bijective and open,${\left[{\varphi }_{\alpha }^0\right]}^{-1}$ is continuous. Therefore,${\varphi }_{\alpha }^0:\left(\frac{F_1\left(\alpha \right)}{K\left(\alpha \right)},{\tau }_{\frac{F_1\left(\alpha \right)}{K\left(\alpha \right)}}^{\alpha }\right)\to \left(F_2\left(\alpha \right),{\nu }_{F_2\left(\alpha \right)}^{\alpha }\right),\mathit{\text{for}}\mathit{\text{all}}\alpha \in A$ is a homeomorphism. □

Authors’ information

SN is a research scholar at the Department of Mathematics, Visva-Bharati and an assistant professor of Mathematics at Govt. College of Education, Burdwan, Kazirhat, Lakurdi, Burdwan, West Bengal, 713102, India. SKS is a professor of Mathematics at the Department of Mathematics, Visva-Bharati, Santiniketan, West Bengal, 731235, India.