General analytical solutions for DC/AC circuit-network analysis

Abstract

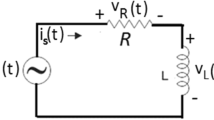
In this work, we present novel general analytical solutions for the currents that are developed in the edges of network-like circuits when some nodes of the network act as sources/sinks of DC or AC current. We assume that Ohm’s law is valid at every edge and that charge at every node is conserved (with the exception of the source/sink nodes). The resistive, capacitive, and/or inductive properties of the lines in the circuit define a complex network structure with given impedances for each edge. Our solution for the currents at each edge is derived in terms of the eigenvalues and eigenvectors of the Laplacian matrix of the network defined from the impedances. This derivation also allows us to compute the equivalent impedance between any two nodes of the circuit and relate it to currents in a closed circuit which has a single voltage generator instead of many input/output source/sink nodes. This simplifies the treatment that could be done via Thévenin’s theorem. Contrary to solving Kirchhoff’s equations, our derivation allows to easily calculate the redistribution of currents that occurs when the location of sources and sinks changes within the network. Finally, we show that our solutions are identical to the ones found from Circuit Theory nodal analysis.