Skip to main content
SpringerLink
Log in

Series expansion solutions for the multi-term time and space fractional partial differential equations in two- and three-dimensions

  • Regular Article
  • Published:
The European Physical Journal Special Topics Aims and scope Submit manuscript

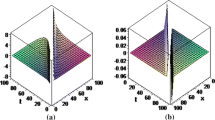
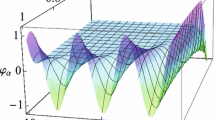
Abstract

Fractional partial differential equations with more than one fractional derivative in time describe some important physical phenomena, such as the telegraph equation, the power law wave equation, or the Szabo wave equation. In this paper, we consider two- and three-dimensional multi-term time and space fractional partial differential equations. The multi-term time-fractional derivative is defined in the Caputo sense, whose order belongs to the interval (1,2],(2,3],(3,4] or (0,m], and the space-fractional derivative is referred to as the fractional Laplacian form. We derive series expansion solutions based on a spectral representation of the Laplacian operator on a bounded region. Some applications are given for the two- and three-dimensional telegraph equation, power law wave equation and Szabo wave equation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Similar content being viewed by others

References

  1. D.A. Benson, S.W. Wheatcraft, M.M. Meerschaert, Water Resour. Res. 36, 1403 (2000)

    Article  ADS  Google Scholar 

  2. D.A. Benson, S.W. Wheatcraft, M.M. Meerschaert, Water Resour. Res. 36, 1413 (2000)

    Article  ADS  Google Scholar 

  3. J. Chen, F. Liu, V. Anh, J. Math. Anal. Appl. 338, 1364 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  4. I.H. Dimovski, Convolutional Calculus (Bulgarian Academy of Sciences, Sofia, 1982)

  5. E.C. Eckstein, J.A. Goldsten, M. Leggas, Electron. J. Differential Equation 3, 39(1999)

    Google Scholar 

  6. R. Hilfer, Applications of Fractional Calculus in Physics (World Scientific, River Edge, New Jersey, 2000)

  7. M. Ilic, F. Liu, I. Turner, V. Anh, Fractional Calculus Appl. Anal. 9, 333 (2006)

    MathSciNet  MATH  Google Scholar 

  8. H. Jiang, F. Liu, I. Turner, K. Burrage, J. Math. Anal. Appl. 389, 1117 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  9. H. Jiang, F. Liu, I. Turner, K. Burrage, Comput. Math. Appl. (2012), doi: 10.1016/j.camwa.2012.02.042

  10. H. Jiang, F. Liu, M.M. Meerschaert, et al., Electr. J. Math. Anal. Appl. 1, 1 (2013)

    Google Scholar 

  11. J.K. Kelly, R.J. McGough, M.M. Meerschaert, J. Acoust. Soc. Am. 124, 2861 (2008)

    Article  ADS  Google Scholar 

  12. C.P. Li, D.L. Qian, Y.Q. Chen, Discr. Dyn. Nature Soc. ID 562494, 15 pages (2011)

    Google Scholar 

  13. C.P. Li, Z.G. Zhao, Eur. Phys. J. Special Topics 193, 5 (2011)

    Article  ADS  Google Scholar 

  14. F. Liu, V. Anh, I. Turner, J. Comput. Appl. Math. 166, 209 (2004)

    Article  MathSciNet  ADS  MATH  Google Scholar 

  15. F. Liu, M M. Meerschaert, R.J. McGugh, et al., Fract. Calculus Appl. Anal. 16, 9 (2013)

    Article  Google Scholar 

  16. Y. Luchko, R. Gorenflo, ACTA Math. Vietnamica 24, 207 (1999)

    MathSciNet  MATH  Google Scholar 

  17. Y. Luchko, J. Math. Anal. Appl. 374, 538 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  18. R. Metzler, J. Klafter, Phys. Rep. 339, 1 (2000)

    Article  MathSciNet  ADS  MATH  Google Scholar 

  19. S. Momani, Z. Odibat, Numer. Meth. Partial Differ. Eq. 24, 1416 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  20. I. Podlubny, Fractional Differential Equations (Academic Press, San Diego, 1999)

  21. M. Stojanovic, J. Comp. Appl. Math. 235, 3121 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  22. X. Wang, F. Liu, J. Fuzhou University 35, 520 (2007)

    MATH  Google Scholar 

  23. Q. Yang, F. Liu, I. Turner, Appl. Math. Modell. 34, 200 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  24. G.M. Zaslavsky, Phys. Rep. 371, 461 (2002)

    Article  MathSciNet  ADS  MATH  Google Scholar 

  25. Y. Zhang, D.A. Benson, D.M. Reeves, Adv. Water Res. 32, 561 (2009)

    Article  Google Scholar 

  26. P. Zhuang, F. Liu, V. Anh, I. Turner, SIAM J. Numer. Anal. 47, 1760 (2009)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Applied Mathematics, Donghua University, Shanghai, 201620, PR China

    H. Ye

  2. Mathematical Sciences, Queensland University of Technology, GPO Box 2434, Brisbane, Qld., 4001, Australia

    F. Liu, I. Turner, V. Anh & K. Burrage

  3. Department of Computing Science and OCISB, Oxford University, Oxford, OXI 3QD, UK

    K. Burrage

Authors
  1. H. Ye
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. F. Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. I. Turner
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. V. Anh
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. K. Burrage
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to F. Liu.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Ye, H., Liu, F., Turner, I. et al. Series expansion solutions for the multi-term time and space fractional partial differential equations in two- and three-dimensions. Eur. Phys. J. Spec. Top. 222, 1901–1914 (2013). https://doi.org/10.1140/epjst/e2013-01972-2

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1140/epjst/e2013-01972-2

Keywords

Search

Navigation