Abstract
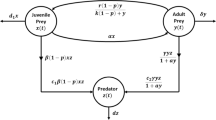
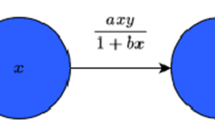
One interesting question in love relationships is: finally, what and when is the end of this love relationship? Using a prey–predator Verhulst–Lotka–Volterra (VLV) model we imply cooperation and competition tendency between people in order to describe a “love dilemma game”. We select the most simple but immediately most complex case for studying the set of nonlinear differential equations, i.e. that implying three persons, being at the same time prey and predator. We describe four different scenarios in such a love game containing either a one-way love or a love triangle. Our results show that it is hard to love more than one person simultaneously. Moreover, to love several people simultaneously is an unstable state. We find some condition in which persons tend to have a friendly relationship and love someone in spite of their antagonistic interaction. We demonstrate the dynamics by displaying flow diagrams.
Article PDF
Similar content being viewed by others
Avoid common mistakes on your manuscript.
References
S.N. Dorogovtsev, J.F.F. Mendes, Evolution of networks: from biological nets to the Internet and WWW (Oxford University Press, Oxford, 2003)
A. Rapoport, A.M. Chammah, Prisoner’s dilemma: a study in conflict and cooperation (University of Michigan Press, Ann Arbor, MI, USA, 1965)
W. Poundstone, Prisoner’s dilemma (Doubleday Publishing Group, New York, NY, USA, 1992)
V. Volterra, Mem. R. Accad. Naz. dei Lincei VI 2, 31 (1926)
N.K. Vitanov, Z.I. Dimitrova, M. Ausloos, Physica A 389, 4970 (2010)
N.K. Vitanov, M. Ausloos, G. Rotundo, Adv. Complex Syst. 15, 1250049 (2012)
P.F. Verhulst, Nouv. Mémoires de l’Académie Royale des Sci. et Belles-Lettres de Bruxelles 18, 14 (1845)
P.F. Verhulst, Mémoires de l’Académie Royale des Sci. des Lettres et des Beaux-Arts de Belgique 20, 1 (1847)
L.F. Caram, C.F. Caiafa, A.N. Proto, M. Ausloos, Physica A 389, 2628 (2010)
L.F. Caram, C.F. Caiafa, A.N. Proto, M. Ausloos, Phys. Rev. E 92, 022805 (2015)
A. Sonubi, A. Arcagni, S. Stefani, M. Ausloos, Phys. Rev. E 94, 022303 (2016)
A. Gadomski, M. Ausloos, T. Casey, Nonlinear Dyn. Psychol. Life Sci. 21, 129 (2017)
G. Rotundo, M. Ausloos, Physica A 389, 5479 (2010)
G. Rotundo, M. Ausloos, Eur. Phys. J. B 86, 169 (2013)
S.H. Strogatz, Nonlinear dynamics and chaos: with applications to physics, biology, chemistry, and engineering (Westview Press, Boulder, CO, USA, 2014)
H. Safdari, M. Kamali, A. Shirazi, M. Khalighi, G.R. Jafari, M. Ausloos, PLoS ONE 11, e0154983 (2016)
F. Hassanibesheli, L. Hedayatifar, H. Safdari, M. Ausloos, G.R. Jafari, Entropy 19, 246 (2017)
M. Saeedian, M. Khalighi, N. Azimi-Tafreshi, G.R. Jafari, M. Ausloos, Phys. Rev. E 95, 022409 (2017)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (https://creativecommons.org/licenses/by/4.0), which permits use, duplication, adaptation, distribution, and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Simin, P.T., Jafari, G.R., Ausloos, M. et al. Dynamical phase diagrams of a love capacity constrained prey–predator model. Eur. Phys. J. B 91, 43 (2018). https://doi.org/10.1140/epjb/e2017-80531-7
Received:
Revised:
Published:
DOI: https://doi.org/10.1140/epjb/e2017-80531-7