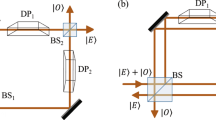
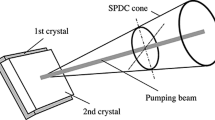
Abstract
Since the 1970s the use of polarization measurements have become standard in optical experiments designed to test the entanglement features of quantum mechanics. Here, we examine the genesis of the conceptual-theoretical and, experimental, configurations that introduced the notion of polarization measurements of quanta traveling in opposite directions. This search uncovers a number of highly relevant publications from the late 1940s that remain largely ignored and unreferenced in today’s literature.
Similar content being viewed by others
References
Aspect, A., P. Grangier and G. Roger. 1982a. Experimental realization of the Einstein-Podolsky-Rosen-Bohm gedankenexperiment : a new violation of Bell’s inequalities. Phys. Rev. Lett. 49 : 91–94
Aspect, A., P. Grangier and G. Roger. 1982b. Experimental test of Bell’s inequalities using time-varying analyzers. Phys. Rev. Lett. 49 : 1804–1807
Bell, J.S. 1964. On the Einstein-Podolsky-Rosen paradox. Physics 1 : 195–200
Bleuler, E. and H.L. Bradt. 1948. Correlation between the states of polarization of the two quanta of annihilation radiation. Phys. Rev. 73 : 1398
Bohm, D. and Y. Aharonov. 1957. Discussion of experimental proof for the paradox of Einstein, Rosen and Podolsky. Phys. Rev. 108 : 1070–1076
Bohm, D. and Y. Aharonov. 1960. Further discussion of possible experimental tests for the paradox of Einstein, Podolsky and Rosen. Nuovo Cimento 17 : 964–976
Clauser, J.F. and M.A. Horne. 1974. Experimental consequences of objective local theories. Phys. Rev. D 10 : 526–535
Clauser, J.F., M.A. Horne, A. Shimony and R.A. Holt. 1969. Proposed experiment to test hidden variable theories. Phys. Rev. Lett. 23 : 880–884
Dalitz, R.H. and F.J. Duarte. 2000. John Clive Ward. Physics Today 53(10) : 99–100
Dirac, P.A.M. 1930. On the annihilation of electrons and protons. Camb. Phil. Soc. 26 : 361–375
Dirac, P.A.M. 1978. The Principles of Quantum Mechanics, 4th edn. Oxford University, Oxford
Dirac, P.A.M. 1987. The inadequacies of quantum field theory, in Paul Adrien Maurice, Dirac, edited by B.N. Kursunoglu and E.P. Wigner. Cambridge, London, Chap. 15
Duarte, F.J. 2009. The man behind an identity in quantum electrodynamics. Aust. Phys. 46 : 171–175
Duncan, A.J. and H. Kleinpoppen. 1988. The experimental investigation of the Einstein-Podolsky-Rosen question and Bell’s inequality, in Quantum Mechanics Versus Local Realism, edited by F. Selleri Plenum, New York, Chap. 7
Einstein, A., B. Podolsky and N. Rosen. 1935. Can quantum mechanical description of physical reality be considered complete? Phys. Rev. 47 : 777–780
Freedman, S.J. and J.F. Clauser. 1972. Experimental tests of local hidden variable theories. Phys. Rev. Lett. 28 : 938–941
Hanna, R.C. 1948. Polarization of annihilation radiation. Nature 162 : 332
Kasday, L.R., J.D. Hullman, and C.S. Wu. 1975. Angular correlation of Compton-scattered annihilation photons and hidden variables. Nuovo Cimento B 17 : 633–661
Mandel L. and E. Wolf. 1995. Optical Coherence and Quantum Optics. Cambridge University, Cambridge
Peres, A. and P. Singer. 1960. On possible experimental tests for the paradox of Einstein, Podolsky and Rosen. Nuovo Cimento 15 : 907–915
Pryce, M.H.L. and J.C. Ward. 1947. Angular correlation effects with annhilation radiation. Nature 160 : 435
Sakharov, A. 1990. Memoirs, Knopf, New York
Selleri F. (Ed.) 1988. Quantum Mechanics Versus Local Realism. Plenum, New York
Shih, Y.H. and C.O. Alley. 1988. New type of Einstein-Podolsky-Rosen-Bohm experiment using pairs of light quanta produced by optical parametric down conversion. Phys. Rev. Lett. 61 : 2921–2924
Snyder, H.S., S. Pasternack and J. Hornbostel. 1948. Angular correlation of scattered annihilation radiation. Phys. Rev. 73 : 440–448
Ward, J.C. 1949. Some Properties of the Elementary Particles. Ph.D. Phil thesis, Oxford University, Oxford
Ward, J.C. 2004. Memoirs of a Theoretical Physicist. Optics Journal, Rochester
Wheeler, J.A. 1946. Polyelectrons. Annals of the New York Academy of Sciences 48 : 219–238
Wu, C.S. and I. Shaknov. 1950. The angular correlation of scattered annihilation radiation. Phys. Rev. 77 : 136
Yang, C.N. 1950. Selection rule for the dematerialization of a particle into two photons. Phys. Rev. 77 : 242–245
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Duarte, F.J. The origin of quantum entanglement experiments based on polarization measurements. EPJ H 37, 311–318 (2012). https://doi.org/10.1140/epjh/e2012-20047-y
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1140/epjh/e2012-20047-y