Abstract
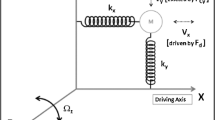
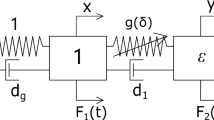
The performance of a ring of linearly coupled, monostable nonlinear oscillators is optimized towards its goal of acting as energy harvester – through piezoelectric transduction – of mesoscopic fluctuations, which are modeled as Ornstein-Uhlenbeck noises. For a single oscillator, the maximum output voltage and overall efficiency are attained for a soft piecewise-linear potential (providing a weak attractive constant force) but they are still fairly large for a harmonic potential. When several harmonic springs are linearly and bidirectionally coupled to form a ring, it is found that counter-phase coupling can largely improve the performance while in-phase coupling worsens it. Moreover, it turns out that few (two or three) coupled units perform better than more.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
L. Mateu, F. Moll, Review of energy harvesting techniques and applications for microelectronics (keynote address), in Proc. SPIE 5837, VLSI Circuits and Systems II (2005), p. 359
R.J.M. Vullers, R. van Schaijk, I. Doms, C. Van Hoof, R. Mertens, Solid-St. Electron. 53, 684 (2009)
F. Yildiz, J. Technol. Studies 35, 40 (2009)
A. Erturk, Ph.D. thesis, Virginia Polytechnic Institute and State University, 2009
H.A. Sodano, D.J. Inman, G. Park, J. Intell. Mater. Syst. Struct. 16, 799 (2005)
L. Tang, Y. Yang, C.K. Soh, J. Intell. Mater. Syst. Struct. 21, 1867 (2010)
L. Gammaitoni, I. Neri, H. Vocca, Appl. Phys. Lett. 94, 164102 (2009)
F. Cottone, H. Vocca, L. Gammaitoni, Phys. Rev. Lett. 102, 080601 (2009)
L. Gammaitoni, I. Neri, H. Vocca, Chem. Phys. Lett. 375, 435 (2010)
G. Litak, E. Manoach, Eur. Phys. J. Special Topics 222, 1479 (2013)
G. Litak, E. Manoach, E. Halvorsen, Eur. Phys. J. Special Topics 224, 671 (2015)
J.I. Deza, R.R. Deza, H.S. Wio, Europhys. Lett. 100, 38001 (2012)
J.I. Peña Rosselló, J.I. Deza, H.S. Wio, R.R. Deza, Anales AFA 25, 54 (2014)
J.I. Peña Rosselló, R.R. Deza, J.I. Deza, H.S. Wio, Papers in Physics 7, 070014 (2015)
L. Gammaitoni, P. Hänggi, P. Jung, F. Marchesoni, Rev. Mod. Phys. 70, 223 (1998)
A.R. Bulsara, L. Gammaitoni, Phys. Today 49, 39 (1996)
J.F. Lindner, B.K. Meadows, W.L. Ditto, M.E. Inchiosa, A.R. Bulsara, Phys. Rev. Lett. 75, 3 (1995)
J.F. Lindner, B.K. Meadows, W.L. Ditto, M.E. Inchiosa, A.R. Bulsara, Phys. Rev. E 53, 2081 (1996)
H.S. Wio, S. Bouzat, B. von Haeften, Physica A 306, 140 (2002)
H.S. Wio, J.A. Revelli, M.A. Rodríguez, R.R. Deza, G.G. Izús, Eur. Phys. J. B 69, 71 (2009)
B. von Haeften, G.G. Izús, H.S. Wio, Phys. Rev. E 72, 021101 (2005)
H.S. Wio, R.R. Deza, J.M. López, An Introduction to Stochastic Processes and Nonequilibrium Statistical Physics, revised edn. (World Scientific, Singapore, 2012)
J.I. Deza, R.R. Deza, H.S. Wio, Nanoenergy Lett. 6, 29 (2013)
P. Hänggi, M. Inchiosa, D. Fogliatti, A. Bulsara, Phys. Rev. E 62, 6155 (2000)
J. Casado-Pascual, J. Gómez-Ordoñez, M. Morillo, P. Hänggi, Phys. Rev. E 67, 036109 (2003)
V. Méndez, D. Campos, W. Horsthemke, Phys. Rev. E 88, 022124 (2013)
M.C. Cross, P.C. Hohenberg, Rev. Mod. Phys. 65, 851 (1993)
M. Cross, H. Greenside, Pattern formation and dynamics in nonequilibrium systems (CUP, Cambridge, 2009)
H.S. Wio, Int. J. Bifurc. Chaos 19, 2813 (2009)
N.W. Ashcroft, D.N. Mermin, Solid state physics (Harcourt, Orlando, FL, 1976)
P. Dayan, L.F. Abbott, Theoretical neuroscience (MIT Press, Cambridge, MA, 2001)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Peña Rosselló, J.I., Wio, H.S., Deza, R.R. et al. Enhancing energy harvesting by coupling monostable oscillators. Eur. Phys. J. B 90, 34 (2017). https://doi.org/10.1140/epjb/e2017-70703-x
Received:
Revised:
Published:
DOI: https://doi.org/10.1140/epjb/e2017-70703-x