Abstract
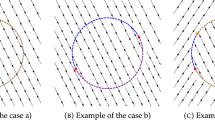
A one-parameter periodic impulsive system of the second order is considered. The conditions for the appearance of the generic Andronov–Hopf bifurcation are discussed. It is shown that this bifurcation leads to the appearance of an almost periodic solution, the trajectory of which completely fills a certain annular region surrounding the rest point. Explicit formulas are also obtained that make it possible to calculate the numerical characteristic of the stability of an impulsive system in the critical case of a pair of complex conjugate multipliers of the monodromy matrix of the linear approximation in the vicinity of the rest point.
Similar content being viewed by others
REFERENCES
J. C. Panetta, ‘‘A mathematical model of periodically pulsed chemotherapy: Tumor recurrence and metastasis in a competition environment,’’ Bull. Math. Biol. 58, 425–447 (1996).
A. Lakmeche and O. Arino, ‘‘Bifurcation of nontrivial periodic solutions of impulsive differential equations arising from chemotherapeutic treatment,’’ Dyn. Control Discrete Impl. Syst. 7, 265–288 (2000).
A. Lakmeche and O. Arino, ‘‘Nonlinear mathematical model of pulsed-therapy of heterogeneous tumors,’’ Nonlin. Anal. Real World Appl. 2, 455–465 (2001).
G. Jiang and Q. Yang, ‘‘Bifurcation analysis in an SIR epidemic model with birth pulse and pulse vaccination,’’ Appl. Math. Comput. 215, 1035–1046 (2009).
A. Boudermine, M. Helal, and A. Lakmeche, ‘‘Bifurcation of nontrivial periodic solutions for pulsed chemotherapy model,’’ Math. Sci. Appl. E-Notes 2 (2), 22–44 (2014).
M. Bachar, J. G. Raimann, and P. Kotanko, ‘‘Impulsive mathematical modeling of ascorbic acid metabolism in healthy subjects,’’ J. Theor. Biol. 392, 35–47 (2016).
Y. Xie, L. Wang, Q. Deng, and Z. Wu, ‘‘The dynamics of an impulsive predator-prey model with communicable disease in the prey species only,’’ Appl. Math. Comput. 292, 320–335 (2017).
A. M. Samoilenko and M. O. Perestyuk, Impulsive Differential Equations, Vol. 14 of World Scientific Series on Nonlinear Science, Series A (Vysha Shkola, Kyev, 1987; World Scientific, River Edge, 1995).
A. M. Samoilenko and M. O. Perestyuk, Impulsive Differential Equations (World Scientific, River Edge, 1995).
D. Bainov and P. Simeonov, Theory of Impulsive Differential Equations: Periodic Solutions and Applications (Longman, Harlow, 1993).
G. T. Stamov, Almost Periodic Solutions of Impulsive Differential Equations (Springer, Heidelberg, New York, 2012).
S. G. Hristova, Qualitative Investigations and Approximate Methods for Impulsive Equations (Nova Science, New York, 2009).
W. M. Haddad, V. Chellabona, and S. G. Nersesov, Impulsive and Hybrid Dynamical Systems: Stability, Dissipativity, and Control (Princeton Univ. Press, Princeton, 2006).
M. U. Akhmet, ‘‘Perturbations and Hopf bifurcation of the planar discontinuous dynamical system,’’ Nonlin. Anal.: TMA 60, 163–178 (2005).
M. Akhmet, Principles of Discontinuous Dynamical Systems (Springer, New York, 2010).
K. E. M. Church and Xinzhi Liu, ‘‘Bifurcation analysis and application for impulsive systems with delayed impulses,’’ Int. J. Bifurc. Chaos 27, 1750186 (2017).
K. Church, ‘‘Invariant manifold theory for impulsive functional differential equations with applications,’’ Ph.D. Thesis (Univ. of Waterloo, 2019).
K. E. M. Church and X. Liu, Bifurcation Theory of Impulsive Dynamical Systems, Vol. 34 of IFSR International Series in Systems Science and Systems Engineering (Springer Nature, Cham, 2021).
Z. Hu and M. Han, ‘‘Periodic solutions and bifurcations of first-order periodic impulsive differential equations,’’ Int. J. Bifurc. Chaos 19, 2515–2530 (2009).
O. V. Anashkin, N. O. Sedova, and O. V. Yusupova, ‘‘Bifurcations of periodic solutions of a differential equation with impulse action,’’ Dinam. Sist. 7 (35), 395–403 (2017).
O. Anashkin, ‘‘Bifurcations of solutions of impulsive differential equations,’’ in Proceedings of the 2018 14th International Conference on Stability and Oscillations of Nonlinear Control Systems (Pyatnitskiy’s Conference) STAB (2018), pp. 1–3. doi 10.1109/STAB.2018.8408341
O. Anashkin and O. Yusupova, ‘‘Sufficient conditions for stability of the equilibrium position of an impulsive system,’’ in Proceedings of the 2020 15th International Conference on Stability and Oscillations of Nonlinear Control Systems (Pyatnitskiy’s Conference) STAB (2020), pp. 1–4. doi 10.1109/STAB49150.2020.9140587
S. V. Babenko and V. I. Slyn’ko, ‘‘Stability of motion of nonlinear systems with impulsive action of the second order in critical cases,’’ Dopov. Nats. Akad. Nauk Ukr., No. 6, 46–52 (2008).
Yu. A. Kuznetsov, Elements of Applied Bifurcation Theory, 3rd ed., Vol. 112 of Applied Mathematical Sciences (Springer, New York, 2004), p. 631.
Y.-H. Wan, ‘‘Computations of the stability condition for the Hopf bifurcation of diffeomorphisms on \(\mathbb{R}^{2}\),’’ SIAM J. Appl. Math. 34, 167–175 (1978).
M. Akhmet and A. Kashkynbayev, Bifurcation in Autonomous and Nonautonomous Differential Equations with Discontinuities (Springer Nature, Higher Education Press, Singapore, 2017).
Author information
Authors and Affiliations
Corresponding authors
Additional information
(Submitted by A. B. Muravnik)
Rights and permissions
About this article
Cite this article
Anashkin, O.V., Yusupova, O.V. Stability in the Critical Case and Bifurcations in Impulsive Systems. Lobachevskii J Math 42, 3574–3583 (2021). https://doi.org/10.1134/S1995080222030039
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1134/S1995080222030039