Circular Vortex Arrays in Generalised Euler’s and Quasi-geostrophic Dynamics

Abstract

We investigate the stability of circular point vortex arrays and their evolution when their dynamics is governed by the generalised two-dimensional Euler’s equations and the three-dimensional quasi-geostrophic equations. These sets of equations offer a family of dynamical models depending continuously on a single parameter $\beta$ which sets how fast the velocity induced by a vortex falls away from it. In this paper, we show that the differences between the stability properties of the classical two-dimensional point vortex arrays and the standard quasi-geostrophic vortex arrays can be understood as a bifurcation in the family of models. For a given $\beta$, the stability depends on the number $N$ of vortices along the circular array and on the possible addition of a vortex at the centre of the array. From a practical point of view, the most important vortex arrays are the stable ones, as they are robust and long-lived. Unstable vortex arrays can, however, lead to interesting and convoluted evolutions, exhibiting quasi-periodic and chaotic motion. We briefly illustrate the evolution of a small selection of representative unstable vortex arrays.

APPENDIX

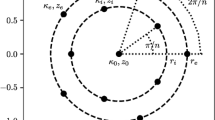
We describe the general equations used for the linear stability analysis for the $N+1$-vortex arrays in generalised three-dimensional QG equations. For the $N$-vortex arrays, the equations are the same, just removing explicitly vortex 0 from the equations. For the two-dimensional generalised Euler equations, we simply remove the $z$-component in all distances. The $N$ peripheral vortices of strength $\kappa_{i}=\kappa_{p}$, $1\leqslant i\leqslant N$ are located along a ring of radius $R$ at a polar angle $\{\theta_{i}\}_{i=1,N}$. The central vortex is located at $(0,0,0)$ and has strength $\kappa_{0}=\kappa_{c}$. Note that in the present study the equations simplify a little with $R=1$ and $z_{i}=0$, $\forall i$:

$$\theta_{i}=\dfrac{i-1}{N}2\pi.$$

(A.1)

Vortex 1 is located at $(R,0,0)$ and is used to evaluate the uniform rotation angular velocity $\Omega$

$$\Omega=\dfrac{v_{1}}{R}=\dfrac{\kappa_{0}}{R^{\beta+2}}+\sum_{i=2}^{N}\kappa_{i}\dfrac{1-\cos\theta_{i}}{R^{\beta+2}\left(\left(1-\cos\theta_{i}\right)^{2}+\sin^{2}\theta_{i}\right)^{(\beta+2)/2}}.$$

(A.2)

Let $(x_{i},y_{i},z_{i})$ be the location of point vortex $i$, we denote

$$r_{ij}^{2}=(x_{i}-x_{j})^{2}+(y_{i}-y_{j})^{2}+(z_{i}-z_{j})^{2}.$$

(A.3)

We consider perturbations of the horizontal position of the vortices

$$(x_{i}^{\prime},y_{i}^{\prime},0)=e^{\sigma t}(\tilde{x}_{i},\tilde{y}_{i},0).$$

(A.4)

We do not consider vertical perturbation since the vertical advection is negligible in the QG model. The equations for the perturbations are obtained by linearising the equations of motion of the vortices in the reference frame rotating with the vortices about their equilibrium position:

$$\displaystyle\dfrac{\mathrm{d}x_{i}^{\prime}}{\mathrm{d}t}=\sigma x_{i}^{\prime}=\Omega y_{i}^{\prime}$$

$$\displaystyle-\sum_{j=0,j\neq i}^{N}\dfrac{\kappa_{j}}{r_{ij}^{\beta+2}}\left[\left(1-(\beta+2)\dfrac{(y_{i}-y_{j})^{2}}{r_{ij}^{2}}\right)(y_{i}^{\prime}-y_{j}^{\prime})-(\beta+2)\dfrac{(y_{i}-y_{j})(x_{i}-x_{j})}{r_{ij}^{2}}(x_{i}^{\prime}-x_{j}^{\prime})\right]$$

(A.5)

$$\displaystyle\dfrac{\mathrm{d}y_{i}^{\prime}}{\mathrm{d}t}=\sigma y_{i}^{\prime}=-\Omega x_{i}^{\prime}$$

$$\displaystyle+\sum_{j=0,j\neq i}^{N}\dfrac{\kappa_{j}}{r_{ij}^{\beta+2}}\left[\left(1-(\beta+2)\dfrac{(x_{i}-x_{j})^{2}}{r_{ij}^{2}}\right)(x_{i}^{\prime}-x_{j}^{\prime})-(\beta+2)\dfrac{(y_{i}-y_{j})(x_{i}-x_{j})}{r_{ij}^{2}}(y_{i}^{\prime}-y_{j}^{\prime})\right],$$

(A.6)

which leads to a $(2N+2)$-eigenvalue problem where $\sigma$ is the eigenvalue and $(x_{0}^{\prime},\ldots,x_{N}^{\prime},y_{0}^{\prime},\ldots,y_{N}^{\prime})$ is the eigenvector. The eigenvalue problem is numerically solved using the standard function dgeev of the linear algebra package Lapack.