Skip to main content
SpringerLink
Log in

From chaos to quasi-periodicity

  • Published:
Regular and Chaotic Dynamics Aims and scope Submit manuscript

Abstract

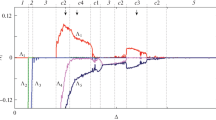
Ensembles of several Rössler chaotic oscillators are considered. It is shown that a typical phenomenon for such systems is the emergence of different and sufficiently high dimensional invariant tori. The possibility of a quasi-periodic Hopf bifurcation and a cascade of such bifurcations based on tori of increasing dimension is demonstrated. The domains of resonance tori are revealed. Boundaries of these domains correspond to the saddle-node bifurcations. Inside the domains of resonance modes, torus-doubling bifurcations and destruction of tori are observed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Heinrich, G., Ludwig, M., Qian, J., Kubala, B. and Marquardt, F., Collective Dynamics in Optomechanical Arrays, Phys. Rev. Lett., 2011, vol. 107, no. 4, 043603, 4 pp.

    Article  Google Scholar 

  2. Zhang, M., Wiederhecker, G. S., Manipatruni, S., Barnard, A., McEuen, P., and Lipson, M., Synchronization of Micromechanical Oscillators Using Light, Phys. Rev. Lett., 2012, vol. 109, no. 23, 233906, 5 pp.

    Article  Google Scholar 

  3. Temirbayev, A., Nalibayev, Ye.D., Zhanabaev, Z. Zh., Ponomarenko, V. I., and Rosenblum, M., Autonomous and Forced Dynamics of Oscillator Ensembles with Global Nonlinear Coupling: An Experimental Study, Phys. Rev. E, 2013, vol. 87, no. 6, 062917, 11 pp.

    Article  Google Scholar 

  4. Martens, E. A., Thutupalli, S., Fourrière, A., and Hallatschek, O., Chimera States in Mechanical Oscillator Networks, Proc. Natl. Acad. Sci., 2013, vol. 110, no. 26, pp. 10563–10567.

    Article  Google Scholar 

  5. Tinsley, M. R., Nkomo, S., and Showalter, K., Chimera and Phase-Cluster States in Populations of Coupled Chemical Oscillators, Nature Phys., 2012, vol. 8, no. 9, pp. 662–665.

    Article  Google Scholar 

  6. Vlasov, V. and Pikovsky, A., Synchronization of a Josephson Junction Array in Terms of Global Variables, Phys. Rev. E, 2013, vol. 88, no. 2, 022908, 5 pp.

    Article  Google Scholar 

  7. Lee, T. E. and Cross, M.C., Pattern Formation with Trapped Ions, Phys. Rev. Lett., 2011, vol. 106, no. 14, 143001, 4 pp.

    Article  Google Scholar 

  8. Lee, T.E. and Sadeghpour, H. R., Quantum Synchronization of Quantum van der Pol Oscillators with Trapped Ions, Phys. Rev. Lett., 2013, vol. 111, no. 23, 234101, 5 pp.

    Article  Google Scholar 

  9. Grebogi, C., Ott, E., and Yorke, J.A., Attractors on an N-torus: Quasiperiodicity Versus Chaos, Phys. D, 1985, vol. 15, no. 3, pp. 354–373.

    Article  MATH  MathSciNet  Google Scholar 

  10. Linsay, P. S. and Cumming, A.W., Three-Frequency Quasiperiodicity, Phase Locking, and the Onset of Chaos, Phys. D, 1989, vol. 40, no. 2, pp. 196–217.

    Article  MATH  MathSciNet  Google Scholar 

  11. Battelino, P.M., Grebogi, C., Ott, E. and Yorke, J.A., Chaotic Attractors on a 3-Torus, and Torus Break-Up, Phys. D, 1989, vol. 39, nos. 2–3, pp. 299–314.

    Article  MATH  MathSciNet  Google Scholar 

  12. Baesens, C., Guckenheimer, J., Kim, S., and MacKay, R. S., Three Coupled Oscillators: Mode-Locking, Global Bifurcations and Toroidal Chaos, Phys. D, 1991, vol. 49, no. 3, pp. 387–475.

    Article  MATH  MathSciNet  Google Scholar 

  13. Emelianova, Yu.P., Kuznetsov, A.P., Sataev, I.R., and Turukina, L. V., Synchronization and Multi-Frequency Oscillations in the Low-Dimensional Chain of the Self-Oscillators, Phys. D, 2013, vol. 244, no. 1, pp. 36–49.

    Article  MATH  Google Scholar 

  14. Kuznetsov, A.P., Kuznetsov, S.P., Sataev, I.R., and Turukina, L. V., About Landau — Hopf Scenario in a System of Coupled Self-Oscillators, Phys. Lett. A, 2013, vol. 377, nos. 45–48, pp. 3291–3295.

    Article  MATH  MathSciNet  Google Scholar 

  15. Emelianova, Yu.P., Kuznetsov, A.P., Turukina, L.V., Sataev, I.R., and Chernyshov, N.Yu., A Structure of the Oscillation Frequencies Parameter Space for the system of Dissipatively Coupled Oscillators, Commun. Nonlinear Sci. Numer. Simul., 2014, vol. 19, no. 4, pp. 1203–1212.

    Article  MathSciNet  Google Scholar 

  16. Li, X.-W. and Zheng, Zh.-G., Phase Synchronization of Coupled Rössler Oscillators: Amplitude Effect, Commun. Theor. Phys., 2007, vol. 47, no. 2, pp. 265–269.

    Article  Google Scholar 

  17. Pazó, D., Sánchez, E. and Matías, M. A., Transition to High-Dimensional Chaos through Quasiperiodic Motion, Internat. J. Bifur. Chaos Appl. Sci. Engrg., 2001, vol. 11, no. 10, pp. 2683–2688.

    Article  Google Scholar 

  18. Pazó, D. and Matías, M.A., Direct Transition to High-Dimensional Chaos through a Global Bifurcation, Europhys. Lett., 2005, vol. 72, no. 2, pp. 176–182

    Article  MathSciNet  Google Scholar 

  19. Rosenblum, M. G., Pikovsky, A. S., and Kurths, J., Phase Synchronization of Chaotic Oscillators, Phys. Rev. Lett., 1996, vol. 76, no. 11, pp. 1804–1807.

    Article  Google Scholar 

  20. Osipov, G.V., Pikovsky, A. S., Rosenblum, M. G., and Kurths, J., Phase Synchronization Effects in a Lattice of Nonidentical Rössler Oscillators, Phys. Rev. E, 1997, vol. 55, no. 3, pp. 2353–2361.

    Article  MathSciNet  Google Scholar 

  21. Pikovsky, A., Rosenblum, M., and Kurths, J., Synchronization: A Universal Concept in Nonlinear Sciences, New York: Cambridge Univ. Press, 2001.

    Book  Google Scholar 

  22. Vitolo, R., Broer, H., and Simó, C., Routes to Chaos in the Hopf-Saddle-Node Bifurcation for Fixed Points of 3D-Diffeomorphisms, Nonlinearity, 2010, vol. 23, no. 8, pp. 1919–1947.

    Article  MATH  MathSciNet  Google Scholar 

  23. Broer, H., Simó, C., and Vitolo, R., Hopf-Saddle-Node Bifurcation for Fixed Points of 3D-Diffeomorphisms: Analysis of a Resonance “bubble”, Phys. D, 2008, vol. 237, no. 13, pp. 1773–1799.

    Article  MATH  MathSciNet  Google Scholar 

  24. Broer, H., Simó, C., and Vitolo, R., The Hopf-Saddle-Node Bifurcation for Fixed Points of 3D-Diffeomorphisms: The Arnol’d Resonance Web, Bull. Belg. Math. Soc. Simon Stevin, 2008, vol. 15, no. 5, pp. 769–787.

    MATH  MathSciNet  Google Scholar 

  25. Vitolo, R., Broer, H., and Simó, C., Quasi-Periodic Bifurcations of Invariant Circles in Low-Dimensional Dissipative Dynamical Systems, Regul. Chaotic Dyn., 2011, vol. 16, nos. 1–2, pp. 154–184.

    Article  MATH  MathSciNet  Google Scholar 

  26. Landau, L. D., On the Problem of Turbulence, Dokl. Akad. Nauk SSSR, 1944, vol. 44, no. 8, pp. 339–342 (Russian).

    Google Scholar 

  27. Hopf, E., A Mathematical Example Displaying the Features of Turbulence, Comm. Pure Appl. Math., 1948, vol. 1, pp. 303–322.

    Article  MATH  MathSciNet  Google Scholar 

  28. Kuznetsov, S.P., Hyperbolic Chaos: A Physicist’s View, Berlin: Springer, 2012.

    Book  Google Scholar 

  29. Khibnik, A. I., Braiman, Y., Protopopescu, V., Kennedy, T.A.B., and Wiesenfeld, K., Amplitude Dropout in Coupled Lasers, Phys. Rev. A., 2000, vol. 62, no. 6, 063815, 5 pp.

    Article  Google Scholar 

  30. Khibnik, A. I., Braiman, Y., Kennedy, T.A. B., and Wiesenfeld, K., Phase Model Analysis of Two Lasers with Injected Field, Phys. D, 1998, vol. 111, nos. 1–4, pp. 295–310.

    Article  MATH  Google Scholar 

  31. Kazakov, A. O., Strange Attractors and Mixed Dynamics in the Problem of an Unbalanced Rubber Ball Rolling on a Plane, Regul. Chaotic Dyn., 2013, vol. 18, no. 5, pp. 508–520.

    Article  MATH  MathSciNet  Google Scholar 

  32. Gonchenko, A. S., Gonchenko, S.V., and Kazakov, A.O., Richness of Chaotic Dynamics in Nonholonomic Models of a Celtic Stone, Regul. Chaotic Dyn., 2013, vol. 18, no. 5, pp. 521–538.

    Article  MATH  MathSciNet  Google Scholar 

  33. Borisov, A.V., Kazakov, A.O., and Sataev, I.R., The Reversal and Chaotic Attractor in the Nonholonomic Model of Chaplygin’s Top, Regul. Chaotic Dyn., 2014, vol. 19, no. 6, pp. 718–733.

    Article  MathSciNet  Google Scholar 

  34. Borisov, A.V., Jalnine, A.Yu., Kuznetsov, S.P., Sataev, I.R., and Sedova, J.V., Dynamical Phenomena Occurring due to Phase Volume Compression in Nonholonomic Model of the Rattleback, Regul. Chaotic Dyn., 2012, vol. 17, no. 6, pp. 512–532.

    Article  MATH  MathSciNet  Google Scholar 

  35. Afraimovich, V. S., Gonchenko, S.V., Lerman, L.M., Shilnikov, A. L., and Turaev, D. V., Scientific Heritage of L.P. Shilnikov, Regul. Chaotic Dyn., 2014, vol. 19, no. 4, pp. 435–460.

    Article  MATH  MathSciNet  Google Scholar 

  36. Jalnine, A.Yu., Generalized Synchronization of Identical Chaotic Systems on the Route from an Independent Dynamics to the Complete Synchrony, Regul. Chaotic Dyn., 2013, vol. 18, no. 3, pp. 214–225.

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Saratov Branch, Kotel’nikov Institute of Radio Engineering and Electronics of RAS, ul. Zelenaya 38, Saratov, 410019, Russia

    Alexander P. Kuznetsov, Igor R. Sataev, Yuliya V. Sedova & Ludmila V. Turukina

  2. Saratov State University, ul. Astrakhanskaya 83, Saratov, 410012, Russia

    Alexander P. Kuznetsov, Natalia A. Migunova & Ludmila V. Turukina

Authors
  1. Alexander P. Kuznetsov
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Natalia A. Migunova
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Igor R. Sataev
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Yuliya V. Sedova
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Ludmila V. Turukina
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Alexander P. Kuznetsov.

Additional information

This is a translation of the paper by A.P. Kuznetsov, N.A. Migunova, I.R. Sataev, Yu.V. Sedova, and L.V. Turukina “Dynamics of Coupled Chaotic Oscillators: from Chaos to Quasiperiodicity”, Nelin. Dinam., 2014, vol. 10, no. 4, pp. 387–405, which was published previously only in the Russian language.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kuznetsov, A.P., Migunova, N.A., Sataev, I.R. et al. From chaos to quasi-periodicity. Regul. Chaot. Dyn. 20, 189–204 (2015). https://doi.org/10.1134/S1560354715020070

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S1560354715020070

MSC2010 numbers

Keywords

Search

Navigation