Skip to main content
SpringerLink
Log in

Method for Forming Autorotations in Controllable Mechanical System with Two Degrees of Freedom

  • SYSTEMS THEORY AND GENERAL CONTROL THEORY
  • Published:
Journal of Computer and Systems Sciences International Aims and scope

Abstract

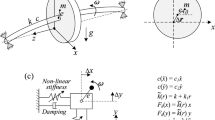
An autonomous mechanical system with two rotational degrees of freedom, undergoing the action of conservative and nonconservative forces, is considered. The corresponding dynamical system contains changeable parameters that can be treated as amplifying coefficients for control actions. It is required to select the values of these parameters in order to form an autorotation mode in the system that possesses the prescribed properties. We propose an iterative search method for the corresponding values of the parameters. This approach is a modification of the Andronov–Pontryagin method and, unlike the latter, it does not assume that there is a small parameter in the system. We provide an example of the application of the proposed method to a model of a wind turbine: the value of the parameter, ensuring the existence of a mode with a high value of the mechanical power trapped from the flow, is selected. In the dynamical system, an attractor that is a trajectory close to a periodic one corresponds to this mode.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1.
Fig. 2.
Fig. 3.
Fig. 4.
Fig. 5.

Similar content being viewed by others

REFERENCES

  1. A. A. Andronov, “Les cycles limites de Poincare et la theorie des oscillations auto-entretenues,” C. R. Acad. Sci. 189, 559–561 (1929).

    Google Scholar 

  2. A. A. Andronov, E. A. Leontovich, I. I. Gordon, and A. G. Maier, Theory of Bifurcations of Dynamic Systems on a Plane (Nauka, Moscow, 1967; Israel Program for Scientific Transl., Jerusalem, 1971).

  3. L. D. Akulenko, D. D. Leshchenko, and F. L. Chernous’ko, “ Fast motion of a heavy rigid body aboud a fixed point in a resistive medium,” Izv. Akad. Nauk, Mekh. Tverd. Tela, No. 3, 5–13 (1982).

    Google Scholar 

  4. E. S. Shalimova, “Steady and periodic modes in the problem of motion of a heavy material point on a rotating sphere (the viscous friction case),” Mosc. Univ. Mech. Bull. 69 (4), 89 (2014).

    Article  Google Scholar 

  5. Yu. D. Selyutskiy, “Dynamics of an elastically mounted wing in flow,” AIP Conf. Proc. 2046, 020085-1 (2018).

    Article  Google Scholar 

  6. M. Z. Dosaev, L. A. Klimina, B. Ya. Lokshin, Yu. D. Selyutskii, and E. S. Shalimova, “On the autorotation modes of a double-rotor Darrieus wind turbine,” Mech. Solids, No. 1 (2021, in press).

  7. L. A. Klimina, “Method for constructing periodic solutions of a controlled dynamic system with a cylindrical phase space,” J. Comput. Syst. Sci. Int. 59, 139 (2020).

    Article  Google Scholar 

  8. V. M. Volosov, “The method of averaging,” Dokl. Akad. Nauk SSSR 137, 21–24 (1961).

    MathSciNet  MATH  Google Scholar 

  9. R. E. Kondrashov and A. D. Morozov, “On global behaviour of the solutions of system of two Duffing—Van der Pole equations,” Nelin. Dinam. 7, 437–449 (2011).

    Article  Google Scholar 

  10. K. Kamiyama, M. Komuro, and T. Endo, “Bifurcation of quasi-periodic oscillations in mutually coupled hard-type oscillators: Demonstration of unstable quasi-periodic orbits,” Int. J. Bifurc. Chaos 22, 1230022 (2012).

    Article  Google Scholar 

  11. F. Schilder, H. M. Osinga, and W. Vogt, “Continuation of quasi-periodic invariant tori,” SIAM J. Appl. Dynam. Syst. 4, 459–488 (2005).

    Article  MathSciNet  Google Scholar 

  12. T. S. Parker and L. O. Chua, Practical Numerical Algorithms for Chaotic Systems (Springer, New York, 1989).

    Book  Google Scholar 

  13. Chr. Kaas-Petersen, “Computation of quasiperiodic solutions of forced dissipative systems,” J. Comput. Phys. 58, 395–408 (1985).

  14. N. N. Bogolyubov, Yu. A. Mitropol’skii, and A. M. Samoilenko, The Method of Accelerated Convergence in Nonlinear Mechanics (Nauk. Dumka, Kiev, 1969) [in Russian].

    Google Scholar 

  15. J. Bush, M. Gameiro, S. Harker, H. Kokubu, K. Mischaikow, I. Obayashi, and P. Pilarczyk, “Combinatorial-topological framework for the analysis of global dynamics,” Chaos 22, 047508 (2012).

    Article  MathSciNet  Google Scholar 

  16. K. Kamiyama, M. Komuro, and T. Endo, “Algorithms for obtaining a saddle torus between two attractors,” Int. J. Bifurc. Chaos 23, 1330032 (2013).

    Article  MathSciNet  Google Scholar 

  17. B. Zhou, F. Thouverez, and D. Lenoir, “A variable-coefficient harmonic balance method for the prediction of quasi-periodic response in nonlinear systems,” Mech. Syst. Signal Process. 64, 233–244 (2015).

    Article  Google Scholar 

  18. G. Chen and J. F. Dunne, “A fast continuation scheme for accurate tracing of nonlinear oscillator frequency response functions,” J. Sound Vibr. 385, 284–299 (2016).

    Article  Google Scholar 

  19. A. M. Samoilenko, “Numerical analytical method of investigating periodic systems of ordinary differential equations. I,” Ukr. Mat. Zh. 17 (04), 82–93 (1965).

    Article  Google Scholar 

  20. A. A. Grin’ and S. V. Rudevich, “Dulac–Cherkas test for determining the exact number of limit cycles of autonomous systems on the cylinder,” Differ. Equat. 55, 319 (2019).

    Article  MathSciNet  Google Scholar 

  21. L. S. Pontryagin, “On dynamical systems close to Hamiltonian systems,” Zh. Eksp. Teor. Fiz. 4 (9), 883–885 (1934).

    Google Scholar 

  22. L. A. Cherkas, A. A. Grin’, and V. I. Bulgakov, Constructive Methods for Studying Limit Cycles of Second-Order Autonomous Systems (Numerical-Algebraic Approach) (GrGU, Grodno, 2013) [in Russian].

  23. A. M. Formalskii, Stabilization and Motion Control of Unstable Objects (Walter de Gruyter, Berlin, Boston, 2015).

    Book  Google Scholar 

  24. A. S. Kravets, Characteristics of Aviation Profiles (Gos. Izd. Oboron. Prom-sti, Moscow, 1939) [in Russian].

    Google Scholar 

  25. M. Z. Dosaev, V. A. Samsonov, Yu. D. Selyutskii, Wen-Lung Lu, and Ching-Huei Lin, “Bifurcation of operation modes of small wind power stations and optimization of their characteristics,” Mech. Solids 44, 214 (2009).

    Article  Google Scholar 

  26. L. A. Klimina and E. S. Shalimova, “Dual-propeller wind turbine with a differential planetary gear,” Mekhatron. Avtomatiz. Upravl. 18, 679–684 (2017).

    Article  Google Scholar 

  27. M. V. Ishkhanyan and L. A. Klimina, “Wind turbine of the Savonius–Magnus type with conical blades: Dynamics and control,” J. Comput. Syst. Sci. Int. 59 (4), 630–638 (2020).

  28. M. Z. Dosaev, L. A. Klimina, Yu. D. Selyutskiy, and E. S. Shalimova, “Twin wind turbine powered by Magnus effect,” Vestn. Mosk. Univ., Ser. 1: Mat., Mekh., No. 4, 65–69 (2020).

  29. L. A. Klimina, “Method for finding periodic trajectories of centrally symmetric dynamical systems on the plane,” Differ. Equations 55, 159 (2019).

    Article  MathSciNet  Google Scholar 

  30. L. A. Klimina and Yu. D. Selyutskiy, “Method to construct periodic solutions of controlled second-order dynamical systems,” J. Comput. Syst. Sci. Int. 58, 503 (2019).

    Article  Google Scholar 

Download references

Funding

This study is supported by the Russian Foundation for Basic Research (grant no. 18-31-20029).

Author information

Authors and Affiliations

  1. Institute of Mechanics, Lomonosov Moscow State University, Moscow, Russia

    L. A. Klimina

Authors
  1. L. A. Klimina
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to L. A. Klimina.

Additional information

Translated by A. Muravnik

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Klimina, L.A. Method for Forming Autorotations in Controllable Mechanical System with Two Degrees of Freedom. J. Comput. Syst. Sci. Int. 59, 817–827 (2020). https://doi.org/10.1134/S1064230720060064

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S1064230720060064

Search

Navigation