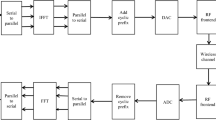
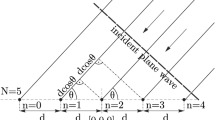
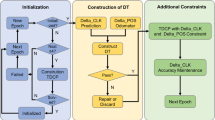
APPENDIX
A.
PSD of the Modulation Term for the Proposed Sine GBOC Modulations
Based on Euler’s formula and defining \(A = \frac{{2\pi f}}{{n{{f}_{{\text{c}}}}}}\), expression (17) can be rewritten as
$${{G}_{{{\text{mod}}}}}\left( f \right) = n + \left[ {\sum\limits_{i = 1}^{n - 1} {\left( {n - i - 2} \right)\left( {{\text{exp(}}ijA{\text{)}} + {\text{exp(}}{\kern 1pt} - {\kern 1pt} ijA{\text{)}}} \right)} } \right] = n + \Phi \left( {jA} \right) + \Phi \left( { - jA} \right),$$
(A.1)
where
$$\begin{gathered} \Phi \left( {jA} \right) = \left( {n - i} \right)\sum\limits_{i = 1}^{n - 1} {{\text{exp(}}ijA{\text{)}}} - 2\sum\limits_{i = 1}^{n - 1} {{\text{exp(}}ijA{\text{)}}} \\ = \frac{{\left( {n - 1} \right){\text{exp(}}jA{\text{)}} + \exp [j\left( {n + 1} \right)A] - n{\text{exp(2}}ijA{\text{)}}}}{{{{{\left( {{\text{exp(}}jA{\text{)}} - 1} \right)}}^{2}}}} - 2\frac{{{\text{exp}}(njA) - {\text{exp(}}jA{\text{)}}}}{{{\text{exp(}}jA{\text{)}} - 1}}, \\ \end{gathered} $$
(A.2)
and
$$\begin{gathered} \Phi \left( { - jA} \right) = \left( {n - i} \right)\sum\limits_{i = 1}^{n - 1} {{\text{exp(}} - ijA{\text{)}}} - 2\sum\limits_{i = 1}^{n - 1} {{\text{exp(}} - ijA{\text{)}}} \\ = \frac{{\left( {n - 1} \right)\exp ( - jA) + \exp [ - \left( {n + 1} \right)jA] - n\exp ( - j2A)}}{{{{{\left( {\exp ( - jA) - 1} \right)}}^{2}}}} - 2\frac{{\exp ( - njA) - \exp ( - jA)}}{{\exp ( - jA) - 1}}\,. \\ \end{gathered} $$
(A.3)
Based on expressions (A.1), (A.2) and (A.3), the modulating term \({{G}_{{{\text{mod}}}}}\left( f \right)\) is
$$\begin{gathered} {{G}_{{{\text{mod}}}}}\left( f \right) = n + \frac{{\left( {n - 3} \right)\exp (jA) - \exp [\left( {n + 1} \right)jA] - \left( {n - 2} \right)\exp (2jA) + 2\exp (njA)}}{{{{{\left( {\exp (jA) - 1} \right)}}^{2}}}} \hfill \\ + \,\,\frac{{\left( {n - 3} \right)\exp ( - jA) - \exp [ - \left( {n + 1} \right)jA] - \left( {n - 2} \right)\exp ( - 2jA) + 2\exp ( - njA)}}{{{{{\left( {\exp ( - jA) - 1} \right)}}^{2}}}}. \hfill \\ \end{gathered} $$
(A.4)
Simplifying expressions (A.4) using Euler’s formula yields
$${{G}_{{{\text{mod}}}}}\left( f \right) = \frac{{16n{{{\sin }}^{4}}\left( {\frac{A}{2}} \right) + 2\left( \begin{gathered} - 8n{{\sin }^{4}}\left( {\frac{A}{2}} \right) + 4{{\sin }^{2}}\left( {\frac{A}{2}} \right) + 16{{\sin }^{4}}\left( {\frac{A}{2}} \right) \\ - \,\,8{{\sin }^{3}}\left( {\frac{A}{2}} \right)\sin \left( {\frac{{2n - 1}}{2}A} \right) - 4{{\sin }^{2}}\left( {\frac{A}{2}} \right)\cos \left[ {\left( {n - 1} \right)A} \right] \\ \end{gathered} \right)}}{{16{{{\sin }}^{4}}\left( {\frac{A}{2}} \right)}}.$$
(A.5)
and its simplest version is:
$${{G}_{{{\text{mod}}}}}\left( f \right) = \frac{{{{{\cos }}^{2}}\left( {\frac{n}{2}A} \right) + 2{{{\sin }}^{2}}\left( {\frac{A}{2}} \right) - \cos \left[ {\left( {n - 1} \right)A} \right]}}{{{{{\sin }}^{2}}\left( {\frac{A}{2}} \right)}}.$$
(A.6)
B.
Proof of Consistency of the Traditional and the Proposed GBOC Modulations
Again, we define \(A = \frac{{2\pi f}}{{n{{f}_{{\text{c}}}}}}\), expression (20) can be rewritten as
$${{G}_{{{\text{GBO}}{{{\text{C}}}_{{\sin }}}\left( {{{f}_{{\text{s}}}}{\text{,}}{{f}_{{\text{c}}}}} \right)}}}\left( f \right) = \frac{{{{f}_{{\text{c}}}}}}{{{{{\left( {\pi f} \right)}}^{2}}}}\left\{ {{{{\sin }}^{2}}\left( {\frac{n}{2}A} \right) - \sin \left( {nA} \right)\sin \left[ {\left( {n - 1} \right)A} \right] + 4{{{\sin }}^{2}}\left( {\frac{{n - 1}}{2}A} \right){{{\cos }}^{2}}\left( {\frac{n}{2}A} \right)} \right\}.$$
(B.1)
After a series of trigonometric transformations yields
$$\begin{gathered} {{G}_{{{\text{GBO}}{{{\text{C}}}_{{\sin }}}\left( {{{f}_{{\text{s}}}},{{f}_{{\text{c}}}}} \right)}}}\left( f \right) = \frac{{{{f}_{{\text{c}}}}}}{{{{{\left( {\pi f} \right)}}^{2}}}}\left[ {{{{\sin }}^{2}}\left( {\frac{n}{2}A} \right) + \cos \left( {nA} \right) - \cos \left( {\left( {n - 1} \right)A} \right) + 2{{{\sin }}^{2}}\left( {\frac{A}{2}} \right)} \right] \\ = \frac{{{{f}_{{\text{c}}}}}}{{{{{\left( {\pi f} \right)}}^{2}}}}\left[ {{{{\cos }}^{2}}\left( {\frac{n}{2}A} \right) - \cos \left( {\left( {n - 1} \right)A} \right) + 2{{{\sin }}^{2}}\left( {\frac{A}{2}} \right)} \right]\,\,. \\ \end{gathered} $$
(B.2)
Also, expression (19) can be rewritten as
$$\begin{gathered} {{G}_{{{\text{GBOC}}\left( {{{{\left\{ {{{s}_{i}}} \right\}}}_{{\chi = 2}}}{\text{, }}{{f}_{{\text{c}}}}} \right)}}}\left( f \right) = \frac{{{{f}_{{\text{c}}}}}}{{{{{\left( {\pi f} \right)}}^{2}}}} \\ \times \,\,\left[ {{{{\cos }}^{2}}\left( {\frac{n}{2}A} \right) + 2{{{\sin }}^{2}}\left( {\frac{A}{2}} \right) - \cos \left( {\left( {n - 1} \right)A} \right)} \right]. \\ \end{gathered} $$
(B.3)
As we can see from expressions (B.2) and (B.3), \({{G}_{{{\text{GBOC}}\left( {{{{\left\{ {{{s}_{i}}} \right\}}}_{{\chi = 2}}}{\text{, }}{{f}_{{\text{c}}}}} \right)}}}\left( f \right)\) is equal to \({{G}_{{{\text{GBO}}{{{\text{C}}}_{{\sin }}}\left( {{{f}_{{\text{s}}}},{{f}_{{\text{c}}}}} \right)}}}\left( f \right)\).
C.
PSD of the Modulation Term for the Proposed Cosine GBOC Modulations
Similar to sine GBOC modulations, the PSD of the modulation term, expression (21) can be rewritten as
$$\begin{gathered} {{G}_{{{\text{mod}}}}}\left( f \right) = n + 2\cos \left[ {\left( {n - 1} \right)A} \right] + \left[ {\sum\limits_{i = 1}^{n - 2} {\left( {n - i - 4} \right)\left( {{\text{exp(}}ijA{\text{)}} + \exp ( - ijA)} \right)} } \right] = n + 8\cos \left[ {\left( {n - 1} \right)A} \right] \\ + \,\,\left[ {\sum\limits_{i = 1}^{n - 1} {\left( {n - i - 4} \right)\left( {{\text{exp(}}ijA{\text{)}} + \exp ( - ijA)} \right)} } \right] = n + 8\cos \left[ {\left( {n - 1} \right)A} \right] + \Phi \left( {jA} \right) + \Phi \left( { - jA} \right)\,, \\ \end{gathered} $$
(C.1)
where
$$\begin{gathered} \Phi \left( {jA} \right) = \left( {n - i} \right)\sum\limits_{i = 1}^{n - 1} {{\text{exp(}}ijA{\text{)}}} - 4\sum\limits_{i = 1}^{n - 1} {{\text{exp(}}ijA{\text{)}}} \\ = \frac{{\left( {n - 1} \right)\exp (jA) + \exp [j\left( {n + 1} \right)A] - n\exp (2jA)}}{{{{{\left( {\exp (jA) - 1} \right)}}^{2}}}} - 4\frac{{\exp (njA) - \exp (jA)}}{{\exp (jA) - 1}} \\ \end{gathered} $$
(C.2)
and
$$\begin{gathered} \Phi \left( { - jA} \right) = \left( {n - i} \right)\sum\limits_{i = 1}^{n - 1} {{\text{exp(}} - ijA{\text{)}}} - 4\sum\limits_{i = 1}^{n - 1} {{\text{exp(}} - ijA{\text{)}}} \\ = \frac{{\left( {n - 1} \right)\exp ( - jA) + \exp [ - \left( {n + 1} \right)jA] - n\exp ( - 2jA)}}{{{{{\left( {\exp ( - jA) - 1} \right)}}^{2}}}} - 4\frac{{\exp ( - njA) - \exp ( - jA)}}{{\exp ( - jA) - 1}}. \\ \end{gathered} $$
(C.3)
From expressions (C.1), (C.2) and (C.3), the modulating term \({{G}_{{{\text{mod}}}}}\left( f \right)\) can be expressed as
$$\begin{gathered} {{G}_{{{\text{mod}}}}}\left( f \right) = n + 8\cos \left[ {\left( {n - 1} \right)A} \right] \\ + \,\,\frac{{\left( {n - 5} \right)\exp (jA) - 3\exp [\left( {n + 1} \right)jA] - \left( {n - 4} \right)\exp (2jA) + 4\exp (njA)}}{{{{{\left( {\exp (jA) - 1} \right)}}^{2}}}} \\ + \,\,\frac{{\left( {n - 5} \right)\exp ( - jA) - 3\exp [ - \left( {n + 1} \right)jA] - \left( {n - 4} \right)\exp ( - 2jA) + 4\exp ( - njA)}}{{{{{\left( {\exp (jA) - 1} \right)}}^{2}}}}. \\ \end{gathered} $$
(C.4)
Again, simplifying expressions (C.4) using Euler’s formula yields
$${{G}_{{{\text{mod}}}}}\left( f \right) = \frac{\begin{gathered} 16n{{\sin }^{4}}\left( {\frac{A}{2}} \right) + 8\cos \left[ {\left( {n - 1} \right)A} \right]16{{\sin }^{4}}\left( {\frac{A}{2}} \right) \\ + \,\,2\left( \begin{gathered} - 8n{{\sin }^{4}}\left( {\frac{A}{2}} \right) + 4{{\sin }^{2}}\left( {\frac{A}{2}} \right) + 32{{\sin }^{4}}\left( {\frac{A}{2}} \right) \\ - 24{{\sin }^{3}}\left( {\frac{A}{2}} \right)\sin \left( {\frac{{2n - 1}}{2}A} \right) - 4{{\sin }^{2}}\left( {\frac{A}{2}} \right)\cos \left[ {\left( {n - 1} \right)A} \right] \\ \end{gathered} \right) \\ \end{gathered} }{{16{{{\sin }}^{4}}\left( {\frac{A}{2}} \right)}}$$
(C.5)
(C.5) can be further simplified as
$${{G}_{{{\text{mod}}}}}\left( f \right) = \frac{{16{{{\sin }}^{2}}\left( {\frac{A}{2}} \right)\cos \left[ {\left( {n - 1} \right)A} \right] + 1 + 8{{{\sin }}^{2}}\left( {\frac{A}{2}} \right) + 3\cos \left( {nA} \right) - 4\cos \left[ {\left( {n - 1} \right)A} \right]}}{{2{{{\sin }}^{2}}\left( {\frac{A}{2}} \right)}}.$$
(C.6)
D.
Proof of Consistency of the Traditional and the Proposed GBOC Modulations
Defining \(A = \frac{{2\pi f}}{{n{{f}_{{\text{c}}}}}}\), expression (24) can be rewritten as
$${{G}_{{{\text{GBO}}{{{\text{C}}}_{{\cos }}}\left( {{{f}_{{\text{s}}}},{{f}_{{\text{c}}}}} \right)}}}\left( f \right) = \frac{{{{f}_{{\text{c}}}}}}{{{{{\left( {\pi f} \right)}}^{2}}}}\left\{ {{{{\sin }}^{2}}\left( {\frac{n}{2}A} \right) + 4{{{\sin }}^{2}}\left[ {\left( {\frac{{n - 2}}{n}} \right)A} \right] - 4\sin \left( {\frac{n}{2}A} \right)\sin \left[ {\left( {\frac{{n - 2}}{n}} \right)A} \right]} \right\}.$$
(D.1)
The trigonometric transformation of expression (D.1) yields
$${{G}_{{{\text{GBO}}{{{\text{C}}}_{{\cos }}}\left( {{{f}_{{\text{s}}}},{{f}_{{\text{c}}}}} \right)}}}\left( f \right) = \frac{{{{f}_{{\text{c}}}}}}{{{{{\left( {\pi f} \right)}}^{2}}}}\left\{ {\frac{1}{2} - \frac{1}{2}\cos \left( {nA} \right) + 2 - 2\cos \left[ {\left( {n - 2} \right)A} \right] + 2\cos \left[ {\left( {n - 1} \right)A} \right] - 2\cos \left( A \right)} \right\}.$$
(D.2)
Transforming expression (23) by trigonometric functions yields
$$\begin{gathered} {{G}_{{{\text{GBOC}}\left( {{{{\left\{ {{{s}_{i}}} \right\}}}_{{\chi = 2}}}{\text{, }}{{f}_{{\text{c}}}}} \right)}}}\left( f \right) = \frac{{{{f}_{{\text{c}}}}}}{{{{{\left( {\pi f} \right)}}^{2}}}}\frac{{\left\{ {16{{{\sin }}^{2}}\left( {\frac{n}{2}A} \right)\cos \left[ {\left( {n - 1} \right)A} \right] + 1 + 8{{{\sin }}^{2}}\left( {\frac{A}{2}} \right) + 3\cos \left( {nA} \right) - 4\cos \left[ {\left( {n - 1} \right)A} \right]} \right\}}}{2} \\ = \frac{{{{f}_{{\text{c}}}}}}{{{{{\left( {\pi f} \right)}}^{2}}}}\frac{{\left\{ {4\cos \left[ {\left( {n - 1} \right)A} \right] + 1 + 4 - 4\cos \left( A \right) + 3\cos \left( {nA} \right) - 8\cos \left( A \right)\cos \left[ {\left( {n - 1} \right)A} \right]} \right\}}}{2}\,. \\ \end{gathered} $$
(D.3)
Finally, the PSD of the proposed GBOC modulations is
$${{G}_{{{\text{GBOC}}\left( {{{{\left\{ {{{s}_{i}}} \right\}}}_{{\chi = 2}}}{\text{, }}{{f}_{{\text{c}}}}} \right)}}}\left( f \right) = \frac{{{{f}_{{\text{c}}}}}}{{{{{\left( {\pi f} \right)}}^{2}}}}\left\{ {\frac{1}{2} - \frac{1}{2}\cos \left( {nA} \right) + 2 - 2\cos \left[ {\left( {n - 2} \right)A} \right] + 2\cos \left[ {\left( {n - 1} \right)A} \right] - 2\cos \left( A \right)} \right\}.$$
(D.4)
Again, the expressions (D.2) and (D.4) are equivalent.