Skip to main content
SpringerLink
Log in

Gradient-Stable Algorithm for the Dynamics of the Phase Field in Modeling of Directional Solidification in Si–As

  • Published:
Technical Physics Aims and scope Submit manuscript

Abstract

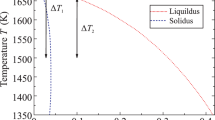
The main provisions of the phase-field method for concentrated solutions and the problems of numerical modeling of a thermodynamically consistent model of the dynamics of the order parameter for phase transformations of the first kind in the framework of the phase-field approach are briefly considered. The model is based on extended irreversible thermodynamics, which uses Gibbs potentials of real systems to describe the state of the system. Numerical algorithms for modeling phase-field equations for both locally equilibrium and locally nonequilibrium models of relaxation dynamics are derived from the gradient-stability condition, which guarantees a decrease in the free energy of the system during relaxation. The estimates of the eigenvalues of the Hessian matrix in the lattice space allow us to construct a gradient-stable algorithm for modeling the time evolution of the phase-field equations in real thermodynamic systems, despite the existing differences in Gibbs energies. The paper analyzes gradient-stable numerical schemes for stability to small deviations for phase field equations in the Fourier approximation. The numerical calculations performed for the SiAs solution demonstrate the stability of the obtained algorithm both with an increase in the time step by several orders of magnitude, and with an increase in the driving forces due to severe supercooling of the system.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1.
Fig. 2.
Fig. 3.
Fig. 4.

Similar content being viewed by others

REFERENCES

  1. I. Singer-Loginova and H. M. Singer, Rep. Prog. Phys. 71, 106501 (2008). https://doi.org/10.1088/0034-4885/71/10/106501

  2. H. Emmerich, Adv. Phys. 57 (1), 1 (2008). https://doi.org/10.1080/00018730701822522

    Article  ADS  Google Scholar 

  3. D. Herlach, P. Galenko, and D. Holland-Moritz, Metastable Solids from Undercooled Melts (Elsevier, Burlington, MA, 2006).

    Google Scholar 

  4. T. M. Rogers, K. R. Elder, and R. C. Desai, Phys. Rev. B 37, 9638 (1988). https://doi.org/10.1007/978-94-011-2540-6_9

    Article  ADS  Google Scholar 

  5. B. P. Vollmayer-Lee and A. D. Rutenberg, Phys. Rev. E 68, 066703 (2003). https://doi.org/10.1103/PhysRevE.68.066703

  6. http://cpddb.nims.go.jp/cpddb/periodic.htm. Accessed March 25, 2021.

  7. D. J. Eyre, Preprint “An unconditionally stable one-step scheme for gradient systems” (1997). http://www.math.utah.edu/eyre/research/methods/stable.ps

  8. D. J. Eyre, in Computational and Mathematical Models of Microstructural Evolution, Ed. by J. W. Bullard (Mater. Res. Soc., Warrendale, PA, 1998), p. 39.

    Google Scholar 

  9. M. Cheng and J. A. Warren, Phys. Rev. E 75, 017702 (2007). https://doi.org/10.1103/PhysRevE.75.017702

  10. M. Cheng and J. A. Warren, J. Comput. Phys. 227, 6241 (2008). https://doi.org/10.1016/j.jcp.2008.03.012

    Article  ADS  MathSciNet  Google Scholar 

  11. M. Cheng, S. Cottenier, and H. Emmerich, arXiv:0807.3579v2 [cond-mat.mtrl-sci] (August 6, 2009).

  12. J. W. Choi, H. G. Lee, D. Jeong, and J. Kim, Phys. A 388 (9), 1791 (2009). https://doi.org/10.1016/j.physa.2009.01.026

    Article  MathSciNet  Google Scholar 

  13. V. Lebedev, A. Sysoeva, and P. Galenko, Phys. Rev. E 83, 026705 (2011). https://doi.org/10.1103/PhysRevE.83.026705

  14. P. K. Galenko, V. G. Lebedev, and A. A. Sysoeva, Comput. Math. Math. Phys. 51, 1074 (2011). https://doi.org/10.1134/S0965542511060078

    Article  MathSciNet  Google Scholar 

  15. I. Steinbach, L. Zhang, and M. Plapp, Acta Mater. 60, 2689 (2012). https://doi.org/10.1016/j.actamat.2012.01.035

    Article  ADS  Google Scholar 

  16. H. F. Wang, P. K. Galenko, X. Zhang, W. W. Kuang, F. Liu, and D. M. Herlach, Acta Mater. 90, 982 (2015). https://doi.org/10.1016/j.actamat.2015.02.021

    Article  Google Scholar 

  17. H. F. Wang, W. W. Kuang, Z. Xiao, and F. Liu, J. Mater. Sci. 50, 1277 (2015). https://doi.org/10.1007/s10853-014-8686-1

    Article  ADS  Google Scholar 

  18. X. Zhang, H. F. Wang, W. W. Kuang, and J. B. Zhang, Acta Mater. 128, 258 (2017). https://doi.org/10.1016/j.actamat.2017.02.026

    Article  ADS  Google Scholar 

  19. V. G. Lebedev and P. K. Galenko, Russ. Metall. (Metally) 8, 785 (2016). https://doi.org/10.1134/S0036029516080097

    Article  ADS  Google Scholar 

  20. http://web.micress.de/. Accessed March 10, 2021.

  21. S. M. Allen and J. W. Cahn, Acta Metall. 27, 1085 (1979). https://doi.org/10.1016/0001-6160(79)90196-2

    Article  Google Scholar 

  22. D. Kessler, J. Cryst. Growth 224 (1–2), 175 (2001). https://doi.org/10.1016/S0022-0248(01)00814-4

    Article  ADS  Google Scholar 

  23. P. Galenko and D. Jou, Phys. Rev. E 71, 046125 (2005). https://doi.org/10.1103/PhysRevE.71.046125

  24. J. W. Cahn and J. E. Hilliard, J. Chem. Phys. 28, 258 (1958). https://doi.org/10.1063/1.1744102

    Article  ADS  Google Scholar 

  25. V. G. Lebedev, E. V. Abramova, D. A. Danilov, and P. K. Galenko, Int. J. Mater. Res. 101 (4), 473 (2010). https://doi.org/10.3139/146.110297

    Article  Google Scholar 

  26. P. K. Galenko, E. V. Abramova, D. Jou, D. A. Danilov, V. G. Lebedev, and D. M. Herlach, Phys. Rev. E 84, 041143 (2011). https://doi.org/10.1103/PhysRevE.84.041143

  27. S. Li, J. Zhang, and P. Wu, J. Cryst. Growth 312, 982 (2010). https://doi.org/10.1016/j.jcrysgro.2009.12.070

    Article  ADS  Google Scholar 

  28. P. K. Galenko and M. D. Krivilyov, Modell. Simul. Mater. Sci. Eng. 8, 67 (2000). https://doi.org/10.1088/0965-0393/8/1/306

    Article  ADS  Google Scholar 

  29. B. Bӧttger, M. Apel, M. Budnitzki, J. Eiken, G. Laschet, and B. Zhou, Comput. Mater. Sci. 184, 109909 (2020). https://doi.org/10.1016/j.commatsci.2020.109909

Download references

ACKNOWLEDGMENTS

The authors are grateful to A.V. Obukhov for a number of valuable remarks on numerical modeling.

Funding

This study was supported in part by the Russian Foundation for Basic Research and by the administration of the Usmurt Republic (research project no. 18-42-180002).

Author information

Authors and Affiliations

  1. Udmurt State University, Izhevsk, Russia

    V. G. Lebedev, A. A. Obukhov & V. P. Bovin

  2. Udmurt Federal Research Center, Ural Brunch, Russian Academy of Sciences, 426067, Izhevsk, Russia

    V. G. Lebedev & V. I. Lad’yanov

Authors
  1. V. G. Lebedev
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. A. A. Obukhov
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. V. P. Bovin
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. V. I. Lad’yanov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to V. G. Lebedev.

Additional information

Translated by N. Wadhwa

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Lebedev, V.G., Obukhov, A.A., Bovin, V.P. et al. Gradient-Stable Algorithm for the Dynamics of the Phase Field in Modeling of Directional Solidification in Si–As. Tech. Phys. 67, 700–706 (2022). https://doi.org/10.1134/S1063784222100048

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S1063784222100048

Keywords:

Search

Navigation