Abstract
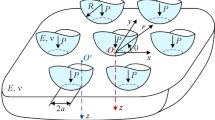
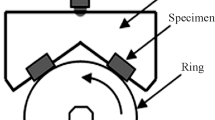
This study presents modeling results on fatigue wear of elastomers. A contact problem solution has been derived for the sliding of a system of asperities over a viscoelastic half-space. The mechanical properties of the viscoelastic half-space are described by relations between stresses and strains given by the Volterra integral operator. The contact problem is solved by the boundary element method using an iterative procedure. Stresses in the subsurface layers of the viscoelastic material are analyzed. The damage function of the surface layer is calculated using a reduced stress criterion, the parameters of which are determined on the basis of available experimental data. The wear process is studied under the assumption that the accumulated damage can be summed up. Within the applied frictional interaction model, the wear process presents the delamination of material surface layers of finite thickness at discrete points in time and continuous surface wear by fatigue mechanism. A model calculation of contact fatigue damage accumulation has shown that the time to the first material delamination (incubation period) depends on the sliding velocity and the viscoelastic properties of the material. By analyzing the dependence of the wear rate on the input parameters of the problem, it was investigated how the sliding velocity affects the time of fatigue damage initiation and the run-in and steady-state wear rates in materials with different rheological properties. Model calculations revealed that the wear rate of material surface layers after the incubation period increases smoothly and then stabilizes. The presence of the steady-state wear rate agrees well with experimental data. The developed method for studying fatigue damage accumulation in the surface layers of viscoelastic materials in frictional interaction can also be applied on the macrolevel to determine possible crack initiation sites.
Similar content being viewed by others
References
Ratner, S.B., Wear of Polymers as a Fatigue Fracture Process, in Theory of Friction and Wear, Ratner, S.B., Klitenik, G.S., and Lurie, E.G., Eds., Moscow: Nauka, 1965, pp.156–159.
Ratner, S.B. and Lurie, E.G., Abrasion of Polymers as a Thermally Activated Kinetic Process, Dokl. AN SSSR, 1966, vol. 166, no. 4, pp. 909–912.
Frictional Wear of Rubber: Coll. Papers, Evstratov, V.F., Ed., Moscow: Knimia, 1964.
Kragelsky, I.V. and Nepomnyashchy, E.F., Fatigue Mechanism in Elastic Contact, in Mechanics and Mechanical Engineering, Moscow: Izd–vo AN SSSR, 1963, pp. 49–56.
Kragelsky, I.V. and Nepomnyashchy, E.F., Theory of Wear of Highly Elastic Materials, in Plastics in Sliding Bearings, Moscow: Nauka, 1965, pp. 49–56.
Kragelsky, I.V., Reznikovsky, M.M., Brodsky, G.I., and Nepomnyashchy, E.F., Friction Contact Fatigue of Highly Elastic Materials, KauchukRezina, 1965, no. 9, pp. 30–34.
Clark, W.T. and Lancaster, J.K., Breakdown and Surface of Carbons during Repeated Sliding, Wear, 1963, vol. 6, no. 6, pp. 467–482.
Kerridge, M. and Lancaster, J.K., The Stages in a Process of Severe Metallic Wear, Proc. Roy. Soc., 1956, vol. 236, pp.250–254.
Eiss, N.S., Jr., Fatigue Wear of Polymers, ACS Symposium Series, 1984, vol. 50, pp. 78–82.
Mars, W.V. and Fatemi, A., A Literature Survey of Fatigue Analysis Approaches for Rubber, Int. J. Fatigue, 2002, vol. 24, pp. 949–961.
Cadwell, S.M., Merrill, R.A., Sloman, C.M., and Yost, F.L., Dynamic Fatigue Life of Rubber, Ind. Eng. Chem., 1940, vol. 12, pp. 19–23.
Fielding, J.H., Flex Life and Crystallization of Synthetic Rubber, Ind. Eng. Chem., 1943, vol. 35, no. 12, pp. 1259–1261.
Handbook of Molded and Extruded Rubber, Goodyear Tire and Rubber Company, 1969.
Ayoub, G., Naït–Abdelaziz, M., and Zaïri, F., Multiaxial Fatigue Life Predictors for Rubbers: Application of Recent Developments to a Carbon–Filled SBR, Int. J. Fatigue, 2014, vol. 66, pp. 168–176.
Jardin, A., Leblond, J.–B., Berghezan, D., and Portigliatti, M., Theoretical Modelling and Experimental Study of the Fatigue of Elastomers under Cyclic Loadings of Variable Amplitude, Comp. Rend. Mécan., 2014, vol. 342, no. 8, pp. 450–458.
Zhang, J., Xue, F., Wang, Y., Zhang, X., and Han, S., Strain Energy–Based Rubber Fatigue Life Prediction under the Influence of Temperature, R. Soc. Open Sci., 2018, vol. 5, p. 180951.
Bezukhov, N.I., Fundamentals of the Theory of Elasticity, Plasticity, and Creep, Moscow: Vysshaya Shkola, 1961.
Kostetsky, B.I., Friction and Wear in Machine Parts, in Proc. 2nd All–Union Conf. on Friction and Wear in Machines, Vol. 4, Moscow: USSR Academy of Sciences, 1951, pp. 201–208.
Goryacheva, I.G., Mechanics of Frictional Interaction, Moscow: Nauka, 2001.
Goryacheva, I.G. and Chekina, O.G., Model of Fatigue Fracture of Surfaces, S v. J. Frict. Wear, 1990, vol. 11, no. 3, pp.389–400.
Goryacheva, I.G. and Chekina, O.G., Surface Wear: From Microfracture Modeling to Shape Change Analysis, Izv. RAN. MTT, 1999, no. 5, pp. 131–147.
Goryacheva, I.G. and Torskaya, E.V., Modeling of Fatigue Wear of a Two–Layered Elastic Half–Space in Contact with Periodic System of Indenters, Wear, 2010, vol. 268, no. 11–12, pp. 1417–1422.
Chekina, O.G., Modeling of Fracture of Surface Layers in Contact of Rough Bodies, Prochn. Plastich., 1996, vol. 1, pp. 186–191.
Aleksandrov, V.M., Goryacheva, I.G., and Torskaya, E.V., Sliding Contact of a Smooth Indenter and a Viscoelastic Half–Space (3D Problem), Dokl. Phys., 2010, vol. 55, no. 2, pp. 77–80.
Goryacheva, I.G., Stepanov, F.I., and Torskaya, E.V., Sliding of a Smooth Indentor over a Viscoelastic Half–Space When There is Friction, J. Appl. Math. Mech., 2015, vol. 79, no. 6, pp. 596–603.
Stepanov, F.I., Sliding of Two Smooth Indenters on a Viscoelastic Foundation in the Presence of Friction, J. Appl. Mech. Tech. Phys., 2015, vol. 56, no. 6, pp. 1071–1077.
Johnson, K.L., Contact Mechanics, Cambridge: Cambridge University Press, 1985.
Stepanov, F.I. and Torskaya, E.V., Study of Stress State of Viscoelastic Half–Space in Sliding Contact with Smooth Indenter, J. Frict. Wear, 2016, vol. 37, no. 2, pp 101–106.
Goryacheva, I.G., Makhovskaya, Yu.Yu., Morozov, A.V., and Stepanov, F.I., Friction of Elastomers: Modeling and Experiment, Moscow: Izhevsk Institute of Computer Science, 2017.
Barenblatt, G.I., Flow, Deformation and Fracture: Lectures on Fluid Mechanics and the Mechanics of Deformable Solids for Mathematicians and Physicists, Cambridge: Cambridge University Press, 2014.
Author information
Authors and Affiliations
Corresponding author
Additional information
Russian Text © I.G. Goryacheva, F.I. Stepanov, E.V. Torskaya, 2018, published in Fizicheskaya Mezomekhanika, 2018, Vol. 21, No. 6, pp. 66–74.
Rights and permissions
About this article
Cite this article
Goryacheva, I.G., Stepanov, F.I. & Torskaya, E.V. Fatigue Wear Modeling of Elastomers. Phys Mesomech 22, 65–72 (2019). https://doi.org/10.1134/S1029959919010107
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1134/S1029959919010107