Abstract
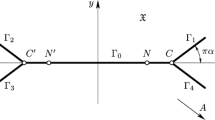
For the Riemann–Hilbert problem in a singularly deformed domain, an asymptotic expansion is found that corresponds to the limit transition from Somov’s magnetic reconnection model to Syrovatskii’s one as the relative shock front length \(\varrho \) tends to zero. It is shown that this passage to the limit corresponding to \(\varrho \to 0\) is performed with the preservation of the reverse current region, while the parameter determining magnetic field refraction on shock waves grows as \({{\varrho }^{{ - 1/2}}}\). Moreover, the correction term to the Syrovatskii field has the order of \(\rho \) and decreases in an inverse proportion to the distance from the current configuration.
Similar content being viewed by others
REFERENCES
D. Biskamp, Magnetic Reconnection in Plasmas (Cambridge Univ. Press, Cambridge, UK, 2000).
E. Priest and T. Forbes, Magnetic Reconnection: MHD Theory and Applications (Cambridge Univ. Press, Cambridge, 2000).
B. V. Somov, Plasma Astrophysics, Part II: Reconnection and Flares (Springer Science + Business Media, New York, 2013).
W. Gonzales and E. Parker, Magnetic Reconnection: Concepts and Applications (Springer Science + Business Media, New York, 2016).
S. I. Syrovatskii, “Dynamic dissipation and particle acceleration,” Astron. Zh. 43 (2), 340–355 (1966).
V. S. Imshennik and S. I. Syrovatskii, “Two-dimensional flow of an ideally conducting gas in the vicinity of the zero line of a magnetic field,” J. Exp. Theor. Phys. 33 (5), 656–664 (1967).
S. I. Syrovatskii, “Formation of current sheets in a plasma with a frozen-in strong magnetic field,” J. Exp. Theor. Phys. 33 (5), 933–940 (1971).
B. V. Somov and S. I. Syrovatskii, “Hydrodynamic plasma flows in a strong magnetic field,” Neutral Current Sheets in Plasmas (Springer, Boston, MA, 1974), pp. 13–71.
P. F. Chen, C. Fang, Y. H. Tang, and M. D. Ding, “Simulation of magnetic reconnection with heat condition,” Astrophys. J. 513, 516–523 (1999).
M. Ugai, “The evolution of fast reconnection in a three-dimensional current sheet system,” Phys. Plasmas 15, 082306 (2008).
M. J. Aschwanden, Physics of the Solar Corona: An Introduction with Problems and Solutions (New York, 2005).
P. A. Gritsyk and B. V. Somov, “X-ray and microwave emissions from the July 19, 2012 solar flare: Highly accurate observations and kinetic models,” Astron. Lett. 42 (8), 531–543 (2016).
K. V. Brushlinskii, A. M. Zaborov, and S. I. Syrovatskii, “Numerical analysis of the current sheet near a magnetic null line,” Sov. J. Plasma Phys. 6 (2), 165–173 (1980).
D. Biskamp, “Magnetic reconnection via current sheets,” Phys. Fluids 29, 1520 (1986).
H. E. Petschek, “Magnetic field annihilation,” AAS-NASA Symposium on the Physics of Solar Flares, Greebelt, October 28–30, 1963 (NASA SP-50, Maryland, 1964), pp. 425–439.
S. A. Markovskii and B. V. Somov, “Some properties of the magnetic reconnection in a current sheet with shock waves,” Proceedings of the 6th Annual Seminar on Problems in Solar Flare Physics (Nauka, Moscow, 1988), pp. 93–110.
S. A. Markovskii and B. V. Somov, “Some properties of the magnetic reconnection in a current sheet with shock waves,” in Solar Plasma Physics (Nauka, Moscow, 1989), p. 45 [in Russian].
S. I. Bezrodnykh and V. I. Vlasov, “The Riemann–Hilbert problem in a complicated domain for a model of magnetic reconnection in a plasma,” Comput. Math. Math. Phys. 42 (3), 263–298 (2002).
S. I. Bezrodnykh, V. I. Vlasov, and B. V. Somov, “Analytical model of magnetic reconnection in the presence of shock waves attached to a current sheet,” Astron. Lett. 33 (2), 130–136 (2007).
S. I. Bezrodnykh, V. I. Vlasov, and B. V. Somov, “Generalized analytical models of Syrovatskii’s current sheet,” Astron. Lett. 37 (2), 113–130 (2011).
V. I. Vlasov, Boundary Value Problems in Domains with Curved Boundaries (Vychisl. Tsentr Akad. Nauk SSSR, Moscow, 1987) [in Russian].
S. I. Bezrodnykh and V. I. Vlasov, “Singular Riemann–Hilbert problem in complex-shaped domains,” Comput. Math. Math. Phys. 54 (12), 1826–1875 (2014).
S. I. Bezrodnykh and V. I. Vlasov, “On a new representation for the solution of the Riemann–Hilbert problem,” Math. Notes 99 (6), 932–937 (2016).
S. I. Bezrodnykh, “Finding the coefficients in the new representation of the solution of the Riemann–Hilbert problem using the Lauricella function,” Math. Notes 101 (5), 759–777 (2017).
S. I. Bezrodnykh, “The Lauricella hypergeometric function \(F_{D}^{{(N)}}\), the Riemann–Hilbert problem, and some applications,” Russ. Math. Surv. 73 (6), 941–1031 (2018).
V. I. Vlasov, S. A. Markovskii, and B. V. Somov, An analytical model of magnetic reconnection in plasma, Available from VINITI, No. 769-V89 (Moscow, 1989).
W. Koppenfels and F. Stallmann, Praxis der Konformen Abbildung (Springer, Berlin, 1959).
M. A. Lavrent’ev and B. V. Shabat, Methods of the Theory of Functions of Complex Variables (Nauka, Moscow, 1987) [in Russian].
Higher Transcendental Functions (Bateman Manuscript Project), Ed. by A. Erdélyi (McGraw-Hill, New York, 1953), Vol. 1.
N. S. Bakhvalov, N. P. Zhidkov, and G. M. Kobel’kov, Numerical Methods (Nauka, Moscow, 1987) [in Russian].
L. V. Kantorovich and V. I. Krylov, Approximate Methods of Higher Analysis (Fizmatgiz, Moscow, 1962; Wiley, New York, 1964).
H. Exton, Multiple Hypergeometric Functions and Application (Willey, New York, 1976).
O. M. Olsson, “Integration of the partial differential equations for the hypergeometric functions \({{F}_{1}}\) and \({{F}_{D}}\) of two and more variables,” J. Math. Phys. 5, 420–430 (1964).
F. D. Gakhov, Boundary Value Problems (Nauka, Moscow, 1977; Dover, New York, 1990).
N. I. Muskhelishvili, Singular Integral Equations (Nauka, Moscow, 1968; Wolters-Noordhoff, Groningen, 1972).
Author information
Authors and Affiliations
Corresponding authors
Additional information
Translated by I. Ruzanova
Rights and permissions
About this article
Cite this article
Bezrodnykh, S.I., Vlasov, V.I. Asymptotics of the Riemann–Hilbert Problem for a Magnetic Reconnection Model in Plasma. Comput. Math. and Math. Phys. 60, 1839–1854 (2020). https://doi.org/10.1134/S0965542520110056
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1134/S0965542520110056