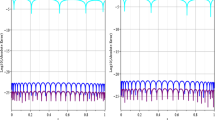
Abstract
Given an ensemble of numerical solutions generated by different algorithms that are guaranteed to have different errors, the triangle inequality is used to find a neighborhood of a numerical solution that contains the true one. By analyzing the distances between the numerical solutions, the latter can be ranged according to their error magnitudes. Numerical tests for the two-dimensional compressible Euler equations demonstrate the possibility of comparing the errors of different methods and determining a domain containing the true solution.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
REFERENCES
A. K. Alekseev and A. E. Bondarev, “On localization of the true solution on an ensemble of computations,” Abstracts of Papers of the 20th International Conference on Computational Mechanics and Modern Software Application Systems, Alushta, May 24–31, 2017 (Mosk. Aviats. Inst., Moscow, 2017), pp. 357–359.
A. K. Alekseev, A. E. Bondarev, and I. M. Navon, “On estimation of discretization error norm via ensemble of approximate solutions,” arXiv:1704.04994 [physics.comp-ph]. April 18, 2017.
A. K. Alexeev and A. E. Bondarev, “On exact solution enclosure on ensemble of numerical simulations,” Math. Montisnigri 38, 63–77 (2017).
D. Burago, Yu. D. Burago, and S. Ivanov, A Course in Metric Geometry (Am. Math. Soc., Providence, R.I., 2001).
L. Wang, Y. Zhang, and J. Feng, “On the Euclidean distance of images,” IEEE Trans. Pattern Anal. Mach. Intell. 27 (8), 1334–1339 (2005).
P. Ch. Mahalanobis, “On the generalized distance in statistics,” Proc. Natl. Inst. Sci. India 2 (1), 49–55 (1936).
V. Ya. Borovoi, Gas Flow and Heat Transfer in Shock Wave-Boundary Layer Interaction Zones (Mashinostroenie, Moscow, 1975) [in Russian].
R. Courant, E. Isaacson, and M. Rees, “On the solution of nonlinear hyperbolic differential equations by finite differences,” Commun. Pure Appl. Math. 5, 243–255 (1952).
A. G. Kulikovskii, N. V. Pogorelov, and A. Yu. Semenov, Mathematical Aspects of Numerical Solution of Hyperbolic Systems (Fizmatlit, Moscow, 2001; Chapman and Hall/CRC, London, 2001).
B. van Leer, “Towards the ultimate conservative difference scheme: V. A second-order sequel to Godunov’s method,” J. Comput. Phys. 32, 101–136 (1979).
M. Sun and K. Katayama, “An artificially upstream flux vector splitting for the Euler equations,” J. Comput. Phys. 189, 305–329 (2003).
S. Osher and S. Chakravarthy, “Very high order accurate TVD schemes,” ICASE Report, No. 84-144, 229–274 (1984).
C.-T. Lin, et al., “High resolution finite volume scheme for the quantum hydrodynamic equations,” J. Comput. Phys. 228 (5), 1713–1732 (2009).
S. Yamamoto and H. Daiguji, “Higher-order-accurate upwind schemes for solving the compressible Euler and Navier–Stokes equations,” Comput. Fluids 22, 259–270 (1993).
S. I. Repin, A Posteriori Estimates for Partial Differential Equations (Walter de Gruyter, Berlin, 2008), Vol. 4.
G. I. Marchuk and V. V. Shaidurov, Improvement of the Accuracy of Solutions to Difference Schemes (Nauka, Moscow, 1979) [in Russian].
FUNDING
This work was supported by the Russian Foundation for Basic Research, projects no. 17-01-444A.
Author information
Authors and Affiliations
Corresponding authors
Additional information
Translated by I. Ruzanova
Rights and permissions
About this article
Cite this article
Alekseev, A.K., Bondarev, A.E. Estimation of the Distance between True and Numerical Solutions. Comput. Math. and Math. Phys. 59, 857–863 (2019). https://doi.org/10.1134/S0965542519060034
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1134/S0965542519060034