Skip to main content
SpringerLink
Log in

On the matrix Fourier filtering problem for a class of models of nonlinear optical systems with a feedback

  • Published:
Computational Mathematics and Mathematical Physics Aims and scope Submit manuscript

Abstract

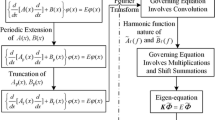
A novel statement of the Fourier filtering problem based on the use of matrix Fourier filters instead of conventional multiplier filters is considered. The basic properties of the matrix Fourier filtering for the filters in the Hilbert–Schmidt class are established. It is proved that the solutions with a finite energy to the periodic initial boundary value problem for the quasi-linear functional differential diffusion equation with the matrix Fourier filtering Lipschitz continuously depend on the filter. The problem of optimal matrix Fourier filtering is formulated, and its solvability for various classes of matrix Fourier filters is proved. It is proved that the objective functional is differentiable with respect to the matrix Fourier filter, and the convergence of a version of the gradient projection method is also proved.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. J. W. Goodman, Introduction to Fourier Optics (McGraw Hill, New York, 1968).

    Google Scholar 

  2. E. V. Degtiarev and M. A. Vorontsov, “Spatial filtering in nonlinear two-dimensional feedback systems: Phasedistortion suppression,” J. Opt. Soc. Am., Ser. B 12, 1238–1248 (1995).

    Article  Google Scholar 

  3. E. W. Justh, M. A. Vorontsov, G. Garhart, L. A. Beresnev, and P. S. Krishnapasad, “Adaptive optics with advanced phase contrast techniques. Part II: High resolution wavefront control,” J. Opt. Soc. Am. A 18, 1300–1311 (2001).

    Article  Google Scholar 

  4. A. V. Larichev, I. P. Nikolaev, and V. I. Shmal’gauzen, “Optical dissipative structures with a controlled spatial period in a nonlinear system with a Fourier filter in the feedback loop,” Kvant. Elektron. 23 (10), 894–899 (1996).

    Google Scholar 

  5. A. V. Larichev, I. P. Nikolaev, and P. Violino, “LCLV-based system for high resolution wavefront correction: Phase knife as a feedback intensity producer,” Opt. Commun. 138, 127–135 (1997).

    Article  Google Scholar 

  6. I. P. Nikolaev, A. V. Larichev, and V. I. Shmal’gauzen, “Controlled optical structures in a nonlinear system involving the suppression of low spatial frequencies in the feedback loop,” Quantum Electron. 30, 617–622 (2000).

    Article  Google Scholar 

  7. A. V. Larichev, I. P. Nikolaev, S. Costamagna, and P. Violino, “Advanced phase knife technique,” Opt. Commun. 121, 95–102 (1995).

    Article  Google Scholar 

  8. B. Heise, M. Reinhardt, S. Schausberger, S. Hauser, S. Bernstein, and D. Stifter, “Fourier plane filtering revisited— analogies in optics and mathematics,” Sampling Theory in Signal & Image Proc. 13 (3), 231–248 (2014).

    MathSciNet  MATH  Google Scholar 

  9. R. Martin, G.-L. Oppo, G. K. Harkness, A. J. Scroggie, and W. J. Firth, “Controlling pattern formation and spatio-temporal disorder in nonlinear optics,” Optics Express 1 (1), 39–44 (1997).

    Article  Google Scholar 

  10. S. J. Jensen, M. Schwab, and C. Denz, “Manipulation, stabilization, and control of pattern formation using Fourier space filtering,” Phys. Rev. Lett. 81, 1614–1617 (1998).

    Article  Google Scholar 

  11. M. Schwab, M. Saffman, C. Denz, and T. Tschudi, “Fourier control of pattern formation in an interferometric feedback configuration,” Opt. Commun. 170, 129–136 (1999).

    Article  Google Scholar 

  12. G. K. Harkness, G.-L. Oppo, E. Benkler, M. Kreuzer, R. Neubecker, and T. Tschudi, “Fourier space control in an LCLV feedback system,” J. Opt. B.: Quantum Semiclass. Opt. 1, 177–182 (1999).

    Article  Google Scholar 

  13. G.-L. Oppo, R. Martin, A. J. Scroggie, G. K. Harkness, A. Lord, and W. J. Firth, “Control of spatio-temporal complexity in nonlinear optics,” Chaos, Solitons Fractals 10, 865–874 (1999).

    Article  Google Scholar 

  14. M. Pesch, E. G. Westhoff, T. Ackermann, and W. Lange, “Direct measurement of multiple instability regions via a Fourier filtering method in an optical pattern forming system,” Phys. Rev., Ser. E 68 (016209) (2003).

    Article  Google Scholar 

  15. B. Gutlich, R. Neubecker, M. Kreuzer, and T. Tschudi, “Control and manipulation of solitary structures in a nonlinear optical single feedback experiment,” Chaos 13 (1), 239–246 (2003).

    Article  Google Scholar 

  16. L. A. Poyneer, B. A. Macintosh, and J.-P. Veran, “Fourier transform wavefront control with adaptive prediction of the atmosphere,” J. Opt. Soc. Am., Ser. A 24, 2645–2660 (2007).

    Article  Google Scholar 

  17. M. Nagashima and B. Agrawal, “Application of complex-valued FXLMS adaptive filter to Fourier basis control of adaptive optics,” in Proc. of the American Control Conference (CA, USA, San Francisco, 2011), pp. 2933–2944.

    Google Scholar 

  18. M. M. Potapov and K. A. Chechkina, “On a model of the amplitude-phase filtering in a nonlinear optical system with a feedback,” Vestn. Mosk. Univ., Ser. 15, Vychisl. Mat. Kibern., No. 4, 31–36 (1997).

    MATH  Google Scholar 

  19. A. V. Razgulin and V. A. Chushkin, “On the optimal Fourier filtration for a class of models of nonlinear optical systems with a feedback,” Comput. Math. Math. Phys. 44, 1528–1538 (2004).

    MathSciNet  MATH  Google Scholar 

  20. A. V. Razgulin, “Projection-difference method for controlled Fourier filtering,” Comput. Math. Modeling 23 (1), 56–71 (2012).

    Article  MathSciNet  MATH  Google Scholar 

  21. M. A. Vorontsov, N. I. Zheleznykh, and V. Yu. Ivanov, “Transverse interactions in 2-D feedback non-linear optical systems,” Opt. Quantum Electron. 22, 501–515 (1990).

    Article  Google Scholar 

  22. V. Yu. Ivanov, A. V. Larichev, and M. A. Vorontsov, “One-dimensional rotatory waves in the optical systems with nonlinear large-scale field interactions,” Proc. SPIE Int. Soc. Opt. Eng. 1402, 145–153 (1991).

    Google Scholar 

  23. S. A. Akhmanov, M. A. Vorontsov, V. Yu. Ivanov, A. V. Larichev, N. I. Zheleznykh, “Controlling transversewave interactions in nonlinear optics: generation and interaction of spatiotemporal structures,” J. Opt. Soc. Amer., Ser. B 9 (1), 78–90 (1992).

    Article  Google Scholar 

  24. A. V. Razgulin, “On self-oscillations in a nonlinear parabolic problem with transformed argument,” Zh. Vychisl. Mat. Mat. Fiz. 33 (1), 69–80 (1993).

    MathSciNet  MATH  Google Scholar 

  25. A. V. Razgulin, “Rotatory waves in an optical system with a two-dimensional fedback,” Mat. Model. 5 (4), 105–119 (1993).

    MATH  Google Scholar 

  26. A. L. Skubachevskii, “On the Hopf bifurcation for the quasi-linear parabolic functional-differential equation,” Differ. Uravn. 34, 1394–1401 (1998).

    MathSciNet  Google Scholar 

  27. A. L. Skubachevskii, “Bifurcation of periodic solutions for nonlinear parabolic functional differential equations arising in optoelectronics,” Nonlinear Analys: TMA 32 (2), 261–278 (1998).

    Article  MathSciNet  MATH  Google Scholar 

  28. V. A. Chushkin and A. V. Razgulin, “Stationary structures in the functional-differential diffusion equation with the reflection of the spatial argument,” Vestn. Mosk. Univ., Ser. 15, Vychisl. Mat. Kibern., No. 2, 13–20 (2003).

    MATH  Google Scholar 

  29. E. P. Belan and O. B. Lykova, “Rotating structures in a parabolic functional-differential equation,” Differ. Equations 40, 1419–1430 (2004).

    Article  MathSciNet  MATH  Google Scholar 

  30. M. M. Potapov, “The nonlinear optics equation with transformations of the independent variable considered as control,” Vestn. Mosk. Univ., Ser. 15, Vychisl. Mat. Kibern., No. 3, 1316 (1997).

    Google Scholar 

  31. A. V. Razgulin, “A class of parabolic functional-differential equations of nonlinear optics,” Differ. Equations 36, 449–456 (2000).

    Article  MathSciNet  MATH  Google Scholar 

  32. A. V. Razgulin, “Parabolic functional differential equations with a controlled transformation of spatial arguments,” Dokl. Math. 72, 551–554 (2005).

    MATH  Google Scholar 

  33. A. V. Razgulin, “The problem of control of a two-dimensional transformation of spatial arguments in a parabolic functional-differential equation,” Differ. Equations 42, 1140–1155 (2006).

    Article  MathSciNet  MATH  Google Scholar 

  34. A. V. Razgulin and I. B. Roganovich, “Convergence of the projection difference scheme for the nonlinear parabolic equation with transformed spatial argument,” Comput. Math. Modeling 12 (3), 262–270 (2001).

    Article  MathSciNet  MATH  Google Scholar 

  35. A. V. Razgulin, “Approximation of the problem of controlling arguments transformation in a nonlinear parabolic equation,” Comput. Math. Math. Phys. 41, 1752–1764 (2001).

    MathSciNet  MATH  Google Scholar 

  36. A. V. Razgulin, “Projection difference scheme for a parabolic functional differential equation with two-dimensional transformation of arguments,” Comput. Math. Math. Phys. 45, 1780–1791 (2005).

    MathSciNet  MATH  Google Scholar 

  37. V. A. Grebennikov and A. V. Razgulin, “Weighted estimate for the convergence rate of a projection difference scheme for a quasilinear parabolic equation,” Comput. Math. Math. Phys. 51, 1208–1221 (2011).

    Article  MathSciNet  MATH  Google Scholar 

  38. H. Brezis, Functional Analysis, Sobolev Spaces and Partial Differential Equations (Springer, London,2010).

    Book  Google Scholar 

  39. J-L. Lions, Quelques méthodes de résolution des problèmes aux limites non linéaires (Dunod, Paris, 1969; Mir, Moscow, 1972).

    MATH  Google Scholar 

  40. J-L. Lions and E. Magenes, Problèmes aux limites non homogènes et applications (Dunod, Paris, 1968; Mir, Moscow, 1971).

    MATH  Google Scholar 

  41. N. Dunford and J. T. Schwartz, Linear Operators: Spectral Theory (Interscience, New York, 1958; Mir, Moscow, 1966).

    MATH  Google Scholar 

  42. M. M. Lavrent’ev and L. Ya. Savel’ev, Operator Theory and Ill-Posed Problems (Institut Matematiki, Novosibirsk, 2010) [in Russian].

    MATH  Google Scholar 

  43. F. P. Vasil’ev, Optimization Methods (Faktorial, Moscow, 2002) [in Russian].

    Google Scholar 

  44. A. V. Razgulin, “Projection-gradient method for quasidifferentiable functionals with Hölder-continuous gradient,” Moscow Univ. Comput. Math. Cybernet. 30, 10–13 (2006).

    MATH  Google Scholar 

  45. Ya. I. Al’ber, “On the minimization of functionals of the class on bounded sets,” Ekon. Mat. Metody 16, 185–190 (1980).

    MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Faculty of Computational Mathematics and Cybernetics, Moscow State University, Moscow, 119991, Russia

    A. V. Razgulin & S. V. Sazonova

Authors
  1. A. V. Razgulin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. S. V. Sazonova
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to A. V. Razgulin.

Additional information

Original Russian Text © A.V. Razgulin, S.V. Sazonova, 2017, published in Zhurnal Vychislitel’noi Matematiki i Matematicheskoi Fiziki, 2017, Vol. 57, No. 9, pp. 1403–1420.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Razgulin, A.V., Sazonova, S.V. On the matrix Fourier filtering problem for a class of models of nonlinear optical systems with a feedback. Comput. Math. and Math. Phys. 57, 1385–1403 (2017). https://doi.org/10.1134/S0965542517090123

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S0965542517090123

Keywords

Search

Navigation