Skip to main content
SpringerLink
Log in

Finite difference schemes for the singularly perturbed reaction-diffusion equation in the case of spherical symmetry

  • Published:
Computational Mathematics and Mathematical Physics Aims and scope Submit manuscript

Abstract

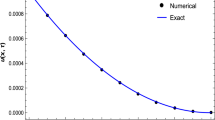
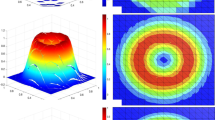
The boundary value problem for the singularly perturbed reaction-diffusion parabolic equation in a ball in the case of spherical symmetry is considered. The derivatives with respect to the radial variable appearing in the equation are written in divergent form. The third kind boundary condition, which admits the Dirichlet and Neumann conditions, is specified on the boundary of the domain. The Laplace operator in the differential equation involves a perturbation parameter ɛ2, where ɛ takes arbitrary values in the half-open interval (0, 1]. When ɛ → 0, the solution of such a problem has a parabolic boundary layer in a neighborhood of the boundary. Using the integro-interpolational method and the condensing grid technique, conservative finite difference schemes on flux grids are constructed that converge ɛ-uniformly at a rate of O(N −2ln2 N + N −10 ), where N + 1 and N 0 + 1 are the numbers of the mesh points in the radial and time variables, respectively.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. G. I. Shishkin, Grid Approximations of Singularly Perturbed Elliptic and Parabolic Equations (Ural. Otd. Ross. Akad. Nauk, Yekaterinburg, 1992) [in Russian].

    Google Scholar 

  2. P. W. Hemker, G. I. Shishkin, and L. P. Shishkina, “Uniform Schemes with High-Order Time-accuracy for Parabolic Singular Perturbation Problems,” IMA J. Numer. Analys. 20(1), 99–121 (2000).

    Article  MATH  MathSciNet  Google Scholar 

  3. G. I. Shishkin, “A Finite Difference Scheme on a Priori Adapted Meshes for a Singularly Perturbed Parabolic Convection-Diffusion Equation,” Numer. Math. Theory Meth. Appl. 1(2), 214–234 (2008).

    MathSciNet  MATH  Google Scholar 

  4. G. I. Shiskhin, “Grid Approximation of Singularly Perturbed Parabolic Equations with Piecewise Continuous Initial-Boundary Conditions,” Proc. Steklov Inst. Math, Suppl. 2, 213–230 (2007).

  5. Heat Engineering Experiments: Handbook, Ed. by V. A. Grigor’ev and V. M. Zorin (Energoizdat, Moscow, 1982) [in Russian].

    Google Scholar 

  6. A. A. Samarskii, Theory of Finite Difference Schemes (Nauka, Moscow, 1989; Marcel Dekker, New York, 2001).

    Google Scholar 

  7. A. A. Samarskii, Introduction to the Theory of Finite Difference Schemes (Nauka, Moscow, 1971) [in Russian].

    Google Scholar 

  8. G. I. Shishkin, “Approximation of Solutions of Singularly Perturbed Boundary Value Problems with a Parabolic Boundary Layer,” Zh. Vychisl. Mat. Mat. Fiz. 29, 963–977 (1989) [USSR Comput. Math. Math. Phys. 29 (7–8), 1–10 (1989)].

    MATH  MathSciNet  Google Scholar 

  9. J. J. H. Miller, E. O’Riordan, and G. I. Shishkin, Fitted Numerical Methods for Singular Perturbation Problems (World Sci., Singapore, 1996).

    MATH  Google Scholar 

  10. A. M. Il’in, A. S. Kalashnikov, and O. A. Oleinik, “Second-Order Linear Parabolic Equations,” Usp. Mat. Nauk 17(3), 3–146 (1962).

    Google Scholar 

  11. O. A. Ladyzhenskaya, V. A. Solonnikov, and N. N. Ural’tseva, Linear and Quasilinear Equations of Parabolic Type (Nauka, Moscow, 1967; American Mathematical Society, Providence, 1968).

    MATH  Google Scholar 

  12. A. Friedman, Partial Differential Equations of Parabolic Type (Prentice-Hall, Inc., Englewood Cliffs, N.J., 1964; Mir, Moscow, 1967).

    MATH  Google Scholar 

  13. G. I. Shishkin, “Grid Approximation of Singularly Perturbed Boundary Value Problem for Quasi-Linear Parabolic Equations in Case of Complete Degeneracy in Spatial Variables,” Soviet J. Numer. Analys. Math. Modelling 6, 243–261 (1991).

    Article  MATH  MathSciNet  Google Scholar 

  14. G. I. Shishkin, “Grid Approximation of a Singularly Perturbed Boundary Value Problem for a Quasi-Linear Elliptic Equation in the Case of the Complete Degeneracy,” Zh. Vychisl. Mat. Mat. Fiz. 31, 1808–1825 (1991).

    MathSciNet  Google Scholar 

  15. A. N. Tikhonov and A. A. Samarskii, Equations of Mathematical Physics (Mosk. Gos. Univ., Moscow, 1999) [in Russian].

    Google Scholar 

  16. B. M. Budak, A. A. Samarskii, and A. N. Tikhonov, A Collection of Problems on Mathematical Physics (Nauka, Moscow, 1980; Pergamon Press, Oxford, 1964).

    Google Scholar 

  17. L. M. Degtyarev and A. P. Favorskii, “A Flux Version of the Double Sweep method,” Zh. Vychisl. Mat. Mat. Fiz. 8, 679–684 (1968).

    Google Scholar 

  18. L. M. Degtyarev and A. P. Favorskii, “A Flux Version of the Double Sweep Method for Difference Problems with Strongly Varying Coefficients,” Zh. Vychisl. Mat. Mat. Fiz. 9, 211–218 (1969).

    MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute of Mathematics and Mechanics, Ural Division, Russian Academy of Sciences, ul. S. Kovalevskoi 16, Yekaterinburg, 620219, Russia

    G. I. Shishkin & L. P. Shishkina

Authors
  1. G. I. Shishkin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. L. P. Shishkina
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to G. I. Shishkin.

Additional information

Original Russian Text © G.I. Shishkin, L.P. Shishkina, 2009, published in Zhurnal Vychislitel’noi Matematiki i Matematicheskoi Fiziki, 2009, Vol. 49, No. 5, pp. 840–856.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Shishkin, G.I., Shishkina, L.P. Finite difference schemes for the singularly perturbed reaction-diffusion equation in the case of spherical symmetry. Comput. Math. and Math. Phys. 49, 810–826 (2009). https://doi.org/10.1134/S0965542509050078

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S0965542509050078

Keywords

Search

Navigation