Skip to main content
SpringerLink
Log in

Trapped modes in a cylindrical elastic waveguide with a damping gasket

  • Published:
Computational Mathematics and Mathematical Physics Aims and scope Submit manuscript

Abstract

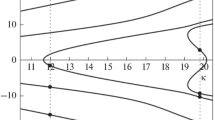
An infinite cylindrical body containing a three-dimensional heavy rigid inclusion with a sharp edge is considered. Under certain constraints on the symmetry of the body, it is shown that any prescribed number of eigenvalues of the elasticity operator can be placed on an arbitrary real interval (0, l) by choosing suitable physical properties of the inclusion. In the continuous spectrum, these points correspond to trapped modes, i.e., to exponentially decaying solutions to the homogeneous problem. The results can be used to design filters and dampers of elastic waves in a cylinder.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. F. Ursell, “Trapping Modes in the Theory of Surface Waves,” Proc. Cambridge Philos. Soc. 47, 347–358 (1951).

    Article  MathSciNet  MATH  Google Scholar 

  2. D. S. Jones, “The Eigenvalues of 2 U + U = 0 When the Boundary Conditions Are Given on Semi-infinite Domains,” Proc. Cambridge Philos. Soc. 49, 668–684 (1953).

    MathSciNet  MATH  Google Scholar 

  3. D. V. Evans, “Trapped Acoustic Modes,” IMA J. Appl. Math., 49, 45–60 (1992).

    Article  MathSciNet  Google Scholar 

  4. A.-S. Bonnet-Bendhia and F. Starling, “Guided Waves by Electromagnetic Gratings and Nonuniqueness Examples for the Diffraction Problem,” Math. Methods Appl. Sci. 77, 305–338 (1994).

    Article  MathSciNet  Google Scholar 

  5. D. V. Evans, M. Levitin, and D. Vasil’ev, “Existence Theorems for Trapped Modes,” J. Fluid Mech. 261, 21–31 (1994).

    Article  MathSciNet  MATH  Google Scholar 

  6. I. Roitberg, D. Vassiliev, and T. Weidl, “Edge Resonance in an Elastic Semi-Strip,” Quart. J. Appl. Math. 51(1), 1–13 (1998).

    Article  MathSciNet  MATH  Google Scholar 

  7. I. V. Kamotskii and S. A. Nazarov, “Elastic Waves Localized near Periodic Sets of Flaws,” Dokl. Akad. Nauk 368, 771–773 (1999) [Dokl. Phys. 44, 715–717 (1999)].

    MathSciNet  Google Scholar 

  8. I. V. Kamotskii and S. A. Nazarov, “Wood’s Anomalies and Surface Waves in the Problem of Scattering by a Periodic Boundary 1, 2,” Mat. Sb. 190(1), 109–138 (1999); 190 (2), 43–70 (1999).

    MathSciNet  Google Scholar 

  9. I. V. Kamotskii and S. A. Nazarov, “The Augmented Scattering Matrix and Exponentially Decaying Solutions of an Elliptic Problem in a Cylindrical Domain,” Zap. Nauchn. Semin. POMI 264, 66–82 (2000).

    Google Scholar 

  10. S. A. Nazarov, “A Criterion for the Existence of Decaying Solutions in the Problem on a Resonator with a Cylindrical Waveguide,” Funk. Anal. Ego Prilozh. 40(2), 20–32 (2006).

    Google Scholar 

  11. S. A. Nazarov, “Artificial Boundary Conditions for Finding Surface Waves in the Problem of Diffraction by a Periodic Boundary,” Zh. Vychisl. Mat. Mat. Fiz. 46, 2267–2278 (2006) [Comput. Math. Math. Phys. 46, (2006)].

    Google Scholar 

  12. S. A. Nazarov and B. A. Plamenevsky, Elliptic Problems in Domains with Piecewise Smooth Boundary (Nauka, Moscow, 1991; Walter de Gruyter, Berlin, 1994).

    Google Scholar 

  13. E. Sanchez-Palencia, “Asymptotic and Spectral Properties of a Class of Singular-Stiff Problems,” J. Math. Pures Appl. 71, 379–406 (1992).

    MathSciNet  MATH  Google Scholar 

  14. M. Lobo and E. Perez, “High Frequency Vibrations in a Stiff Problem,” Math. Models Methods Appl. Sci. 7, 291–311 (1977).

    Article  MathSciNet  Google Scholar 

  15. M. H. Lobo, S. A. Nazarov, and M. E. Perez, “Asymptotically Sharp Uniform Estimates in a Scalar Spectral Stiffness Problem,” C.R. Mecanique 331, 325–330 (2003).

    Article  MATH  Google Scholar 

  16. M. I. Lobo, S. A. Nazarov, and M. E. Perez, “Eigenoscillations of a Strongly Inhomogeneous Elastic Body: Asymptotics and Uniform Estimates of Remainders,” Dokl. Akad. Nauk 389, 173–176 (2003) [Dokl. Math. 67, 271–274 (2003)].

    MathSciNet  MATH  Google Scholar 

  17. M. Lobo, S. A. Nazarov, and E. Perez, “Eigen-Oscillations of Contrasting Nonhomogeneous Bodies: Asymptotic and Uniform Estimates for Eigenvalues,” IMA J. Appl. Math, 70, 419–458 (2005).

    Article  MathSciNet  MATH  Google Scholar 

  18. S. A. Nazarov, Asymptotic Theory of Thin Plates and Bars: Dimensuin Reduction and Integral Estimates (Nauchnaya Kniga, Novosibirsk, 2001) [in Russian].

    Google Scholar 

  19. M. Sh. Birman and M. Z. Solomyak, Spectral Theory of Self-Adjoint Operators in Hilbert Spaces (Leningr. Gos. Univ., Leningrad, 1980) [in Russian].

    Google Scholar 

  20. I. V. Kamotskii and S. A. Nazarov, “On Eigenfunctions Localized in a Neighborhood of the Lateral Surface of a Thin Domain,” in Problems in Mathematical Analysis (Nauchnaya Kniga, Novosibirsk, 1999), 19, 105–148 [in Russian].

    Google Scholar 

  21. S. A. Nazarov, “Localization Effects for Eigenfunctions near the Edge of a Thin Domain,” Math. Bohemica 127(2), 283–292 (2002).

    MATH  Google Scholar 

  22. S. A. Nazarov, “The Polynomial Property of Self-Adjoint Elliptic Boundary Value Problems and the Algebraic Description of Their Attributes,” Usp. Mat. Nauk 54(5), 77–142 (1999).

    Google Scholar 

  23. S. A. Nazarov, “Self-Adjoint Elliptic Boundary Value Problems: The Polynomial Property and Formally Positive Operators,” in Problems in Mathematical Analysis (S.-Peterb. Gos. Univ., St. Petersburg, 1997), Vol. 16, pp. 167–192 [in Russian].

    Google Scholar 

  24. I. Ts. Gokhberg and M. G. Krein, Introduction to the Theory of Linear Non-Self-Adjoint Operators (Nauka, Moscow, 1965; Am. Math. Soc., Providence, R.I., 1969).

    Google Scholar 

  25. V. A. Kondrat’ev, “Boundary Value Problems for Elliptic Equations in Domains with Conical or Corner Points, Tr. Mosk. Mat. O-va 16, 219–292 (1963).

    Google Scholar 

  26. J.-L. Lions and E. Magenes, Problemes aux limites non homogéenes et applications (Dunod, Paris, 1968; Mir, Moscow, 1971).

    Google Scholar 

  27. S. A. Nazarov, “On the Asymptotics of the Spectrum of a Thin Plate Problem of Elasticity,” Sib. Mat. Zh. 41, 895–912 (2000).

    MATH  Google Scholar 

  28. S. G. Lekhnitskii, Elasticity Theory of Anisotropic Bodies (Nauka, Moscow, 1977) [in Russian].

    Google Scholar 

  29. O. A. Ladyzhenskaya, The Boundary Value Problems of Mathematical Physics (Nauka, Moscow, 1973; Springer-Verlag, New York, 1985).

    Google Scholar 

  30. S. A. Nazarov, “Weighting Functions and Invariant Integrals,” Vychisl. Mekh. Deform. Tverd. Tela, 1, 17–31 (1990).

    Google Scholar 

  31. V. G. Maz’ya and B. A. Plamenevskii, “On the Coefficients in Asymptotics of Solutions of Elliptic Boundary Value Problems in Domains with Conical Points,” Math. Nachr. 76, 29–60 (1977).

    Article  MathSciNet  MATH  Google Scholar 

  32. V. G. Maz’ya and B. A. Plamenevskii, “Estimates in L p and Hölder Classes and the Miranda-Agmon Maximum Principle for Solutions to Elliptic Boundary Value Problems in Domains with Singular Points on the Boundary,” Math. Nachr. 77, 25–82 (1977).

    Google Scholar 

  33. R. Duduchava and W. L. Wendland, “The Wiener-Hopf Method for Systems of Pseudodifferential Equations with an Application to Crack Problems,” Integral Equations Operator Theory 23, 294–335 (1995).

    Article  MathSciNet  MATH  Google Scholar 

  34. M. Costabel and M. Dauge, “Crack Singularities for General Elliptic Systems,” Math. Nachr. 235, 29–49 (2002).

    Article  MathSciNet  MATH  Google Scholar 

  35. N. Kh. Arutyunyan and S. A. Nazarov, “On Singularities of the Stress Function at the Corner Points of the Transverse Cross Section of a Twisted Bar with a Thin Reinforcing Layer,” Prikl. Mat. Mekh. 47(1), 122–132 (1983).

    MathSciNet  Google Scholar 

  36. M. Costabel and M. Dauge, “A Singularly Perturbed Mixed Boundary Value Problem,” Commun. Part. Differ. Equations 21, 1919–1949 (1996).

    MathSciNet  MATH  Google Scholar 

  37. S. A. Nazarov, “A Crack at the Interface of Anisotropic Bodies: Singularities of the Elastic Fields and a Criterion for Fracture When the Crack Surfaces Are in Contact,” Prikl. Mat. Mekh. 69, 520–532 (2005).

    MATH  Google Scholar 

  38. S. A. Nazarov, “Uniform Estimates of Remainders in Asymptotic Expansions of Solutions to the Problem of Eigenoscillations of a Piezoelectric Plate,” in Problems in Mathematical Analysis (Nauchnaya Kniga, Novosibirsk, 2003), Vol. 25, pp. 99–188 [in Russian].

    Google Scholar 

  39. D. Gomez, M. Lobo, S. A. Nazarov, and E. Perez, “Spectral Stiff Problems in Domains Surrounded by Thin Bands: Asymptotic and Uniform Estimates for Eigenvalues,” J. Math. Pures Appl. 85, 598–632 (2006).

    MathSciNet  MATH  Google Scholar 

  40. M. I. Vishik and L. A. Lyusternik, “Regular Degeneracy and Boundary Layer for Linear Differential Equations with Small Parameter,” Usp. Mat. Nauk 12(5), 3–122 (1957).

    MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute of Mechanical Engineering Problems, Russian Academy of Sciences, Vasil’evskii Ostrov, Bol’shoi pr. 61, St. Petersburg, 199178, Russia

    S. A. Nazarov

Authors
  1. S. A. Nazarov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to S. A. Nazarov.

Additional information

Original Russian Text © S.A. Nazarov, 2008, published in Zhurnal Vychislitel’noi Matematiki i Matematicheskoi Fiziki, 2008, Vol. 48, No. 5, pp. 863–881.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Nazarov, S.A. Trapped modes in a cylindrical elastic waveguide with a damping gasket. Comput. Math. and Math. Phys. 48, 816–833 (2008). https://doi.org/10.1134/S0965542508050102

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S0965542508050102

Keywords

Search

Navigation