Skip to main content
SpringerLink
Log in

A dynamic mesh adaptation method for magnetohydrodynamics problems

  • Published:
Computational Mathematics and Mathematical Physics Aims and scope Submit manuscript

Abstract

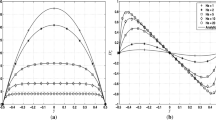
A dynamic adaptation method is used to numerically solve the MHD equations. The basic idea behind the method is to use an arbitrary nonstationary coordinate system for which the numerical procedure and the mesh refinement mechanism are formulated as a unified differential model. Numerical examples of multidimensional MHD flows on dynamic adaptive meshes are given to illustrate the method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. S. K. Godunov, A. V. Zabrodin, M. Ya. Ivanov, et al., Numerical Solution of Multidimensional Problems in Gas Dynamics (Nauka, Moscow, 1976) [in Russian].

    Google Scholar 

  2. H. A. Dwyer, “Grid Adaptation for Problems in Fluid Dynamics,” AIAA J. 22, 1705–1712 (1984).

    Article  MATH  Google Scholar 

  3. E. S. Oran and J. P. Boris, Numerical Simulation of Reactive Flow (Elsevier, New York, 1987; Mir, Moscow, 1990).

    MATH  Google Scholar 

  4. L. V. Kruglyakova, A. V. Neledova, V. F. Tishkin, and A. Yu. Filatov, “Unstructured Adaptive Meshes for Problems in Mathematical Physics (Review),” Mat. Model. 10(3), 93–116 (1998).

    MathSciNet  Google Scholar 

  5. A. N. Gil’manov, Adaptive Mesh Methods in Gas Dynamics (Fizmatlit, Moscow, 2000) [in Russian].

    Google Scholar 

  6. A. G. Kulikovskii, N. V. Pogorelov, and A. Yu. Semenov, Mathematical Aspects of Numerical Solution of Hyperbolic Systems (Fizmatlit, Moscow, 2001; Chapman & Hall/CRC, London, 2001).

    MATH  Google Scholar 

  7. M. J. Berger and J. Oliger, “Adaptive Mesh Refinement for Hyperbolic Partial Differential Equations,” J. Comput. Phys. 53, 484–512 (1984).

    Article  MATH  MathSciNet  Google Scholar 

  8. M. J. Berger and P. Colella, “Local Adaptive Mesh Refinement for Shock Hydrodynamics,” J. Comput. Phys. 82, 64–84 (1989).

    Article  MATH  Google Scholar 

  9. V. D. Liseikin, “Survey of Methods for Structured Adaptive Mesh Generation,” Zh. Vychisl. Mat. Mat. Fiz. 36, 3–41 (1996).

    MathSciNet  Google Scholar 

  10. J. U. Brackbill and J. S. Saltzman, “Adaptive Zoning for Singular Problems in Two Dimensions,” J. Comput. Phys. 46, 342–368 (1982).

    Article  MATH  MathSciNet  Google Scholar 

  11. A. F. Sidorov and O. V. Ushakov, “An Algorithm for Optimal Mesh Generation and Applications,” in Numerical Methods in Continuum Mechanics (Inst. Teor. Prikl. Mekh. Sib. Otd. Akad. Nauk SSSR, Novosibirsk, 1985), Vol. 18, No. 5, pp. 101–115 [in Russian].

    Google Scholar 

  12. D. V. Rudenko and S. V. Utyuzhnikova, “Use of Dynamically Adaptive Grids for Modeling Three-Dimensional Unsteady Gas Flows with High Gradients,” Zh. Vychisl. Mat. Mat. Fiz. 42, 395–409 (2002) [Comput. Math. Math. Phys. 42, 377–390 (2002)].

    MATH  Google Scholar 

  13. N. A. Gil’manov, “Application of Dynamically Adaptive Grids to the Analysis of Flows with a Multiscale Structure,” Zh. Vychisl. Mat. Mat. Fiz. 41, 311–326 (2001) [Comput. Math. Math. Phys. 41, 289–303 (2001)].

    MathSciNet  Google Scholar 

  14. V. I. Mazhukin and L. Yu. Takoeva, “Construction Principles for Dynamically Adaptive Grids in One-Dimensional Boundary Value Problems,” Mat. Model. 2(3), 101–117 (1990).

    MATH  MathSciNet  Google Scholar 

  15. N. A. Dar’in, V. I. Mazhukin, and A. A. Samarskii, “Finite-Difference Method for Solving Gas Dynamics Equations on Dynamically Adaptive Grids,” Zh. Vychisl. Mat. Mat. Fiz. 28, 1210–1225 (1988).

    MATH  MathSciNet  Google Scholar 

  16. N. A. Dar’in and V. I. Mazhukin, “Mathematical Modeling of Unsteady Two-Dimensional Boundary Value Problems on Dynamically Adaptive Grids,” Mat. Model. 1(3), 29–43 (1989).

    MATH  MathSciNet  Google Scholar 

  17. V. I. Mazhukin, A. A. Samarskii, and A. V. Shapranov, “Dynamic Adaptation Method in the Burgers Problem,” Dokl. Akad. Nauk 333, 165–169 (1993).

    MathSciNet  Google Scholar 

  18. P. V. Breslavskii and V. I. Mazhukin, “Dynamic Adaptation Method in Gas Dynamics Problems,” Mat. Model. 7(12), 48–78 (1995).

    MathSciNet  Google Scholar 

  19. V. I. Mazhukin, A. A. Samarskii, and M. M. Chuiko, “A Method of Dynamic Adaptation for Numerical Solution to Time-Dependent Multidimensional Stefan Problems,” Dokl. Akad. Nauk 368, 307–310 (1999) [Dokl. Math. 60, 192–195 (1999)].

    MATH  MathSciNet  Google Scholar 

  20. M. M. Demin, V. I. Mazhukin, and A. A. Shapranov, “Dynamic Adaptation Method for a Laminar Combustion Problem,” Zh. Vychisl. Mat. Mat. Fiz. 41, 648–661 (2001) [Comput. Math. Math. Phys. 41, 609–621 (2001)].

    MathSciNet  Google Scholar 

  21. B. L. Rozhdestvenskii and N. N. Yanenko, Systems of Quasilinear Equations with Applications to Gas Dynamics (Nauka, Moscow, 1968) [in Russian].

    Google Scholar 

  22. W. H. Hui, P. Y. Li, and Z. W. Li, “A Unified Coordinate System for Solving the Two-Dimensional Euler Equations,” J. Comput. Phys. 153, 596–637 (1999).

    Article  MATH  MathSciNet  Google Scholar 

  23. W. H. Hui and S. Kudriakov, “A Unified Coordinate System for Solving the Three-Dimensional Euler Equations,” J. Comput. Phys. 172, 235–260 (2001).

    Article  MATH  MathSciNet  Google Scholar 

  24. Z. N. Wu, “A Note on the Unified Coordinate System for Computing Shock Waves,” J. Comput. Phys. 180, 110–119 (2002).

    Article  MATH  MathSciNet  Google Scholar 

  25. C. Mazeran and B. Despres, “Lagrangian Gas Dynamics in Two Dimensions and Lagrangian Systems,” Arch. Ration. Mech. Anal. 178, 327–372 (2005).

    Article  MATH  MathSciNet  Google Scholar 

  26. A. E. Dudorov, A. G. Zhilkin, and O. A. Kuznetsov, “High Accuracy Quasi-Monotone Difference Scheme for MHD Equations,” Mat. Model. 11(1), 101–116 (1999).

    MathSciNet  Google Scholar 

  27. P. D. Lax, “Weak Solutions of Nonlinear Hyperbolic Equations and Their Numerical Computation,” Commun. Pure Appl. Math. 7(1), 159–193 (1954).

    Article  MATH  MathSciNet  Google Scholar 

  28. S. R. Chakravarthy and S. Osher, “A New Class of High Accuracy TVD Schemes for Hyperbolic Conservation Laws,” AIAA Paper, No. 85-0363 (1985).

  29. A. Harten, “High Resolution Schemes for Hyperbolic Conservation Laws,” J. Comput. Phys. 49, 357–393 (1983).

    Article  MATH  MathSciNet  Google Scholar 

  30. K. V. Vyaznikov, V. F. Tishkin, and A. P. Favorskii, “Construction of High Accuracy Monotone Difference Schemes for Systems of Hyperbolic Equations,” Mat. Model. 1(5), 68–78 (1989).

    MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute of Astronomy, Russian Academy of Sciences, ul. Pyatnitskaya 48, Moscow, 119017, Russia

    A. G. Zhilkin

  2. Chelyabinsk State University, ul. Brat’ev Kashirinykh 129, Chelyabinsk, 454021, Russia

    A. G. Zhilkin

Authors
  1. A. G. Zhilkin
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to A. G. Zhilkin.

Additional information

Original Russian Text © A.G. Zhilkin, 2007, published in Zhurnal Vychislitel’noi Matematiki i Matematicheskoi Fiziki, 2007, Vol. 47, No. 11, pp. 1898–1912.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Zhilkin, A.G. A dynamic mesh adaptation method for magnetohydrodynamics problems. Comput. Math. and Math. Phys. 47, 1819–1832 (2007). https://doi.org/10.1134/S0965542507110085

Download citation

  • Received:

  • Accepted:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S0965542507110085

Keywords

Search

Navigation