Skip to main content
SpringerLink
Log in

Possible Scenarios of a Phase Transition from Isotropic Liquid to a Hexatic Phase in the Theory of Melting in Two-Dimensional Systems

  • Published:
Theoretical and Mathematical Physics Aims and scope Submit manuscript

Abstract

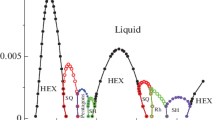
A two-stage process consisting of two continuous Berezinskii-Kosterlitz-Thouless-type transitions with an intermediate anisotropic liquid, a hexatic phase, is a well-known scenario of melting in two-dimensional systems. A direct first-order transition, similar to melting in three-dimensional systems, is another scenario variant. We prove the possibility in principle of the existence of a third scenario according to which melting occurs via two transitions, but in contrast to predictions of the Berezinskii-Kosterlitz-Thouless theory, the transition from an isotropic liquid to a hexatic phase is a first-order transition. Such a scenario was recently observed in a computer simulation of two-dimensional systems and then in a real experiment. Our proof is based on an analysis of branching solutions of an exact closed nonlinear integral equation for a two-particle conditional distribution function.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. K. J. Strandburg, ed., Bond-Orientational Order in Condensed Matter Systems, Springer, New York (1992).

    Google Scholar 

  2. W. F. Brinkman, D. S. Fisher, and D. E. Moncton, “Melting of two-dimensional solids,” Science, 217, 693–700 (1982).

    Article  ADS  Google Scholar 

  3. K. J. Strandburg, “Two-dimensional melting,” Rev. Modern Phys., 60, 161–207 (1988).

    Article  ADS  Google Scholar 

  4. H. Kleinert, Gauge Fields in Condensed Matter, Vol. 2, Stresses and Defects: Differential Geometry, Crystal Melting, World Scientific, Singapore (1989).

  5. M. A. Glaser and N. A. Clark, Melting and Liquid Structure in Two Dimensions (Adv. Chem. Phys., Vol. 83, I. Prigogine and S. A. Rice, eds.), Wiley, New York (1993).

  6. V. N. Ryzhov, E. E. Tareyeva, Yu. D. Fomin, and E. N. Tsiok, “Berezinskii-Kosterlitz-Thouless transition and two-dimensional melting,” Phys. Usp., 60, 857–885 (2017).

    Article  ADS  Google Scholar 

  7. B. I. Halperin and D. R. Nelson, “Theory of two-dimensional melting,” Phys. Rev. Lett., 41, 121–124 (1978).

    Article  ADS  MathSciNet  Google Scholar 

  8. D. R. Nelson and B. I. Halperin, “Dislocation-mediated melting in two dimensions,” Phys. Rev. B, 19, 2457–2484 (1979).

    Article  ADS  Google Scholar 

  9. A. P. Young, “Melting and the vector Coulomb gas in two dimensions,” Phys. Rev. B, 19, 1855–1866 (1979).

    Article  ADS  Google Scholar 

  10. V. L. Berezinskii, “Destruction of long-range order in one-dimensional and two-dimensional systems having a continuous symmetry group: I. Classical systems,” Sov. Phys. JETP, 32, 493–500 (1971).

    ADS  MathSciNet  Google Scholar 

  11. V. L. Berezinskii, “Destruction of long-range order in one-dimensional and two-dimensional systems with a continuous symmetry group: II. Quantum systems,” Sov. Phys. JETP, 34, 610–616 (1971).

    ADS  MathSciNet  Google Scholar 

  12. J. M. Kosterlitz and D. J. Thouless, “Ordering, metastability, and phase transitions in two-dimensional systems,” J. Phys. C, 6, 1181–1203 (1973).

    Article  ADS  Google Scholar 

  13. N. N. Bogolubov, “Quasi-averages in problems of statistical mechanics [in Russian],” in: Collected Scientific Works Statistical Mechanics, Vol. 6, Equilibrium Statistical Mechanics: 1945–1986, Nauka, Moscow (2006), pp. 236–360; English transl. in: Quantum Statistical Mechanics: Selected Works of N N Bogolubov (N. N. Bogolubov Jr., ed.), World Scientific, Hackensack, N. J. (2015), pp. 21–99.

    Google Scholar 

  14. N. D. Mermin, “Crystalline order in two dimensions,” Phys. Rev., 176, 250–254 (1968); Erratum, Phys. Rev. B, 20, 4762–4762 (1979); Erratum, Phys. Rev. B, 74, 149902 (2006).

    Article  ADS  Google Scholar 

  15. V. N. Ryzhov, “Statistical theory of crystallization in classical systems,” Theor. Math. Phys., 55, 399–405 (1983).

    Article  MathSciNet  Google Scholar 

  16. V. N. Ryzhov and E. E. Tareeva, “Towards a statistical theory of freezing,” Phys. Lett. A, 75, 88–90 (1979).

    Article  ADS  Google Scholar 

  17. V. N. Ryzhov and E. E. Tareeva, “Statistical theory of crystallization in a system of hard spheres,” Theor. Math. Phys., 48, 835–840 (1981).

    Article  Google Scholar 

  18. M. Baus, “The present status of the density-functional theory of the liquid-solid transition,” J. Phys.: Condens. Matter, 2, 2111–2126 (1990).

    ADS  Google Scholar 

  19. Y. Singh, “Density-functional theory of freezing and properties of the ordered phase,” Phys. Rep., 207, 351–444 (1991).

    Article  ADS  Google Scholar 

  20. V. N. Ryzhov and E. E. Tareyeva, “Two-stage melting in two dimensions: First-principles approach,” Phys. Rev. B, 51, 8789–8794 (1995).

    Article  ADS  Google Scholar 

  21. V. N. Ryzhov and E. E. Tareeva, “Microscopic description of two-stage melting in two dimensions,” JETP, 81, 1115–1123 (1995).

    ADS  Google Scholar 

  22. V. N. Ryzhov and E. E. Tareyeva, “Melting in two dimensions: First-order versus continuous transition,” Phys. A, 314, 396–404 (2002).

    Article  Google Scholar 

  23. L. M. Pomirchi, V. N. Ryzhov, and E. E. Tareeva, “Melting of two-dimensional systems: Dependence of the type of transition on the radius of the potential,” Theor. Math. Phys., 130, 101–110 (2002).

    Article  MATH  Google Scholar 

  24. E. S. Chumakov, Y. D. Fomin, E. L. Shangina, E. E. Tareyeva, E. N. Tsiok, and V. N. Ryzhov, “Phase diagram of the system with the repulsive shoulder potential in two dimensions: Density functional approach,” Phys. A, 432, 279–286 (2015); arXiv:1412.0466v1 [cond-mat.stat-mech] (2014).

    Article  MathSciNet  MATH  Google Scholar 

  25. V. N. Ryzhov and E. E. Tareeva, “Microscopic approach to calculation of the shear and bulk moduli and the frank constant in two-dimensional melting,” Theor. Math. Phys., 92, 922–930 (1992).

    Article  Google Scholar 

  26. E. P. Bernard and W. Krauth, “Two-step melting in two dimensions: First-order liquid-hexatic transition,” Phys. Rev. Lett., 107, 155704 (2011); arXiv:1102.4094v2 [cond-mat.soft] (2011).

    Article  ADS  Google Scholar 

  27. M. Engel, J. A. Anderson, S. C. Glotzer, M. Isobe, E. P. Bernard, and W. Krauth, “Hard-disk equation of state: First-order liquid-hexatic transition in two dimensions with three simulation methods,” Phys. Rev. E, 87, 042134 (2013); arXiv:1211.1645v2 [cond-mat.stat-mech] (2012).

    Article  ADS  Google Scholar 

  28. W. Qi and M. Dijkstra, “Destabilisation of the hexatic phase in systems of hard disks by quenched disorder due to pinning on a lattice,” Soft Matter, 11, 2852–2856 (2015).

    Article  ADS  Google Scholar 

  29. S. C. Kapfer and W. Krauth, “Two-dimensional melting: From liquid-hexatic coexistence to continuous transitions,” Phys. Rev. Lett., 114, 035702 (2015); arXiv:1406.7224v2 [cond-mat.stat-mech] (2014).

    Article  ADS  Google Scholar 

  30. W. Qi, A. P. Gantapara, and M. Dijkstra, “Two-stage melting induced by dislocations and grain boundaries in monolayers of hard spheres,” Soft Matter, 10, 5449–5457 (2014); arXiv:1307.1311v2 [cond-mat.soft] (2013).

    Article  ADS  Google Scholar 

  31. W.-K. Qi, S.-M. Qin, X.-Y. Zhao, and Y. Chen, “Coexistence of hexatic and isotropic phases in two-dimensional Yukawa systems,” J. Phys.: Condens. Matter, 20, 245102 (2008).

    Google Scholar 

  32. A. L. Thorneywork, J. L. Abbott, D. G. A. L. Aarts, and R. P. A. Dullens, “Two-dimensional melting of colloidal hard spheres,” Phys. Rev. Lett., 118, 158001 (2017).

    Article  ADS  Google Scholar 

  33. E. A. Gaiduk, Yu. D. Fomin, E. N. Tsiok, and V. N. Ryzhov, “The influence of random pinning on the melting scenario of two-dimensional soft-disk systems,” arXiv:1812.02007v2 [cond-mat.soft] (2018).

    Google Scholar 

  34. Yu. D. Fomin, E. A. Gaiduk, E. N. Tsiok, and V. N. Ryzhov, “The phase diagram and melting scenarios of twodimensional Hertzian spheres,” Molecular Phys., 116, 3258–3270 (2018); arXiv:1801.10029v1 [cond-mat.soft] (2018).

    Article  ADS  Google Scholar 

  35. E. N. Tsiok, D. E. Dudalov, Yu. D. Fomin, and V. N. Ryzhov, “Random pinning changes the melting scenario of a two-dimensional core-softened potential system,” Phys. Rev. E, 92, 032110 (2015); arXiv:1507.01802v2 [cond-mat.soft] (2015).

    Article  ADS  Google Scholar 

  36. E. N. Tsiok, Y. D. Fomin, and V. N. Ryzhov, “Random pinning elucidates the nature of melting transition in two-dimensional core-softened potential system,” Phys. A, 490, 819–827 (2018); arXiv:1701.03643v1 [condmat. soft] (2017).

    Article  Google Scholar 

  37. N. P. Kryuchkov, S. O. Yurchenko, Y. D. Fomin, E. N. Tsiok, and V. N. Ryzhov, “Complex crystalline structures in a two-dimensional core-softened system,” Soft Matter, 14, 2152–2162 (2018); arXiv:1712.04707v1 [condmat.soft] (2017).

    Article  ADS  Google Scholar 

  38. S. T. Chui, “Grain-boundary theory of melting in two dimensions,” Phys. Rev. B, 28, 178–194 (1983).

    Article  ADS  Google Scholar 

  39. V. N. Ryzhov, “Dislocation-disclination melting of two-dimensional lattices,” JETP, 73, 899–905 (1991).

    Google Scholar 

  40. V. N. Ryzhov, “Disclination-mediated melting of two-dimensional lattices,” Theor. Math. Phys., 88, 990–997 (1991).

    Article  MathSciNet  Google Scholar 

  41. V. N. Ryzhov, E. E. Tareyeva, Yu. D. Fomin, E. N. Tsiok, and E. S. Chumakov, “Renormalization group study of the melting of a two-dimensional system of collapsing hard disks,” Theor. Math. Phys., 191, 842–855 (2017).

    Article  MathSciNet  MATH  Google Scholar 

  42. V. N. Ryzhov and E. E. Tareeva, “Statistical mechanics of vortex system in a thin-film superconductor in the ring approximation,” Theor. Math. Phys., 96, 1062–1068 (1993).

    Article  Google Scholar 

  43. V. N. Ryzhov and E. E. Tareyeva, “Statistical mechanics of vortex systems in two-dimensional superconductors,” Phys. Rev. B, 48, 12907–12911 (1993).

    Article  ADS  Google Scholar 

  44. V. N. Ryzhov and E. E. Tareyeva, “Results for the phase diagram of the vortex system in two-dimensional superconductors,” Phys. Rev. B, 49, 6162–6173 (1994).

    Article  ADS  Google Scholar 

  45. D. Yu. Irz, V. N. Ryzhov, and E. E. Tareyeva, “Vortex-vortex interaction in superconducting film of finite thickness,” Phys. Lett. A, 207, 374–378 (1995).

    Article  ADS  MATH  Google Scholar 

  46. D. Yu. Irz, V. N. Ryzhov, and E. E. Tareeva, “The statistical mechanics of vortex system in thin superconducting film in cycle approximation: III. Finite size vortex core effects,” Theor. Math. Phys., 107, 499–510 (1996).

    Article  MATH  Google Scholar 

  47. D. Yu. Irz, V. N. Ryzhov, and E. E. Tareyeva, “First-order vortex unbinding transition in thin superconducting films,” Phys. Rev. B, 54, No. 5, 3051–3054 (1996).

    Article  ADS  Google Scholar 

  48. V. N. Ryzhov and E. E. Tareeva, “Microscopic description of bond orientational order in simple liquids,” Theor. Math. Phys., 73, 1344–1352 (1987).

    Article  Google Scholar 

  49. V. N. Ryzhov and E. E. Tareyeva, “Bond orientational order in simple liquids,” J. Phys. C, 21, 819–824 (1988).

    Article  ADS  Google Scholar 

  50. V. N. Ryzhov, “Orientational ordering of bonds in simple three-dimensional liquids,” Theor. Math. Phys., 80, 745–752 (1989).

    Article  Google Scholar 

  51. V. N. Ryzhov, “Local structure and bond orientational order in a Lennard-Jones liquid,” J. Phys.: Condens. Matter, 2, 5855–5865 (1990).

    ADS  Google Scholar 

  52. R. Balescu, Equilibrium and Nonequilibrium Statistical Mechanics, Wiley, New York (1975).

    MATH  Google Scholar 

  53. N. N. Bogoliubov, Collected Scientific Works Statistical Mechanics [in Russian], Vol. 6, Equilibrium Statistical Mechanics: 1945–1986, Nauka, Moscow (2006).

  54. E. E. Tareyeva and V. N. Ryzhov, “Classical many-particle distribution functions: some new applications,” PEPAN, 31, No. 7B, 184–189 (2000).

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Vereshchagin Institute for High Pressure Physics, RAS, Troitsk, Moscow Oblast, Russia

    V. N. Ryzhov & E. E. Tareyeva

Authors
  1. V. N. Ryzhov
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. E. E. Tareyeva
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to E. E. Tareyeva.

Additional information

This research was supported by the Russian Foundation for Basic Research (Grant No. 17-02-00320).

Translated from Teoreticheskaya i Matematicheskaya Fizika, Vol. 200, No. 1, pp. 147–157, July, 2019.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ryzhov, V.N., Tareyeva, E.E. Possible Scenarios of a Phase Transition from Isotropic Liquid to a Hexatic Phase in the Theory of Melting in Two-Dimensional Systems. Theor Math Phys 200, 1053–1062 (2019). https://doi.org/10.1134/S0040577919070092

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S0040577919070092

Keywords

Search

Navigation