Skip to main content
SpringerLink
Log in

Nonlocal Reductions of The Multicomponent Nonlinear Schrödinger Equation on Symmetric Spaces

  • Published:
Theoretical and Mathematical Physics Aims and scope Submit manuscript

Abstract

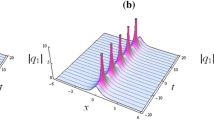
Our aim is to develop the inverse scattering transform for multicomponent generalizations of nonlocal reductions of the nonlinear Schrödinger (NLS) equation with \(\mathcal{PT}\) symmetry related to symmetric spaces. This includes the spectral properties of the associated Lax operator, the Jost function, the scattering matrix, the minimum set of scattering data, and the fundamental analytic solutions. As main examples, we use theManakov vector Schrödinger equation (related to A.III-symmetric spaces) and the multicomponent NLS (MNLS) equations of Kulish–Sklyanin type (related to BD.I-symmetric spaces). Furthermore, we obtain one- and two-soliton solutions using an appropriate modification of the Zakharov–Shabat dressing method. We show that the MNLS equations of these types admit both regular and singular soliton configurations. Finally, we present different examples of one- and two-soliton solutions for both types of models, subject to different reductions.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. V. E. Zakharov and A. B. Shabat, “A scheme for integrating the nonlinear equations of mathematical physics by the method of the inverse scattering problem: I,” Funct. Anal. Appl., 8, 226–235 (1974); “Integration of nonlinear equations of mathematical physics by the method of inverse scattering: II,” Funct. Anal. Appl., 13, 166–174 (1979).

    Article  MATH  Google Scholar 

  2. L. A. Takhtadzhyan and L. D. Faddeev, Hamiltonian Methods in the Theory of Solitons [in Russian], Nauka, Moscow (1986); English transl., Springer, Berlin (2007).

    MATH  Google Scholar 

  3. V. E. Zakharov, S. V. Manakov, S. P. Novikov and L. P. Pitaevskii, Theory of Solitons: The inverse Scattering Method [in Russian], Nauka, Moscow (1980); English transl., Plenum, New York (1984).

    MATH  Google Scholar 

  4. V. S. Gerdjikov, G. Vilasi, and A. B. Yanovski, Integrable Hamiltonian Hierarchies: Spectral and Geometric Methods (Lect. Notes Phys., Vol., 748), Springer, Berlin (2008).

    Book  MATH  Google Scholar 

  5. M. J. Ablowitz, B. Prinari, and A. D. Trubatch, Discrete and Continuous Nonlinear Schrödinger Systems (London Math. Soc. Lect. Note Ser., Vol. 302), Cambridge Univ. Press, Cambridge (2004).

    MATH  Google Scholar 

  6. R. Beals and R. R. Coifman, “Scattering and inverse scattering for first order systems,” Commun. Pure Appl. Math., 37, 39–90 (1984); “Scattering and inverse scattering for first order systems: II,” Inverse Problems, 3, 577–594 (1987).

    Article  MathSciNet  MATH  Google Scholar 

  7. P. G. Drazin and R. S. Johnson, Solitons: An Introduction, Cambridge Univ. Press, Cambridge (1989).

    Book  MATH  Google Scholar 

  8. V. S. Gerdjikov, “Generalised Fourier transforms for the soliton equations: Gauge-covariant formulation,” Inverse Problems, 2, 51–74 (1986).

    Article  ADS  MathSciNet  Google Scholar 

  9. S. V. Manakov, “On the theory of two-dimensional stationary self-focusing of electromagnetic waves,” Sov. Phys. JETP, 38, 248–253 (1974).

    ADS  Google Scholar 

  10. C. R. Menyuk, “Nonlinear pulse propagation in birefringent optical fibres,” IEEE J. Quantum Electron, 23, 174–176 (1987).

    Article  ADS  Google Scholar 

  11. J. R. Ackerhalt and P. W. Milonni, “Solitons and four-wave mixing,” Phys. Rev. A, 33, 3185–3198 (1986).

    Article  ADS  Google Scholar 

  12. S. Helgason, Differential Geometry, Lie Groups, and Symmetric Spaces (Grad. Stud. Math., Vol. 34), Amer. Math. Soc., Providence, R. I. (2001).

    Book  MATH  Google Scholar 

  13. R. Gilmore, Lie Groups, Lie Algebras, and Some of Their Applications, Dover, Mineola, N. Y. (2005).

    MATH  Google Scholar 

  14. A. P. Fordy and P. P. Kulish, “Nonlinear Schrödinger equations and simple Lie algebras,” Commun. Math. Phys., 89, 427–443 (1983).

    Article  ADS  MATH  Google Scholar 

  15. C. Athorne and A. Fordy, “Generalised KdV and MKdV equations associated with symmetric spaces,” J. Phys. A: Math. Gen., 20, 1377–1386 (1987).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  16. A. P. Fordy, “Derivative nonlinear Schrödinger equations and Hermitian symmetric spaces,” J. Phys. A: Math. Gen., 17, 1235–1245 (1984).

    Article  ADS  MATH  Google Scholar 

  17. O. Loos, Symmetric Spaces, Vols. 1 and 2, W. A. Benjamin, New York (1969).

    MATH  Google Scholar 

  18. P. P. Kulish and E. K. Sklyanin, “O(N)-invariant nonlinear Schrödinger equation: A new completely integrable system,” Phys. Lett. A, 84, 349–352 (1981).

    Article  ADS  MathSciNet  Google Scholar 

  19. V. G. Drinfeld and V. V. Sokolov, “Lie algebras and equations of Korteweg–de Vries type,” J. Soviet Math., 30, 1975–2036 (1985).

    Article  MATH  Google Scholar 

  20. V. S. Gerdjikov, G. G. Grahovski, and N. A. Kostov, “Reductions of N-wave interactions related to low-rank simple Lie algebras: I. Z2-reductions,” J. Phys. A: Math. Gen., 34, 9425–9461 (2001); arXiv:nlin.SI/0006001v3 (2000).

    Article  ADS  MATH  Google Scholar 

  21. V. S. Gerdjikov, G. G. Grahovski, R. I. Ivanov, and N. A. Kostov, “N-wave interactions related to simple Lie algebras: Z2-reductions and soliton solutions,” Inverse Problems, 17, 999–1015 (2001); arXiv:nlin.SI/0009034v3 (2000).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  22. A. V. Mikhailov, “The reduction problem and the inverse scattering problem,” Phys. D, 3, 73–117 (1981).

    Article  MATH  Google Scholar 

  23. M. Ablowitz and Z. Musslimani, “Integrable nonlocal nonlinear Schrödinger equation,” Phys. Rev. Lett., 110, 064105 (2013).

    Article  ADS  Google Scholar 

  24. M. Ablowitz and Z. Musslimani, “Integrable discrete PT symmetric model,” Phys. Rev. E, 90, 032912 (2014).

    Article  ADS  Google Scholar 

  25. M. J. Ablowitz and Z. H. Musslimani, “Inverse scattering transform for the integrable nonlocal nonlinear Schrödinger equation,” Nonlinearity, 29, 915–946 (2016).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  26. V. S. Gerdjikov and A. Saxena, “Complete integrability of nonlocal nonlinear Schrödinger equation,” J. Math. Phys., 58, 013502 (2017); arXiv:1510.0480v2 [nlin.SI] (2015).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  27. V. E. Zakharov and A. V. Mikhailov, “On the integrability of classical spinor models in two-dimensional space–time,” Commun. Math. Phys., 74, 21–40 (1980).

    Article  ADS  MathSciNet  Google Scholar 

  28. F. K. Abdullaev, Y. V. Kartashov, V. V. Konotop, and D. A. Zezyulin, “Solitons in PT-symmetric nonlinear lattices,” Phys. Rev. A, 83, 041805 (2011).

    Article  ADS  Google Scholar 

  29. I. V. Barashenkov, “Hamiltonian formulation of the standard PT -symmetric nonlinear Schrödinger dimer,” Phys. Rev. A, 90, 045802 (2014).

    Article  ADS  Google Scholar 

  30. I. V. Barashenkov, D. E. Pelinovsky, and P. Dubard, “Dimer with gain and loss: Integrability and PT -symmetry restoration,” J. Phys. A: Math. Theor., 48, 325201 (2015).

    Article  MathSciNet  MATH  Google Scholar 

  31. C. E. Rüter, K. G. Makris, R. El-Ganainy, D. N. Christodoulides, M. Segev, and D. Kip, “Observation of parity-time symmetry in optics,” Nature Phys., 6, 192–195 (2010).

    Article  ADS  Google Scholar 

  32. A. A. Zyablovsky, A. P. Vinogradov, A. A. Pukhov, A. V. Dorofeenko, and A. A. Lisyansky, “PT -symmetry in optics,” Phys. Usp., 57, 1063–1082 (2014).

    Article  ADS  Google Scholar 

  33. C. M. Bender and S. Boettcher, “Real spectra in non-Hermitian Hamiltonians having PT symmetry,” Phys. Rev. Lett., 80, 5243–5246 (1998); C. M. Bender, S. Boettcher, and P. N. Meisinger, “PT -symmetric quantum mechanics,” J. Math. Phys., 40, 2201–2229 (1999).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  34. A. Mostafazadeh, “Pseudo-hermiticity versus PT -symmetry: The necessary condition for the reality of the spectrum of a non-Hermitian Hamiltonian,” J. Math. Phys., 43, 205–214 (2002); arXiv:math-ph/0107001v3 (2001); “Pseudo-hermiticity versus PT -symmetry: II. A complete characterization of non-Hermitian Hamiltonians with a real spectrum,” J. Math. Phys., 43, 2814–2816 (2002); arXiv:math-ph/0110016v2 (2001); “Pseudo-Hermiticity versus PT -symmetry: III: Equivalence of pseudo-hermiticity and the presence of antilinear symmetries,” J. Math. Phys., 43, 3944–3951 (2002); arXiv:math-ph/0203005v2 (2002).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  35. A. Mostafazadeh, “Pseudo-Hermiticity and generalized PT -and CPT -symmetries,” J. Math. Phys., 44, 974–989 (2003); arXiv:math-ph/0209018v3 (2002); “Exact PT -symmetry is equivalent to Hermiticity,” J. Phys. A: Math. Gen., 36, 7081–7091 (2003); arXiv:quant-ph/0304080v2 (2003).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  36. C. M. Bender, “Making sense of non-Hermitian Hamiltonians,” Rep. Progr. Phys., 70, 947–1018 (2007); arXiv: hep-th/0703096v1 (2007).

    Article  ADS  MathSciNet  Google Scholar 

  37. A. Fring, “PT -symmetric deformations of integrable models,” Phil. Trans. Roy. Soc. London Ser. A, 371, 20120046 (2013); arXiv:1204.2291v1 [hep-th] (2012).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  38. A. Fring, “PT -symmetric deformations of the Korteweg–de Vries equation,” J. Phys. A: Math. Theor., 40, 4215–4224 (2007); arXiv:math-ph/0701036v1 (2007).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  39. V. S. Gerdjikov, “Basic aspects of soliton theory,” in: Proc. Sixth Intl. Conf. on Geometry, Integrability, and Quantization (Varna, Sofia, Bulgaria, 2–10 June 2005, I. M. Mladenov and A. C. Hirshfeld, eds.), Softex, Sofia (2005), pp. 78–125.

    Google Scholar 

  40. V. S. Gerdjikov, D. J. Kaup, N. A. Kostov, and T. I. Valchev, “On classification of soliton solutions of multicomponent nonlinear evolution equations,” J. Phys. A: Math. Theor., 41, 315213 (2008).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  41. V. S. Gerdjikov, “On nonlocal models of Kulish–Sklyanin type and generalized Fourier transforms,” in: Advanced Computing in Industrial Mathematics (Stud. Comput. Intell., Vol. 681, K. Georgiev, M. Todorov, and I. Georgiev, eds.), Springer, Cham (2017), pp. 37–52.

    Chapter  Google Scholar 

  42. V. S. Gerdjikov, “Kulish–Sklyanin-type models: Integrability and reductions,” Theor. Math. Phys., 192, 1097–1114 (2017); arXiv:1702.04010v2 [nlin.SI] (2017).

    Article  MathSciNet  MATH  Google Scholar 

  43. V. S. Gerdjikov and G. G. Grahovski, “Multi-component NLS models on symmetric spaces: Spectral properties versus representations theory,” SIGMA, 6, 044 (2010).

    MathSciNet  MATH  Google Scholar 

  44. V. S. Gerdjikov, “On reductions of soliton solutions of multi-component NLS models and spinor Bose–Einstein condensates,” in: Application of Mathematics in Technical and Natural Sciences (AIP Conf. Proc., Vol. 1186, M. D. Todorov and C. I. Christov, eds.), AIP, Melville, N. Y. (2009), pp. 15–27.

    Google Scholar 

  45. G. G. Grahovski, “On the reductions and scattering data for the generalized Zakharov–Shabat systems,” in: Nonlinear Physics: Theory and Experiment II (M. J. Ablowitz, M. Boiti, F. Pempinelli, and B. Prinari, eds.), World Scientific, Singapore (2003), pp. 71–78.

    Chapter  Google Scholar 

  46. T. I. Valchev, “On Mikhailov’s reduction group,” Phys. Lett. A, 379, 1877–1880 (2015).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  47. V. A. Atanasov, V. S. Gerdjikov, G. G. Grahovski, and N. A. Kostov, “Fordy–Kulish model and spinor Bose–Einstein condensate,” J. Nonlinear Math. Phys., 15, 291–298 (2008).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  48. G. G. Grahovski, V. S. Gerdjikov, and N. A. Kostov, “On the multicomponent NLS type equations on symmetric spaces: Reductions and soliton solutions,” in: Proc. Sixth Intl. Conf. on Geometry, Integrability, and Quantization (Varna, Sofia, Bulgaria, 2–10 June 2005, I. M. Mladenov and A. C. Hirshfeld, eds.), Softex, Sofia (2005), pp. 203–217.

    Google Scholar 

  49. J. Yang, Nonlinear Waves in Integrable and Nonintegrable Systems, SIAM, Philadelphia (2010).

    Book  MATH  Google Scholar 

  50. V. S. Gerdjikov and P. P. Kulish, “The generating operator for the n×n linear system,” Phys. D, 3, 549–564 (1981).

    Article  MathSciNet  MATH  Google Scholar 

  51. R. Ivanov, “On the dressing method for the generalised Zakharov–Shabat system,” Nucl. Phys. B, 694, 509–524 (2004); arXiv:math-ph/0402031v1 (2004).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  52. V. S. Gerdjikov, G. G. Grahovski, and N. A. Kostov, “On N-wave type systems and their gauge equivalent,” Eur. J. Phys. B, 29, 243–248 (2002).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  53. V. S. Gerdjikov, “Algebraic and analytic aspects of N-wave type equations,” in: The Legacy of the Inverse Scattering Transform in Applied Mathematics (Contemp. Math., Vol. 301, J. Bona, R. Choudhury, and D. Kaup, eds.), Amer. Math. Soc., Providence, R. I. (2002), pp. 35–68; arXiv:nlin.SI/0206014v1 (2002).

    Chapter  Google Scholar 

  54. A. B. Shabat, “Inverse-scattering problem for a system of differential equations,” Funct. Anal. Appl., 9, 244–247 (1975); “An inverse scattering problem,” Funct. Anal. Appl., 15, 1824–1834 (1979).

    Article  MathSciNet  MATH  Google Scholar 

  55. E. V. Doktorov and S. B. Leble, A Dressing Method in Mathematical Physics (Math. Phys. Stud., Vol. 28), Springer, Dordrecht (2007).

    MATH  Google Scholar 

  56. V. S. Gerdjikov, G. G. Grahovski, A. V. Mikhailov, and T. I. Valchev, “Rational bundles and recursion operators for integrable equations on A.III-type symmetric spaces,” Theor. Math. Phys., 167, 740–750 (2011).

    Article  MathSciNet  MATH  Google Scholar 

  57. V. S. Gerdjikov, G. G. Grahovski, A. V. Mikhailov, and T. I. Valchev, “Polynomial bundles and generalised Fourier transforms for integrable equations on A.III-type symmetric spaces,” SIGMA, 7, 096 (2011).

    MathSciNet  MATH  Google Scholar 

  58. G. G. Grahovski and M. Condon, “On the Caudrey–Beals–Coifman system and the gauge group action,” J. Nonlin. Math. Phys., 15 (suppl. 3), 197–208 (2008); arXiv:0710.3302v1 [nlin.SI] (2007).

    Article  MathSciNet  MATH  Google Scholar 

  59. G. G. Grahovski, V. S. Gerdjikov, N. A. Kostov, and V. A. Atanasov, “New integrable multi-component NLS type equations on symmetric spaces: ℤ4 and ℤ6 reductions,” in: Proc. Seventh Intl. Conf. on Geometry, Integrability, and Quantization (I. M. Mladenov and M. de Leon, eds.), Softex, Sofia (2006), pp. 154–175.

    Google Scholar 

  60. M. Gürses, “Nonlocal Fordy–Kulish equations on symmetric spaces,” Phys. Lett. A, 381, 1791–1794 (2017); arXiv:1702.03731v2 [nlin.SI] (2017).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  61. M. J. Ablowitz, X.-D. Luo, and Z. H. Musslimani, “Inverse scattering transform for the nonlocal nonlinear Schrödinger equation with nonzero boundary conditions,” arXiv:1612.02726v1 [nlin.SI] (2016).

    MATH  Google Scholar 

  62. M. Li and T. Xu, “Dark and antidark soliton interactions in the nonlocal nonlinear Schrödinger equation with the self-induced parity-time-symmetric potential,” Phys. Rev. E, 91, 033202 (2015).

    Article  ADS  MathSciNet  Google Scholar 

  63. V. S. Gerdjikov, G. G. Grahovski, and R. I. Ivanov, “The N-wave equations with PT-symmetry,” Theor. Math. Phys., 188, 1305–1321 (2016).

    Article  MathSciNet  MATH  Google Scholar 

  64. V. S. Gerdjikov, G. G. Grahovski, and R. I. Ivanov, “On integrable wave interactions and Lax pairs on symmetric spaces,” Wave Motion, 71, 53–70 (2017).

    Article  MathSciNet  Google Scholar 

  65. A. G. Reiman, “A unified Hamiltonian system on polynomial bundles, and the structure of stationary problems [in Russian],” Zap. Nauch. Semin. LOMI, 131, 118–127 (1983).

    MATH  Google Scholar 

  66. A. G. Reiman and M. A. Semenov-Tyan-Shanskii, “Current algebras and nonlinear partial differential equations,” Dokl. Akad. Nauk SSSR, 21, 630–634 (1980).

    Google Scholar 

  67. V. S. Gerdjikov, N. A. Kostov, and T. I. Valchev, “N-wave equations with orthogonal algebras: ℤ2 and ℤ2×ℤ2 reductions and soliton solutions,” SIGMA, 3, 039 (2007).

    MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematical Sciences, University of Essex, Colchester, UK

    G. G. Grahovski, J. I. Mustafa & H. Susanto

Authors
  1. G. G. Grahovski
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. J. I. Mustafa
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. H. Susanto
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to G. G. Grahovski.

Additional information

Prepared from an English manuscript submitted by the authors; for the Russian version, see Teoreticheskaya i Matematicheskaya Fizika, Vol. 197, No. 1, pp. 45–67, October, 2018.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Grahovski, G.G., Mustafa, J.I. & Susanto, H. Nonlocal Reductions of The Multicomponent Nonlinear Schrödinger Equation on Symmetric Spaces. Theor Math Phys 197, 1430–1450 (2018). https://doi.org/10.1134/S0040577918100033

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S0040577918100033

Keywords

Search

Navigation