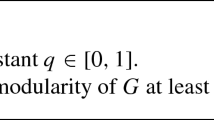Skip to main content
SpringerLink
Log in

New Modularity Bounds for Graphs \(G(n,r,s)\) and \(G_p(n,r,s)\)

  • LARGE SYSTEMS
  • Published:
Problems of Information Transmission Aims and scope Submit manuscript

Abstract

We analyze the behavior of the modularity of \(G(n,r,s)\) graphs in the case of \(r=o(\sqrt{{n}})\) and \(n\to\infty\) and also that of \(G_p(n,r,s)\) graphs for fixed \(r\) and \(s\) as \(n\to\infty\). For \(G(n,r,s)\) graphs with \(r\ge cs^2\), we obtain substantial improvements of previously known upper bounds. Upper and lower bounds previously obtained for \(G(n,r,s)\) graphs are extended to the family of \(G_p(n,r,s)\) graphs with \(p=p(n)=\omega\bigl(n^{-\frac{r-s-1}{2}}\bigr)\) and fixed \(r\) and \(s\).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1.

Similar content being viewed by others

References

  1. Newman, M.E.J. and Girvan, M., Finding and Evaluating Community Structure in Networks, Phys. Rev. E, 2004, vol. 69, no. 2, pp. 026113 (15 pp.). https://doi.org/10.1103/PhysRevE.69.026113

    Article  Google Scholar 

  2. Lancichinetti, A. and Fortunato, S., Limits of Modularity Maximization in Community Detection, Phys. Rev. E, 2011, vol. 84, no. 6, pp. 066122 (8 pp.). https://doi.org/10.1103/PhysRevE.84.066122

    Article  Google Scholar 

  3. Miasnikof, P., Prokhorenkova, L., Shestopaloff, A.Y., and Raigorodskii, A., A Statistical Test of Heterogeneous Subgraph Densities to Assess Clusterability, Learning and Intelligent Optimization (13th Int. Conf. LION’13, Chania, Crete, Greece, May 27–31, 2019, Revised Selected Papers), Matsatsinis, N.F., Marinakis, Y., and Pardalos, P.M., Eds., Lect. Notes Comput. Sci., vol. 11968, Cham: Springer, 2000, pp. 17–29. https://doi.org/10.1007/978-3-030-38629-0_2

  4. Newman, M.E.J., Fast Algorithm for Detecting Community Structure in Networks, Phys. Rev. E, 2004, vol. 69, no. 6, pp. 066133 (5 pp.). https://doi.org/10.1103/PhysRevE.69.066133

    Article  Google Scholar 

  5. Ostroumova Prokhorenkova, L., General Results on Preferential Attachment and Clustering Coefficient, Optim. Lett., 2017, vol. 11, no. 2, pp. 279–298. https://doi.org/10.1007/s11590-016-1030-8

    Article  MathSciNet  Google Scholar 

  6. Porter, M.A., Onnela, J.-P., and Mucha, P.J., Communities in Networks, Notices Amer. Math. Soc., 2009, vol. 56, no. 9, pp. 1082–1097. Available at https://www.ams.org/notices/200909/rtx090901082p.pdf.

    MathSciNet  MATH  Google Scholar 

  7. Brandes, U., Delling, D., Gaertler, M., Görke, R., Hoefer, M., Nikoloski, Z., and Wagner, D., On Finding Graph Clusterings with Maximum Modularity, Graph-Theoretic Concepts in Computer Science (33rd Int. Workshop WG’2007, Dornburg, Germany, June 21–23, 2007, Revised Papers), Brandstadt, A., Kratsch, D., and Muller, H., Eds., Lect. Notes Comput. Sci., vol. 4769, Berlin: Springer, 2007, pp. 121–132. https://doi.org/10.1007/978-3-540-74839-7_12

  8. De Montgolfier, F., Soto, M., and Viennot, L., Asymptotic Modularity of Some Graph Classes, Algorithms and Computation (Proc. 22nd Int. Sympos. ISAAC’2011, Yokahama, Japan, Dec. 5–8, 2011), Asano, T., Nakano, S., Okamoto, Y., and Watanabe, O., Eds., Lect. Notes Comput. Sci., vol. 7074, Berlin: Springer, 2011, pp. 435–444. https://doi.org/10.1007/978-3-642-25591-5_45

  9. Trajanovski, S., Wang, H., and Van Mieghem, P., Maximum Modular Graphs, Eur. Phys. J. B, 2012, vol. 85, no. 7, Art. 244 (14 pp.) https://doi.org/10.1140/epjb/e2012-20898-3

    Article  MathSciNet  Google Scholar 

  10. McDiarmid, C. and Skerman, F., Modularity of Regular and Treelike Graphs, J. Complex Netw., 2018, vol. 6, no. 4, pp. 596–619. https://doi.org/10.1093/comnet/cnx046

    Article  MathSciNet  Google Scholar 

  11. Ostroumova Prokhorenkova, L., Prałat, P., and Raigorodskii, A., Modularity in Several Random Graph Models, Electron. Notes Discrete Math., 2017, vol. 61, pp. 947–953. https://doi.org/10.1016/j.endm.2017.07.058

    Article  Google Scholar 

  12. Bollobás, B., The Isoperimetric Number of Random Regular Graphs, European J. Combin., 1988, vol. 9, no. 3, pp. 241–244. https://doi.org/10.1016/S0195-6698(88)80014-3

    Article  MathSciNet  Google Scholar 

  13. McDiarmid, C. and Skerman, F., Modularity of Erdős-Rényi Random Graphs, Random Structures Algorithms, 2020, vol. 57, no. 1, pp. 211–243. https://doi.org/10.1002/rsa.20910

    Article  MathSciNet  Google Scholar 

  14. Bollobás, B., Narayanan, B.P., and Raigorodskii, A.M., On the Stability of the Erdős-Ko-Rado Theorem, J. Combin. Theory Ser. A, 2016, vol. 137, pp. 64–78. https://doi.org/10.1016/j.jcta.2015.08.002

    Article  MathSciNet  Google Scholar 

  15. MacWilliams, F.J. and Sloane, N.J.A., The Theory of Error-Correcting Codes, Amsterdam: North-Holland, 1977. Translated under the title Teoriya kodov, ispravlyayushchikh oshibki, Moscow: Svyaz’, 1979.

    MATH  Google Scholar 

  16. Frankl, P. and Wilson, R.M., Intersection Theorems with Geometric Consequences, Combinatorica, 1981, vol. 1, no. 4, pp. 357–368. https://doi.org/10.1007/BF02579457

    Article  MathSciNet  Google Scholar 

  17. Kahn, J. and Kalai, G., A Counterexample to Borsuk’s Conjecture, Bull. Amer. Math. Soc. (N.S.), 1993, vol. 29, no. 1, pp. 60–62. https://doi.org/10.1090/S0273-0979-1993-00398-7

    Article  MathSciNet  Google Scholar 

  18. Raigorodskii, A.M., Around Borsuk’s Hypothesis, Sovrem. Mat. Fundam. Napravl., 2007, vol. 23, pp. 147–164 [J. Math. Sci. (N.Y.) (Engl. Transl.), 2007, vol. 154, no. 4, pp. 604–623]. https://doi.org/10.1007/s10958-008-9196-y

    Google Scholar 

  19. Ipatov, M.M., Koshelev, M.M., and Raigorodskii, A.M., Modularity of Some Distance Graphs, submitted to European J. Combin.

  20. Ipatov, M.M., Exact Modularity of Line Graphs of Complete Graphs, Moscow J. Comb. Number Theory, 2021, vol. 10, no. 1, pp. 61–75. https://doi.org/10.2140/moscow.2021.10.61

    Article  MathSciNet  Google Scholar 

  21. Koshelev, M.M., New Lower Bound on the Modularity of Johnson Graphs, Moscow J. Comb. Number Theory, 2021, vol. 10, no. 1, pp. 77–82. https://doi.org/10.2140/moscow.2021.10.77

    Article  MathSciNet  Google Scholar 

  22. Hoeffding, W., Probability Inequalities for Sums of Bounded Random Variables, J. Amer. Statist. Assoc., 1963, vol. 58, no. 301, pp. 13-30. https://doi.org/10.2307/2282952

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgment

The authors are grateful to Prof. A.M. Raigorodskii for posing the problem and for discussing the obtained results.

Funding

The research was supported by the President of the Russian Federation Council for State Support of Leading Scientific Schools, grant no. NSh-2540.2020.1, and the Theoretical Physics and Mathematics Advancement Foundation “BASIS.”

Author information

Authors and Affiliations

  1. Moscow Institute of Physics and Technology (National Research University), Moscow, Russia

    N. M. Derevyanko

  2. Lomonosov Moscow State University, Moscow, Russia

    M. M. Koshelev

Authors
  1. N. M. Derevyanko
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. M. M. Koshelev
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

Translated from Problemy Peredachi Informatsii, 2021, Vol. 57, No. 4, pp. 87–109 https://doi.org/10.31857/S0555292321040082.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Derevyanko, N., Koshelev, M. New Modularity Bounds for Graphs \(G(n,r,s)\) and \(G_p(n,r,s)\). Probl Inf Transm 57, 380–401 (2021). https://doi.org/10.1134/S0032946021040086

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S0032946021040086

Key words

Search

Navigation