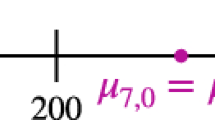Abstract
The nonlinear dynamics of the free surface of an ideal incompressible non-conducting fluid with a high dielectric constant subjected to a strong horizontal electric field is simulated using the method of conformal transformations. It is shown that in the initial stage of interaction of counter-propagating periodic waves of significant amplitude, there is a direct energy cascade leading to energy transfer to small scales. This results in the formation of regions with a steep wave front at the fluid surface, in which the dynamic pressure and the pressure exerted by the electric field undergo a discontinuity. It has been demonstrated that the formation of regions with high gradients of the electric field and fluid velocity is accompanied by breaking of surface waves; the boundary inclination angle tends to 90◦, and the surface curvature increases without bound.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
J. R. Melcher and W. J. Schwarz, “Interfacial Relaxation Overstability in a Tangential Electric Field,” Phys. Fluids 11, 2604–2616 (1968).
L. L. Barannyk, D. T. Papageorgiou, and P. G. Petropoulos, “Suppression of Rayleigh–Taylor Instability Using Electric Fields,” Math. Comput. Simulat. 82, 1008–1016 (2012).
M. F. El-Sayed, “Electro-Aerodynamic Instability of a Thin Dielectric Liquid Sheet Sprayed with an Air Stream,” Phys. Rev. E 60, 7588–7591 (1999).
N. M. Zubarev and O. V. Zubareva, “Nondispersive Propagation of Waves with Finite Amplitudes on the Surface of Dielectric Liquid in a Tangential Electric Field,” Pis’ma Zh. Tekh. Fiz. 32 (200), 40–44 (2006).
N. M. Zubarev and E. A. Kochurin, “Three-Dimensional Nonlinear Waves at the Interface between Dielectric Fluids in an External Horizontal Electric Field,” Prikl. Mekh. Tekh. Fiz. 54 (2), 52–58 (2013) [J. Appl. Mech. Tech. Phys. 54 (2), 212–217 (2013)].
N. M. Zubarev and E. A. Kochurin, “The Interaction of Strongly Nonlinear Waves on the Free Surface a Non-Conducting Fluid in a Horizontal Electric Field,” Pis’ma Zh. Eksp. Teor. Fiz. 99 (11), 729–734 (2014).
V. E. Zakharov, A. I. Dyachenko, and O. A. Vasilyev, “New Method for Nmerical Simulation of a Nonstationary Potential Flow of Incompressible Fluid with a Free Surface,” Europ. J. Mech., B: Fluids 21, 283–291 (2002).
P. G. Frik, Turbulence: Methods and Approaches: A Lecture Course (Perm State Technical University, Perm, 1999) [in Russian].
R. J. LeVeque, Finite Difference Methods for Ordinary and Partial Differential Equations (SIAM, Philadelphia, 2007).
L. D. Landau and E. M. Lifshits, Course of Theoretical Physics, Vol. 6: Fluid Mechanics (Nauka, Moscow, 1986; Pergamon Press, Oxford-Elmsford, New York, 1987).
D. W. Moore, “The Spontaneous Appearance of a Singularity in the Shape of an Evolving Vortex Sheet,” Proc. Roy. Soc. London, Ser. A 365, 105–119 (1979).
N. M. Zubarev and E. A. Kuznetsov, “Singularity Formation on a Fluid Interface during the Kelvin-Helmholtz Instability Development,” Zh. Eksp. Tekh. Fiz. 145 (6), 1–11 (2014).
D. Pelinovsky, E. Pelinovsky, E. Kartashova, et al., “Universal Power Law for the Energy Spectrum of Breaking Riemann Waves,” Pis’ma Zh. Eksp. Teor. Fiz. 98 (3), 265–269 (2013).
Author information
Authors and Affiliations
Corresponding author
Additional information
Original Russian Text © E.A. Kochurin.
Translated from Prikladnaya Mekhanika i Tekhnicheskaya Fizika, Vol. 59, No. 1, pp. 91–98, January–February, 2018.
Rights and permissions
About this article
Cite this article
Kochurin, E.A. Formation of Regions with High Energy and Pressure Gradients at the Free Surface of Liquid Dielectric in a Tangential Electric Field. J Appl Mech Tech Phy 59, 79–85 (2018). https://doi.org/10.1134/S0021894418010108
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1134/S0021894418010108