Abstract
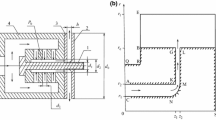
Results of experimental investigations and numerical simulations of supersonic gas flows in radial nozzles with different nozzle widths are presented. It is demonstrated that different types of the flow are formed in the nozzle with a fixed nozzle radius and different nozzle widths: supersonic flows with oblique shock waves inducing boundary layer separation are formed in wide nozzles, and flows with a normal pseudoshock separating the supersonic and subsonic flow domains are formed in narrow nozzles (micronozzles). The pseudoshock structure is studied, and the total pressure loss in the case of the gas flow in a micronozzle is determined.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
N. P. Gridnev, S. S. Katsnelson, and V. P. Fomichev, Nonhomogeneous MHD Flows with the T-Layer (Nauka, Novosibirsk, 1984) [in Russian].
P. S. Moller, “Radial Flow without Swirl between Parallel Disks Having Both Supersonic and Subsonic Region,” J. Basic Eng., Ser. D 88 (1), 153–154 (1966).
S. I. Kim and S. O. Park, “Oscillatory Behavior of Supersonic Impinging Jet Flow,” ShockWaves 4 (4), 259–272 (2005).
S. V. Klinkov, V. F. Kosarev, and V. N. Zaikovskii, “Application of Radial Supersonic Nozzles in Cold Spraying,” in Abstr. of the Int. Conf. on Methods of Aerophysical Research, Kazan, August 19–25, 2012 (Kazan. Fed. Univ., Kazan, 2012), Part 1, pp. 153–154.
S. P. Kiselev, V. P. Kiselev, and V. N. Zaikovskii, “Numerical Simulation of the Spaying Process of Al Particles on the Tube Surface by a Radial Nozzle,” in Abstract of the Int. Conf. on the Methods of Aerophysical Research, Novosibirsk, June 30 to July 6, 2014 (Avtograf, Novosibirsk, 2014), Part 1, pp. 109–110.
F. R. Menter, “Two-Equation Eddy-Viscosity Turbulence Models for Engineering Applications,” AIAA J. 32 (8), 1598–1605 (1994).
S. P. Kiselev, V. P. Kiselev, and V. N. Zaikovskii, “On the Mechanism of Self-Oscillations of a Supersonic Radial Jet Exhausting into an Ambient Space,” Prikl. Mekh. Tekh. Fiz. 57 (2), 53–63 (2016) [J. Appl. Mech. Tech. Phys. 57 (2), 237–246 (2016)].
I. I. Lipatov, V. Yu. Liapidevskii, and A. A. Chesnokov, “Model of an Unsteady Pseudoshock in a Barotropic Gas Flow,” Dokl. Akad. Nauk 466 (5), 545–549 (2016).
O. V. Guskov, V. I. Kopchenov, I. I. Lipatov, et al., Processes of Supersonic Flow Deceleration in Channels (Fizmatlit, Moscow, 2008) [in Russian].
V. I. Penzin, Supersonic Flow Deceleration in Channels (TsAGI, Moscow, 2012) [in Russian].
G. N. Abramovich, Applied Gas Dynamics (Nauka, Moscow, 1976) [in Russian].
Author information
Authors and Affiliations
Corresponding author
Additional information
Original Russian Text © S.P. Kiselev, V.P. Kiselev, V.N. Zaikovskii.
Rights and permissions
About this article
Cite this article
Kiselev, S.P., Kiselev, V.P. & Zaikovskii, V.N. Supersonic Gas Flows in Radial Nozzles. J Appl Mech Tech Phy 58, 1021–1032 (2017). https://doi.org/10.1134/S0021894417060086
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1134/S0021894417060086