Abstract
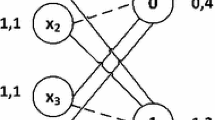
Lower and upper bounds are obtained for the size ζ(n, r, s, k) of a minimum system of common representatives for a system of families of k-element sets. By ζ(n, r, s, k) wemean themaximum (over all systems Σ = {M1, …, Mr} of sets Mi consisting of at least s subsets of {1, …, n} of cardinality not exceeding k) of the minimum size of a system of common representatives of Σ. The obtained results generalize previous estimates of ζ(n, r, s, 1).
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
V. E. Tarakanov, Combinatorial Problems and (0, 1)-Matrices (Nauka, Moscow, 1985) [in Russian].
A. M. Raigorodsky, Systems of Common Representatives in Combinatorics and Their Applications to Geometry (MTsNMO, Moscow, 2009) [in Russian].
P. Erdős and J. Spencer, Probabilistic Methods in Combinatorics (Academic Press, New York–London, 1974; Mir, Moscow, 1976).
J. Balogh, D. Cherkashin, and S. Kiselev, “Coloring general Kneser graphs and hypergraphs via high-discrepancy hypergraphs,” European J. Combin. 79, 228–236 (2019).
L. E. Shabanov, “Tura´ n-type results for distance graphs in an infinitesimal plane layer,” J.Math. Sci. (N. Y.) 236 (5), 554–578 (2019).
N. N. Kuzyurin, “Asymptotic investigation of the set covering problem,” Probl. Kibernetiki 37, 19–56 (1980).
A. M. Raigorodsky, “The Borsuk problem for integral polytopes,” Mat. Sb. 193 (10), 139–160 (2002) [Sb. Math. 193 (10), 1535–1556 (2002)].
A. M. Raigorodsky, “The problems of Borsuk and Gru¨ nbaum on lattice polytopes,” Izv. Ross. Akad. Nauk Ser. Mat. 69 (3), 81–108 (2005) [Izv.Math.69 (3), 513–537 (2005)].
A. M. Raigorodsky, “Three lectures on the Borsuk partition problem,” in Surveys in Contemporary Mathematics, LondonMath. Soc. Lecture Note Ser. (Cambridge Univ. Press, Cambridge, 2007), Vol. 347, pp. 202–248.
L. I. Bogolyubskii and A. M. Raigorodsky, “A Remark on Lower Bounds for the Chromatic Numbers of Spaces of Small Dimension with Metrics ℓ1 and ℓ2,” Mat. Zametki 105 (2), 187–213 (2019) [Math. Notes 105 (2), 180–203 (2019)].
A. M. Raigorodsky, “Combinatorial geometry and coding theory,” Fund. Inform. 145 (3), 359–369 (2016).
A. M. Raigorodsky, “The defects of admissible balls and octahedra in a lattice, and systems of generic representatives,” Mat. Sb. 189 (6), 117–141 (1998) [Sb.Math. 189 (6), 931–954 (1998)].
A. M. Raigorodsky, “A Probabilistic Approach to the Problem of the Defects of Admissible Sets in a Lattice,” Mat. Zametki 68 (6), 910–916 (2000) [Math. Notes 68 (6), 770–774 (2000)].
A. M. Raigorodsky, “On a problem in the geometry of numbers,” Tr. Inst. Mat. 15 (1), 111–117 (2007).
A. A. Bagan and A. M. Raigorodsky, “Defect of an Admissible Octahedron in a Centering of an Integer Lattice Generated by a Given Number of Vectors,” Mat. Zametki 99 (3), 457–459 (2016) [Math. Notes 99 (3), 457–459 (2016)].
A. A. Bagan, “Defect of an admissible octahedron in a centering obtained by adding rational vectors to an integer lattice,” Mosc. J. Comb. Number Theory 5 (1-2), 3–13 (2015).
M. A. Fadin, “Defect of an octahedron in a rational lattice,” DiscreteMath. (2019) (in press).
M. A. Fadin and A. M. Raigorodsky, “Maximum defect of an admissible octahedron in a rational lattice,” UspekhiMat. Nauk 74 (3 (447)), 191–192 (2019) [in Russian].
Funding
This work was supported by the Russian Foundation for Basic Research (grant 18-01-00355) and by the Presidential Program for the State Support of Leading Scientific Schools (grant NSh-6760.2018.1).
Author information
Authors and Affiliations
Corresponding authors
Additional information
Russian Text © The Author(s), 2019, published in Matematicheskie Zametki, 2019, Vol. 106, No. 3, pp. 387–394.
Rights and permissions
About this article
Cite this article
Kovalenko, K.D., Raigorodsky, A.M. Systems of Representatives. Math Notes 106, 372–377 (2019). https://doi.org/10.1134/S0001434619090062
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1134/S0001434619090062