Abstract
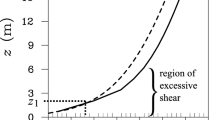
A condition is derived for consistency of the standard∈-equation with Monin–Obukhov (MO) similarity theory of thestably-stratified surface layer. The condition is derivedby extending the procedure used to derive the analogous condition forneutral theory to stable stratification. It is shown that consistencywith MO theory requires a function of flux Richardson number, Rif, to be absorbed into either of two closure parameters, c∈ 1 or c∈ 2.Inconsistency, on the other hand, results if constant values of these are maintained for all Rif, as is done in standardapplication of the equation, and the large overpredictions ofturbulence found in such application to the one-dimensionalstable atmospheric boundary layer (1D-SBL) are traced to thisinconsistency. Guided by this, we formulate a MO-consistent∈-equation by absorbing the aforementioned function intoc∈ 1, and combine this with a Level-2.5 second-orderclosure model for vertical eddy viscosity and diffusivities.Numerical predictions of the 1D-SBL by the modified model converge to a quasi-steady state, rectifying the predictive failure of the standard∈ -equation for the case.Quasi-steady predictions of non-dimensional variables agree stronglywith Nieuwstadt's theory. Qualitative accuracy of predictionsis inferred from comparisons to field data, large-eddy simulationresults and Rossby-number similarity relationships.
Similar content being viewed by others
References
André, J. C. and Mahrt, L.: 1982, 'The Nocturnal Surface Inversion and Influence of Clear-Air Radiative Cooling', J. Atmos. Sci. 39, 864–878.
Andrén, A.: 1990, 'Evaluation of a Turbulence Closure Scheme Suitable for Air Pollution Applications', J. Appl. Math. Phys. 29, 224–239.
Andrén, A.: 1991, 'A TKE-Dissipation Model for the Atmospheric Boundary Layer', Boundary-Layer Meteorol. 56, 207–221.
Apsley, D. D. and Castro, I. P.: 1997, 'A Limited-Length-Scale k-ε Model for the Neutral and Stably-Stratified Atmospheric Boundary Layer', Boundary-Layer Meteorol. 83, 75–98.
Arya, S. P. S.: 1975, 'Geostrophic Drag and Heat Transfer Relations for the Atmospheric Boundary Layer', Quart. J. Roy. Meteorol. Soc. 101, 147–161.
Baumert, H. and Peters, H.: 2000, 'Second-Moment Closures and Length Scales forWeakly Stratified Turbulent Shear Flows', J. Geophys. Es.-Oceans 105, 6453–6468.
Brost, R. A. and Wyngaard, J. C.: 1978, 'A Model Study of the Stably-Stratified Planetary Boundary Layer', J. Atmos. Sci. 35, 1427–1440.
Brown, A. R., Derbyshire, S. H., and Mason, P. J.: 'Large-Eddy Simulation of Stable Atmospheric Boundary Layers with a Revised Stochastic Subgrid Model', Quart. J. Roy. Meteorol. Soc. 120, 1485–1512.
Businger, J. A., Wyngaard, J. C., Izumi, Y., and Bradley, E. F.: 1971, 'Flux-Profile Relationships in the Atmospheric Surface Layer', J. Atmos. Sci. 28, 181–189.
Caughey, S. J. Wyngaard, J. C., and Kaimal, J. C.: 1979, 'Turbulence in the Evolving Stable Boundary Layer', J. Atmos. Sci. 36, 1041–1052.
Coleman, G., Ferziger, J., and Spalart, P.: 1992, 'Direct Simulation of the Stably Stratified Turbulent Ekman Layer', J. Fluid Mech. 244, 677.
Delage, Y.: 1997, 'Parameterising Sub-Grid Scale Vertical Transport in Atmospheric Models under Statically Stable Conditions', Boundary-Layer Meteorol. 82, 23–48.
Derbyshire, S. H.: 1990, 'Nieuwstadt's Stable Boundary Layer Revisited', Quart J. Roy. Meteorol. Soc. 116, 127–158.
Derbyshire, S. H.: 1995, 'Stable Boundary Layers: Observations, Models and Variability Part I: Modelling and Measurements', Boundary-Layer Meteorol. 74, 19–54.
Durbin, P. A. and Pettersson-Reif, B. A.: 2001, Statistical Theory and Modeling for Turbulent Flow, John Wiley and Sons, Chichester, U.K., 282 pp.
Duynkerke, P. G.: 1988, 'Application of the E-ε Turbulence Closure Model to the Neutral and Stable Atmospheric Boundary Layer', J. Atmos. Sci. 45, 865–880.
Freedman, F. R. and Jacobson, M. Z.: 2002, 'Transport-Dissipation Analytical Solutions to the E-ε Turbulence Model and their Role in Predictions of the Neutral ABL', Boundary-Layer Meteorol. 102, 117–138.
Garratt, J. R.: 1992, The Atmospheric Boundary Layer, University Press, Cambridge, 316 pp.
Grant, A. L. M.: 1997, 'An Observational Study of the Evening Transition Boundary Layer', Quart. J. Roy. Meteorol. Soc. 123, 657–677.
Högström, U.: 1988, 'Non-Dimensional Wind and Temperature Profiles in Atmospheric Surface-Layer: A Reevaluation', Boundary-Layer Meteorol. 42, 55–78.
Howell, J. F. and Sun, J.: 1999, 'Surface-Layer Fluxes in Stable Conditions', Boundary-Layer Meteorol. 90, 495–520.
Kosovic, B. and Curry, J. A.: 2000, 'A Large Eddy Simulation of a Quasi-Steady, Stably Stratified Atmospheric Boundary Layer', J. Atmos. Sci. 57, 1052–1068.
Lacser, A. and Arya, S. P. S.: 1986, 'A Comparative Assessment ofMixing-Length Parameterizations in the Stably Stratified Nocturnal Boundary Layer (NBL)', Boundary-Layer Meteorol. 36, 53–70.
Launder, B. E.: 1989, 'Second-Moment Closure... and Future?', Int. J. Heat Fluid Flow 10, 282–300.
Lenschow, D. H., Li, X. S., Zhu, C. J., and Stankov, B. B.: 1998, 'The Stably Stratified Boundary-Layer over the Great Plains: 1. Mean and Turbulence Structure', Boundary-Layer Meteorol. 42, 95–121.
Mahrt, L.: 1999, 'Stratified Atmospheric Boundary Layers', Boundary-Layer Meteorol. 90, 375–396.
Mellor, G. L. and Yamada, T.: 1982, 'Development of a Turbulence Closure Model for Geophysical Fluid Problems', Rev. Geophys. Space Phys. 20, 851–875.
Nieuwstadt, F. T.M.: 1984, 'The Turbulent Structure of Stable, Nocturnal Boundary Layer', J. Atmos. Sci. 41, 2202–2216.
Nieuwstadt, F. T. M.: 1985, 'A Model for Stationary, Stable Boundary Layer', in J. C. R. Hunt (ed.), Turbulence and Diffusion in Stable Environments, Clarendon Press, pp. 149–179.
Ohya, Y.: 2001, 'Wind-Tunnel Study of Atmospheric Stable Boundary Layers over a Rough Surface', Boundary-Layer Meteorol. 98, 57–82.
Sorbjan, Z.: 1989, Structure of the Atmospheric Boundary Layer, Prentice Hall, Englewood, 317 pp.
Speziale, C. G. and Bernard, P. S.: 1992, 'The Energy Decay of Self-Preserving Isotropic Turbulence Revisited', J. Fluid Mech. 241, 645–667.
Speziale, C. G. and Gatski, T. B.: 1996, 'Analysis and Modelling of Anisotropies in the Dissipation Rate of Turbulence', J. Fluid Mech. 344, 155–180.
Speziale, C. G. and MacGiolla-Mhuiris, N.: 1989, 'On the Prediction of Equilibrium States in Homogeneous Turbulence', J. Fluid Mech. 209, 591–615.
Tennekes, H. and Lumley, J. L.: 1972, A First Course on Turbulence, MIT Press, Cambridge, MA, 300 pp.
Wilcox, D. C.: 1998, Turbulence Modeling for CFD, DCW Industries Inc., La Canada, CA.
Wyngaard, J. C.: 1975, 'Modeling the Planetary Boundary Layer-Extension to the Stable Case', Boundary-Layer Meteorol. 71, 277–296.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Freedman, F.R., Jacobson, M.Z. Modification Of The Standard ∈-Equation For The Stable Abl Through Enforced Consistency With Monin–Obukhov Similarity Theory. Boundary-Layer Meteorology 106, 383–410 (2003). https://doi.org/10.1023/A:1021251523246
Issue Date:
DOI: https://doi.org/10.1023/A:1021251523246