THE PHASE SPACE STRUCTURE OF THE EXTENDED SITNIKOV-PROBLEM

Abstract

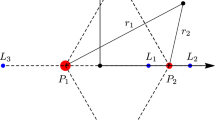
In this article we treat the 'Extended Sitnikov Problem' where three bodies of equal masses stay always in the Sitnikov configuration. One of the bodies is confined to a motion perpendicular to the instantaneous plane of motion of the two other bodies (called the primaries), which are always equally far away from the barycenter of the system (and from the third body). In contrary to the Sitnikov Problem with one mass less body the primaries are not moving on Keplerian orbits. After a qualitative analysis of possible motions in the 'Extended Sitnikov Problem' we explore the structure of phase space with the aid of properly chosen surfaces of section. It turns out that for very small energies H the motion is possible only in small region of phase space and only thin layers of chaos appear in this region of mostly regular motion. We have chosen the plane ($r,\dot r$) as surface of section, where r is the distance between the primaries; we plot the respective points when the three bodies are 'aligned'. The fixed point which corresponds to the 1 : 2 resonant orbit between the primaries' period and the period of motion of the third mass is in the middle of the region of motion. For low energies this fixed point is stable, then for an increased value of the energy splits into an unstable and two stable fixed points. The unstable fixed point splits again for larger energies into a stable and two unstable ones. For energies close toH = 0 the stable center splits one more time into an unstable and two stable ones. With increasing energy more and more of the phase space is filled with chaotic orbits with very long intermediate time intervals in between two crossings of the surface of section. We also checked the rotation numbers for some specific orbits.