Abstract
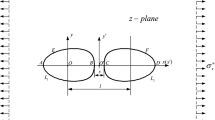
When concentrated forces are applied at any points of the outer region of an ellipse in an infinite plate, the complex potentials are determined using the conformal mapping method and Cauchy’s integral formula. And then, based on the superposition principle, the analytical solutions for stress around an elliptical hole in an infinite plate subjected to a uniform far-field stress and concentrated forces, are obtained. Tangential stress concentration will occur on the hole boundary when only far-field uniform loads are applied. When concentrated forces are applied in the reversed directions of the uniform loads, tangential stress concentration on the hole boundary can be released significantly. In order to minimize the tangential stress concentration, we need to determine the optimum positions and values of the concentrated forces. Three different optimization methods are applied to achieve this aim. The results show that the tangential stress can be released significantly when the optimized concentrated forces are applied.
Similar content being viewed by others
References
T.T. Rosen, K.N. Magne, E. Andreas, Design procedure for reducing the stress concentration around circular holes in laminated composites, Composites 26 (1995) 815–828.
L.M. Vovk, V.F. Kozhevnikov, I.E. Semenov-Ezhov, Stress concentration around holes in plates and lugs with symmetric reinforcements in uniaxial tension, Russ. Eng. Res 21 (11) (2001) 27–31.
S. Engels, W. Becker, Optimization of hole reinforcements by doublers, Struct. Multidisc Optim 20 (2000) 57–66.
G.S. Giare, R. Shabahang, The Reduction of stress concentration around the hole in an isotropic plate using composite materials, Eng. Fract. Mech 32 (5) (1989) 757–166.
G.N. Savin, Stress Concentration Around Holes, Pergamon Press, New York, 1961.
P.E. Erickson, W.F. Riley, Minimizing stress concentrations around circular hole in uniaxially loaded plates, Exp. Mech 18 (3) (1978) 97–100.
U.C. Jindal, Reduction of stress concentration around a hole in a uniaxially loaded plate, J. Strain Anal. Eng 18 (2) (1983) 135–141.
J. Huang, S. Venkataraman, R.J. Rapoff, R.T. Haftka, Optimization of axisymmetric elastic modulus distributions around a hole for increased strength, J. Struct. Multidisc Optim 25 (4) (2003) 225–236.
A. Muc, A. Ulatowska, Local fibre reinforcement of holes in composite multilayered plates, Compos. Struct 94 (2012) 1413–1419.
R. Sburlati, Stress concentration factor due to a functionally graded ring around a hole in an isotropic plate, Int. J. Solids Struct 50 (22–23) (2013) 3649–3658.
R. Sburlati, S.R. Atashipour, S.A. Atashipour, Reduction of the stress concentration factor in a homogeneous panel with hole by using a functionally graded layer, Composite Part B 61 (2014) 99–109.
Q.Q. Yang, C.F. Gao, Reduction of the stress concentration around an elliptic hole by using a functionally graded layer, Acta Mech 227 (2016) 2427–2437.
X.L. Gao, A general solution of an infinite elastic plate with an elliptic hole under biaxial loading, Int. J. Pres. Ves. Piping 67 (1996) 95–104.
N.I. Muskhelishvili, Some Basic Problems of the Mathematical Theory of Elasticity, Noordhoff, Groningen, 1963.
S.P. Timoshenko, J.N. Goodier, Theory of Elasticity, 3rd ed, McGraw-Hill, New York, 1970.
C.K. Chao, F.M. Chen, Revisit of an elliptic hole problem by using complex variable method, J. Chin. Inst. Eng 39 (1) (2016) 121–130.
Y.Z. Chen, A new analysis method of an infinite plate containing elliptical hole and applied by concentrated forces and a couple, Shanghai J. Mech 15 (4) (1994) 64–66.
A.Z. Lu, H.Y. Chen, Y. Qin, N. Zhang, Shape optimization of the support section of a tunnel at great depths, Comput. Geotech 61 (2014) 190–197.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Lu, A., Zhang, N. & Zeng, G. Minimizing stress concentrations around an elliptical hole by concentrated forces acting on the uniaxially loaded plate. Acta Mech. Solida Sin. 30, 318–326 (2017). https://doi.org/10.1016/j.camss.2017.07.002
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1016/j.camss.2017.07.002