Introduction

As demand for cheaper and more efficient space access increases, applied-field magnetoplasmadynamic thrusters (AF-MPDTs) operating in a low-discharge current regime promise to become a viable technology for future LEO and deep-space missions. If power requirements can be decreased to the level typically available on-board a modern small satellite (<1 kW), this technology has the potential to become more attractive to end-users. However, a decrease in power and discharge current reduces the thrust generated. Increasing the applied magnetic field strength can mitigate this effect [15].

Recent progress in high-temperature superconductor (HTS) and miniaturized cryocooler technology could enable the application of higher magnetic fields for in-space applications which were previously infeasible [3, 22]. For this reason, the resulting low-power and high B-field operational regime has not been widely investigated. Consequently, it is currently unknown if scaling laws typically used for analyzing AF-MPDTs can be extended to this unexplored parameter space. Understanding the performance of AF-MPDTs in this regime is crucial to determining their application and could be used by mission planners to inform their design decisions.

AF-MPDTs were initially developed to counter problems associated with the high current requirements of self-field MPDTs. At the higher power end of this thruster technology, research into the field at the University of Stuttgart in more recent years [4] has brought forward a 100 kW thruster [5]. At the very low power end of around 50 W, excellent performance has recently been achieved through the combination of a micro-cathode arc thruster with a second AF-MPD stage [36]. However, most previous AF-MPDT thrusters and modeling efforts have been focused on high-power scenarios with low applied-field strengths [9]. Therefore a review and modeling approach is required to better understand the underlying physical processes and expected performance at the lower power of this novel class of AF-MPDTs. The way a greater magnetic field strength may benefit thruster performance parameters is also investigated.

As a first step, experimental data and operational and physical parameters from thrusters with power levels below 50 kW, or with applied magnetic field strengths above 500 mT, were collected in a database and reviewed. In the second step, existing performance models were reviewed, and their accuracy was assessed when applied to the data. Finally, by analyzing the models for dependencies or correlations where its accuracy scales with some operational or structural parameter, a corrected empirical “low-power” model was formulated to better predict performance in this regime. This model’s results and implications for designing a novel AF-MPDT thruster are discussed.

Section 2 comprises a brief overview of thruster performance, followed by an overview of the different published thrust and voltage models. Section 3 reviews previous experimental studies and describes the data collected for each. In section 4, the accuracy of the models is compared, and an improved model is formulated, followed by a discussion of some of its implications in section 5.

AF-MPDT Performance Models

There are several parameters that can characterize the performance of an electric thruster. The most apparent is thrust T, which scales directly with the mass flow rate \(\dot{m}\) of the propellant and the effective exhaust velocity \(v_e\). The specific impulse \(I_{\text {sp}}\) is commonly used as measure of how efficiently a propulsion system creates thrust, and is defined as \(I_{\text {sp}}=(T/\dot{m}g_0)\), where \(g_0\) is 9.8 ms\({}^{-2}\).

For electric thrusters specifically, the thruster or electrical efficiency is defined as the ratio of the kinetic power of the jet, which is responsible for the thrust production, to the total electrical power required by the system:

$$\begin{aligned} \eta _T = \frac{\text {jet kinetic power}}{\text {electrical power}} = \frac{0.5 \dot{m}v_e^2}{JV} . \end{aligned}$$
(1)

This efficiency takes into consideration all the energy losses that occur. The thrust to power ratio (T/P\({}_e\)) of the thruster is another crucial parameter that can be written as

$$\begin{aligned} \frac{T}{P_e} = \frac{2\eta _T}{g_0 I_{\text {sp}}} . \end{aligned}$$
(2)

It is seen that in order to fully predict the electric thruster performance, knowledge of both the thrust T and the discharge voltage V is required. This is why as part of this work the focus is placed on both the modeling of thrust and the discharge voltage. Numerous models have been formulated in the past that attempt to predict the thrust and voltage of an AF-MPDT in dependence on its operational (e.g., current, mass flow) and physical (e.g., anode radius) parameters.

AF-MPDT Acceleration Mechanisms

In general, three different acceleration mechanisms that contribute to the total thrust can be distinguished in an AF-MPDT [18, 30].

Gas-dynamic/electrothermal acceleration (GD): The GD acceleration component is due to Joule heating and the thermodynamic expansion of the hot gases in a nozzle. It is expected to be only weakly dependent on the magnetic field strength and current, and scale with the mass flow rate.

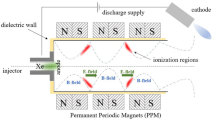
Self-field acceleration (SF): This acceleration mechanism occurs as a result of the interaction between the discharge current J between the anode and cathode, and the self-induced azimuthal magnetic field \(B_{\theta }\) (see Fig. 1a). The resulting force \(J \times B_{\theta }\) has a radial component \(J_z \times B_{\theta }\), also called the “pinching” or “pumping” component [18], as well as an axial “blowing” component \(J_r \times B_{\theta }\).

Applied-field acceleration (AF): This acceleration component occurs as a result of the externally applied magnetic field. Two different sub-mechanisms can be distinguished. The first is the “swirl acceleration” (see Fig. 1b). Owing to the applied axial B-field \(B_{\text {a}}\) interacting with the radial component of the current \(J_r\), a resulting azimuthal force \(J_r \times B_{a,z}\) is exerted on the plasma. This causes it to swirl and subsequently expand in the magnetic nozzle formed by the applied magnetic field, gradually transferring its swirl kinetic energy to axial kinetic energy. This swirling motion also induces an azimuthal Hall current \(J_H\), assuming the magnetic field strength is large enough and the mass flows small enough (meaning the Hall parameter is large). The result of the Lorentz force produced by the interaction of the Hall current with the applied magnetic field \(J_H \times B_{\text {a}}\) is the Hall acceleration (see Fig. 1c). Again, a radial “pinching” and axial “blowing” component can be distinguished.

Fig. 1
figure 1

Schematics of the different acceleration mechanisms in an AF-MPD thruster. Red represents the discharge current, blue the applied magnetic field, orange the Hall current, and green the different resulting force components. A cylindrical coordinate system is used to describe these physical models

Full size image

Thrust Models

Most thrust models assume that the total thrust \(T_{\text {AFMPDT}}\) of an AF-MPDT can be formulated as the sum of three components:

$$\begin{aligned} T_{\text {AFMPDT}} = T_{\text {GD}} + T_{\text {SF}} + T_{\text {AF}} , \end{aligned}$$
(3)

where \(T_{\text {GD}}\) is the thrust component resulting from the gas-dynamic acceleration, \(T_{\text {SF}}\) is the thrust resulting from the self-field acceleration and \(T_{\text {AF}}\) is the part of the thrust associated with the applied-field acceleration. Many publications assume the same model for the self-field and gas-dynamic acceleration, however, significant differences exist in models of the applied-field component.

The gas-dynamic thrust component \(T_{\text {GD}}\) is most commonly modeled as

$$\begin{aligned} T_{\text {GD}} = k_{\text {GD}}\dot{m}c_{\text {s}} , \end{aligned}$$
(4)

where \(k_{\text {GD}}\) is a non-dimensional coefficient, \(c_{\text {s}}\) is the ion sound speed and \(\dot{m}\) is the propellant mass flow [12, 16, 18, 32]. Different authors assume different values for the ion speed sound and the coefficient. In this work, \(c_{\text {s}}\) is set to be 1900 m/s and \(k_{\text {GD}}\) is set to unity.

The self-field thrust component is most commonly assumed to be calculated as modeled by Maecker [23] and later modified by Jahn [14]:

$$\begin{aligned} T_{\text {SF}} = \frac{\mu_0}{4\pi } \left[ \ln \left( \frac{r_{\text {a}}}{r_{\text {c}}}\right) + 3/4\right] J^2 . \end{aligned}$$
(5)

Here, \(r_{\text {a}}\) and \(r_{\text {c}}\) are the anode and cathode radius respectively, \(\mu_0\) is the magnetic permeability of free space and J is the discharge current.

A number of different models and approaches exist for the applied field thrust component. Apart from early models derived through energy conservation by Sasoh et al. [31], most assume a thrust that scales with the current J, the axial magnetic field strength \(B_{\text {a}}\), and the anode radius \(r_{\text {a}}\). To allow for better comparison, only such models are considered within this work.

Tikhonov et al.  [32] assumes a thrust as the result of the Hall current crossing the magnetic field and derives the following semi-analytical model:

$$\begin{aligned} T_{\text {Tikhonov}} = 2k_{\text {Tikhonov}}JB_{\text {a}}r_{\text {a}} , \end{aligned}$$
(6)

where \(k_{\text {Tikhonov}}\) is an empirically determined coefficient that varies depending on which propellant is being used. For Argon, \(k_{\text {Tikhonov}}\) = 0.058 [12] and for Xenon, \(k_{\text {Tikhonov}}\) = 0.1.

Herdrich et al. [12] modified the Tikhonov scaling model by introducing the anode radius as a dependent variable within the coefficient \(k_{\text {Tikhonov}}\). Fitting the modified equation to the experimental data for the Sverdrup Myers 100 kW AF-MPDT [28] and the University of Stuttgart’s X16 thruster [2] yields the following equation for the AF-thrust:

$$\begin{aligned} T_{\text {Herdrich}} = 2.924JB_{\text {a}}r_{\text {a}}^{5/3} . \end{aligned}$$
(7)

Fradkin et al. [10] performed tests on a 25 kW hollow cathode lithium MPD thruster, referred to as an MPD Arcjet. Apart from incorporating a unique propellant feed system and comparing performance differences between a hollow cathode and a conventional rod cathode, a thrust model is derived numerically. Assuming a strictly cylindrical anode shape and axial magnetic field, the torque rotating the plasma and its expansion within the thruster is modeled. This gives the following,

$$\begin{aligned} T_{\text {Fradkin}} = \frac{1}{\sqrt{2}}B_{\text {a}}Jr_{\text {a}}\left[ 1- \frac{3}{2}\left( \frac{r_{\text {c}}}{r_{\text {a}}}\right)^2\right] . \end{aligned}$$
(8)

Building on the result of Fradkin et al., Albertoni et al. [1] fit the Fradkin model to the experimental data of the Alta 100 kW AF-MPD thruster and the so-called “Hybrid Plasma Thruster”. In the analysis, a scaling factor of 0.25 is found to yield the best fit:

$$\begin{aligned} T_{\text {Albertoni}} = 0.25T_{\text {Fradkin}} . \end{aligned}$$
(9)

Myers et al. [26] initially assumed a proportionality of \(T \sim JB_{\text {a}}r_{\text {a}}^2\) in their experimental results, however, a dependence on cathode length \(l_{\text {c}}\) and radius was observed. The final model is

$$\begin{aligned} T_{\text {Myers}} = JB_{\text {a}}\frac{r_{\text {a}}^2}{500r_{\text {c}}l_{\text {c}}} . \end{aligned}$$
(10)

Most recently, Coogan et al. [9] conducted a critical review of AF-MPD thrust models and compiled a comprehensive database of available experimental thrust data. A new model was derived empirically by assuming a general functional expression and deriving a formula through non-dimensional analysis using the Buckingham Pi theorem. The final thrust model is

$$\begin{aligned} T_{\text {Coogan}} = 1.14JB_{\text {a}}r_{\text {a}}\phi^{-0.13}{({}^{r_{\text {a}}}/{}_{r_{\text {c}}})}^{-0.3}(10+{}^{l_{\text {c}}}/{}_{l_{\text {a}}})^{-0.67} , \end{aligned}$$
(11)

where \(\phi\) is a measure of how well the magnetic field lines and the anode contour align:

$$\begin{aligned} \phi = \frac{r_{\text {ae}}^2r_{\text {B}}^3}{r_{\text {a0}}^2(r_{\text {B}}^2 + l_{\text {a}}^2)^{{}^{3}/{}_{2}}} , \end{aligned}$$
(12)

where \(r_{\text {a0}}\) is the anode radius at its narrowest or at the backplate, \(r_{\text {ae}}\) is the anode radius at the exit plane, \(r_{\text {B}}\) is the magnetic solenoid radius and \(l_{\text {a/c}}\) is the anode/cathode length.

The total thrust model by Mikellides et al. [24] is not based on the three different acceleration components but instead derived from numerical simulation results that were performed for a 100 kW class argon AF-MPD thruster:

$$\begin{aligned} T_{\text {Mik}} = \frac{25}{A^{0.25}}\sqrt{\frac{r_{\text {c}}}{r_{\text {a}}\overline{\phi }}}\frac{R(R+1)\sqrt{R-1}}{\sqrt{R^{3.8}-1}}\sqrt{\dot{m}JB_{\text {a}}} , \end{aligned}$$
(13)

where R is the ratio \(r_{\text {a}}/r_{\text {c}}\) and \(\overline{\phi }\) is the ionization factor. In this work, that factor is derived from the simulation results for the specific regime and propellant. For this review, it is assumed to be \(\overline{\phi } = 1\). It was shown later on by the author that the factor had little impact on the results.

Voltage Models

The total discharge voltage of an AF-MPDT can be understood as the sum of the voltages assigned to the different power components and losses that occur in an AF-MPDT. Existing models formulate a voltage model based on these different power components by dividing the power component by the current.

Lev et al. [21] formulated two different models for the total discharge voltage, one as published in an article and another in a Ph.D. dissertation [20]. The model from the article is

$$\begin{aligned} V_{\text {LevArt}} = V_{\text {emf}} + V_{\text {i}} + \phi _{\text {a}} + \phi _{\textrm{c}} + V_{\text {e}} , \end{aligned}$$
(14)

where \(V_{\text {emf}}\) is the back electromotive voltage (or kinetic voltage), \(V_{\text {i}}\) the ionization voltage, \(\phi_{\text {a/c}}\) the anode and cathode material work function and \(V_{\text {e}}\) the electrode sheath voltage fall. \(V_{\text {emf}}\) can be written as

$$\begin{aligned} V_{\text {emf}} = \frac{T^2}{2\dot{m}J} . \end{aligned}$$
(15)

This represents the power and voltage component that goes towards actual thrust production. \(V_{\text {i}}\) is the voltage needed to ionize the propellant:

$$\begin{aligned} V_{\text {i}} = \frac{\dot{m}}{m_iJ}\epsilon _i , \end{aligned}$$
(16)

where \(\epsilon_i\) is the first ionization energy of the propellant. The atomic mass of the propellant is \(m_i\) and is related to the molar mass M via Avogadro’s constant (\(m_i = M/N_A\)). \(\phi_{\text {a}}\) and \(\phi_{\text {a}}\) are the work functions of the anode and cathode material respectively and are assumed to be the voltage needed to extract electrons from the surface.

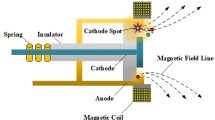
Finally, \(V_{\text {e}}\) is the electrode sheath voltage fall. Early experimental work performed by Myers et al. [27] and Gallimore et al. [11] demonstrated a dependency of the anode sheath voltage on the three main operational parameters, propellant mass flow \(\dot{m}\) , current J and the applied magnetic field strength \(B_{\text {a}}\). Expanding on this work through theoretical studies and experimentation, Lev et al. [19] formulated the following equation for the anode voltage fall as a function of those parameters:

$$\begin{aligned} V_{\text {e}} = \frac{(C_1J(C_2 + B_{\text {a}}) + C_3B_{\text {a}})}{\dot{m}^n} . \end{aligned}$$
(17)

The parameters C and n are derived empirically and have values of \(C_1 = 6.18 \cdot 10^{-4}\), \(C_2 = 0.1\), \(C_3 = 0.9272\) and \(n=0.5\). These and all further empirically derived constants in the models were assumed to be constant after it was demonstrated that leaving them variable had a negligible effect on the empirical modeling results discussed in section 4.

The model from the dissertation [20] expands on this model:

$$\begin{aligned} V_{\text {LevDiss}} = V_{\text {emf}} + V_{\text {heat}} + V_{\text {i}} + \phi_{\text {a}} + \phi_{\textrm{c}} + V_{\text {a}} . \end{aligned}$$
(18)

A voltage component from the power needed to heat up the plasma \(V_{\text {heat}}\) is added and calculated using

$$\begin{aligned} V_{\text {heat}} = \frac{\dot{m}}{m_iJ}(k_{\text {B}}T_{\text {e}} + k_{\text {B}}T_i) . \end{aligned}$$
(19)

The electron temperature \(T_{\text {e}}\) in the acceleration region is assumed to be 0.4 eV, the ion temperature \(T_i\) is set to be constant at 1 eV. \(V_{\text {a}}\) is the anode sheath voltage fall for which the following expression is derived:

$$\begin{aligned} V_{\text {a}} = \frac{k_{\text {B}}T_{\text {e}}}{e}\ln \left( \frac{J/A_{\text {a}} + A_R T_{\text {a}}^2 e^{\left( -\frac{e\phi _{\text {a}}}{k_{\text {B}}T_{\text {a}}}\right) }}{0.25en_{\text {e}}\left( \frac{8k_{\text {B}} T_{\text {e}}}{\pi m_{\text {e}}}\right) ^{1/2}} \right) , \end{aligned}$$
(20)

where \(A_{\text {a}}\) is the inner anode surface area. Assuming an anode shape of a truncated cone, the surface area can be calculated via \(A_{\text {a}} = (r_{\text {ae}}+r_{\text {a0}})\sqrt{(r_{\text {ae}}-r_{\text {a0}})^2 + l_{\text {a}}^2}\pi\). A\({}_R\) is the Richardson-Dushman coefficient. The anode temperature \(T_{\text {a}}\) can be calculated using an empirically derived Eq. [20]:

$$\begin{aligned} T_{\text {a}} = 1080 + 2375B_{\text {a}} - 5\cdot 10^6 \dot{m} + (1.366 - 1.33\cdot 10^4\dot{m})J . \end{aligned}$$
(21)

The electron density \(n_{\text {e}}\) is calculated using

$$\begin{aligned} n_{\text {e}} = C_3\dot{m}e^{-(C_1JB_{\text {a}}+C_2J^2)} , \end{aligned}$$
(22)

where \(C_1=0.19\), \(C_2 = 2\cdot 10^{-6}\) and \(C_3 = 2\cdot 10^{21}\) are empirically derived constants.

Albertoni et al. [1] also formulated an equation for the voltage as the sum of different components:

$$\begin{aligned} V_{\text {Alb}} = \sum\limits_i V_{\text {i}} = \frac{T^2}{J2\dot{m}} + \frac{1}{J}\frac{(q\epsilon _i + 3/2k_{\text {B}} T_{\text {e}})\dot{m}}{m_i} + \phi _{\text {a}} + \frac{5}{2}\frac{k_{\text {B}} T_{\text {e,A}}}{q} + V_{\text {A}} + V_C . \end{aligned}$$
(23)

The main components of \(V_{\text {Alb}}\) are the same as those in the model of [20]. The first term is the electromotive voltage and the second is \(V_{\text {heat}}\) and \(V_{\text {i}}\) combined. The cathode sheath voltage \(V_C\) is assumed to be on the same order of magnitude as \(\epsilon_i\). A slightly different expression is derived for the anode sheath voltage:

$$\begin{aligned} V_{\text {A}} = \frac{k_{\text {B}}T_{e,A}}{e}\left[ \ln \left( \frac{\sqrt{m_i/m_{\text {e}}}}{0.61\sqrt{2\pi }} \right) - \ln \left( 1 + \frac{J/A_{\text {a}}}{0.61en_{\text {s}}\sqrt{k_{\text {B}}T_{\text {e,A}}/m_i}}\right) \right] , \end{aligned}$$
(24)

where \(T_{\text {e,A}}\) is the electron temperature at the anode sheath layer and is set to 2 eV and \(m_{\text {e}}\) is the electron mass. The electron number density at the sheath edge \(n_{\text {s}}\) is calculated using

$$\begin{aligned} n_{\text {s}} = k_3 \dot{m}\exp [-(k_1 B_{\text {a}} J + k_2 J^2)r_{\text {a}}^2] , \end{aligned}$$
(25)

where \(k_1 = 0.2\), \(k_2 = 2 \cdot 10^{-5}\) and \(k_3 = 5 \cdot 10^{18}\).

Data Collection

Experimental data collected was used to evaluate the existing models. Since this work focuses on modeling low-power AF-MPDTs at high magnetic field strengths, the review is limited to existing experimental thrusters with data at power levels below 50 kW or magnetic field strengths above 500 mT. Table 1 lists those thrusters that fall within that range of interest in chronological order.

Table 1 Identified AF-MPDTs and their range in power, discharge current and applied magnetic field strength as well as the cathode type
Full size table

H2-2-D was an AF-MPD thruster (or Hall current accelerator as it was called at the time) developed by NASA in 1966 as part of a larger series of experimental thrusters with different designs, propellants, and operational parameters [6]. As the name suggests, it utilized hydrogen as a propellant. It was part of the low-power 10-kW engine series, had a cylindrical anode, and yielded the best performance results of the thrusters tested. A maximum efficiency of 26 % and a maximum thrust per power ratio of 13 mN/kW were reported.

Electro-Optical Systems Inc. developed the LAJ-AF series of AF-MPD thrusters under a contract with the Air Force Aero-Propulsion Laboratory [6, 7, 25]. Different lithium thruster designs with changes in dimensions, power levels, and propellant feeding mechanisms were tested, and their performance was characterized. The LAJ-AF 2, 3, and 6D fall within the operating range of interest.

The LAJ-AF-2 was the second thruster configuration tested with lithium injected through the cathode. It has a cylindrical anode and a solid Tungsten rod cathode. The LAJ-AF-3 thruster was the same model with a slightly different cathode designed to allow for a stationary arc attachment point. A maximum efficiency of 71.8 % and a maximum thrust to power ratio of 40 mN/kW were calculated from the experimental data. The LAJ-AF-6D model was endurance tested and had a water-cooled cathode. A maximum efficiency of 54 % and a maximum T/P of 52.5 mN/kW were measured.

Research was conducted at Waseda University in Japan on a 1kW-class applied-field MPD arc jet thruster [29]. It featured a convergent-divergent anode and a pointed rod cathode. Both the anode diameter at its narrowest point as well as the cathode diameter and location were varied to evaluate the discharge stability and impact on thrust. The applied magnetic field strength was in the range of 100 to 200 mT, and propellant mass flow was between 0.2 and 5 mg/s. A maximum efficiency of 8.5 % and a T/P ratio of 26 mN/kW were measured.

Ongoing research at Nagoya University developed an AF-MPD thruster design in 2017 with a hollow thermionic emission cathode with a lanthanum hexaboride (LaB\({}_6\)) emitter that can discharge at current levels down to 10 A [13, 15]. This thruster was the first experimental demonstration where a thermionic emission hollow cathode was used as an on-axis electron source in an AF-MPDT. In the data presented, different cathode orifice radii and cathode lengths were tested, and the propellant was injected entirely through the cathode. Apart from demonstrating the feasibility of using this type of cathode, different applied magnetic field strengths, current regimes, and mass flow rates were tested, and their impact on the thruster performance was determined. An efficiency of 25.5 % and a T/P ratio of 17 mN/kW were reported.

In 2018, the results of an investigation on low-power AF-MPDT performance at Beihang University in China were published [8, 17, 34]. Two thruster designs were tested, one with a converging-diverging anode (CD) and one with a cylindrical anode (CYL). The cathode was a simple mono-channel tube. A focus was placed on investigating the performance at high mass flow rates and low discharge currents, with a fixed Argon mass flow rate of 21 mg/s and discharge currents from 100 to 180 A. For both variants, the propellant is injected through both the cathode and the anode back-plate at different ratios in order to analyze the impact the feeding regime might have on the performance. The applied magnetic field strength varied from 0 to 133 mT.

Performance improved when a larger amount of propellant was injected through the cathode. In general, the cylindrical thruster design outperformed the converging-diverging design. A greater applied magnetic field meant a greater thrust efficiency at any of the tested current or propellant mass flow regimes. However, the effect was more pronounced for the cylindrical configuration. The tests revealed the importance of the anode design, especially with respect to the magnetic field and how it brings on the transition from a thermo-electric acceleration dominated arc-jet mode to an electro-magnetic acceleration dominated MPDT mode. A maximum T/P ratio of 64 mN/kW and an efficiency of 21 % were measured.

Most recently, in 2020, Russian company SuperOX in collaboration with the National Research Nuclear University in Moscow presented the first results of an AF-MPD thruster using high-temperature superconductor tape to produce an applied magnetic field strength of up to 1 Tesla [33]. The thruster has a simple cylindrical anode design with a multi-cavity cathode consisting of multiple solid rods. Two different cathode diameters of 10 and 17 mm were tested. Xenon, Argon, and Krypton are used as propellants at different mass flow rates ranging from 15 to 50 mg/s and are injected only via the cathode.

A maximum thrust per power ratio of 48 mN/kW and an efficiency of 54 % were reported. Unfortunately, only limited experimental data and no information on any physical parameters of the thruster are given in the report. It was thus not included in the database and the present work.

For each of the aforementioned thrusters, the following experimental data and parameters are collected in a database (where available):

  • Operational parameters: discharge current J, applied mag- field \(B_{\text {a}}\), anode/cathode propellant mass flow \(\dot{m}_{a/c}\)

  • Structural parameters: cathode radius \(r_{\text {c}}\), anode radius at exit plane \(r_{\text {ae}}\), narrowest anode radius \(r_{\textrm{a0}}\), mean anode radius \(r_{\text {a}}\) (assumed to be the mean of \(r_{\text {ae}}\) and \(r_{\textrm{a0}}\) ), magnetic coil radius \(r_{\text {B}}\), cathode length \(l_{\text {c}}\), anode length \(l_{\text {a}}\).

  • Experimental values: thrust T, voltage V

  • Other: propellant (Type, molar mass M, ionization energy \(\epsilon_i\)), cathode material work function \(\phi_{\text {c}}\), anode material work function \(\phi_{\text {a}}\)

Some of the data were tabulated, whereas other data was extracted from plots.

Improved Model Development

Existing Model Evaluation

To evaluate the accuracy of the different models, the total thrust and voltage according to each model are calculated for the operational and physical parameters of every experimental datapoint within the database. The difference between the calculated values and the experimentally measured values as a fraction of the experimentally measured value is then determined:

$$\begin{aligned} \Delta X \, [\%] = \frac{X_{\text {calc.}}-X_{\text {exp.}}}{X_{\text {exp.}}}\cdot 100 . \end{aligned}$$
(26)

Here, X is either thrust T or voltage V. An ideal model would yield a relative difference of \(\Delta T/V = 0\,\%\) across the entire database, a value greater than zero means the thrust or voltage is over-predicted, whereas a value below zeros means the thrust or voltage is under-predicted.

The mean of the relative difference as well as the relative standard deviation across the entire database are calculated for each model and compared. Since the values are scattered, just considering the mean alone would not accurately reflect the accuracy of the models, so the standard deviation was also calculated. Figure 2 gives an example of the resulting relative difference \(\Delta T\) as well as its mean \(\langle \Delta T\rangle\) and standard deviation \(\sigma_{\Delta T}\) for the Tikhonov thrust model when compared to experimental results.

Fig. 2
figure 2

The relative difference between the Tikhonov thrust model results and experimental thrust values \({\Delta }T_{\text {Tikhonov}}\) across the database, showing the resulting mean and standard deviation

Full size image

Table 2 shows the results of this comparative study for the different thrust models that were reviewed. The Coogan [9] and the Albertoni [1] model have the lowest mean relative error with -11 %, meaning that both models tend to slightly under-predict the total thrust. The Albertoni model, however, has a significantly smaller standard deviation of 21 % compared to 29 % for the Coogan model. This means it more accurately predicts the thrust within a given range. The model by Herdrich [12] tends to over-predict the thrust with a mean relative error of 16 %. The models by Fradkin [10], Myers [28], and Mikellides [24] are the least accurate with mean relative differences of over 100 % and even greater standard deviations.

Table 2 Performance of Thrust Models
Full size table

Table 3 lists the results of the study for the voltage models that were reviewed. It should be noted that the experimentally measured thrust was used as the required input for the voltage models. Here, the Albertoni [1] model has the lowest mean relative error of -41 %. Both the Albertoni and Lev’s dissertation [20] models have a standard deviation of 24 %. In general, the voltage models perform significantly worse than the thrust models.

Table 3 Voltage Model Performance
Full size table

Model Improvement Method

The model evaluation results are analyzed for correlations or linear dependencies where the relative difference \(\Delta X\) scales with a test parameter. Since many other parameters are changed in parallel whenever plotting over a single parameter, large scattering is expected.

As a first step and to quantify any such hidden relationship, Pearson’s correlation coefficient is calculated. Pearson’s correlation coefficient \(r_{xy}\) is a commonly used statistical test that measures the linear correlation between two sets of data x and y. The coefficient is calculated using

$$\begin{aligned} r_{xy} = \frac{\sum ^n_{i=1}(x_i - \overline{x})(y_i-\overline{y})}{\sqrt{\sum^n_{i=1}(x_i - \overline{x})^2}\sqrt{\sum ^n_{i=1}(y_i - \overline{y})^2}} . \end{aligned}$$
(27)

The coefficient always has a value between -1 and 1. A coefficient of either exactly -1 or 1 means that a linear equation perfectly describes the relationship between the two variables whereas a value of zero means there is no linear correlation whatsoever. To determine whether or not a discovered linear relationship is reliable, the sample size must also be taken into consideration. For a sample size of around n = 300 and a desired level of significance of 0.01, r\({}_{xy}\) should be greater than 0.15 for the correlation to be considered significant [35].

After the coefficients are calculated, all relationships with a coefficient over a specific value are further analyzed. This is done by approximating the dependencies with a simple linear regression fit. From the fit results, both the coefficient of determination \(R^2\) as well as the residual standard mean error (RMSE) can be calculated and interpreted. A larger value of \(R^2\) and a smaller RMSE are generally desirable as they are an indicator of the goodness of the fit. In this case, they are also a further indicator of how significant the correlation is.

Once the most significant correlation is identified, the function \(f_{\Delta X} (y)\) (where y is an operational or structural parameter) resulting from the fit can be used to improve upon the model. This is done by assuming that all the points on the identified function should have a \(\Delta T\) or \(\Delta V\) = 0 (since an ideal model would have a \(\Delta T\) or \(\Delta V\) of zero for all parameter values) and shifting their values according to their parameter value y. The function \(f_ {\Delta X} (y)\) can then be used as a parameter-dependent scaling factor for the model it was derived from to yield an improved fit:

$$\begin{aligned} X_{LP}(y) = \frac{1}{1+f_{\Delta X}(y)/100} \cdot X_{\text {theoretical}}(y) . \end{aligned}$$
(28)

The revised model will be referred to as the low-power (“LP”) model. Again, X is either T or V.

Improved “Low-Power” LP Performance Models

Based on the results of the existing model evaluation, only the Coogan, Tikhonov, Albertoni, and Herdrich thrust models as well as the Lev Dissertation and Albertoni voltage models are considered to be improved upon. The test parameters \(r_{\text {B}}\) and \(l_{\text {a}}\) are excluded as they only extend over a small data range with limited data points, since they were not varied as much as other parameters in the available experimental results. The correlation coefficients calculated for X = \(\Delta T\) or \(\Delta V\) for the different models and y = \(J,B_{\text {a}},\dot{m},r_{\text {a}},r_{\text {c}},l_{\text {c}}\) are listed in Table 4.

Table 4 Correlation Coefficients r\({}_{xy}\)
Full size table

For the thrust models, the highest correlation coefficients are observed for the relationship between \(\Delta T_{\text {Coogan}}\) and \(B_{\text {a}}\), as well as between \(\Delta T_{\text {Herdrich}}\) and r\({}_a\) and l\({}_c\). For the voltage models, the strongest correlation is observed between Lev’s voltage model and \(B_{\text {a}}\) and \(r_{\text {a}}\), as well as between the Albertoni model and \(r_{\text {a}}\). A simple linear regression fit was performed and evaluated for these combinations. The \(R^2\) and RMSE values listed in Table 5 are obtained.

Table 5 Goodness of Fit Parameters
Full size table

The two correlations that are identified as being the most significant due to having the highest \(R^2\) and simultaneously the lowest RMSE are between the applied magnetic field strength and the thrust model by Coogan [9], as well as between the anode radius and the voltage model from Lev’s dissertation. Figure 3 shows these correlations as well as the linear fits found that best describe them.

Fig. 3
figure 3

The most significant observed correlations between the relative difference \({\Delta }\)X of a model and a parameter. (a) shows the relative difference between the Coogan thrust model results and experimental thrust values as a function of the applied magnetic field strength. (b) shows the relative difference between the Lev voltage model results and experimental voltage values as a function of the mean anode radius. Linear regression fits are included

Full size image

It can be seen that the Coogan thrust model tends to under-predict the thrust at lower applied magnetic fields and over-predicts for higher B fields. The same trend is also observed for each individual thruster. The Lev voltage model increasingly under-predicts the voltage for greater anode radii.

These correlations yield the final revised performance models. The complete LP thrust model based on the Coogan model is:

$$\begin{aligned} T_{\text {LP}} = \frac{1}{1+\gamma /100} \left( \frac{\mu _0}{4\pi } \left[ \ln \left( \frac{r_{\text {a}}}{r_{\text {c}}}\right) + 3/4\right] J^2 + k_{\text {GD}}\dot{m}c_s \right. \nonumber \\ \left. + 1.14JB_{\text {a}}r_{\text {a}}\phi ^{-0.13}{}^{r_{\text {a}}}/{}_{r_{\text {c}}}^{-0.3}(10 + {}^{l_{\text {c}}}/{}_{l_{\text {a}}})^{-0.67} \right) , \end{aligned}$$
(29)
$$\begin{aligned} \textrm{where} \qquad \gamma = p_1 B_{\text {a}} + p_2 \qquad \textrm{with} \qquad p_1 = 102.4 \; \left[ \frac{1}{T}\right] \quad \& \quad p_2 = -41.07 , \end{aligned}$$
(30)
$$\begin{aligned} \textrm{and}\qquad \phi = \frac{r_{\text {ae}}^2 r_{\text {B}}^3}{r_{\text {ae}}^2(r_{\text {B}}^2 +l_{\text {a}}^2)^{1.5}} . \end{aligned}$$
(31)

The corrected LP voltage model based on Lev’s dissertation model (Eq. 18) is:

$$\begin{aligned} V_{\text {LP}} = \frac{1}{\left( 1 + \beta /100 \right) } V_{\text {LevDiss}} , \end{aligned}$$
(32)
$$\begin{aligned} \textrm{where} \qquad \beta = k_1 r_{\text {a}} + k_2 \qquad \textrm{with} \qquad k_1 = -2110 \; \left[ \frac{1}{m}\right] \quad \& \quad k_2 = - 7.5 . \end{aligned}$$
(33)

Together, \(T_{\text {LP}}\) and \(V_{\text {LP}}\) form the improved LP performance model. The resulting accuracy of the LP-model, when applied to the database, is shown in Table 6. The accuracy of the non-corrected “non-LP” model found to be the most accurate in the comparative study (Albertoni; thrust Eq. 9, voltage Eq. 23.) is shown by comparison.

Table 6 Accuracy of Revised LP Model
Full size table

The uncertainty in the models results can be estimated to be the mean of the model accuracy plus one standard deviation. A systematic error of 10 % can furthermore be assumed for the measured experimental data and added through Pythagorean addition. This gives an LP-model uncertainty of 27 % for thrust prediction and 29 % for voltage prediction. The uncertainties for performance parameters that are calculated with T and V (namely T/P and \(\eta\)) can then be calculated with the equation for Gaussian error propagation and thus also depend on the absolute values of T and V.

An improvement in accuracy can be seen for both the thrust and voltage models when compared to the most accurate models from the review. For both, the mean relative error is at zero or close to zero. The most substantial improvement is visible for the voltage model, where the mean relative error of the uncorrected model is 41 %. The model improvement becomes particularly apparent for performance parameters such as the thrust to power ratio or thruster efficiency, that rely on both accurate thrust and voltage prediction. As an example, Fig. 4 shows the relative difference between the experimentally determined and predicted thrust over power ratio \(\Delta\)TP across the database over the total power for the LP and non-LP model. A significant improvement in accuracy is demonstrated across the entire database and power range, particularly at lower power levels.

Fig. 4
figure 4

The relative difference between the experimentally determined and predicted thrust to power ratio \(\Delta\)TP as a function of the power for the LP and non-LP model

Full size image

Results and Discussion

The main result of this work is the aforementioned derived LP performance model. This improved low-power model reveals some interesting performance behavior and parameter impacts at the low-power end, some of which are shown here for an example AF-MPDT.

Fig. 5
figure 5

Calculated T/P ratio and efficiency depending on different parameters for varying applied magnetic field strengths using the revised LP model for an AF-MPDT. Unless used as a variable, J = 30 A, \({\dot{m}}\) = 2 mg/s, \({r_{\text {a}}}\) = 30 mm, \({r_{\text {c}}}\) = 2 mm, \({l_{\text {a}}}\) = 130 mm, \({l_{\text {c}}}\) = 50 mm, \({r_{\text {B}}}\) = 80 mm, Tungsten anode, LaB6 cathode, Argon propellant. The magnetic field strength is according to the legend in (e)

Full size image

Figure 5a shows both the T/P ratio and the thruster efficiency depending on the discharge current J for different applied magnetic field strengths. A general increase of the maximum values reached for both T/P and the efficiency is visible for an increase in \(B_{\text {a}}\). For greater magnetic field strengths, the T/P ratio reaches a maximum with an increase in the current before decreasing. The maximum current reached is also found to be dependent on the mass flow rate, shifting to higher currents for higher mass flow rates. For lower field strengths, T/P decreases with the discharge current.

In Fig. 5b, T/P and \(\eta\) are plotted over the mass flow for different \(B_{\text {a}}\). T/P generally increases with the mass flow, and for greater magnetic field strengths, greater mass flows are needed to reach higher T/P. In this example, a weaker magnetic field of 0.1 T yields a greater T/P at a mass flow of 2 mg/s than a stronger magnetic field. The thruster efficiency is generally larger for greater magnetic field strengths but decreases with an increase in mass flow.

In Fig. 5c, the same performance parameters are shown for varying anode radii. Both vary significantly and reach maximum values at certain anode radii. The location and height of these maxima vary with the magnetic field strength. For stronger magnetic fields, higher values for efficiency and T/P are reached.

Finally, in Fig. 5d, T/P and \(\eta\) are shown for different power levels between 0.5 and 4 kW for varying current. A general increase in efficiency is observed with power. The predicted T/P ratio generally decreases with power. For greater field strengths, a maximum is reached before decreasing.

Conclusion

In this work, an improved model for predicting the performance of an AF-MPDT in a low-power, high magnetic field strength regime is derived and presented. As part of its development, a comprehensive extract of existing thrusters operating in this regime as well as existing thrust and voltage models is given. A parametric analysis based on the improved model performed shows that careful consideration is required when designing an AF-MPD thruster, as it can be optimized depending on the boundaries set for the physical and operational parameters and desired performance. If this is done, an AF-MPDT in this regime could benefit from greater applied magnetic field strengths and reach T/P ratios and efficiencies comparable to those of other EP technologies.

The derived LP model developed can act as a valuable tool when commencing the design process of an AF-MPDT. If more experimental data becomes available, the model could further be improved upon with the outlined method. However, a more in-depth numerical simulation approach should be considered to better understand the physical relationships in this novel regime and formulate more accurate models.