Skip to main content
SpringerLink
Log in

Nonlinear high-fidelity modeling of spacecraft relative motion via orbit element differences

  • Original Paper
  • Published:
Aerospace Systems Aims and scope Submit manuscript

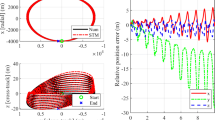
Abstract

The ultimate need to design and develop high-fidelity dynamical models for future space missions is necessitated by the continuous and enormous interest in spacecraft formation flying, rendezvous and spacecraft proximity operations. In this paper, to obtain high-fidelity dynamics, higher-order relative motion model is developed via nonlinear mapping of orbit element differences and Hill coordinates. First, second-order variation of parameter technique of calculus of variations is applied to the direction cosine matrix (DCM), which maps vector components in inertial frame to vector components in Deputy Hill frame, and deputy spacecraft inertial position and velocity vectors in Deputy Hill frame. Second, after series of transformations and elimination of higher-order terms greater than quadratic terms, new, nonlinearly mapped radial, along-track and cross-track relative motion position and velocity equations are obtained. Using the new equation of motion, nonlinear state space model is developed. The new equations, validated via numerical simulations, are amenable for the analysis of spacecraft relative motion, formation flying, rendezvous and proximity operations in both circular and elliptical orbits.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

Availability of data and material (data transparency)

All data and materials as well as software application or custom code support our published claims and comply with field standards.

References

  1. Clohessy WH, Wiltshire RS (1960) Terminal guidance system for satellite rendezvous. J Aerosp Sci 27:653–658

    Article  MATH  Google Scholar 

  2. Hill G (1878) Researches in lunar theory. Am J Math 21:5–26

    Article  MathSciNet  MATH  Google Scholar 

  3. Wertz J (2011) Space mission engineering: the new SMAD. Microcosm Press, Cleveland

    Google Scholar 

  4. Tschauner J, Hempel P (1965) Rendezvous zu einem in elliptisher bahn umlaufenden ziel. Astron. Acta 2:104–109

    MATH  Google Scholar 

  5. Walsh M. T, Peck M. A(2017)A general approach for calculating farfield orbital rendezvous maneuvers. In: AIAA guidance, navigation, and control conference 1730

  6. Scharf D P, Hadaegh F Y, Ploen S R(2003)A survey of spacecraft formation flying guidance and control (part i): guidance. In: Proceedings of the American control conference, pp 1733–1739

  7. Gim D-W, Terry Alfriend K (2003) State transition matrix of relative motion for the perturbed noncircular reference orbit. J Guid Control Dyn 26:956–971

    Article  Google Scholar 

  8. Vallado DA (2013) Fundamentals of astrodynamics and applications. Microcosm Press, Cleveland

    MATH  Google Scholar 

  9. Batin RH (1999) An introduction to the mathematics and methods of astrodynamics, revised edition. AIAA, Reston

    Book  Google Scholar 

  10. Ogundele AD (2021) Harmonic balance analysis and simulations of spacecraft rendezvous and formation flying dynamics. Aerosp Syst (AS). https://doi.org/10.1007/s42401-021-00083-0

    Article  Google Scholar 

  11. De Vries JP (1963) Elliptic elements in terms of small increments of position and velocity components. Am Inst Aeronaut Astronaut J 1(11):2626–2629

    Article  Google Scholar 

  12. Yamanaka K, Ankersen F (2002) New state transition matrix for relative motion on an arbitrary elliptical orbit. J Guid Control Dyn 25(1):60–66

    Article  Google Scholar 

  13. Schaub H, Junkins JL (2014) Analytical mechanics of space systems. AIAA, New York

    MATH  Google Scholar 

  14. Alfriend K. T, Schaub H, Gim D Gravitational perturbations, nonlinearity and circular orbit assumption effects on formation flying control strategy. In: AAS guidance and control conference Breckenridge, CO Feb. 2–6, 2000 AAS Publications Office, P.O. Box 28130, San Diego, CA 92198

  15. London HS (1963) Second approximation to the solution of the rendezvous equations. Am Inst Aeronaut Astronaut J 7:1691–1693

    Article  MATH  Google Scholar 

  16. Ogundele AD, Agboola OA, Sinha SC (2021) Mathematical modeling and simulation of nonlinear spacecraft rendezvous and formation flying problems via averaging method. Commun Nonlinear Sci Numer Simul 95:105668. https://doi.org/10.1016/j.cnsns.2020.105668

    Article  MathSciNet  MATH  Google Scholar 

  17. Lawden DF (1963) Optimal trajectories for space navigation, 2nd edn. Butterworth, London

    MATH  Google Scholar 

  18. Carter TE, Humi M (1987) Fuel-optimal rendezvous near a point in general Keplerian orbit. J Guid Control Dyn 10(6):567–573

    Article  MATH  Google Scholar 

  19. Carter TE (1998) State transition matrices for terminal rendezvous studies: brief survey and new examples. J Guid Control Dyn 21(1):148–155

    Article  MATH  Google Scholar 

  20. Ogundele A. D, Sinclair A. J, Sinha S. C(2018) Approximate closed form solutions of spacecraft relative motion via Abel and Riccati equations. Adv Astronaut Sci 162:2685–2704 (2018). ISSN: 00653438, Univelt Inc. Paper AAS 17–791 presented at the 2017 AAS/AIAA astrodynamics specialist conference in Columbia River Gorge, Stevenson, August 20–24

  21. Gurfil P, Kasdin NJ (2004) Nonlinear modeling of spacecraft relative motion in the configuration space. J Guid Control Dyn 27:154–157

    Article  Google Scholar 

  22. Palmer P (2006) Optimal relocation of satellites flying in near-circular-orbit formations. J Guid Control Dyn 29(3):519–526

    Article  Google Scholar 

  23. Schaub H, Alfriend KT (2002) Hybrid cartesian and orbit element feedback law for formation flying spacecraft. J Guid Control Dyn 25(2):383–393

    Article  Google Scholar 

  24. Inalhan G, Tillerson M, How J (2002) Relative dynamics and control of spacecraft formations in eccentric orbits. J Guid Control Dyn 25:48–59

    Article  MATH  Google Scholar 

  25. Vadali SR (2009) Model for linearized satellite relative motion about a j2-perturbed mean circular orbit. J Guid Control Dyn 32:1687–1691

    Article  Google Scholar 

  26. Ogundele AD (2021) Approximate analytic solution of nonlinear Riccati spacecraft formation flying dynamics in terms of orbit element differences. Aerosp Sci Technol 113:106686. https://doi.org/10.1016/j.ast.2021.106686

    Article  Google Scholar 

  27. Ogundele AD, Agboola OA (2021) Nonlinear mapping of orbit element differences for spacecraft formation flying. In: Paper AAS 21–341 presented at 31st space flight mechanics meeting conference, February 1–4, in Charlotte, North Carolina, USA

  28. Ogundele AD, Sinclair AJ, Sinha SC (2021) Closed form parametric solutions of nonlinear Abel-type and Riccati-type spacecraft relative motion. Acta Astronaut 178:733–742. https://doi.org/10.1016/j.actaastro.2020.10.009

    Article  Google Scholar 

  29. Ogundele AD, Agboola OA, Sinha SC (2021) Application of Lyapunov Floquet transformation to the nonlinear spacecraft relative motion with periodic-coefficients. Acta Astronaut 187:24–35. https://doi.org/10.1016/j.actaastro.2021.06.024

    Article  Google Scholar 

  30. Liberzon D (2012) Calculus of variations and optimal control theory: a concise introduction. Princeton University Press, Princeton

    Book  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Engineering and Space Systems Department, National Space Research and Development Agency, Abuja, Nigeria

    Ayansola D. Ogundele & Olufemi A. Agboola

  2. Department of Electronic and Electrical Engineering, Ladoke Akintola University of Technology (LAUTECH), Ogbomoso, Nigeria

    Olasunkanmi F. Oseni

Authors
  1. Ayansola D. Ogundele
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Olufemi A. Agboola
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Olasunkanmi F. Oseni
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

ADO: conceptualization, methodology, writing—original draft, writing—review and editing, literature search, software, formal analysis and validation. OAA: conceptualization, methodology, writing—review and editing, software and formal analysis. OFO: methodology, review and editing, software, formal analysis and validation.

Corresponding author

Correspondence to Ayansola D. Ogundele.

Ethics declarations

Conflict of interest

The authors have no financial or personal relationship with other people or organizations that could inappropriately influence or bias the content of the paper. There is no conflict of interest with other people or organizations that could inappropriately influence or bias the content of the paper.

Compliance with ethical standards

The research meets all applicable standards with regards to the ethics of experimentation and research integrity. The following is being certified/declared true.As an expert scientist, the paper has been submitted with full responsibility following due ethical procedure and there is no duplicate publication, fraud, plagiarism, or concerns about animal or human experimentation.

Additional information

This is the revised version of the paper AAS 21-341 presented at 31st AAS/AIAA Space Flight Mechanics Meeting. The Sheraton Charlotte Hotel in Charlotte, North Carolina. February 1–4, 2021.

Appendix A: Normalized nonlinear terms of the nonlinear mapped relative motion orbit element differences

Appendix A: Normalized nonlinear terms of the nonlinear mapped relative motion orbit element differences

$$\begin{aligned} {{\tilde{F}}_1}= & {} - \left( {\frac{{4{q_1}}}{p}} \right) \delta a\delta {q_1} - \left( {\frac{{4{q_2}}}{p}} \right) \delta a\delta {q_2}\nonumber \\&+ \frac{{2R}}{{{p^4}}}\left\{ {{p^3}\sin \theta - \frac{{{V_r}}}{{{V_t}}}\left( {{R^2}\left( {2a{q_1} + R\cos \theta } \right) + {p^3}\cos \theta } \right) } \right\} \nonumber \\&\times \delta \theta \delta {q_1}\nonumber \\&- \frac{{2R}}{{{p^4}}}\left\{ {{p^3}\cos \theta + \frac{{{V_r}}}{{{V_t}}}\left( {{R^2}\left( {2a{q_2} + R\sin \theta } \right) + {p^3}\sin \theta } \right) } \right\} \nonumber \\&\times \delta \theta \delta {q_2}\nonumber \\&- \left( {2\cos i} \right) \delta \theta \delta \varOmega + \left( {\sin i\sin 2\theta } \right) \delta i\delta \varOmega \nonumber \\&+ \frac{{2{R^4}}}{{{p^5}}}\left\{ {\left( {2a{q_1} + R\cos \theta } \right) \sin \theta + \left( {2a{q_2} + R\sin \theta } \right) \cos \theta } \right\} \nonumber \\&\times \delta {q_1}\delta {q_2}\nonumber \\&+ \frac{1}{p}\left\{ {p\left( {2{{\left( {\frac{{{V_r}}}{{{V_t}}}} \right) }^2} - 1} \right) + R\left( {{q_1}\cos \theta + {q_2}\sin \theta } \right) } \right\} \delta {\theta ^2}\nonumber \\&- \frac{1}{2}\left( {1 - \cos 2\theta } \right) \delta {i^2} \nonumber \\&+ \frac{2}{{{p^5}}}\left\{ { - a{p^4} + {R^4}\left( {2a{q_1} + R\cos \theta } \right) \cos \theta } \right\} \delta q_1^2\nonumber \\&+ \frac{2}{{{p^5}}}\left\{ { - a{p^4} + {R^4}\left( {2a{q_2} + R\sin \theta } \right) \sin \theta } \right\} \delta q_2^2 \nonumber \\&- \left( {1 - {{\sin }^2}i{{\sin }^2}\theta } \right) \delta {\varOmega ^2}\nonumber \\ {{{{\tilde{F}}}}_2}= & {} \left( {\frac{1}{a}} \right) \delta a\delta \theta + \left( {\frac{{\cos i}}{a}} \right) \delta a\delta \varOmega - \frac{1}{p}\left( {2a{q_1} + R\cos \theta } \right) \delta \theta \delta {q_1}\nonumber \\&- \frac{1}{p}\left( {2a{q_2} + R\sin \theta } \right) \delta \theta \delta {q_2} + \left( {\frac{{{V_r}\cos i}}{{{V_t}}}} \right) \delta \theta \delta \varOmega \nonumber \\&- \left( {2\sin i{{\sin }^2}\theta } \right) \delta i\delta \varOmega \nonumber \\&- \frac{{\cos i}}{p}\left( {2a{q_1} + R\cos \theta } \right) \delta \varOmega \delta {q_1}\nonumber \\&- \frac{{\cos i}}{p}\left( {2a{q_2} + R\sin \theta } \right) \delta \varOmega \delta {q_2} + \left( {\frac{{{V_r}}}{{{V_t}}}} \right) \delta {\theta ^2}\nonumber \\&- \frac{1}{2}\left( {\sin 2\theta } \right) \delta {i^2} + \frac{1}{2}\sin 2\theta \left( {1 - {{\cos }^2}i} \right) \delta {\varOmega ^2}\nonumber \\ {{{{\tilde{F}}}}_3}= & {} \left( {\frac{{\sin \theta }}{a}} \right) \delta a\delta i \nonumber \\&- \frac{1}{a}\left( {\sin i\cos \theta } \right) \delta a\delta \varOmega + \left\{ {\left( {\frac{{{V_r}}}{{{V_t}}}} \right) \sin \theta + 2\cos \theta } \right\} \delta \theta \delta i\nonumber \\&- \sin i\left\{ {\left( {\frac{{{V_r}}}{{{V_t}}}} \right) \cos \theta - 2\sin \theta } \right\} \delta \theta \delta \varOmega \nonumber \\&- \frac{{\sin \theta }}{p}\left( {2a{q_1} + R\cos \theta } \right) \delta i\delta {q_1}\nonumber \\&- \frac{{\sin \theta }}{p}\left( {2a{q_2} + R\sin \theta } \right) \delta i\delta {q_2} \nonumber \\&+ \frac{1}{p}\sin i\cos \theta \left( {2a{q_1} + R\cos \theta } \right) \delta \varOmega \delta {q_1}\nonumber \\&+ \frac{1}{p}\sin i\cos \theta \left( {2a{q_2} + R\sin \theta } \right) \delta \varOmega \delta {q_2} \nonumber \\&+ \frac{1}{2}\left( {\sin 2i\sin \theta } \right) \delta {\varOmega ^2} \nonumber \\ \end{aligned}$$
(40)

and

$$\begin{aligned} {{{{\tilde{F}}}}_4}= & {} \frac{1}{{2ap}}\left( {p + 2R} \right) \delta a\delta \theta + \frac{1}{{ap{V_t}}}\left( {3{V_r}a{q_1} - h\sin \theta } \right) \delta a\delta {q_1}\nonumber \\&+ \frac{1}{{ap{V_t}}}\left( {3{V_r}a{q_2} + h\cos \theta } \right) \delta a\delta {q_2} + \left( {\frac{{3\cos i}}{{2a}}} \right) \delta a\delta \varOmega \nonumber \\&- \frac{1}{{{p^4}V_t^2}}\left\{ {{p^2}V_t^2a{q_1}\left( {p + 2R} \right) } \right. \nonumber \\&\left. { + 2R{V_r}\left( {{p^3}\left( {{V_t}\sin \theta - {V_r}\cos \theta } \right) - {R^2}{V_r}\left( {2a{q_1} + R\cos \theta } \right) } \right) } \right\} \nonumber \\&\times \delta \theta \delta {q_1}\nonumber \\&- \frac{1}{{{p^4}V_t^2}}\left\{ {{p^2}V_t^2a{q_2}\left( {p + 2R} \right) } \right. \nonumber \\&\left. { - 2R{V_r}\left( {{p^3}\left( {{V_t}\cos \theta + {V_r}\sin \theta } \right) + {R^2}{V_r}\left( {2a{q_2} + R\sin \theta } \right) } \right) } \right\} \nonumber \\&\times \delta \theta \delta {q_2}\nonumber \\&+ \frac{{{V_r}}}{{{V_t}}}\left( {2\cos i} \right) \delta \theta \delta \varOmega + \frac{1}{{{V_t}}}\left( {2{V_t}\sin i\cos 2\theta } \right) \delta i\delta \varOmega \nonumber \\&+ \frac{1}{{{V_t}{p^5}}}\left\{ {2a{p^3}\left( {3{V_r}a{q_1}{q_2} - h\left( {{q_1}\cos \theta - {q_2}\sin \theta } \right) } \right) } \right. \nonumber \\&\left. { - 2{V_r}{R^4}\left( {\left( {2a{q_1} + R\cos \theta } \right) \sin \theta + \left( {2a{q_2} + R\sin \theta } \right) \cos \theta } \right) } \right\} \nonumber \\&\quad \delta {q_1}\delta {q_2}\nonumber \\&- \frac{1}{p}\cos i\left( {3a{q_1} + 2R\cos \theta } \right) \delta \varOmega \delta {q_1} \nonumber \\&- \frac{1}{p}\cos i\left( {3a{q_2} + 2R\sin \theta } \right) \nonumber \\&\times \delta \varOmega \delta {q_2}\nonumber \\&+ \left( {\frac{{3{V_r}}}{{4{V_t}{a^2}}}} \right) \delta {a^2} \nonumber \\&- \frac{{{V_r}}}{{p{V_t}}}\left\{ {2p{{\left( {\frac{{{V_r}}}{{{V_t}}}} \right) }^2} + R\left( {{q_1}\cos \theta + {q_2}\sin \theta } \right) - p} \right\} \delta {\theta ^2}\nonumber \\&+ \frac{1}{{2{V_t}}}\left\{ { - 2\left( {{V_r}{{\sin }^2}\theta + {V_t}\sin 2\theta } \right) + {V_r}\left( {1 - \cos 2\theta } \right) } \right\} \delta {i^2}\nonumber \\&+ \frac{1}{{{V_t}{p^5}}}\left\{ {a{p^3}\left( {{V_r}\left( {p + 3aq_1^2} \right) + 2h{q_1}\sin \theta } \right) } \right. \nonumber \\&\left. { - 2{V_r}\left( { - a{p^4} + {R^4}\left( {2a{q_1} + R\cos \theta } \right) \cos \theta } \right) } \right\} \delta q_{1}^{2}\nonumber \\&+ \frac{1}{{{V_t}{p^5}}}\left\{ {a{p^3}\left( {{V_r}\left( {p + 3aq_2^2} \right) - 2h{q_2}\cos \theta } \right) } \right. \nonumber \end{aligned}$$
$$\begin{aligned}&\left. { - 2{V_r}\left( { - a{p^4} + {R^4}\left( {2a{q_2} + R\sin \theta } \right) \sin \theta } \right) } \right\} \delta q_2^2 \nonumber \\&+ \left( {{{\sin }^2}i\sin 2\theta } \right) \delta {\varOmega ^2}\nonumber \\ {{{{\tilde{F}}}}_5}= & {} - \left( {\frac{{{V_r}}}{{2a{V_t}}}} \right) \delta a\delta \theta + \frac{1}{{ap}}\left( {3a{q_1} - R\cos \theta } \right) \delta a\delta {q_1} \nonumber \\&+ \frac{1}{{ap}}\left( {3a{q_2} - R\sin \theta } \right) \delta a\delta {q_2}\nonumber \\&- \left( {\frac{{3{V_r}\cos i}}{{2a{V_t}}}} \right) \delta a\delta \varOmega \nonumber \\&+ \frac{1}{{{V_t}{p^4}}}\left\{ {{p^3}\left( {{V_r}\left( {2R\cos \theta - a{q_1}} \right) - 3h\sin \theta } \right) } \right. \nonumber \\&\left. { + {V_r}\left( {2a{q_1} + R\cos \theta } \right) \left( {2{R^3} + {p^3}} \right) } \right\} \delta \theta \delta {q_1}\nonumber \\&+ \frac{1}{{{V_t}{p^4}}}\left\{ {{p^3}\left( {{V_r}\left( {2R\sin \theta - a{q_2}} \right) + 3h\cos \theta } \right) } \right. \nonumber \\&\left. { + {V_r}\left( {2a{q_2} + R\sin \theta } \right) \left( {2{R^3} + {p^3}} \right) } \right\} \delta \theta \delta {q_2}\nonumber \\&- \frac{{\cos i}}{p}\left\{ {p{{\left( {\frac{{{V_r}}}{{{V_t}}}} \right) }^2} + R - p} \right\} \delta \theta \delta \varOmega \nonumber \\&- \left( {2\sin i\sin 2\theta } \right) \delta i\delta \varOmega + \frac{1}{{{p^5}}}\left\{ {6a{p^4}{q_1}{q_2} - 2{R^5}\sin 2\theta } \right. \nonumber \\&\left. { + 2Ra\left( {{p^3} - 2{R^3}} \right) \left( {{q_2}\cos \theta + {q_1}\sin \theta } \right) } \right\} \delta {q_1}\delta {q_2}\nonumber \\&+ \frac{{\cos i}}{{p{V_t}}}\left\{ {3{V_r}a{q_1} + R\left( {{V_t}\sin \theta + {V_r}\cos \theta } \right) } \right\} \delta \varOmega \delta {q_1}\nonumber \\&+ \frac{{\cos i}}{{p{V_t}}}\left\{ {3{V_r}a{q_2} + R\left( {{V_r}\sin \theta - {V_t}\cos \theta } \right) } \right\} \delta \varOmega \delta {q_2}\nonumber \\&+ \left( {\frac{3}{{4{a^2}}}} \right) \delta {a^2} - \frac{1}{p}\left\{ {3p{{\left( {\frac{{{V_r}}}{{{V_t}}}} \right) }^2} + R\left( {{q_1}\cos \theta + {q_2}\sin \theta } \right) } \right\} \nonumber \\&\times \delta {\theta ^2}\nonumber \\&- \left( {\cos 2\theta } \right) \delta {i^2}\nonumber \\&+ \frac{1}{{{p^5}}}\left\{ 3a{p^4}\left( {1 + q_1^2} \right) + 2R\cos \theta \left( a{q_1}\left( {{p^3} - 2{R^3}} \right) \right. \right. \nonumber \\&\quad \left. \left. - {R^4}\cos \theta \right) \right\} \delta q_1^2\nonumber \\&+ \frac{1}{{{p^5}}}\left\{ 3a{p^4}\left( {1 + q_2^2} \right) + 2R\sin \theta \left( a{q_2}\left( {{p^3} - 2{R^3}} \right) \right. \right. \nonumber \\&\left. \left. - {R^4}\sin \theta \right) \right\} \delta q_2^2\nonumber \\&+ \left( {{{\sin }^2}i\cos 2\theta } \right) \delta {\varOmega ^2}\nonumber \\ {{{{\tilde{F}}}}_6}= & {} - \frac{1}{{2a{V_t}}}\left( {{V_t}\cos \theta + 3{V_r}\sin \theta } \right) \delta a\delta i \nonumber \\&+ \frac{{\sin i}}{{2a{V_t}}}\left( {3{V_r}\cos \theta - {V_t}\sin \theta } \right) \delta a\delta \varOmega \nonumber \\&- \frac{1}{{2pV_t^2}}\left\{ {2p\sin \theta \left( {2V_t^2 + V_r^2} \right) + 2p{V_r}{V_t}\cos \theta } \right. \nonumber \\&\left. { - h{V_t}\left( {{q_1}\sin 2\theta + 2{q_2}{{\sin }^2}\theta } \right) } \right\} \delta \theta \delta i \nonumber \\&+ \frac{{\sin i}}{{2pV_t^2}}\left\{ {2p\cos \theta \left( {2V_t^2 + V_r^2} \right) } \right. \nonumber \\&\left. { - 2p{V_r}{V_t}\sin \theta - h{V_t}\left( {2{q_1}{{\cos }^2}\theta + {q_2}\sin 2\theta } \right) } \right\} \delta \theta \delta \varOmega \nonumber \\&+ \frac{1}{{2p{V_t}}}\left\{ {{V_r}\left( {R\sin 2\theta + 6a{q_1}\sin \theta } \right) + 2{V_t}\left( {R + a{q_1}\cos \theta } \right) } \right\} \delta i\delta {q_1}\nonumber \\&+ \frac{1}{{p{V_t}}}\left\{ {R{V_r}{{\sin }^2}\theta + a{q_2}\left( {3{V_r}\sin \theta + {V_t}\cos \theta } \right) } \right\} \delta i\delta {q_2}\nonumber \\&- \frac{{\sin i}}{{p{V_t}}}\left\{ {R{V_r}{{\cos }^2}\theta + a{q_1}\left( {3{V_r}\cos \theta - {V_t}\sin \theta } \right) } \right\} \delta \varOmega \delta {q_1}\nonumber \\&- \frac{{\sin i}}{{2p{V_t}}}\left\{ {{V_r}\left( {R\sin 2\theta + 6a{q_2}\cos \theta } \right) - 2{V_t}\left( {R + a{q_2}\sin \theta } \right) } \right\} \delta \varOmega \delta {q_2}\nonumber \\&+ \frac{1}{2}\left( {\sin 2i\cos \theta } \right) \delta {\varOmega ^2} \end{aligned}$$
(41)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ogundele, A.D., Agboola, O.A. & Oseni, O.F. Nonlinear high-fidelity modeling of spacecraft relative motion via orbit element differences. AS 5, 591–605 (2022). https://doi.org/10.1007/s42401-022-00155-9

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s42401-022-00155-9

Keywords

Search

Navigation