Skip to main content
SpringerLink
Log in

The Generalized Carrier–Greenspan Transform for the Shallow Water System with Arbitrary Initial and Boundary Conditions

  • Original Article
  • Published:
Water Waves Aims and scope Submit manuscript

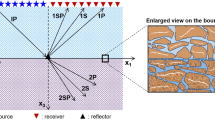
Abstract

We put forward a solution to the initial boundary value (IBV) problem for the nonlinear shallow water system in inclined channels of arbitrary cross section by means of the generalized Carrier–Greenspan hodograph transform (Rybkin et al. in J Fluid Mech, 748:416–432, 2014). Since the Carrier–Greenspan transform, while linearizing the shallow water system, seriously entangles the IBV in the hodograph plane, all previous solutions required some restrictive assumptions on the IBV conditions, e.g., zero initial velocity, smallness of boundary conditions. For arbitrary non-breaking initial conditions in the physical space, we present an explicit formula for equivalent IBV conditions in the hodograph plane, which can readily be treated by conventional methods. Our procedure, which we call the method of data projection, is based on the Taylor formula and allows us to reduce the transformed IBV data given on curves in the hodograph plane to the equivalent data on lines. Our method works equally well for any inclined bathymetry (not only plane beaches) and, moreover, is fully analytical for U-shaped bays. Numerical simulations show that our method is very robust and can be used to give express forecasting of tsunami wave inundation in narrow bays and fjords.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

Notes

  1. We outline the main results here in Introduction. The derivations are given in the main text.

  2. \(\left\| \cdot \right\| \) stands for the Euclidean norm.

References

  1. Alekseenko, S., Dontsova, M., Pelinovsky, D.: Global solutions to the shallow water system with a method of an additional argument. Appl. Anal. 69(9), 1444–1465 (2017)

    Article  MathSciNet  Google Scholar 

  2. Anderson, D., Harris, M., Hartle, H., Nicolsky, D., Pelinovsky, E., Raz, A., Rybkin, A.: Run-up of long waves in piecewise sloping u-shaped bays. J. Pure Appl. Geophys. 174, 3185–3207 (2017)

    Article  Google Scholar 

  3. Antuono, M., Brocchini, M.: The boundary value problem for the nonlinear shallow water equations. Stud. Appl. Math. 119, 73–93 (2007)

    Article  MathSciNet  Google Scholar 

  4. Antuono, M., Brocchini, M.: Solving the nonlinear shallow-water equations in physical space. J. Fluid Mech. 643, 207–232 (2010)

    Article  MathSciNet  Google Scholar 

  5. Carrier, G., Greenspan, H.: Water waves of finite amplitude on a sloping beach. J. Fluid Mech. 01, 97–109 (1958)

    Article  MathSciNet  Google Scholar 

  6. Carrier, G., Wu, T., Yeh, H.: Tsunami run-up and draw-down on a plane beach. J. Fluid Mech. 475, 79–99 (2003)

    Article  MathSciNet  Google Scholar 

  7. Chugunov, V., Fomin, S., Noland, W., Sagdiev, B.: Tsunami runup on a sloping beach. Comput. Math. Methods 2, e1081 (2020)

    Article  Google Scholar 

  8. Chugunov, V., Fomin, S., Shankar, R.: Influence of underwater barriers on the distribution of tsunami waves. J. Geophys. Res. Oceans 119, 7568–7591 (2014)

    Article  Google Scholar 

  9. Craig, W.: Surface water waves and tsunamis. J. Dyn. Differ. Equ. 18(3), 525–549 (2006)

    Article  MathSciNet  Google Scholar 

  10. Craig, W., Groves, M.: Hamiltonian long-wave approximations to the water-wave problem. Wave Motion 19, 367–389 (1994)

    Article  MathSciNet  Google Scholar 

  11. Craig, W., Guyenne, P., Kalisch, H.: A new model for large amplitude long internal waves. C. R. Mec. 332, 525–530 (2004)

    Article  Google Scholar 

  12. Craig, W., Guyenne, P., Kalisch, H.: Hamiltonian long wave expansions for free surfaces and interfaces. Commun. Pure Appl. Math. 58(12), 1587–1641 (2005)

    Article  MathSciNet  Google Scholar 

  13. Craig, W., Guyenne, P., Nicholls, D., Sulem, C.: Hamiltonian long-wave expansions for water waves over a rough bottom. Proc. R. Soc. Lond. Ser. A 461, 839–873 (2005)

    MathSciNet  MATH  Google Scholar 

  14. Craig, W., Wayne, C.: Mathematical aspects of surface water waves. Russ. Math. Surv. 62(3), 453–473 (2007)

    Article  Google Scholar 

  15. Didenkulova, I., Pelinovsky, E.: Non-linear wave evolution and run-up in an inclined channel of a parabolic cross-section. Phys. Fluids 23, 086602 (2011)

    Article  Google Scholar 

  16. Didenkulova, I., Pelinovsky, E.: Rogue waves in nonlinear hyperbolic systems (shallow-water framework). Nonlinearity 24, R1–R18 (2011)

    Article  MathSciNet  Google Scholar 

  17. Dobrokhotov, S., Medvedev, S., Minenkov, D.: On transforms reducing one-dimensional systems of shallow-water to the wave equation with sound speed \(c^2 = x\). Math. Notes 93, 704–714 (2013)

    Article  MathSciNet  Google Scholar 

  18. Dobrokhotov, S., Nazaikinskii, V., Tirozzi, B.: Asymptotic solution of the one-dimensional wave equation with localized initial data and with degenerating velocity: I. Russ. J. Math. Phys. 17(4), 434–450 (2010)

    Article  MathSciNet  Google Scholar 

  19. Dobrokhotov, S., Tirozzi, B.: Localized solutions of one-dimensional non-linear shallow-water equations with velocity \(c=\sqrt{x}\). Russ. Math. Surv. 65(1), 177–179 (2010)

    Article  Google Scholar 

  20. Garayshin, V., Harris, M., Nicolsky, D., Pelinovsky, E., Rybkin, A.: An analytical and numerical study of long wave run-up in u-shaped and v-shaped bays. Appl. Math. Comput. 297, 187–197 (2016)

    MathSciNet  MATH  Google Scholar 

  21. Harris, M., Nicolsky, D., Pelinovsky, E., Pender, J., Rybkin, A.: Run-up of nonlinear long waves in u-shaped bays of finite length: analytical theory and numerical computations. J. Ocean Eng. Mar. Energy 2, 113–127 (2016)

    Article  Google Scholar 

  22. Harris, M., Nicolsky, D., Pelinovsky, E., Rybkin, A.: Runup of nonlinear long waves in trapezoidal bays: 1-D analytical theory and 2-D numerical computations. Pure Appl. Geophys. 172, 885–899 (2015)

    Article  Google Scholar 

  23. Johnson, R.S.: A Modern Introduction to the Mathematical Theory of Water Waves. Cambridge University Press, Cambridge (1997)

    Book  Google Scholar 

  24. Kanoglu, U.: Nonlinear evolution and runup-drawdown of long waves over a sloping beach. J. Fluid Mech. 513, 363–372 (2004)

    Article  MathSciNet  Google Scholar 

  25. Kanoglu, U., Synolakis, C.: Initial value problem solution of nonlinear shallow water-wave equations. Phys. Rev. Lett. 148501, 97 (2006)

    Google Scholar 

  26. Kanoglu, U., Synolakis, C. E.: Tsunami dynamics, forecasting, and mitigation. In: Shroder, J. F., Ellis, J. T., Sherman, D. (Eds.) Chapter 2 Hazards and Disasters Series: Coastal and Marine Hazards, Risks, and Disasters. Elsevier, pp. 15–57 (2015). https://doi.org/10.1016/B978-0-12-396483-0.00002-9

  27. Kanoglu, U., Titov, V., Bernard, E., Synolakis, C.: Tsunamis: bridging science, engineering and society. Philos. Trans. R. Soc. A 373(2053), 20140369 (2015)

    Article  Google Scholar 

  28. Lannes, D.: The water waves problem: mathematical analysis and asymptotics. In: Mathematical Surveys and Monographs. American Mathematical Society, Providence vol. 188, p 321 (2013) ISBN 978-0-8218-9470-5

  29. Madsen, P., Fuhrman, D., Schäffer, H.: On the solitary wave paradigm for tsunamis. J. Geophys. Res. Oceans 113, C12012 (2008). https://doi.org/10.1029/2008JC004932

  30. Nicolsky, D., Pelinovsky, E., Raza, A., Rybkin, A.: General initial value problem for the nonlinear shallow water equations: runup of long waves on sloping beaches and bays. Phys. Lett. A 382(38), 2738–2743 (2018)

    Article  MathSciNet  Google Scholar 

  31. NTHMP (ed.): Proceedings and results of the 2011 NTHMP Model Benchmarking Workshop, NOAA Special Report, Boulder, CO. U.S. Department of Commerce/NOAA/NTHMP, National Tsunami Hazard Mapping Program [NTHMP], pp. 436 (2012)

  32. Pelinovsky, E.: Waves in geophysical fluids. In: Grue, J., Trulsen, K. (Eds.) Hydrodynamics of Tsunami Waves, pp. 1–48. CISM Courses and Lectures, No. 489. Springer, Berlin (2006)

  33. Raz, A., Nicolsky, D., Rybkin, A., Pelinovsky, E.: Long wave run-up in asymmetric bays and in fjords with two separate heads. J. Geophys. Res. Oceans 123(3), 2066–2080 (2018)

    Article  Google Scholar 

  34. Rybkin, A., Pelinovsky, E., Didenkulova, I.: Non-linear wave run-up in bays of arbitrary cross-section:generalization of the Carrier–Greenspan approach. J. Fluid Mech. 748, 416–432 (2014)

    Article  MathSciNet  Google Scholar 

  35. Stoker, J.: Water Waves: The Mathematical Theory with Applications. Interscience Publishers, New York (1957)

    MATH  Google Scholar 

  36. Synolakis, C.: The runup of solitary waves. J. Fluid Mech. 185, 523–545 (1987)

    Article  MathSciNet  Google Scholar 

  37. Synolakis, C.: Tsunami runup on steep slopes: how good linear theory really is? Nat. Hazards 4, 221–234 (1991)

    Article  Google Scholar 

  38. Synolakis, C., Bernard, E.: Tsunami science before and beyond Boxing Day 2004. Philos. Trans. R. Soc. A 364, 2231–2265 (2006)

    Article  MathSciNet  Google Scholar 

  39. Synolakis, C., Bernard, E., Titov, V., Kanoglu, U., Gonzalez, F.: Validation and verification of tsunami numerical models. Pure Appl. Geophys. 165, 2197–2228 (2008)

    Article  Google Scholar 

  40. Tuck, E., Hwang, L.: Long wave generation on a sloping beach. J. Fluid Mech. 51, 449–461 (1972)

    Article  Google Scholar 

  41. Zahibo, N., Pelinovsky, E., Golinko, V., Osipenko, N.: Tsunami wave runup on coasts of narrow bays. Int. J. Fluid Mech. Res. 33, 106–118 (2006)

    Article  Google Scholar 

Download references

Acknowledgements

We would like to thank anonymous referees for careful reading of the manuscript and valuable comments, which have been very helpful in improving the manuscript. Also, we are grateful to Dillon Gillespie for his help with computations of the Bessel–Fourier expansion. Alexei Rybkin acknowledges support from National Science Foundation Grant (NSF) award DMS-1411560 and DMS-1716975. Dmitry Nicolsky acknowledges support from the Geophysical Institute, University of Alaska Fairbanks. Efim Pelinovsky acknowledges support by Laboratory of Dynamical Systems and Applications NRU HSE, by the Ministry of science and higher education of the RF Grant ag. 075-15-2019-1931 and by FRBR Grant 18-05-80019 and 20-05-00162. Maxwell Buckel was supported by the National Science Foundation Research Experience for Undergraduate program (Grant DMS-1411560).

Author information

Authors and Affiliations

  1. Department of Mathematics and Statistics, University of Alaska Fairbanks, Fairbanks, USA

    Alexei Rybkin & Maxwell Buckel

  2. Geophysical Institute, University of Alaska Fairbanks, Fairbanks, USA

    Dmitry Nicolsky

  3. National Research University-Higher School of Economics, Moscow, Russia

    Efim Pelinovsky

  4. Institute of Applied Physics, Nizhny Novgorod, Russia

    Efim Pelinovsky

  5. Nizhny Novgorod State Technical University n.a. R.E. Alekseev, Nizhny Novgorod, Russia

    Efim Pelinovsky

Authors
  1. Alexei Rybkin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Dmitry Nicolsky
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Efim Pelinovsky
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Maxwell Buckel
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Efim Pelinovsky.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Rybkin, A., Nicolsky, D., Pelinovsky, E. et al. The Generalized Carrier–Greenspan Transform for the Shallow Water System with Arbitrary Initial and Boundary Conditions. Water Waves 3, 267–296 (2021). https://doi.org/10.1007/s42286-020-00042-w

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s42286-020-00042-w

Keywords

Search

Navigation