A scaling property of Farey fractions. Part III: representation formulas

Abstract

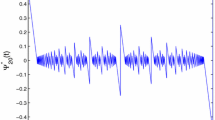
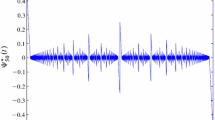
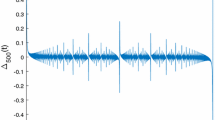
The Farey sequence of order n consists of all reduced fractions a / b between 0 and 1 with positive denominator b less or equal to n. The sums of the inverse denominators 1 / b of the Farey fractions in prescribed intervals with rational bounds have simple main terms, whereas the deviations are determined by a sequence of polygonal functions $f_n$. In a former paper we obtained a limit function for $n \rightarrow \infty$ which describes an asymptotic scaling property of functions $f_n$ in the vicinity of any fixed fraction a / b and which is independent of a / b. In this paper we derive new representation formulas for $f_n$ and related functions which give much better remainder term estimates. We also combine these results with those from our previous papers in order to prove that the sequence of functions $f_n$ converges pointwise to zero.