Introduction

Surrogate bone materials are often used in testing when natural bone is not obtainable, or if more consistent mechanical properties are required than what would be found in a natural material that is not as homogeneous. For example, surrogate bone material is used to evaluate the performance of machine tools used in the medical industry [1], or to obtain diagnostic information such as limb loading or implant behavior [2–4]. One of the primary challenges in developing a bone surrogate material is the ability to accurately mimic the material properties of natural cortical bone, including its rate and orientation mechanical response. In addition to having the correct material response, a surrogate material must also be biocompatable in order to not pose a health risk if used as an implant, such as mechanical fasteners and bone adhesives. Mechanical fasteners are extensively used for traumatic injury [5, 6] and adhesives are actively researched in order to find the ideal chemical composition that allows for temporary fixation while the body has time to regrow and heal [7]. This body of work investigates rate-dependent behavior as a function of microstructure in dry ox cortical bone compared to a commercially available dry cortical bone surrogate, and determines the role microstructure plays in the dynamic fragmentation of these materials.

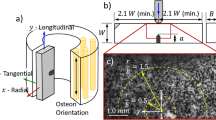
Material Microstructure

Cortical bone is commonly classified as an organic composite made from three primary components: the osteon, cement lines, and Haversian canals that are interconnected orthogonally by Volkmann canals [8–10]. A schematic of the microstructure is provided in Fig. 1. The osteons act as longitudinal support structures within the cortical bone and are bonded together along cement lines, while the Haversian canals are the pathways for interstitial fluid and pass through the center of the osteon [11]. With this configuration, cortical bone can be treated as a transversely isotropic material with a preferential longitudinal direction parallel to the osteons [12, 13].

Fig. 1
figure 1

Schematic of long bone showing cortical region (not to scale) and microstructure. The osteons run parallel to the length of the bone, giving it a preferential direction with Haversian canals in the middle of the osteon

Full size image

The orientation of cortical bone within the human body determines the physiological response during impact events in everyday motion, as well as under extreme loading conditions. It should be noted that while the orientation dependence of the osteon has a large effect on the overall strength of the bone, there are other systems acting in unison that provide support, such as tendons, ligaments, and overall mineral content [14, 15]. For this work, the physiological sub-components are not considered in the response of the material and the focus is on how the microstructure of cortical bone and a cortical bone surrogate material affects the uniaxial compressive quasi-static and dynamic material response, and resulting fragmentation.

Bone Background

Extensive studies on cortical bone have been performed to help characterize the behavior of this naturally hierarchical structure [16–20]. Uniaxial compression testing of cortical bone is typically performed at strain-rates of 10−3 s−1 to 103 s−1 which represents physiological loading rates of mammals walking or lightly exercising to high impact events such as falls or automobile accidents. Insight on typical strain-rate behavior of human bone has been gained through in-vivo testing of cortical tibia bone subjected to light and moderate loading activity [16]. Generally speaking, cortical bone is well adapted to the human body by absorbing greater energy during impact loading due to its strain-rate sensitivity. Testing in literature is typically focused on human and bovine cortical bone. These materials are, in a general sense, comparable since they have a similar microstructures (i.e. the periodicity and size of osteons and cement lines) and constitutive responses. Often bovine bone is used in instances where human bone may be difficult to acquire or poses biological issues such as communicable diseases.

Early studies on the compressive strength of cortical bone by McElhaney [17] provided insight into its strain-rate behavior. Testing was performed in quasi-static loading and using a drop-weight compression test on wet bovine femoral specimens. Measured compressive strength was 175 ± 32 and 365 ± 38 MPa, respectively. Testing on embalmed-dry human femoral bone was 15% weaker at both rates [17]. Conventional uniaxial dynamic compression tests performed with a Kolsky (split-Hopkinson) bar have also been used to characterize cortical bone and to investigate the strain-rate sensitivity behavior [18–20]. A more recent study by Sanborn et al. [18] on human femoral bone yielded similar compressive strengths, with longitudinal strength of 152 ± 22 MPa at a strain-rate of 10−3 s−1 increasing to 319 ± 24 MPa at 103 s−1. When loaded in the transverse orientation, the strengths decreased to 87 ± 22 MPa at 10−3 s−1 and 179 ± 26 MPa at 103 s−1 [18].

Compression testing at strain-rates of 103 s−1 were carried out by Adharapurapu et al. [19] on both wet and dried bovine femoral cortical bone. They reported average results which show that cortical bone has a longitudinal strength of 459 to 556 MPa for wet and dried bone, and 296 to 363 MPa for transverse strength in the same conditions [19]. Similar compression testing performed by Ferreira et al. [20] on wet bovine femoral cortical bone found an ultimate compressive strength of 240 ± 66 to 281 ± 42 MPa for transverse and longitudinal orientations, respectively.

The spread of results in similar testing highlights the variability in specimens for the same mammalian species and bone type (femoral cortical), as well as comparisons to human specimens. The cause for variability can come from physical traits such as specimen age post-mortem [21] or age of the animal prior to harvesting the bone [22]. Variability also can arise from the storage and preservation methods used in other work [23].

The first case of specimen age was investigated by Tennyson et al. for bovine femoral bone in which they found a 33 % reduction in stiffness after letting the specimen age in cold water for a span of 14 days [21]. The second case relates to the breakdown of bone that accumulates within the body as it ages. Qualitative studies of the breakdown process were done on human femoral bone by Schaffler et al. [22] by observing the quantity of microcracks in specimens with age and noting a large increase in microcrack density over the age of 40 with a greater density in female specimens. Quantitative data that supports Schaffler’s findings was obtained by Zioupos et al. [24] in their research on fracture toughness values, stiffness, and strength of human femoral cortical bone showing a reduction in all three with increase in age. A study of the ideal storage conditions for bone by Stefan et al. suggest that if storage is required, it is best to freeze wet bone instead of using a preservation or embalming approach which can chemically alter the bone, and hence alter the mechanical properties [23].

Research on the fracture properties of both bovine and human cortical bone have been performed to help characterize the behavior with respect to the loading orientation and rate. Notable work in this field came from Bonfield and Behiri where they determined the off-axis relation for fracture toughness using fresh-frozen bovine femoral bone that was recovered with Ringer’s solution in compact tension tests. Longitudinal loading gave a measured \(K_c\) value of 3 MPa\(\sqrt{\mathrm{m}}\) which increased to 6.5 MPa \(\sqrt{\mathrm{m}}\) in the transverse orientation [25]. More recently, the rate-dependent anisotropic fracture properties of wet human cortical bone in four-point bend was examined by Shannahan et al. [26], determining a quasi-static mode-I (opening) stress intensity factor upon crack initiation of 8.4 MPa \(\sqrt{\mathrm{m}}\), and a dynamic mode-I stress intensity factor of 2.6 MPa \(\sqrt{\mathrm{m}}\). Their results suggest that the conventional assumption of isotropy is a conservative estimate for bone fracture initiation at low loading rates, but overestimates fracture strength at dynamic loading rates [26]. A thorough review of the fracture behavior of human and bovine specimens has been compiled by Ritchie et al. [12].

Fragmentation Background

Previous studies on the fragmentation of cortical bone tend to not focus on the mechanics of the process, but rather, are more limited to archaeological surveys of mammalian bone fragments [27, 28]. Pioneering studies of engineering (i.e. nonbiological) material fragmentation were carried out by Mott [29] during the Second World War to better understand how shell casing and explosive ordinances break apart. His work focused on modeling the material as a rapidly expanding ring that produced distributions of fragment sizes depending on the expansion rate of the ring and any localized hardening [29]. It has since been shown for many brittle materials, including structural ceramics such as boron carbide (B4C) [30] and aluminum oxynitride (AlON) [31], that fragmentation resulting from dynamic compressive loading produces a range of resulting fragment sizes that can be directly correlated to the microstructural inherent flaws and failure mechanisms [32]. The fragmentation of boron carbide tested under uniform dynamic compression was investigated by Hogan et al. and found that fragments for hard ceramics follow a bimodal distribution. The two modes indicate failure as a structural process (larger fragments) or as a secondary process from Poisson effects that produces smaller fragments [30]. In general, it has been shown for these structural ceramics that distributions of fragment sizes will vary depending on the material and applied strain-rate [33]. Ductile materials typically have an exponential fragment size distribution while brittle materials tend to follow a power-law distribution [34, 35]. One advantage of utilizing the power-law distribution is that it is not dependent on a characteristic fragment length (typically an average fragment size). Leveraging these findings, this study explores the dynamic fragmentation of two nominally brittle materials with differing microstructures from traditional structural ceramics. This effort will be accomplished by testing the mechanical response of dry ox and bone surrogate at varying strain-rates and orientations and analyzing the resulting fragmentation characteristics as a function microstructure.

Experimental Method

Specimens of dry cortical bone from an ox femur and a human cortical bone surrogate made from bovine cortical bone and a resin binder material were tested at quasi-static and dynamic uniaxial compression strain-rates of 10−3 and 103 s−1. The resulting fragmentation characteristics from optical micrographs were analyzed within MATLAB to measure characteristic geometrical features. Additionally, scanning electron microscope (SEM) images of the fragments are examined to aid in correlating the resulting fragment sizes to failure processes during fragmentation.

Materials

Dry ox cortical bone was cut from a single 20 × 20 × 4 mm femoral coupon using a low-speed diamond wafering blade to 3.5 mm cubic specimens. The surrogate cortical bone material was obtained from BoneSim Laboratories (BoneSim 1800 [1]). The material consists of a proprietary blend of dry bovine cortical bone that has been ground to a particle size of 200–750 μm, mixed with a cyanoacrylate based adhesive and allowed to cure while subjected to die pressing to form a transverse isotropic disc. The surrogate material came from the manufacturer as a 50 mm round compressed disc 9.7 mm thick. One manufactured disc was used to cut 7 mm cubic specimens. Orientations were noted during processing with respect to the osteon path and die compaction direction. The loading sides of the specimens were polished to a high degree of parallelism to ensure uniform loading across the surfaces, and to a 3 μm diamond finish on the loading faces.

Fig. 2
figure 2

Optical microscopy image of ox bone in longitudinal orientation showing osteon arrangement. Haversian canals are visible as black dots in the center of the osteon

Full size image
Fig. 3
figure 3

Optical microscopy image of bone surrogate prior to testing in the longitudinal orientation showing random orientation of bone fragments. A collection of osteons and pores in the resin binder is highlighted and confirmed using SEM in Fig. 4

Full size image

The loading surface for longitudinal orientation of the bone and surrogate prior to testing can be seen in Figs. 2 and 3. The surrogate has a uniform distribution of randomly oriented bone fragments, with few having the osteon running parallel to the die-press direction as highlighted in Fig. 3. In this regard, there is little similarity in the microstructure of the ox bone and bone surrogate. The bone surrogate lacks the osteon support structures found in the ox bone and instead has a large quantity of interfacial flaws and pores in the binder material.

A scanning electron microscope (SEM, FEI XL30) with energy dispersive x-ray spectroscopy (EDX) was used to examine the surrogate material and identify the elemental composition of regions within the material which are provided in Fig. 4 and Table 1.

Fig. 4
figure 4

SEM of typical surface of surrogate material. EDX scans were recorded at the points marked EDX-1 and EDX-2. Region R1 is inspected at higher magnifications in Fig. 5

Full size image

EDX measurements were recorded for a period of 100 s at the locations marked ‘EDX-1’ and ‘EDX-2’ in Fig. 4. The location ‘EDX-1’ was identified as an osteon in Fig. 3 and ‘EDX-2’ appeared as a distinct region separate from the previous measurement and is believed to be the resin binder.

Table 1 EDX weight percentage measurements for two distinct regions in surrogate material for 100 s (refer to Fig. 4)
Full size table
Fig. 5
figure 5

SEM of bone surrogate region ‘R1’ (Fig. 4) illustrating pre-existing damage. Inset image shows evidence of crack bridging

Full size image

The osteon identified in Fig. 4 was found to have the majority of its weight percentage (wt%) as calcium and phosphorus, which is typical of bone. The darker regions in Fig. 4 had a significantly smaller weight percent of calcium and phosphorus with the majority of its composition coming from carbon. The contrast provided by the SEM allowed for an identification of 78 % cortical bone content with the remainder being the cyanoacrylate based adhesive acting as a binder. The granular bone particles were found to have a random orientation within the binder with respect to the osteon path and surrogate press direction.

Extensive pre-existing damage in the form of microcracks was found on the bone fragments in the surrogate material. The binder showed evidence of numerous pores in the bulk of the material as well as along the interface between the binder and bone. A region designated ‘R1’ in Fig. 4 was identified as having damage and is shown in more detail in Fig. 5. The inset shows that at higher magnifications there is evidence of crack bridging which is explained in greater detail by Ritchie for metals, ceramics, composites [36], and cortical bone [12], where for bone the microcracks are bridged by collagen fibers within the tissue. Microcracks and crack bridging was found throughout the bone surrogate specimens prior to testing.

Experimental Configuration

Both materials were subject to uniaxial compression using a Shimadzu AG-IS 50 kN loading frame at a loading rate of 0.15 mm s-1 (strain-rate or \(\dot{\varepsilon }\) of 10−3 s−1) in longitudinal and transverse orientations. Dynamic uniaxial compression was performed for both materials and orientations with a Kolsky bar at a strain-rate of 103 s−1. A schematic of the bar arrangement is shown in Fig. 6.

Fig. 6
figure 6

Kolsky bar arrangement for dynamic uniaxial compression. Callout image of ox bone specimen between platens, and incident and transmitted bars

Full size image

Its principle of operation is through the propagation of a stress pulse. Two round hardened steel bars known as the incident and transmitted bar are supported by linear bearings. A specimen is placed between the two bars and a stress pulse is generated in the incident bar by impacting it with a striker shot from a gas gun. The governing equations for a Kolsky bar are based on 1D wave mechanics and are provided in Eqs. 1–3. Strain-rate is determined through the reflected strain in the incident bar \(\varepsilon _R\) with the time component coming from the longitudinal wave speed of the bar, denoted \(c_b\). The length is normalized by the specimen length in the loading direction, \(L_0\). By integrating the strain-rate over the loading time of the test the strain for the specimen, \(\varepsilon (t)\) can be calculated. Finally, the strain recorded in the transmitted bar can be used with the Young’s modulus of the bar, \(E_b\) and ratio of bar area to specimen area, \(A_b/A_0\) to calculate the stress in the specimen, \(\sigma (t)\). In order to reach a state of uniform loading, the stress pulse needs to traverse the length of the specimen a few times (incident pulse with first and second reflections within the specimen). For the size of the specimens used in this experiment and estimating the material wave speed at 2990 m/s for bone, it would take 3.5–7 μs for the ox bone and bone surrogate to reach a uniform loading state. More details on this type of testing can be found in [37, 38].

$$\begin{aligned} \dot{\varepsilon }(t)= & {} -\frac{2c_b}{L_0}\varepsilon _R(t)\end{aligned}$$
(1)
$$\begin{aligned} {\varepsilon }(t)= & {} \int _0^t \dot{\varepsilon }(t)dt \end{aligned}$$
(2)
$$\begin{aligned} {\sigma }(t)=\, & {} \frac{E_b A_b}{A_0}\varepsilon _T(t) \end{aligned}$$
(3)

A 150 mm long 12.7 mm diameter striker was loaded into a light-gas gun to provide the input pulse when incident upon the Kolsky bar. The specimen was placed between a pair of aluminum oxide (Al2O3) platens located between the incident and transmission bars. A small amount of silicone grease was used between the bars, platens, and specimen to reduce interface friction between the specimen and platens. A small piece of copper, 4.2 mm round by 0.5 mm thick was placed in front of the incident bar as a pulse shaper to extend the loading time and help obtain uniform loading conditions[37].

Data collection was performed with a 200 MHz LeCroy HDO 4024 oscilloscope in conjunction with a pair of half-bridge circuits instrumented on each bar. The strain gages are bonded to each bar 180° apart to allow cancellation of bending within the bar and provide pulse characterization as the stress wave propagates along the bar.

Fragment Characterization

A containment box made from 2 mm thick polycarbonate was placed around the testing section of the Kolsky bar and used to capture fragments from the dynamic loading. Care was taken to collect the majority of fragments for analysis. It should be noted that the aluminum oxide platens were recovered completely intact with no signs of damage and all fragments are believed to be from the tested specimen. The fragments were then imaged using a Zeiss Axiocam MRc with a low-power objective lens and analyzed using MATLAB’s Image Processing Toolbox to determine relevant geometric characteristics [39].

The analysis process consists of first converting the images to grayscale in order to adjust contrast and sharpness of the fragment outline then further converted to a binary image. An idealized ellipse is fit to each fragment, which is then used to determine parameters such as major and minor axis length. The major axis length is used for the characteristic size L of a fragment and the ratio of the two axes defines the shape or aspect ratio (AR). The area of each fragment was also measured using contiguous pixel mapping. An example of a surrogate fragment is shown in Fig. 7 along with a typical analysis of the surface. Details for this technique can be found in [40].

Fig. 7
figure 7

a Optical microscopy of typical surrogate fragment. b Fragment processed and fit with idealized ellipse: 3.9, 3.2 mm, 1.25 (major axis, minor axis, AR)

Full size image

Results

The following section outlines the results of mechanical testing and fragmentation analysis. Specimens of each material were tested in the longitudinal and transverse orientation for both uniaxial quasi-static and dynamic loading. The resulting fragments were then analyzed for geometric parameters, and fragment size distributions for type of dependence on fragment length L.

Uniaxial Compressive Results

A summary of the compressive strengths are provided in Table 2. The mechanical response for both materials and orientations under quasi-static loading is provided in Fig. 8. The dry ox bone was found to have a quasi-static compressive strength 3–4 times greater than the surrogate material. One reason for this difference may be due to the fact that the commercially available surrogate bone aims to match wet cortical bone which has a smaller maximum compressive strength as compared to the dry bone used in this study. For example, Adharapurapu et al. found that wet bone was 20 % weaker than its dry counterpart [19]. The dry ox bone also had a microstructure that favors the longitudinal direction, whereas the surrogate did not have this orientational dependency. The bone was found to have a different mechanical response between the longitudinal and transverse orientation, which is attributed to the inherent microstructural anisotropy. Longitudinal strength of the dry cortical bone was 38 % higher than transverse strength at quasi-static loading rates and 60 % higher at strain-rates of 103 s−1. The surrogate does not appear to show a significant difference between loading orientations despite the die-pressing, perhaps due to its random bone fragment orientations and relatively uniform distribution, and hence nominally isotropic microstructure.

Table 2 Summary of results from quasi-static and dynamic uniaxial compression testing, reported values are averaged from two replicated tests with standard deviation (±SD)
Full size table
Fig. 8
figure 8

Characteristic results for quasi-static (\(\dot{\varepsilon }=10^{-3}\) s−1) uniaxial compressive response of ox bone and bone surrogate in both the longitudinal and transverse orientation

Full size image
Fig. 9
figure 9

Stress history of dynamic loading (\(\dot{\varepsilon }=10^3\) s−1) for ox bone and surrogate in longitudinal and transverse loading, portion of unloading is shown to illustrate peak compressive stress

Full size image

Stress history or \(\sigma _c(t)\) is provided for the dynamic tests in Fig. 9. The ox bone had a peak compressive strength 66 % greater in the longitudinal orientation and 43 % greater in the transverse orientation for dynamic loading compared to the quasi-static. The surrogate had similar compressive strengths when loaded longitudinally in dynamic testing as compared to the transverse orientation with overlapping error between the two results. The strain-rate sensitivity was also less than what was found with the ox bone, having a peak compressive strength increase of 20–27 % over the quasi-static values when tested at dynamic loading conditions. It should be noted that the quasi-static and dynamic stress histories as shown in Figs. 8 and 9 through the peak stress, where both materials behaved in a nominally brittle manner in that when the sample could no longer hold load, it failed via full fragmentation. Normalized dynamic loading results are provided in Fig. 10. The dynamic compressive stress and loading time is normalized by the dynamic maximum compressive strength and loading time of the longitudinally-oriented ox bone. From Fig. 10, the ox bone exhibits an orientation dependency as shown by the difference in heights, while the bone surrogate does not.

Fig. 10
figure 10

Dynamic maximum strength and loading time normalized by dynamically loaded (\(\dot{\varepsilon }=10^3\) s−1) longitudinal ox bone data \(\sigma _{c,BL}\)

Full size image

Fragmentation Analysis

Captured fragments were imaged and processed using the method described in the experimental procedure. Results of the processed data were compared between individual tests, as well as combining the fragments from identical tests. Scatter plots of the distribution were used to compare the individual tests to the combined data, and it was found that both data sets produced similarly trended distributions. In order to increase the fidelity of the results, the combined data sets are used for the rest of the analysis which allows for an increase of representative population for each material and orientation with respect to loading, while not affecting the overall distributions. Fragment characteristics for each material set are provided in Table 3. It should be noted that the ox bone had a smaller data set which is related to the smaller size of the original test specimen as compared to the bone surrogate (see "Materials" Section).

Table 3 Characteristics of fragments larger than 100 μm generated during dynamic uniaxial compressive loading. Standard error of the mean (±SEM) is provided for the fragment size, AR, and area
Full size table

Scatter plots for the distribution of fragment shape and size are provided in Figs. 11 and 12.

Fig. 11
figure 11

Ox bone fragment size characteristics from dynamic loading (\(\dot{\varepsilon }=10^3\) s−1)

Full size image
Fig. 12
figure 12

Bone surrogate fragment size characteristics from dynamic loading (\(\dot{\varepsilon }=10^3\) s−1). Shaded regions highlight the two fragment size regimes

Full size image

The distribution of fragments for the ox bone was found to contain both a population of small (<100 μm) and large fragments in the longitudinal and transverse loading orientations as evidenced by the fragment size characteristics. Fragments generated during the dynamic loading of the surrogate fell into two primary size regimes for both orientations. Larger fragments were generated on the order of 1 mm which represent the family size for structurally dependent failure, whereas smaller fragments found with a typical size of 50−100 μm represent Poisson effects and comminution of larger fragments. The analysis of this work will focus on the larger fragments as these represent the structural failure that is linked to the material microstructure.

To gain more insight on the fragmentation, the size of the fragments was plotted against different distributions, including exponential, log-normal, Weibull, Rayleigh and a power-law. It was found that the bone and bone surrogate fragment sizes most closely exhibited a power-law dependency, as has been shown to be suitable for other brittle solids [41]. For example, the power-law distribution was observed in earlier research by Bergstrom on static compression testing of glass spheres [42], and on torsional Hopkinson bar testing of ferroelectric ceramics by Costin [43]. Based on their findings, Grady formulated a power-law fit specifically for brittle materials [41] which has the form,

$$\begin{aligned} G(L)=\frac{1}{1+\left( \frac{L_i-L_{min}}{a}\right) ^b}, \end{aligned}$$
(4)

where a is a scaling parameter to normalize the fragment length and is related to the applied strain-rate where higher strain-rates have a smaller value. The parameter b is for shape fitting and has a reducing absolute value for higher strain-rates. Using the above power-law distribution form, the dynamic fragmentation distributions of the bone and surrogate are provided in Fig. 13.

Fig. 13
figure 13

Cumulative distributions of fragment size for L ≥ 100 μm of surrogate material from dynamic loading (\(\dot{\varepsilon }=10^3\) s−1). Experimental data is fit with a power-law distribution from Eq. 4 (for scaling parameters see Table 4)

Full size image
Table 4 Scaling parameters used in the power law fit, Eq. 4. Parameter a normalizes the fragment length and b shapes the profile. The absolute value of each parameter decreases with increasing strain-rate
Full size table

Discussion

Uniaxial Compressive Response

The uniaxial compression strength of the cortical bone was found to be similar to other published data for both quasi-static and dynamic compressive loading when taking into account the variability (species [17], age [21], storage conditions [23]) of specimens among published work. A comparison of the longitudinal compressive strength and strain-rate from present work and published data is provided in Fig. 14. Early studies from McElhaney on embalmed human femoral bones show a relatively low strength [17], owed likely to the embalming process and chemicals used, as demonstrated by Stefan et al. [23]. Testing of wet bovine femoral bone by Ferreria et al. achieved a lower strength than that of Adharapurapu [19, 20]. The dry bovine femoral bone that Adharapurapu investigated had a compressive strength that matched the ox bone at strain-rates of 103 s−1 found in this study, and was 44% weaker than the ox bone at quasi-static rates of 10−3 s−1. The difference between strength at lower loading rates could be due to the difference in species, as well as age and conditioning of the specimen.

Fig. 14
figure 14

A comparison of longitudinal compressive strength and applied strain-rate from present work and published data. (D) dry bovine femur, (W) wet (fresh) bovine femur, (E) embalmed-dry human femoral [17, 19, 20]

Full size image

Testing of the dry ox cortical bone provided a baseline to compare the behavior of the surrogate material. Bone exhibits anisotropic behavior due to the microstructure highlighted in Fig. 1, whereas the surrogate appears nominally homogeneous as evidenced in Figs. 3 and 4. Cortical bone appears to have both a rate and orientation dependency, with the longitudinal direction having a higher load bearing capacity owed to the preferential osteon direction. The cortical bone compressive strength increased 67 % in the longitudinal direction from quasi-static to dynamic loading, and 43 % in transverse (with errors of 4 and 9 %, respectively).

Overall, the quasi-static compressive strength for the surrogate was lower than the ox femur. This is likely because the ox bone is dry and consequently more brittle than wet bone specimens the surrogate aims to simulate. Similar work by Adharapurapu et al. has shown that dry bone is capable of supporting more load than wet bone due to the increase in stiffness as moisture is removed, leading to a more brittle material [19].

The bone surrogate material also exhibited a rate dependency with peak stress. When loaded dynamically, the compressive strength increased 27 % in the longitudinal orientation from quasi-static loading, but exhibited no statistically significant difference in the transverse with loading rate. The bone surrogate aims to be a consistent homogenized material with low variability between testing. While the surrogate had less deviation from the mean values, the overall strength was weaker and thus the coefficient of variation (ratio of standard deviation to mean) can be used to measure the consistency of testing results [44]. The quasi-static compressive response of ox bone had a coefficient of variation for longitudinal and transverse loading of 0.02 and 0.09 compared to the bone surrogate of 0.06 and 0.11. The dynamic compressive response was marginally better for the surrogate material which had a coefficient of variation of 0.07 for both longitudinal and transverse orientations. This metric was similar for ox bone under dynamic compression at 0.04 for longitudinal and 0.09 for transverse.

Pre-existing damage within the untested surrogate material can be observed in region R1 as shown in Fig. 5. A large quantity of flaws with a characteristic length of 2−4 μm are visible. At higher magnification there is evidence of crack bridging at the flaw which is typical of cortical bone [12]. Damage of this magnitude is common throughout the bone regions in the material and is attributed to mechanical processing of the bone (grinding and die pressing) during the manufacturing of the material. One possible reason for the smaller rate dependency of the surrogate is due to the activation of numerous flaws in the surrogate that do not exist in the natural bone. Flaws were found to exist along the interfaces between the resin binder and the bone due to poor adhesion or as defects such as pores in the bulk of the resin binder, and the implications of these types of flaws and locations on their fragmentation will be discussed in the next section.

Resulting Fragmentation

An analysis of the bone fragments shows a relatively uniform distribution between small and large fragments when compared to the bone surrogate (Fig. 11). One possibility for the spread of fragment sizes is that the failure mechanism lies along the cement lines of the osteon where fracture occurs due to localized plasticity and pore collapse. The failure process generates longer fragments during loading on the order of 500–1000 μm, with smaller fragments generated on the length scales of typical Volkmann canal spacing that connect adjacent Haversian canals perpendicular to the osteon [10]. Given these findings, we hypothesize that the canal systems act as points for the crack to change direction and break off a larger osteon structure. Evidence of this behavior is provided in Figs. 15 and 16. Interestingly, the fragments analyzed in Fig. 15 show the different canal paths exposed. Previous work on the fracture mechanics of cortical bone [12, 25] suggested that the cement lines between osteons provides the weak path that the crack would follow. Since the canal systems are exposed, this would suggest that the osteon split during the fracture process to expose the inner surfaces of the osteon canal system.

Fig. 15
figure 15

SEM image of fragments generated from dynamic loading of dry ox bone in the longitudinal orientation. Splitting of the osteon revealed the internal canal system

Full size image
Fig. 16
figure 16

SEM image of fragments generated from dynamic loading of dry ox bone in the transverse orientation. The extensive crushing shown is from the surface of a single fragment. The textured surface was present on all fragments with no visible indication of a canal system

Full size image

The transverse loading of dry ox bone produced an even distribution of fragments that suggests failure of the osteon through a comminution behavior before reaching a weak cement line that deflects the crack from perpendicular to parallel to the osteon, consequently producing a more uniform distribution of fragment sizes [45]. Figure 16 shows evidence of the comminution behavior. After studying multiple transverse fragments there was no definitive evidence of osteon splitting like what was seen in the longitudinal fragments.

The characteristic length of a typical surrogate fragment fell within two regimes, as shown in Fig. 12. Like their brittle structural ceramic counterparts, larger fragments (on the order of 1000 μm) are believed to be generated through structural failure, while smaller fragments (on the order of 50 μm or less) are formed from secondary processes. It is likely that smaller fragments resulted from the crushing of osteons as few instances of preferential directions could be found in microscopy (such as the longitudinal bone section shown in Fig. 3). An analog to this behavior in ceramics and rocks is transgranular fracture [32, 40]. SEM images of the surrogate did not show noticeable differences in the longitudinal and transverse loading specimens. In both orientations, a common finding was a large number of pores existing along the interface of the ground bone and binder as shown in Fig. 17. The presence of the pores suggests that there was a lack of proper wetting of the ground bone by the binder phase, leading to a weak interaction of the adhesive and bone. The quantity and size of pores was not consistent, with sizes ranging from a few microns to over 100 μm.

Fig. 17
figure 17

SEM image of fragments generated from dynamic loading of bone surrogate in longitudinal orientation (transverse is similar). A large number of pores exist along the interface of the bone and adhesive, leading to failure from crack growth

Full size image

The two-parameter power-law fit of the distribution shows a decrease in the size parameter a at higher strain-rates. The decrease is due to the resulting fragments being smaller at higher compressive loads, likely from the activation and initiation of more flaw sites within the material. The absolute value of the parameter b also decreased at higher strain-rates for similar reasons, providing a better shape-fit of the distribution. These parameters are expected to be refined and updated as more experimental data is made available for varying strain-rates and materials such as other bone surrogates or natural bone under different conditioning (specimen age, for instance). This work demonstrates the applicability of Grady’s power-law to describe the fragmentation behavior beyond classical brittle materials such as structural ceramics and glass, and is shown to capture the distribution of both dry ox cortical bone and a bone surrogate material.

Conclusion

This study investigated the loading rate and orientation dependence on compressive strength, and resulting dynamic fragmentation behavior of dry ox cortical bone and a bone surrogate material. Uniaxial compressive tests were conducted at quasi-static (10−3 s−1) and dynamic (103 s−1) strain-rates, loading in the longitudinal and transverse material orientations (with respect to the die-press direction of the bone surrogate and osteon of the cortical bone). The bone surrogate was found to have a rate dependency, but not a statistically significant orientation dependency on compressive strength; whereas the dry ox bone exhibited both rate and orientation dependence. The bone surrogate had a maximum compressive strength of around 83 MPa (±9 MPa) under quasi-static loading, increasing to 102 MPa (±7 MPa) under dynamic loading, regardless of orientation. Under quasi-static loading, the dry ox bone had a maximum compressive strength of 336 MPa (±6 MPa) in the longitudinal orientation, and 244 MPa (±22 MPa) in the transverse. These strengths increased by over 50% under dynamic loading to 559 MPa (±23 MPa) in the longitudinal orientation and 350 MPa (±34 MPa) in the transverse. Dynamic fragmentation of ox bone produced fragments with a mean size of 266 ± 28 μm in the longitudinal orientation, and 410 ± 19 μm in transverse; whereas the bone surrogate produced larger fragments with mean sizes of 431 ± 14 μm in longitudinal and 694 ± 25 μm in transverse.

Microstructural characterization revealed that the bone surrogate generated fragments which fell into two size regimes, a larger resulting from structural failure along the binder and bone interface, and a smaller resulting from Poisson driven effects due to further comminution of the fragments. The dry cortical bone produced a uniform distribution of fragment sizes on the order microstructure features of the osteons and the underlying canal system, whereas the bone surrogate contained intrinsic flaws in a nominally homogeneous microstructure. Both the dry cortical bone and bone surrogate exhibited fragment size distributions with a power-law dependence on length. The findings of this study provide insight on understanding and predicting dynamic fragmentation of cortical bone, and can aid in development of next generation bone surrogate materials.