A Modified Method for a Cauchy Problem of the Helmholtz Equation

Abstract

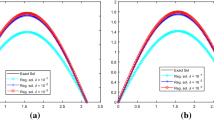
In this paper, a Cauchy problem for the Helmholtz equation is investigated. It is well known that this problem is severely ill-posed in the sense that the solution (if it exists) does not depend continuously on the given Cauchy data. To overcome such difficulties, we propose a modified regularization method to approximate the solution of this problem, and then analyze the stability and convergence of the proposed regularization method based on the conditional stability estimates. Finally, we present two numerical examples to illustrate that the proposed regularization method works well.

Appendices

Appendix 1

Proof to Lemma 2.2

The proof is inspired by the idea of [13]. The condition $\left\| v(\cdot ,1)\right\| \le E$ leads to

$$\begin{aligned} \frac{\pi }{2}\sum _{n=1}^{\infty }b_n^2\cosh ^2\left( \sqrt{n^2-k^2}\right) \le E^2. \end{aligned}$$

(7.1)

The condition $\left\| \phi \right\| \le \varepsilon$ means

$$\begin{aligned} \frac{\pi }{2}\sum _{n=1}^{\infty }b_n^2 \le \varepsilon ^2.\quad \end{aligned}$$

(7.2)

Now define a function $\tau _1(y)$ as

$$\begin{aligned} \tau _1(y)= \left\{ \begin{aligned}&\cos \left( \sqrt{k^2-n^2}y\right) ,\quad 1\le n<k,\\&\cosh \left( \sqrt{n^2-k^2}y\right) ,\quad [k]+1\le n<N_1,\\&m_1(y),\quad n\ge N_1,\\ \end{aligned} \right. \end{aligned}$$

where $N_1=\min \left\{ n|\cosh \left( \sqrt{n^2-k^2}y\right) \ge m_1(y), n>k\right\}$ and $m_1(y)=(1-y)(2E)^y\varepsilon ^{-y}$. Denote $\xi =\sqrt{n^2-k^2}$ for $n>k$. From (2.3), we have

$$\begin{aligned} v(x,y)= & {} \sum _{n=1}^\infty b_n \sin (n x) \cosh (\xi y)-\sum _{n=1}^\infty b_n \sin (n x) \tau _1(y)+\sum _{n=1}^\infty b_n \sin (n x) \tau _1(y)\\= & {} \sum _{n=N_1}^\infty b_n\sin (n x) \left( \cosh (\xi y)-m_1(y)\right) +\sum _{n=1}^\infty b_n \sin (n x) \tau _1(y). \end{aligned}$$

Further, from (7.1) and (7.2), we have

$$\begin{aligned} \left\| v(\cdot ,y) \right\|\le & {} \left( \frac{\pi }{2}\sum _{n=N_1}^\infty b_n^2 \cosh ^2(\xi )\left( \frac{\cosh (\xi y)-m_1(y)}{\cosh (\xi )}\right) ^2\right) ^{\frac{1}{2}}+\left( \frac{\pi }{2}\sum _{n=1}^\infty b_n^2\tau _1^2( y)\right) ^{\frac{1}{2}}\\\le & {} E\sup _{n\ge N_1}A_1(n) + \varepsilon \sup _{n\ge 1} \left| \tau _1(y)\right| , \end{aligned}$$

where

$$\begin{aligned} A_1(n)=\left| \frac{\cosh (\xi y)-m_1(y)}{\cosh (\xi )}\right| . \end{aligned}$$

In the following, we estimate $A_1(n)$.

$$\begin{aligned} A_1(n)=\frac{\mathrm{{e}}^{\xi y}+\mathrm{{e}}^{-\xi y}-2m_1(y)}{\mathrm{{e}}^\xi +\mathrm{{e}}^{-\xi }}\le \frac{2\left( \mathrm{{e}}^{\xi y}-m_1(y)\right) }{\mathrm{{e}}^\xi }. \end{aligned}$$

Note that $F(\xi )=\frac{\mathrm{{e}}^{\xi y}-m_1(y)}{\mathrm{{e}}^\xi }$ over $\xi >0$ attains its maximum $F_{\mathrm{{max}}}=y(1-y)^{\frac{1}{y}-1}\left( m_1(y)\right) ^{-\left( \frac{1}{y}-1\right) }$, then we have

$$\begin{aligned} A_1(n)\le 2y(1-y)^{\frac{1}{y}-1}\left( m_1(y)\right) ^{-\left( \frac{1}{y}-1\right) }. \end{aligned}$$

Meanwhile, note that $\left| \tau _1(y)\right| \le m_1(y)$ for $n>k$, thus we have

$$\begin{aligned} \left\| v(\cdot ,y) \right\| \le 2Ey(1-y)^{\frac{1}{y}-1}\left( m_1(y)\right) ^{-\left( \frac{1}{y}-1\right) }+\varepsilon m_1(y). \end{aligned}$$

(7.3)

It is easy to check that the function $F_1(m_1)=2Ey(1-y)^{\frac{1}{y}-1}\left( m_1(y)\right) ^{-\left( \frac{1}{y}-1\right) }+\varepsilon m_1(y)$ reaches its minimum at $m_1(y)=(1-y)(2E)^y\varepsilon ^{-y}$. Hence, by (7.3), we have

$$\begin{aligned} \left\| v(\cdot ,y)\right\| \le \left( 2E\right) ^y\varepsilon ^{1-y}\le 2E^y\varepsilon ^{1-y}. \end{aligned}$$

$\square$

Appendix 2

Proof to Lemma 2.3

The condition $\left\| w(\cdot ,1)\right\| \le E$ is equivalent to

$$\begin{aligned} \frac{\pi }{2}\sum _{n=1}^{\infty }c_n^2\frac{\sinh ^2 \left( \sqrt{n^2-k^2}\right) }{\left( \sqrt{n^2-k^2}\right) ^2}\le E^2. \end{aligned}$$

(8.1)

The condition $\left\| \psi \right\| \le \varepsilon$ means

$$\begin{aligned} \frac{\pi }{2}\sum _{n=1}^{\infty }c_n^2 \le \varepsilon ^2.\quad \end{aligned}$$

(8.2)

Define the following function

$$\begin{aligned} \tau _2(y)= \left\{ \begin{aligned}&\frac{\sin \left( \sqrt{k^2-n^2}y\right) }{\sqrt{k^2-n^2}},\quad 1\le n<k,\\&\frac{\sinh \left( \sqrt{n^2-k^2}y\right) }{\sqrt{n^2-k^2}},\quad [k]+1\le n<N_2,\\&m_2(y),\quad n\ge N_2,\\ \end{aligned} \right. \end{aligned}$$

where $N_2\!=\!\min \left\{ n\mid \frac{\sinh \left( \sqrt{n^2-k^2}y\right) }{\sqrt{n^2-k^2}} \ge m_2(y),n\!>\!k \right\}$, note that $g(n)=\frac{\sinh \left( \sqrt{n^2-k^2}y\right) }{\sqrt{n^2-k^2}}$ is strictly monotonically increasing for $n>k$, and $m_2(y)=\left( 1-\mathrm{{e}}^{-2\rho }\right) ^{-y}(2\rho )^{-(1-y)}(1-y)E^y\varepsilon ^{-y}$, where $\rho =\sqrt{\left( [k]+1\right) ^2-k^2}$. Taking the similar procedure of estimating $\left\| v(\cdot ,y)\right\|$, from (2.4), (8.1) and (8.2), note that $\left| \tau _2(y)\right| \le m_2(y)$ for $n>k$, we have

$$\begin{aligned} \left\| w(\cdot ,y)\right\|\le & {} \left( \frac{\pi }{2}\sum _{n=N_2}^\infty c_n^2 \frac{\sinh ^2(\xi )}{\xi ^2}\left( \frac{\sinh (\xi y)-\xi m_2(y)}{\sinh (\xi )}\right) ^2\right) ^{\frac{1}{2}}\!+\left( \frac{\pi }{2}\sum _{n=1}^\infty c_n^2\tau _2^2( y)\right) ^{\frac{1}{2}}\\\le & {} E\sup _{n\ge N_2}A_2(n) + \varepsilon m_2(y), \end{aligned}$$

where

$$\begin{aligned} A_2(n)=\left| \frac{\sinh (\xi y)-\xi m_2(y)}{\sinh (\xi )}\right| . \end{aligned}$$

Now we firstly estimate $A_2(n)$.

$$\begin{aligned} A_2(n)=\frac{\mathrm{{e}}^{\xi y}-\mathrm{{e}}^{-\xi y}-2\xi m_2(y)}{\mathrm{{e}}^\xi -\mathrm{{e}}^{-\xi }}\le \frac{\mathrm{{e}}^{\xi y}-2\rho m_2(y)}{\mathrm{{e}}^\xi (1-\mathrm{{e}}^{-2\rho })}. \end{aligned}$$

By a simple calculation, we can obtain that $G(\xi )=\frac{\mathrm{{e}}^{\xi y}-2\rho m_2(y)}{\mathrm{{e}}^\xi }$ over $\xi >0$ attains its maximum $G_{\max }=(2\rho )^{-(\frac{1}{y}-1)} y(1-y)^{\frac{1}{y}-1}\left( m_2(y)\right) ^{-\left( \frac{1}{y}-1\right) }$. Then, we have

$$\begin{aligned} \left\| w(\cdot ,y)\right\| \le \left( 1-\mathrm{{e}}^{-2\rho }\right) ^{-1}(2\rho )^{-(\frac{1}{y}-1)}E y(1-y)^{\frac{1}{y}-1}\left( m_2(y)\right) ^{-\left( \frac{1}{y}-1\right) }+\varepsilon m_2(y). \end{aligned}$$

(8.3)

It is easy to check that the function $F_2(m_2)=\left( 1-\mathrm{{e}}^{-2\rho }\right) ^{-1}(2\rho )^{-(\frac{1}{y}-1)}Ey(1-y)^{\frac{1}{y}-1}\left( m_2(y)\right) ^{-\left( \frac{1}{y}-1\right) }+\varepsilon m_2(y)$ reaches its minimum at $m_2(y)=\left( 1-\mathrm{{e}}^{-2\rho }\right) ^{-y}(1-y)(2\rho )^{-(1-y)}E^y\varepsilon ^{-y}$. Hence, using the inequality $1-\mathrm{{e}}^{-r}\le r$ for $r \ge 0$, we obtain

$$\begin{aligned} \left\| w(\cdot ,y) \right\| \le \left( 1-e^{-2\rho }\right) ^{-y}(2\rho )^{-(1-y)}E^y\varepsilon ^{1-y}\le \left( 1-e^{-2\rho }\right) ^{-1}E^y\varepsilon ^{1-y}. \end{aligned}$$

$\square$

Appendix 3

Proof to Theorem 2.5

In the following, we firstly estimate $\Vert v(\cdot , 1)\Vert$, whose proof is inspired by the idea of paper [22]. By (2.3), the condition $\left\| \frac{\partial ^p v(\cdot ,1)}{\partial y^p} \right\| \le E_p$ leads to

$$\begin{aligned} \left\{ \begin{aligned}&\frac{\pi }{2}\sum _{n=1}^{\infty }b_n^2\left( \sqrt{n^2-k^2}\right) ^{2p}\sinh ^2\left( \sqrt{n^2-k^2}\right) \le E_p^2,\quad p \; \text{ is } \text{ odd },\\&\frac{\pi }{2}\sum _{n=1}^{\infty }b_n^2\left( \sqrt{n^2-k^2}\right) ^{2p}\cosh ^2\left( \sqrt{n^2-k^2}\right) \le E_p^2,\quad p \; \text{ is } \text{ even }. \end{aligned} \right. \end{aligned}$$

(9.1)

Denote $\zeta =\sqrt{k^2-n^2}$ for $n \le k$, $\xi =\sqrt{n^2-k^2}$ for $n>k$, $\nu =\left( \ln \left( \frac{E_p}{\varepsilon } \left( \ln \frac{E_p}{\varepsilon }\right) ^{-p}\right) \right) ^{-1}$ and ${\gamma }=\frac{\xi }{\sqrt{1+\nu ^2n^2}}$. Note that $\frac{\cosh (\xi )}{\sinh (\xi )}=\frac{e^\xi +e^{-\xi }}{e^\xi \left( 1-e^{-2\xi }\right) }\le \frac{2}{1-\mathrm{{e}}^{-2\rho }}$, where $\rho =\sqrt{\left( [k]+1\right) ^2-k^2}$.

For the case of $k\ge 1$, from (2.3), (7.2) and (9.1), we have

$$\begin{aligned} \left\| v(\cdot ,1) \right\|\le & {} \left( \frac{\pi }{2}\sum _{n=1}^{[k]}b_n^2\cos ^2\left( \zeta \right) \right) ^{\frac{1}{2}}+ \left( \frac{\pi }{2}\sum _{n=[k]+1}^{\infty }b_n^2\left( \cosh (\xi )-\cosh (\gamma )\right) ^2\right) ^{\frac{1}{2}}\\&+\left( \frac{\pi }{2}\sum _{n=[k]+1}^{\infty }b_n^2 \cosh ^2(\gamma )\right) ^{\frac{1}{2}}\nonumber \\\le & {} \varepsilon +\frac{2E_p}{1-\mathrm{{e}}^{-2\rho }}\sup \limits _{n\ge [k]+1}B(n)+\varepsilon \sup \limits _{n\ge [k]+1} D(n), \end{aligned}$$

where

$$\begin{aligned} B(n)=\left| \frac{\cosh (\xi )-\cosh (\gamma )}{\xi ^p\cosh (\xi )}\right| , \quad D(n)=\left| \cosh (\gamma )\right| . \end{aligned}$$

For D(n), we have $D(n) \le \mathrm{{e}}^{\gamma }\le \mathrm{{e}}^{\frac{1}{\nu }}$. In the following, we estimate B(n). Note that $\xi > \gamma$, we have

$$\begin{aligned} B(n) = \frac{(\mathrm{{e}}^{\xi } - \mathrm{{e}}^{\gamma })-(\mathrm{{e}}^{\xi } - \mathrm{{e}}^{\gamma })/\mathrm{{e}}^{(\xi + \gamma )}}{(\mathrm{{e}}^\xi + \mathrm{{e}}^{-\xi })\xi ^{p}} \le \frac{\mathrm{{e}}^{\xi }-\mathrm{{e}}^{\gamma }}{\mathrm{{e}}^{\xi }\xi ^{p}}=\left( 1-\mathrm{{e}}^{-(\xi -\gamma )}\right) \xi ^{-p}. \end{aligned}$$

Case 1 For large values of n with $\xi =\sqrt{n^2 -k^2} \ge \nu ^{-\frac{2}{3}}$, we have

$$\begin{aligned} B(n)\le \xi ^{-p} \le \nu ^{\frac{2p}{3}}. \end{aligned}$$

Case 2 For $\xi < \nu ^{-\frac{2}{3}}$, note that $\xi -\gamma \le \frac{1}{2} \nu ^2 n^2\xi$ and $1-\mathrm{{e}}^{-r}\le r$ for $r\ge 0$ , we have

$$\begin{aligned} B(n) \le (\xi -\gamma )\xi ^{-p}\le \frac{1}{2}\nu ^2n^2\xi ^{1-p} = \frac{1}{2}\nu ^2\xi ^{3-p} + \frac{1}{2}\nu ^2 k^2 \xi ^{1-p}, \end{aligned}$$

(9.2)

• (1) For $n=[k]+1$, by (9.2), $B(n) \le \frac{1}{2}\nu ^2([k]+1)^2\rho ^{1-p}$;

• (2) For $n >[k]+1$, by (9.2), note that $\xi >1$,

• (a) If $0< p <3$, $B(n) \le \frac{1}{2}(1+k^2)\nu ^2 \xi ^{3-p} \le \frac{1}{2}(1+k^2)\nu ^{\frac{2p}{3}}$;

• (b) If $p \ge 3$, $B(n) \le \frac{1}{2}(1+k^2)\nu ^2$.

Hence, by cases 1 and 2, we obtain that

$$\begin{aligned} B(n) \le \max {\left\{ \nu ^{\frac{2p}{3}}, \frac{1}{2}\nu ^2([k]+1)^2\rho ^{1-p}, \frac{1}{2}(1+k^2)\nu ^{\frac{2p}{3}},\frac{1}{2}(1+k^2)\nu ^2 \right\} }. \end{aligned}$$

(9.3)

Now combining the above discussion, we have

$$\begin{aligned} \left\| v(\cdot ,1) \right\|\le & {} \varepsilon +\frac{E_p}{1-\mathrm{{e}}^{-2\rho }}\max {\left\{ 2\nu ^{\frac{2p}{3}}, \nu ^2([k]+1)^2\rho ^{1-p}, (1+k^2)\nu ^{\frac{2p}{3}},(1+k^2)\nu ^2 \right\} }\nonumber \\&+ \frac{E_p}{\left( \ln {\frac{E_p}{\varepsilon }}\right) ^{p}}. \end{aligned}$$

(9.4)

For the case of $0 < k < 1$, the first term in the right-hand side of Eq. (9.4) can be vanished.

Now we estimate $\Vert w(\cdot , 1)\Vert$. By (2.4), the condition $\left\| \frac{\partial ^p w(\cdot ,1)}{\partial y^p} \right\| \le E_p$ leads to

$$\begin{aligned} \left\{ \begin{aligned}&\frac{\pi }{2}\sum _{n=1}^{\infty }c_n^2\left( \sqrt{n^2-k^2}\right) ^{2p}\frac{\cosh ^2\left( \sqrt{n^2-k^2}\right) }{\left( \sqrt{n^2-k^2}\right) ^2}\le E_p^2,\quad p \; \text{ is } \text{ odd },\\&\frac{\pi }{2}\sum _{n=1}^{\infty }c_n^2\left( \sqrt{n^2-k^2}\right) ^{2p}\frac{\sinh ^2\left( \sqrt{n^2-k^2}\right) }{\left( \sqrt{n^2-k^2}\right) ^2}\le E_p^2,\quad p \; \text{ is } \text{ even }. \end{aligned} \right. \end{aligned}$$

(9.5)

Taking the similar procedure of estimating $\left\| v(\cdot ,1)\right\|$, when $k\ge 1$, recalling that $\gamma =\xi /\sqrt{1+\nu ^2n^2}$, we have

$$\begin{aligned} \left\| w(\cdot ,1) \right\|\le & {} \left( \frac{\pi }{2}\sum _{n=1}^{[k]}c_n^2\frac{\sin ^2\left( \zeta \right) }{\zeta ^2}\right) ^{\frac{1}{2}}+\left( \frac{\pi }{2}\sum _{n=[k]+1}^{\infty }c_n^2\left( \frac{\sinh (\xi )}{\xi }-\frac{\sinh (\gamma )}{\gamma }\right) ^2\right) ^{\frac{1}{2}}\nonumber \\&+\left( \frac{\pi }{2}\sum _{n=[k]+1}^{\infty }c_n^2\left( \frac{\sinh (\gamma )}{\gamma }\right) ^2\right) ^{\frac{1}{2}}\nonumber \\\le & {} \varepsilon +E_p\sup \limits _{n\ge [k]+1}B_1(n)+\varepsilon \sup \limits _{n\ge [k]+1}D_1(n), \end{aligned}$$

(9.6)

where

$$\begin{aligned} B_1(n)= \left| \frac{\sinh (\xi )-\sqrt{1+\nu ^2n^2}\sinh (\gamma )}{\xi ^p\sinh (\xi )}\right| ,\quad D_1(n)=\left| \frac{\sinh (\gamma )}{\gamma }\right| . \end{aligned}$$

Recalling that $1-\mathrm{{e}}^{-r}\le r$ for $r\ge 0$, we have

$$\begin{aligned} D_1(n)=\frac{\mathrm{{e}}^\gamma (1-\mathrm{{e}}^{-2\gamma })}{2\gamma }\le \mathrm{{e}}^\gamma \le \mathrm{{e}}^{\frac{1}{\nu }}. \end{aligned}$$

(9.7)

In the following, we estimate $B_1(n)$.

Case 1 For large values of n with $\xi =\sqrt{n^2 -k^2} \ge \nu ^{-\frac{2}{3}}$, using Taylor expansion $\sinh (s)=\sum _{n=1}^{\infty }\frac{s^{2n-1}}{(2n-1)!}$, recalling that $\gamma =\xi /\sqrt{1+\nu ^2n^2}$, we get $\sqrt{1+\nu ^2n^2}\frac{\sinh (\gamma )}{\sinh (\xi )}<1$, hence

$$\begin{aligned} B_1(n)=\xi ^{-p}\left( 1-\sqrt{1+\nu ^2n^2}\frac{\sinh (\gamma )}{\sinh (\xi )}\right) \le \xi ^{-p}\le \nu ^{\frac{2}{3}p}. \end{aligned}$$

(9.8)

Case 2 For $\xi <\nu ^{-\frac{2}{3}}$, we have

$$\begin{aligned} B_1(n)\le \xi ^{-p}\left( C_1(n)+C_2(n)\right) , \end{aligned}$$

(9.9)

where $C_1(n)=\left| \frac{\sinh (\xi )-\sinh (\gamma )}{\sinh (\xi )}\right|$ and $C_2(n)=\left| \frac{\sinh (\gamma )-\sqrt{1+\nu ^2n^2}\sinh (\gamma )}{\sinh (\xi )}\right|$. Now let us estimate $C_1(n)$. Recalling that $\xi -\gamma \le \frac{1}{2}\nu ^2n^2\xi$, we have

$$\begin{aligned} C_1(n)= & {} \frac{\mathrm{{e}}^\xi -\mathrm{{e}}^\gamma -\left( \mathrm{{e}}^{-\xi }-\mathrm{{e}}^{-\gamma } \right) }{\mathrm{{e}}^\xi \left( 1-\mathrm{{e}}^{-2\xi }\right) }\le \frac{ \left( \mathrm{{e}}^\xi -\mathrm{{e}}^\gamma \right) \left( 1+\mathrm{{e}}^{-(\xi +\gamma )}\right) }{\mathrm{{e}}^\xi (1-\mathrm{{e}}^{-2\rho })}\le \frac{2\left( 1-\mathrm{{e}}^{-(\xi -\gamma )}\right) }{1-\mathrm{{e}}^{-2\rho }}\nonumber \\\le & {} 2\left( 1-\mathrm{{e}}^{-2\rho }\right) ^{-1}(\xi -\gamma )\le \left( 1-\mathrm{{e}}^{-2\rho }\right) ^{-1}\xi n^2\nu ^2. \end{aligned}$$

(9.10)

Next we estimate $C_2(n)$. Recalling that $\sqrt{1+\nu ^2n^2}\le 1+\frac{1}{2}\nu ^2n^2$, and by (9.7), we have

$$\begin{aligned} C_2(n)=\frac{\left( \sqrt{1+\nu ^2n^2}-1\right) \gamma \sinh {(\gamma )}/\gamma }{\mathrm{{e}}^\xi (1-\mathrm{{e}}^{-2\xi })/2}\le \frac{\nu ^2n^2\xi \mathrm{{e}}^\gamma }{\mathrm{{e}}^\xi (1-\mathrm{{e}}^{-2\rho })}\le (1-\mathrm{{e}}^{-2\rho })^{-1}\xi n^2\nu ^2. \end{aligned}$$

(9.11)

Combining (9.9)–(9.11), by (9.2), for $\xi <\nu ^{-\frac{2}{3}}$, we obtain

$$\begin{aligned} B_1(n)\le & {} 2(1-\mathrm{{e}}^{-2\rho })^{-1}\xi ^{1-p} n^2\nu ^2\nonumber \\\le & {} 4(1-\mathrm{{e}}^{-2\rho })^{-1}\max {\left\{ \frac{1}{2}\nu ^2([k]+1)^2\rho ^{1-p}, \frac{1}{2}(1+k^2)\nu ^{\frac{2p}{3}},\frac{1}{2}(1+k^2)\nu ^2 \right\} }.\nonumber \\ \end{aligned}$$

(9.12)

Further, from (9.8) and (9.12), we obtain

$$\begin{aligned} B_1(n)\!\le \!4(1-\mathrm{{e}}^{-2\rho })^{-1}\max {\left\{ \nu ^{\frac{2p}{3}}, \frac{1}{2}\nu ^2([k]\,{+}\,1)^2\rho ^{1-p}, \frac{1}{2}(1\,{+}\,k^2)\nu ^{\frac{2p}{3}},\frac{1}{2}(1\,{+}\,k^2)\nu ^2 \right\} }. \end{aligned}$$

(9.13)

Hence, from (9.6)–(9.7) and (9.13), we have

$$\begin{aligned} \left\| w(\cdot ,1) \right\|\le & {} \varepsilon \!+\!\frac{2E_p}{1-\mathrm{{e}}^{-2\rho }}\max {\left\{ 2\nu ^{\frac{2p}{3}}, \nu ^2([k]+1)^2\rho ^{1-p}, (1+k^2)\nu ^{\frac{2p}{3}},(1+k^2)\nu ^2 \right\} }\nonumber \\&+ \, \frac{E_p}{\left( \ln {\frac{E_p}{\varepsilon }}\right) ^{p}}. \end{aligned}$$

(9.14)

For the case of $0 < k < 1$, the first term in the right-hand side of Eq. (9.14) can be vanished.

Finally, using the triangle inequality $\left\| u(\cdot ,1)\right\| \le \left\| v(\cdot ,1)\right\| +\left\| w(\cdot ,1)\right\|$, by (9.4) and (9.14), the stability result (2.11) can directly be obtained. $\square$

Appendix 4

Theorem 9.1

Let $u=v+w$ be the exact solution of problem (1.1) with the exact data $\left( \phi , \psi \right)$ and $u_\alpha ^\delta =v_\alpha ^\delta +w_\alpha ^\delta$ be the regularized solution with the filter function q given by (4.1). Further, let the measured data $\phi ^\delta$, $\psi ^\delta$ satisfy (1.2) and assume that the a-priori bound assumption (2.10) holds. Choose the regularization parameter $\alpha$ as

$$\begin{aligned} \alpha =\left( \frac{\delta }{E_p}\right) ^{4/5}. \end{aligned}$$

(10.1)

Then, at $y=1$, for $k>0$, we have

$$\begin{aligned}&\left\| u_\alpha ^\delta (\cdot ,1)-u(\cdot ,1) \right\| \nonumber \\&\quad \le 2\left( \delta + \delta ^{\frac{1}{5}}E_p^{\frac{4}{5}} + \frac{2E_p}{1-\mathrm{{e}}^{-2\rho }}\max \left\{ \rho ^{-p} \left( \frac{\delta }{E_p}\right) ^{\frac{8}{15}}, 2\left( \frac{4}{15}\ln \frac{E_p}{\delta }\right) ^{-p}\right\} \right) ,\nonumber \\ \end{aligned}$$

(10.2)

where $\rho =\sqrt{([k]+1)^2-k^2}$.

Proof

From the proof of Theorem 3.2 in [27], we have

$$\begin{aligned} \left\| v_\alpha ^\delta (\cdot ,1)-v(\cdot ,1)\right\| \le \delta +\frac{\delta }{\alpha }+\frac{2E_p}{1-\mathrm{{e}}^{-2\rho }}\max \left\{ \rho ^{-p} \alpha ^{\frac{2}{3}}, 2\left( \ln \frac{1}{\root 3 \of {\alpha }}\right) ^{-p}\right\} . \end{aligned}$$

For $n>k$, note that $\xi =\sqrt{n^2-k^2}>0$ and $\sinh {(\xi )}/\xi \le \cosh {(\xi )}$, then similar to the proof of Theorem 3.2 in [27], we have

$$\begin{aligned} \left\| w_\alpha ^\delta (\cdot ,1)-w(\cdot ,1)\right\| \le \delta +\frac{\delta }{\alpha }+\frac{2E_p}{1-\mathrm{{e}}^{-2\rho }}\max \left\{ \rho ^{-p} \alpha ^{\frac{2}{3}}, 2\left( \ln \frac{1}{\root 3 \of {\alpha }}\right) ^{-p}\right\} . \end{aligned}$$

Further, by (10.1), using the triangle inequality $\left\| u_\alpha ^\delta (\cdot ,1)-u(\cdot ,1)\right\| \le \left\| v_\alpha ^\delta (\cdot ,1)-\right. \left. v(\cdot ,1)\right\| + \left\| w_\alpha ^\delta (\cdot ,1)-w(\cdot ,1)\right\|$, the conclusion (10.2) can be obtained. $\square$