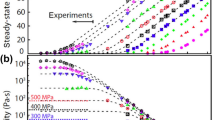
Abstract
Nonequilibrium molecular dynamics (NEMD) simulations have provided unique insights into the nanoscale behaviour of lubricants under shear. This review discusses the early history of NEMD and its progression from a tool to corroborate theories of the liquid state, to an instrument that can directly evaluate important fluid properties, towards a potential design tool in tribology. The key methodological advances which have allowed this evolution are also highlighted. This is followed by a summary of bulk and confined NEMD simulations of liquid lubricants and lubricant additives, as they have progressed from simple atomic fluids to ever more complex, realistic molecules. The future outlook of NEMD in tribology, including the inclusion of chemical reactivity for additives, and coupling to continuum methods for large systems, is also briefly discussed.
Article PDF
Similar content being viewed by others
Avoid common mistakes on your manuscript.
References
Holmberg K, Erdemir A. Influence of tribology on global energy consumption, costs and emissions. Friction 5(3): 263–284 (2017)
Urbakh M, Klafter J, Gourdon D, Israelachvili J. The nonlinear nature of friction. Nature 430(6999): 525–528 (2004)
Aghababaei R, Warner D H, Molinari J F. Critical length scale controls adhesive wear mechanisms. Nat Commun 7: 11816 (2016)
Allen M P, Tidesley D J. Computer Simulation of Liquids. Oxford (UK): Clarendon Press, 1989.
Metropolis N, Ulam S. The Monte Carlo method. J Am Stat Assoc 44(247): 335–341 (1949)
Goldstein M. Viscous liquids and the glass transition: A potential energy barrier picture. J Chem Phys 51(9): 3728–3739 (1969)
Levesque D, Verlet L, Kürkijarvi J. Computer ”Experiments” on classical fluids. IV. Transport properties and timecorrelation functions of the Lennard-Jones liquid near its triple point. Phys Rev A 7(5): 1690–1700 (1973)
Siu S W I, Pluhackova K, Böckmann R A. Optimization of the OPLS-AA force field for long hydrocarbons. J Chem Theory Comput 8(4): 1459–1470 (2012)
Murzyn K, Bratek M, Pasenkiewicz-Gierula M. Refined OPLS all-atom force field parameters for n-pentadecane, methyl acetate, and dimethyl phosphate. J Phys Chem B 117(51): 16388–16396 (2013)
Ewen J P, Gattinoni C, Thakkar F M, Morgan N, Spikes H, Dini D. A comparison of classical force-fields for molecular dynamics simulations of lubricants. Materials 9(8): 651 (2016)
Szlufarska I, Chandross M, Carpick R W. Recent advances in single-asperity nanotribology. J Phys D Appl Phys 41(12): 123001 (2008)
Vanossi A, Manini N, Urbakh M, Zapperi S, Tosatti E. Colloquium. Modeling friction: From nanoscale to mesoscale. Rev Mod Phys 85(2): 529–552 (2013)
Dong Y L, Li Q Y, Martini A. Molecular dynamics simulation of atomic friction: A review and guide. J Vac Sci Technol A, 31(3): 030801 (2013)
Sawyer W G, Argibay N, Burris D L, Krick B A. Mechanistic studies in friction and wear of bulk materials. Annu Rev Mater Res 44: 395–427 (2014)
Alder B J, Wainwright T E. Phase transition for a hard sphere system. J Chem Phys 27(5): 1208–1209 (1957)
Frenkel D. Entropy-driven phase transitions. Phys. A 263(1–4): 26–38 (1999)
Rahman A. Correlations in the motion of atoms in liquid argon. Phys Rev 136(2A): 405–411 (1964)
Eyring H, Ree T. Significant liquid structures, VI. The vacancy theory of liquids. Proc Natl Acad Sci USA 47(4): 526–537 (1961)
Ediger M D. Spatially heterogeneous dynamics in supercooled liquids. Annu Rev Phys Chem 51: 99–128 (2000)
Edward J T. Molecular volumes and the Stokes-Einstein equation. J Chem Educ 47(4): 261 (1970)
Hansen J P, McDonald I R. Theory of Simple Liquids. 4th ed. Amsterdam (Netherlands): Academic Press, 2013.
Alder B J, Gass D M, Wainwright T E. Studies in molecular dynamics. VIII. The transport coefficients for a hard-sphere fluid. J Chem Phys 53(10): 3813–3826 (1970)
Hefland E. Transport coefficients from dissipation in a canonical ensemble. Phys Rev 119(1): 1–9 (1960)
Green M S. Markoff random processes and the statistical mechanics of time-dependent phenomena. II. Irreversible processes in fluids. J Chem Phys 22(3): 398–413 (1954)
Kubo R. Statistical-mechanical theory of irreversible processes. I. General theory and simple applications to magnetic and conduction problems. J Phys Soc Jpn 12(6): 570–586 (1957)
Ladd A J C, Alley W E, Alder B J. Structural relaxation in dense hard-sphere fluids. J Stat Phys 48(5–6): 1147–1156 (1987)
Ashurst W T, Hoover W G. Dense-fluid shear viscosity via nonequilibrium molecular dynamics. Phys Rev A 11(2): 658–678 (1975)
Bitsanis I, Magda J J, Tirrell M, Davis H T. Molecular dynamics of flow in micropores. J Chem Phys 87(3): 1733–1750 (1987)
Ewen J P, Gattinoni C, Zhang J, Heyes D M, Spikes H A, Dini D. On the effect of confined fluid molecular structure on nonequilibrium phase behaviour and friction. Phys Chem Chem Phys 19(27): 17883–17894 (2017)
Evans D J, Morriss G P. Nonlinear-response theory for steady planar Couette flow. Phys Rev A 30(3): 1528–1530 (1984)
Lees A W, Edwards S F. The computer study of transport processes under extreme conditions. J Phys C Solid State Phys 5(15): 1921–1928 (1972)
Evans D J, Morriss G P. Statistical Mechanics of Nonequilibrium Liquids. 2nd ed. Cambridge (UK): Cambridge University Press, 2008.
Todd B D, Daivis P J. Nonequilibrium Molecular Dynamics: Theory, Algorithms and Applications. Cambridge (UK): Cambridge University Press, 2017.
Heyes D M, Kim J J, Montrose C J, Litovitz T A. Time dependent nonlinear shear stress effects in simple liquids: A molecular dynamics study. J Chem Phys 73(8): 3987–3996 (1980)
Maginn E J, Elliott J R. Historical perspective and current outlook for molecular dynamics as a chemical engineering tool. Ind Eng Chem Res 49(7): 3059–3078 (2010)
Götz A W, Williamson M J, Xu D, Poole D, Le Grand S, Walker R C. Routine microsecond molecular dynamics simulations with AMBER on GPUs. 1. Generalized born. J Chem Theory Comput 8(5): 1542–1555 (2012)
Bair S, McCabe C, Cummings P T. Comparison of nonequilibrium molecular dynamics with experimental measurements in the nonlinear shear-thinning regime. Phys Rev Lett 88(5): 058302 (2002)
Bair S, McCabe C, Cummings P T. Calculation of viscous EHL traction for squalane using molecular simulation and rheometry. Tribol Lett 13(4): 251–254 (2002)
Morriss G P, Evans D J. Application of transient correlation functions to shear flow far from equilibrium. Phys Rev A 35(2): 792–797 (1987)
Evans D J, Morriss G P. Transient-time-correlation functions and the rheology of fluids. Phys Rev A 38(8): 4142–4148 (1988)
Spikes H, Zhang J. History, origins and prediction of elastohydrodynamic friction. Tribol Lett 56(1): 1–25 (2014)
Taylor R, de Kraker B R. Shear rates in engines and implications for lubricant design. Proc Inst Mech Eng Part J J Eng Tribol 231(9): 1106–1116 (2017)
Andersen H C. Molecular dynamics simulations at constant pressure and/or temperature. J Chem Phys 72(4): 2384–2393 (1980)
Berendsen H J C, Postma J P M, Van Gunsteren W F, DiNola A, Haak J R. Molecular dynamics with coupling to an external bath. J Chem Phys 81(8): 3684–3690 (1984)
Schneider T, Stoll E. Molecular-dynamics study of a three-dimensional one-component model for distortive phase transitions. Phys Rev B 17(3): 1302–1322 (1978)
Español P, Warren P. Statistical mechanics of dissipative particle dynamics. Eur Lett 30(4): 191–196 (1995)
Nosé S. A molecular dynamics method for simulations in the canonical ensemble. Mol Phys 52(2): 255–268 (1984)
Hoover W G. Canonical dynamics: Equilibrium phase-space distributions. Phys Rev A 31(3): 1695–1697 (1985)
Mundy C J, Siepmann J I, Klein M L. Decane under shear: A molecular dynamics study using reversible NVT-SLLOD and NPT-SLLOD algorithms. J Chem Phys 103(23): 10192–10200 (1995)
Delhommelle J, Petravic J, Evans D J. On the effects of assuming flow profiles in nonequilibrium simulations. J Chem Phys 119(21): 11005–11010 (2003)
Delhommelle J, Petravic J, Evans D J. Reexamination of string phase and shear thickening in simple fluids. Phys Rev E 68(3): 031201 (2003)
Delhommelle J, Evans D J. Configurational temperature profile in confined fluids. II. Molecular fluids. J Chem Phys 114(14): 6236–6241 (2001)
Braga C, Travis K P. A configurational temperature Nosé-Hoover thermostat. J Chem Phys 123(13): 134101 (2005)
Yong X, Zhang L T. Thermostats and thermostat strategies for molecular dynamics simulations of nanofluidics. J Chem Phys 138(8): 084503 (2013)
Khare R, de Pablo J, Yethiraj A. Molecular simulation and continuum mechanics study of simple fluids in non-isothermal planar Couette flows. J Chem Phys 107(7): 2589–2596 (1997)
Bernardi S, Todd B D, Searles D J. Thermostating highly confined fluids. J Chem Phys 132(24): 244706 (2010)
Martini A, Hsu H Y, Patankar N A, Lichter S. Slip at high shear rates. Phys Rev Lett 100(20): 206001 (2008)
Berro H, Fillot N, Vergne P, Tokumasu T, Ohara T, Kikugawa G. Energy dissipation in non-isothermal molecular dynamics simulations of confined liquids under shear. J Chem Phys 135(13): 134708 (2011)
De Luca S, Todd B D, Hansen J S, Daivis P J. A new and effective method for thermostatting confined fluids. J Chem Phys 140(5): 054502 (2014)
Kantorovich L, Rompotis N. Generalized Langevin equation for solids. II. Stochastic boundary conditions for nonequilibrium molecular dynamics simulations. Phys Rev B 78(9): 094305 (2008)
Toton D, Lorenz C D, Rompotis N, Martsinovich N, Kantorovich L. Temperature control in molecular dynamic simulations of non-equilibrium processes. J Phys Condens Matter 22(7): 074205 (2010)
Gattinoni C, Maćkowiak S, Heyes D M, Brańka A C, Dini D. Boundary-controlled barostats for slab geometries in molecular dynamics simulations. Phys Rev E 90(4): 043302 (2014)
Lupkowski M, Van Swol F. Ultrathin films under shear. J Chem Phys 95(3): 1995–1998 (1991)
Gao J P, Luedtke W D, Landman U. Structure and solvation forces in confined films: Linear and branched alkanes. J Chem Phys 106(10): 4309–4318 (1997)
Snook I K, Van Megen W. Solvation forces in simple dense fluids. I. J Chem Phys 72(5): 2907–2913 (1980)
Gao J P, Luedtke W D, Landman U. Origins of solvation forces in confined films. J Phys Chem B 101(20): 4013–4023 (1997)
Persson B N J, Mugele F. Squeeze-out and wear: Fundamental principles and applications. J Phys Condens Matter 16(10): R295–R355 (2004)
Fajardo O Y, Bresme F, Kornyshev A A, Urbakh M. Electrotunable friction with ionic liquid lubricants: How important is the molecular structure of the ions? J Phys Chem Lett 6(20): 3998–4004 (2015)
Leng Y S. Hydration force and dynamic squeeze-out of hydration water under subnanometer confinement. J Phys Condens Matter 20(35): 354017 (2008)
Leng Y S, Xiang Y, Lei Y J, Rao Q. A comparative study by the grand canonical Monte Carlo and molecular dynamics simulations on the squeezing behavior of nanometers confined liquid films. J Chem Phys 139(7): 074704 (2013)
Lei Y J, Leng Y S. Stick-slip friction and energy dissipation in boundary lubrication. Phys Rev Lett 107(14): 147801 (2011)
Ewald P P. Die Berechnung optischer und elektrostatischer Gitterpotentiale. Ann Phys 369(3): 253–287 (1921)
Luty B A, Davis M E, Tironi I G, Van Gunsteren W F. A comparison of particle-particle, particle-mesh and Ewald methods for calculating electrostatic interactions in periodic molecular systems. Mol Simul 14(1): 11–20 (1994)
Feller S E, Pastor R W, Rojnuckarin A, Bogusz S, Brooks B R. Effect of electrostatic force truncation on interfacial and transport properties of water. J Phys Chem 100(42): 17011–17020 (1996)
Yeh I C, Berkowitz M L. Ewald summation for systems with slab geometry. J Chem Phys 111(7): 3155–3162 (1999)
Allen W, Rowley R L. Predicting the viscosity of alkanes using nonequilibrium molecular dynamics: Evaluation of intermolecular potential models. J Chem Phys 106(24): 10273–10281 (1997)
Payal R S, Balasubramanian S, Rudra I, Tandon K, Mahlke I, Doyle D, Cracknell R. Shear viscosity of linear alkanes through molecular simulations: Quantitative tests for n-decane and n-hexadecane. Mol Simulat 38(14–15): 1234–1241 (2012)
Fischer J, Paschek D, Geiger A, Sadowski G. Modeling of aqueous poly(oxyethylene) solutions: 1. Atomistic simulations. J Phys Chem B 112(8): 2388–2398 (2008)
Moore J D, Cui S T, Cochran H D, Cummings P T. Rheology of lubricant basestocks: A molecular dynamics study of C30 isomers. J Chem Phys 113(19): 8833–8840 (2000)
Ye X G, Cui S T, de Almeida V F, Khomami B. Effect of varying the 1–4 intramolecular scaling factor in atomistic simulations of long-chain N-alkanes with the OPLS-AA model. J Mol Model 19(3): 1251–1258 (2013)
Pan G A, McCabe C. Prediction of viscosity for molecular fluids at experimentally accessible shear rates using the transient time correlation function formalism. J Chem Phys 125(19): 194527 (2006)
Delhommelle J, Cummings P T. Simulation of friction in nanoconfined fluids for an arbitrarily low shear rate. Phys Rev B 72(17): 172201 (2005)
Bernardi S, Brookes S J, Searles D J, Evans D J. Response theory for confined systems. J Chem Phys 137(7): 074114 (2012)
Bocquet L, Barrat J L. Hydrodynamic boundary conditions, correlation functions, and Kubo relations for confined fluids. Phys Rev E 49(4): 3079–3092 (1994)
Petravic J, Harrowell P. On the equilibrium calculation of the friction coefficient for liquid slip against a wall. J Chem Phys 127(17): 174706 (2007)
Bocquet L, Barrat J L. On the Green-Kubo relationship for the liquid-solid friction coefficient. J Chem Phys 139(4): 044704 (2013)
Müller-Plathe F. Reversing the perturbation in nonequilibrium molecular dynamics: An easy way to calculate the shear viscosity of fluids. Phys Rev E 59(5): 4894–4898 (1999)
Bordat P, Müller-Plathe F. The shear viscosity of molecular fluids: A calculation by reverse nonequilibrium molecular dynamics. J Chem Phys 116(8): 3362–3369 (2002)
Müller T J, Müller-Plathe F. Determining the local shear viscosity of a lipid bilayer system by reverse non-equilibrium molecular dynamics simulations. ChemPhysChem 10(13): 2305–2315 (2009)
Voeltzel N, Giuliani A, Fillot N, Vergne P, Joly L. Nanolubrication by ionic liquids: Molecular dynamics simulations reveal an anomalous effective rheology. Phys Chem Chem Phys 17(35): 23226–23235 (2015)
Ramasamy U S, Len M, Martini A. Correlating molecular structure to the behavior of linear styrene–butadiene viscosity modifiers. Tribol Lett 65(4): 147 (2017)
Len M, Ramasamy U S, Lichter S, Martini A. Thickening mechanisms of polyisobutylene in polyalphaolefin. Tribol Lett 66(1): 5 (2018)
Tenney C M, Maginn E J. Limitations and recommendations for the calculation of shear viscosity using reverse nonequilibrium molecular dynamics. J Chem Phys 132(1): 014103 (2010)
Todd B D, Daivis P J. Nonequilibrium molecular dynamics simulations of planar elongational flow with spatially and temporally periodic boundary conditions. Phys Rev Lett 81(5): 1118–1121 (1998)
Bair S. Rheology and high-pressure models for quantitative elastohydrodynamics. Proc Inst Mech Eng Part J J Eng Tribol 223(4): 617–628 (2008)
Hajizadeh E, Todd B D, Daivis P J. Shear rheology and structural properties of chemically identical dendrimer-linear polymer blends through molecular dynamics simulations. J Chem Phys 141(19): 194905 (2014)
Hajizadeh E, Todd B D, Daivis P J. A molecular dynamics investigation of the planar elongational rheology of chemically identical dendrimer-linear polymer blends. J Chem Phys 142(17): 174911 (2015)
Baig C, Edwards B J, Keffer D J, Cochran H D. Rheological and structural studies of liquid decane, hexadecane, and tetracosane under planar elongational flow using nonequilibrium molecular-dynamics simulations. J Chem Phys 122(18): 184906 (2005)
Daivis P J, Todd B D. A simple, direct derivation and proof of the validity of the SLLOD equations of motion for generalized homogeneous flows. J Chem Phys 124(19): 194103 (2006)
Hunt T A, Bernardi S, Todd B D. A new algorithm for extended nonequilibrium molecular dynamics simulations of mixed flow. J Chem Phys 133(15): 154116 (2010)
Hartkamp R, Bernardi S, Todd B D. Transient-time correlation function applied to mixed shear and elongational flows. J Chem Phys 136(6): 064105 (2012)
Thompson P A, Robbins M O. Origin of stick-slip motion in boundary lubrication. Science 250(4982): 792–794 (1990)
Gee M L, McGuiggan P M, Israelachvili J N, Homola A M. Liquid to solid-like transitions of molecularly thin films under shear. J Chem Phys 93(3): 1895–1906 (1990)
Leng Y, Cummings P T. Fluidity of hydration layers nanoconfined between mica surfaces. Phys Rev Lett 94(2): 026101 (2005)
Stevens M J, Mondello M, Grest G S, Cui S T, Cochran H D, Cummings P T. Comparison of shear flow of hexadecane in a confined geometry and in bulk. J Chem Phys 106(17): 7303–7314 (1997)
Zhang J F, Todd B D, Travis K P. Viscosity of confined inhomogeneous nonequilibrium fluids. J Chem Phys 121(21): 10778–10786 (2004)
Zhang J F, Todd B D, Travis K P. Erratum: “Viscosity of confined inhomogeneous nonequilibrium fluids” [J Chem Phys 121: 10778 (2004)]. J Chem Phys 122(21): 219901 (2005)
Liem S Y, Brown D, Clarke J H R. Investigation of the homogeneous-shear nonequilibrium-molecular-dynamics method. Phys Rev A 45(6): 3706–3713 (1992)
Todd B D, Evans D J, Travis K P, Daivis P J. Comment on “Molecular simulation and continuum mechanics study of simple fluids in nonisothermal planar Couette flows” [J Chem Phys 107: 2589 (1997)]. J Chem Phys 111(23): 10730 (1999)
Cui S T, Cummings P T, Cochran H D, Moore J D, Gupta S A. Nonequilibrium molecular dynamics simulation of the rheology of linear and branched alkanes. Int J Thermophys 19(2): 449–459 (1998)
Mundy C J, Balasubramanian S, Bagchi K, Siepmann J I, Klein M L. Equilibrium and non-equilibrium simulation studies of fluid alkanes in bulk and at interfaces. Faraday Discuss 104: 17–36 (1996)
Todd B D, Daivis P J. Homogeneous non-equilibrium molecular dynamics simulations of viscous flow: Techniques and applications. Mol Simul 33(3): 189–229 (2007)
Morriss G P, Daivis P J, Evans D J. The rheology of n alkanes: Decane and Eicosane. J Chem Phys 94(11): 7420–7433 (1991)
Weeks J D, Chandler D, Andersen H C. Role of repulsive forces in determining the equilibrium structure of simple liquids. J Chem Phys 54(12): 5237–5247 (1971)
Khare R, de Pablo J, Yethiraj A. Rheological, thermodynamic, and structural studies of linear and branched alkanes under shear. J Chem Phys 107(17): 6956–6964 (1997)
Jorgensen W L, Madura J D, Swenson C J. Optimized intermolecular potential functions for liquid hydrocarbons. J Am Chem Soc 106(22): 6638–6646 (1984)
Siepmann J I, Karaborni S, Smit B. Simulating the critical behaviour of complex fluids. Nature 365(6444): 330–332 (1993)
McCabe C, Cui S T, Cummings P T. Characterizing the viscosity-temperature dependence of lubricants by molecular simulation. Fluid Phase Equilib 183–184: 363–370 (2001)
McCabe C, Cui S T, Cummings P T, Gordon P A, Saeger R B. Examining the rheology of 9-octylheptadecane to giga-pascal pressures. J Chem Phys 114(4): 1887–1891 (2001)
Liu P Z, Yu H L, Ren N, Lockwood F E, Wang Q J. Pressure-viscosity coefficient of hydrocarbon base oil through molecular dynamics simulations. Tribol Lett 60(1): 34 (2015)
Ramasamy U S, Bair S, Martini A. Predicting pressureviscosity behavior from ambient viscosity and compressibility: Challenges and opportunities. Tribol Lett 57(2): 11 (2015)
Bair S, Vergne P, Kumar P, Poll G, Krupka I, Hartl M, Habchi W, Larsson R. Comment on “History, origins and prediction of elastohydrodynamic friction” by Spikes and Jie. Tribol Lett 58(1): 16 (2015)
Spikes H, Zhang J. Reply to the comment by Scott Bair, Philippe Vergne, Punit Kumar, Gerhard Poll, Ivan Krupka, Martin Hartl, Wassim Habchi, Roland Larson on “History, origins and prediction of elastohydrodynamic friction” by Spikes and Jie in Tribology Letters. Tribol Lett 58(1): 17 (2015)
Voeltzel N, Vergne P, Fillot N, Bouscharain N, Joly L. Rheology of an Ionic Liquid with variable carreau exponent: A full picture by molecular simulation with experimental contribution. Tribol Lett 64(2): 25 (2016)
Spikes H A. Comment on: Rheology of an Ionic Liquid with Variable Carreau Exponent: A full picture by molecular simulation with experimental contribution, by Nicolas Voeltzel, Philippe Vergne, Nicolas Fillot, Nathalie Bouscharain, Laurent Joly, Tribology Letters (2016) 64: 25. Tribol Lett 65(2): 72 (2017)
Voeltzel N, Vergne P, Fillot N, Bouscharain N, Joly L. Reply to the “Comment on ‘Rheology of an ionic liquid with variable carreau exponent: A full picture by molecular simulation with experimental contribution’, by N. Voeltzel, P. Vergne, N. Fillot, N. Bouscharain, L. Joly, Tribology Letters (2016) 64: 25” by H. A. Spikes. Tribol Lett 65(2): 73 (2017)
Bird R B, Armstrong R C, Hassager O. Dynamics of Polymeric Liquids: Volume 1: Fluid Mechanics. 2nd ed. New York (USA): John Wiley and Sons, 1987.
Carreau P J. Rheological equations from molecular network theories. J Rheol 16(1): 99–127 (1972)
Liu P Z, Lu J, Yu H L, Ren N, Lockwood F E, Wang Q J. Lubricant shear thinning behavior correlated with variation of radius of gyration via molecular dynamics simulations. J Chem Phys 147(8): 084904 (2017)
Bair S, Winer W O. A quantitative test of the Einstein-Debye relation using the shear dependence of viscosity for low molecular weight liquids. Tribol Lett 26(3): 223–228 (2007)
Jadhao V, Robbins M O. Probing large viscosities in glass-formers with nonequilibrium simulations. Proc Natl Acad Sci USA 114(30): 7952–7957 (2017)
Eyring H. Viscosity, plasticity, and diffusion as examples of absolute reaction rates. J Chem Phys 4(4): 283–291 (1936)
Travis K P, Todd B D, Evans D J. Departure from Navier-Stokes hydrodynamics in confined liquids. Phys Rev E 55(4): 4288–4295 (1997)
Abraham F F. The interfacial density profile of a Lennard- Jones fluid in contact with a (100) Lennard-Jones wall and its relationship to idealized fluid/wall systems: A Monte Carlo simulation. J Chem Phys 68(8): 3713–3716 (1978)
Horn R G, Israelachvili J N. Direct measurement of structural forces between two surfaces in a nonpolar liquid. J Chem Phys 75(3): 1400–1411 (1981)
Magda J J, Tirrell M, Davis H T. Molecular dynamics of narrow, liquid-filled pores. J Chem Phys 83(4): 1888–1901 (1985)
Bitsanis I, Somers S A, Davis H T, Tirrell M. Microscopic dynamics of flow in molecularly narrow pores. J Chem Phys 93(5): 3427–3431 (1990)
Berro H. A molecular dynamics approach to nano-scale lubrication. Ph.D Thesis. Lyon (France): L’Institut National des Sciences Appliquées de Lyon, 2010.
Israelachvili J N. Measurement of the viscosity of liquids in very thin films. J Colloid Interface Sci 110(1): 263–271 (1986)
Somers S A, Davis H T. Microscopic dynamics of fluids confined between smooth and atomically structured solid surfaces. J Chem Phys 96(7): 5389–5407 (1992)
Jabbarzadeh A, Harrowell P, Tanner R I. Crystal bridge formation marks the transition to rigidity in a thin lubrication film. Phys Rev Lett 96(20): 206102 (2006)
Granick S. Motions and relaxations of confined liquids. Science 253(5026): 1374–1379 (1991)
Klein J, Kumacheva E. Simple liquids confined to molecularly thin layers. I. Confinement-induced liquid-tosolid phase transitions. J Chem Phys 108(16): 6996–7009 (1998)
Klein J, Kumacheva E. Confinement-induced phase transitions in simple liquids. Science 269(5225): 816–819 (1995)
Thompson P A, Grest G S, Robbins M O. Phase transitions and universal dynamics in confined films. Phys Rev Lett 68(23): 3448–3451 (1992)
Gao J P, Luedtke W D, Landman U. Layering transitions and dynamics of confined liquid films. Phys Rev Lett 79(4): 705–708 (1997)
Docherty H, Cummings P T. Direct evidence for fluidsolid transition of nanoconfined fluids. Soft Matter 6(8): 1640–1643 (2010)
Van Alsten J, Granick S. Molecular tribometry of ultrathin liquid films. Phys Rev Lett 61(22): 2570–2573 (1988)
Lin Z Q, Granick S. Platinum nanoparticles at mica surfaces. Langmuir 19(17): 7061–7070 (2003)
Cummings P T, Docherty H, Iacovella C R, Singh J K. Phase transitions in nanoconfined fluids: The evidence from simulation and theory. AIChE J 56(4): 842–848 (2010)
Jabbarzadeh A, Atkinson J D, Tanner R I. The effect of branching on slip and rheological properties of lubricants in molecular dynamics simulation of Couette shear flow. Tribol Int 35(1): 35–46 (2002)
Gupta S A, Cochran H D, Cummings P T. Shear behavior of squalane and tetracosane under extreme confinement. II. Confined film structure. J Chem Phys 107(23): 10327–10334 (1997)
Gao J P, Luedtke W D, Landman U. Structures, solvation forces and shear of molecular films in a rough nanoconfinement. Tribol Lett 9(1–2): 3–13 (2000)
Jabbarzadeh A, Atkinson J D, Tanner R I. Effect of the wall roughness on slip and rheological properties of hexadecane in molecular dynamics simulation of Couette shear flow between two sinusoidal walls. Phys Rev E 61(1): 690–699 (2000)
Cui S T, McCabe C, Cummings P T, Cochran H D. Molecular dynamics study of the nano-rheology of n-dodecane confined between planar surfaces. J Chem Phys 118(19): 8941–8944 (2003)
Zhu Y X, Granick S. Superlubricity: A paradox about confined fluids resolved. Phys Rev Lett 93(9): 096101 (2004)
Jabbarzadeh A, Harrowell P, Tanner R I. Very low friction state of a dodecane film confined between mica surfaces. Phys Rev Lett 94(12): 126103 (2005)
Jabbarzadeh A, Harrowell P, Tanner R I. Low friction lubrication between amorphous walls: Unraveling the contributions of surface roughness and in-plane disorder. J Chem Phys 125(3): 034703 (2006)
Jabbarzadeh A, Harrowell P, Tanner R I. The structural origin of the complex rheology in thin dodecane films: Three routes to low friction. Tribol Int 40(10–12): 1574–1586 (2007)
Martini A, Liu Y, Snurr R Q, Wang Q J. Molecular dynamics characterization of thin film viscosity for EHL simulation. Tribol Lett 21(3): 217–225 (2006)
Hu Y Z, Zhu D. A full numerical solution to the mixed lubrication in point contacts. J Tribol 122(1): 1–9 (1999)
Robbins M O, Smith E D. Connecting molecular-scale and macroscopic tribology. Langmuir 12(19): 4543–4547 (1996)
Rosenhek-Goldian I, Kampf N, Yeredor A, Klein J. On the question of whether lubricants fluidize in stick-slip friction. Proc Natl Acad Sci USA 112(23): 7117–7122 (2015)
Jee A Y, Lou K, Granick S. Scrutinizing evidence of no dilatancy upon stick–slip of confined fluids. Proc Natl Acad Sci USA 112(36): E4972 (2015)
Dhinojwala A, Bae S C, Granick S. Shear-induced dilation of confined liquid films. Tribol Lett 9(1–2): 55–62 (2000)
Zhu Y X, Granick S. Rate-dependent slip of Newtonian liquid at smooth surfaces. Phys Rev Lett 87(9): 096105 (2001)
Neto C, Evans D R, Bonaccurso E, Butt H J, Craig V S J. Boundary slip in Newtonian liquids: A review of experimental studies. Rep Prog Phys 68(12): 2859–2897 (2005)
Savio D, Fillot N, Vergne P, Zaccheddu M. A model for wall slip prediction of confined n-alkanes: Effect of wallfluid interaction versus fluid resistance. Tribol Lett 46(1): 11–22 (2012)
Fillot N, Berro H, Vergne P. From continuous to molecular scale in modelling elastohydrodynamic lubrication: Nanoscale surface slip effects on film thickness and friction. Tribol Lett 43(3): 257–266 (2011)
Washizu H, Hyodo S A, Ohmori T, Nishino N, Suzuki A. Macroscopic no-slip boundary condition confirmed in full atomistic simulation of oil film. Tribol Online 9(2): 45–50 (2014)
Jabbarzadeh A, Atkinson J D, Tanner R I. Wall slip in the molecular dynamics simulation of thin films of hexadecane. J Chem Phys 110(5): 2612–2620 (1999)
Pit R, Hervet H, Leger L. Direct experimental evidence of slip in hexadecane: Solid interfaces. Phys Rev Lett 85(5): 980–983 (2000)
Blake T D. Slip between a liquid and a solid: D. M. Tolstoi’s (1952) theory reconsidered. Colloids Surf 47: 135–145 (1990)
Spikes H, Granick S. Equation for slip of simple liquids at smooth solid surfaces. Langmuir 19(12): 5065–5071 (2003)
De Gennes P G. On fluid/wall slippage. Langmuir 18(9): 3413–3414 (2002)
Wang F C, Zhao Y P. Slip boundary conditions based on molecular kinetic theory: The critical shear stress and the energy dissipation at the liquid–solid interface. Soft Matter 7(18): 8628–8634 (2011)
Vadakkepatt A, Dong Y L, Lichter S, Martini A. Effect of molecular structure on liquid slip. Phys Rev E 84(6): 066311 (2011)
Majumdar A, Bhushan B. Role of fractal geometry in roughness characterization and contact mechanics of surfaces. J Tribol 112(2): 205–216 (1990)
Savio D, Pastewka L, Gumbsch P. Boundary lubrication of heterogeneous surfaces and the onset of cavitation in frictional contacts. Sci Adv 2(3): e1501585 (2016)
Reynolds O. On the theory of lubrication and its application to Mr. Beauchamp Tower’s experiments, including an experimental determination of the viscosity of olive oil. Proc Roy Soc Lond 40(242–245): 191–203 (1886)
Zhu Y X, Granick S. Limits of the hydrodynamic no-slip boundary condition. Phys Rev Lett 88(10): 106102 (2002)
Savio D, Fillot N, Vergne P, Hetzler H, Seemann W, Morales-Espejel G E. A multiscale study on the wall slip effect in a ceramic-steel contact with nanometer-thick lubricant film by a nano-to-elastohydrodynamic lubrication approach. J Tribol 137(3): 031502 (2015)
Ponjavic A, Wong J S S. The effect of boundary slip on elastohydrodynamic lubrication. RSC Adv 4(40): 20821–20829 (2014)
Wong P L, Li X M, Guo F. Evidence of lubricant slip on steel surface in EHL contact. Tribol Int 61: 116–119 (2013)
Martinie L, Vergne P. Lubrication at extreme conditions: A discussion about the limiting shear stress concept. Tribol Lett 63(2): 21 (2016)
Heyes D M, Smith E R, Dini D, Spikes H A, Zaki T A. Pressure dependence of confined liquid behavior subjected to boundary-driven shear. J Chem Phys 136(13): 134705 (2012)
Gattinoni C, Heyes D M, Lorenz C D, Dini D. Traction and nonequilibrium phase behavior of confined sheared liquids at high pressure. Phys Rev E 88(5): 052406 (2013)
Maćkowiak S, Heyes D M, Dini D, Brańka A C. Non-equilibrium phase behavior and friction of confined molecular films under shear: A non-equilibrium molecular dynamics study. J Chem Phys 145(16): 164704 (2016)
Thompson P A, Robbins M O. Shear flow near solids: Epitaxial order and flow boundary conditions. Phys Rev A 41(12): 6830–6837 (1990)
Butler S, Harrowell P. Factors determining crystal-liquid coexistence under shear. Nature 415(6875): 1008–1011 (2002)
Butler S, Harrowell P. Simulation of the coexistence of a shearing liquid and a strained crystal. J Chem Phys 118(9): 4115–4126 (2003)
Butler S, Harrowell P. Structure and stability of the interface between a strained crystal and a shearing liquid. Phys Rev E 67(5): 051503 (2003)
Varnik F, Bocquet L, Barrat J L, Berthier L. Shear localization in a model glass. Phys Rev Lett 90(9): 095702 (2003)
Rottler J, Robbins M O. Shear yielding of amorphous glassy solids: Effect of temperature and strain rate. Phys Rev E 68(1): 011507 (2003)
Heyes D M. The Liquid State: Applications of Molecular Simulations. New York (USA): Wiley, 1998.
Bair S, Winer W O. The high pressure high shear stress rheology of liquid lubricants. J Tribol 114(1): 1–9 (1992)
Bair S, Qureshi F, Winer W O. Observations of shear localization in liquid lubricants under pressure. J Tribol 115(3): 507–513 (1993)
Bair S, McCabe C. A study of mechanical shear bands in liquids at high pressure. Tribol Int 37(10): 783–789 (2004)
Ponjavic A, Chennaoui M, Wong J S S. Through-thickness velocity profile measurements in an elastohydrodynamic contact. Tribol Lett 50(2): 261–277 (2013)
Ponjavic A, di Mare L, Wong J S S. Effect of pressure on the flow behavior of polybutene. J Polym Sci B Polym Phys 52(10): 708–715 (2014)
Galmiche B, Ponjavic A, Wong J S S. Flow measurements of a polyphenyl ether oil in an elastohydrodynamic contact. J Phys Condens Matter 28(13): 134005 (2016)
Šperka P, Křupka I, Hartl M. Evidence of plug flow in rolling-sliding elastohydrodynamic contact. Tribol Lett 54(2): 151–160 (2014)
Sperka P, Krupka I, Hartl M. Lubricant flow in thin-film elastohydrodynamic contact under extreme conditions. Friction 4(4): 380–390 (2016)
Zhang J, Tan A, Spikes H. Effect of base oil structure on elastohydrodynamic friction. Tribol Lett 65(1): 13 (2017)
Spikes H, Tysoe W. On the commonality between theoretical models for fluid and solid friction, wear and tribochemistry. Tribol Lett 59(1): 14 (2015)
Washizu H, Ohmori T, Suzuki A. Molecular origin of limiting shear stress of elastohydrodynamic lubrication oil film studied by molecular dynamics. Chem Phys Lett 678: 1–4 (2017)
Bodnarchuk M S, Heyes D M, Breakspear A, Chahine S, Dini D. A molecular dynamics study of CaCO3 nanoparticles in a hydrophobic solvent with a stearate co-surfactant. Phys Chem Chem Phys 17(20): 13575–13581 (2015)
Bodnarchuk M S, Dini D, Heyes D M, Breakspear A, Chahine S. Molecular dynamics studies of overbased detergents on a water surface. Langmuir 33(29): 7263–7270 (2017)
Tomlinson A, Danks T N, Heyes D M, Taylor S E, Moreton D J. Interfacial characterization of succinimide surfactants. Langmuir 13(22): 5881–5893 (1997)
Tomlinson A, Scherer B, Karakosta E, Oakey M, Danks T N, Heyes D M, Taylor S E. Adsorption properties of succinimide dispersants on carbonaceous substrates. Carbon 38(1): 13–28 (2000)
Ramasamy U S, Lichter S, Martini A. Effect of molecularscale features on the polymer coil size of model viscosity index improvers. Tribol Lett 62(2): 23 (2016)
Jiang S Y, Frazier R, Yamaguchi E S, Blanco M, Dasgupta S, Zhou Y H, Cagin T, Tang Y C, Goddard III W A. The SAM model for wear inhibitor performance of dithiophosphates on iron oxide. J Phys Chem B 101(39): 7702–7709 (1997)
Zhou Y H, Jiang S Y, Çaǧın T, Yamaguchi E S, Frazier R, Ho A, Tang Y C, Goddard III W A. Application of the self-assembled monolayer (SAM) model to dithiophosphate and dithiocarbamate engine wear inhibitors. J Phys Chem A 104(11): 2508–2524 (2000)
Minfray C, Le Mogne T, Martin J M, Onodera T, Nara S, Takahashi S, Tsuboi H, Koyama M, Endou A, Takaba H, et al. Experimental and molecular dynamics simulations of tribochemical reactions with ZDDP: Zinc phosphate–iron oxide reaction. Tribol Trans 51(5): 589–601 (2008)
Berro H, Fillot N, Vergne P. Molecular dynamics simulation of surface energy and ZDDP effects on friction in nano-scale lubricated contacts. Tribol Int 43(10): 1811–1822 (2010)
Ramachandran S, Tsai B L, Blanco M, Chen H, Tang Y C, Goddard III W A. Self-assembled monolayer mechanism for corrosion inhibition of iron by imidazolines. Langmuir 12(26): 6419–6428 (1996)
Ramachandran S, Tsai B L, Blanco M, Chen H, Tang Y C, Goddard III W A. Atomistic simulations of oleic imidazolines bound to ferric clusters. J Phys Chem A 101(1): 83–89 (1997)
Xia S W, Qiu M, Yu L M, Liu F G, Zhao H Z. Molecular dynamics and density functional theory study on relationship between structure of imidazoline derivatives and inhibition performance. Corros Sci 50(7): 2021–2029 (2008)
Spikes H. Friction modifier additives. Tribol Lett 60(1): 5 (2015)
Beltzer M, Jahanmir S. Role of dispersion interactions between hydrocarbon chains in boundary lubrication. ASLE Trans 30(1): 47–54 (1987)
Lundgren S M, Ruths M, Danerlöv K, Persson K. Effects of unsaturation on film structure and friction of fatty acids in a model base oil. J Colloid Interface Sci 326(2): 530–536 (2008)
Campen S, Green J H, Lamb G D, Spikes H A. In situ study of model organic friction modifiers using liquid cell AFM; Saturated and Mono-unsaturated carboxylic acids. Tribol Lett 57(2): 18 (2015)
Wood M H, Casford M T, Steitz R, Zarbakhsh A, Welbourn R J L, Clarke S M. Comparative adsorption of saturated and unsaturated fatty acids at the iron oxide/oil interface. Langmuir 32(2): 534–540 (2016)
Rai B, Pradip P. Modeling self-assembly of surfactants at interfaces. Curr Opin Chem Eng 15: 84–94 (2017)
Briscoe B J, Evans D C B. The shear properties of Langmuir-Blodgett layers. Proc Roy Soc A Math Phys Eng Sci 380(1779): 389–407 (1982)
Moller M A, Tildesley D J, Kim K S, Quirke N. Molecular dynamics simulation of a langmuir-blodgett film. J Chem Phys 94(12): 8390–8401 (1991)
Karaborni S, Verbist G. Effect of chain conformation on the tilt behaviour in langmuir monolayers. Eur Lett 27(6): 467–472 (1994)
Davidson J E, Hinchley S L, Harris S G, Parkin A, Parsons S, Tasker P A. Molecular dynamics simulations to aid the rational design of organic friction modifiers. J Mol Graphics Modell 25(4): 495–506 (2006)
Greenfield M L, Ohtani H. Molecular dynamics simulation study of model friction modifier additives confined between two surfaces. Tribol Lett 7(2–3): 137–145 (1999)
Ruths M, Ohtani H, Greenfield M, Granick S. Exploring the “friction modifier” phenomenon: Nanorheology of n-alkane chains with polar terminus dissolved in n-alkane solvent. Tribol Lett 6(3–4): 207–214 (1999)
Greenfield M L, Ohtani H. Packing of simulated friction modifier additives under confinement. Langmuir 21(16): 7568–7578 (2005)
Doig M, Camp P J. The structures of hexadecylamine films adsorbed on iron-oxide surfaces in dodecane and hexadecane. Phys Chem Chem Phys 17(7): 5248–5255 (2015)
Wood M H, Welbourn R J L, Charlton T, Zarbakhsh A, Casford M T, Clarke S M. Hexadecylamine adsorption at the iron oxide-oil interface. Langmuir 29(45): 13735–13742 (2013)
Bradley-Shaw J L, Camp P J, Dowding P J, Lewtas K. Molecular dynamics simulations of glycerol monooleate confined between mica surfaces. Langmuir 32(31): 7707–7718 (2016)
Bradley-Shaw J L, Camp P J, Dowding P J, Lewtas K. Glycerol monooleate reverse micelles in nonpolar solvents: Computer simulations and small-angle neutron scattering. J Phys Chem B 119(11): 4321–4331 (2015)
Glosli J N, McClelland G M. Molecular dynamics study of sliding friction of ordered organic monolayers. Phys Rev Lett 70(13): 1960–1963 (1993)
Kong Y C, Tildesley D J, Alejandre J. The molecular dynamics simulation of boundary-layer lubrication. Mol Phys 92(1): 7–18 (1997)
Kong Y C, Tildesley D J. The effect of molecular geometry on boundary layer lubrication. Mol Simul 22(2): 149–168 (1999)
Eder S J, Vernes A, Betz G. On the derjaguin offset in boundary-lubricated nanotribological systems. Langmuir 29(45): 13760–13772 (2013)
Eder S, Vernes A, Vorlaufer G, Betz G. Molecular dynamics simulations of mixed lubrication with smooth particle post-processing. J Phys Condens Matter 23(17): 175004 (2011)
Doig M, Warrens C P, Camp P J. Structure and friction of stearic acid and oleic acid films adsorbed on iron oxide surfaces in squalane. Langmuir 30(1): 186–195 (2014)
Campen S, Green J, Lamb G, Atkinson D, Spikes H. On the Increase in Boundary Friction with Sliding Speed. Tribol Lett 48(2): 237–248 (2012)
Ewen J P, Gattinoni C, Morgan N, Spikes H, Dini D. Nonequilibrium molecular dynamics simulations of organic friction modifiers adsorbed on iron oxide surfaces. Langmuir 32(18): 4450–4463 (2016)
Ewen J P, Echeverri Restrepo S, Morgan N, Dini D. Nonequilibrium molecular dynamics simulations of stearic acid adsorbed on iron surfaces with nanoscale roughness. Tribol Int 107: 264–273 (2017)
Koike A, Yoneya M. Molecular dynamics simulations of sliding friction of Langmuir-Blodgett monolayers. J Chem Phys 105(14): 6060–6067 (1996)
Koike A, Yoneya M. Effects of molecular structure on frictional properties of langmuir-Blodgett monolayers. Langmuir 13(6): 1718–1722 (1997)
Lewis J B, Vilt S G, Rivera J L, Jennings G K, McCabe C. Frictional properties of mixed fluorocarbon/hydrocarbon silane monolayers: A simulation study. Langmuir 28(40): 14218–14226 (2012)
Lorenz C D, Chandross M, Grest G S, Stevens M J, Webb III E B. Tribological properties of alkylsilane self-assembled monolayers. Langmuir 21(25): 11744–11748 (2005)
Lorenz C D, Chandross M, Lane J M D, Grest G S. Nanotribology of water confined between hydrophilic alkylsilane self-assembled monolayers. Modell Simul Mater Sci Eng 18(3): 034005 (2010)
Black J E, Iacovella C R, Cummings P T, McCabe C. Molecular dynamics study of alkylsilane monolayers on realistic amorphous silica surfaces. Langmuir 31(10): 3086–3093 (2015)
Summers A Z, Iacovella C R, Billingsley M R, Arnold S T, Cummings P T, McCabe C. Influence of surface morphology on the shear-induced wear of alkylsilane monolayers: Molecular dynamics study. Langmuir 32(10): 2348–2359 (2016)
Tupper K J, Brenner D W. Molecular dynamics simulations of friction in self-assembled monolayers. Thin Solid Films 253(1–2): 185–189 (1994)
Ramin L, Jabbarzadeh A. Frictional properties of two alkanethiol self assembled monolayers in sliding contact: Odd-even effects. J Chem Phys 137(17): 174706 (2012)
Ramin L, Jabbarzadeh A. Effect of water on structural and frictional properties of self assembled monolayers. Langmuir 29(44): 13367–13378 (2013)
Kroto H W, Heath J R, O’Brien S C, Curl R F, Smalley R E. C60: Buckminsterfullerene. Nature 318(6042): 162–163 (1985)
Ghaednia H, Babaei H, Jackson R L, Bozack M J, Khodadadi J M. The effect of nanoparticles on thin film elasto-hydrodynamic lubrication. Appl Phys Lett 103(26): 263111 (2013)
Ewen J P, Gattinoni C, Thakkar F M, Morgan N, Spikes H A, Dini D. Nonequilibrium molecular dynamics investigation of the reduction in friction and wear by carbon nanoparticles between iron surfaces. Tribol Lett 63(3): 38 (2016)
Liang Q, Tsui O K C, Xu Y B, Li H N, Xiao X D. Effect of C60 molecular rotation on nanotribology. Phys Rev Lett 90(14): 146102 (2003)
Coffey T, Krim J. C60 molecular bearings and the phenomenon of nanomapping. Phys Rev Lett 96(18): 186104 (2006)
Lee K, Hwang Y, Cheong S, Choi Y, Kwon L, Lee J, Kim S H. Understanding the role of nanoparticles in nano-oil lubrication. Tribol Lett 35(2): 127–131 (2009)
Zhmud B, Pasalskiy B. Nanomaterials in lubricants: An industrial perspective on current research. Lubricants 1(4): 95–101 (2013)
Tevet O, Von-Huth P, Popovitz-Biro R, Rosentsveig R, Wagner H D, Tenne R. Friction mechanism of individual multilayered nanoparticles. Proc Natl Acad Sci USA 108(50): 19901–19906 (2011)
Lahouij I, Bucholz E W, Vacher B, Sinnott S B, Martin J M, Dassenoy F. Lubrication mechanisms of hollow-core inorganic fullerene-like nanoparticles: Coupling experimental and computational works. Nanotechnology 23(37): 375701 (2012)
Joly-Pottuz L, Bucholz E W, Matsumoto N, Phillpot S R, Sinnott S B, Ohmae N, Martin J M. Friction properties of carbon nano-onions from experiment and computer simulations. Tribol Lett 37(1): 75–81 (2010)
Bucholz E W, Phillpot S R, Sinnott S B. Molecular dynamics investigation of the lubrication mechanism of carbon nano-onions. Comput Mater Sci 54: 91–96 (2012)
Bucholz E W, Sinnott S B. Computational investigation of the mechanical and tribological responses of amorphous carbon nanoparticles. J Appl Phys 113(7): 073509 (2013)
Hu C Z, Bai M L, Lv J Z, Liu H, Li X J. Molecular dynamics investigation of the effect of copper nanoparticle on the solid contact between friction surfaces. Appl Surf Sci 321: 302–309 (2014)
Hu C Z, Bai M L, Lv J Z, Kou Z H, Li X J. Molecular dynamics simulation on the tribology properties of two hard nanoparticles (diamond and silicon dioxide) confined by two iron blocks. Tribol Int 90: 297–305 (2015)
Eder S J, Feldbauer G, Bianchi D, Cihak-Bayr U, Betz G, Vernes A. Applicability of macroscopic wear and friction laws on the atomic length scale. Phys Rev Lett 115(2): 025502 (2015)
Barwell F T. Wear of metals. Wear 1(4): 317–332 (1958)
Bowden F P, Tabor D. The Friction and Lubrication of Solids. Oxford (UK): Oxford University Press, 1950.
Hunter C N, Check M H, Hager C H, Voevodin A A. Tribological properties of carbon nanopearls synthesized by nickel-catalyzed chemical vapor deposition. Tribol Lett 30(3): 169–176 (2008)
Hu C Z, Bai M L, Lv J Z, Wang P, Li X J. Molecular dynamics simulation on the friction properties of nanofluids confined by idealized surfaces. Tribol Int 78: 152–159 (2014)
Hu C Z, Bai M L, Lv J Z, Li X J. Molecular dynamics simulation of mechanism of nanoparticle in improving load-carrying capacity of lubricant film. Comput Mater Sci 109: 97–103 (2015)
Peña-Parás L, Gao H Y, Maldonado-Cortés D, Vellore A, García-Pineda P, Montemayor O E, Nava K L, Martini A. Effects of substrate surface roughness and nano/micro particle additive size on friction and wear in lubricated sliding. Tribol Int 119: 88–98 (2018)
Horstemeyer M F. Integrated Computational Materials Engineering (ICME) for Metals: Using Multiscale Modeling to Invigorate Engineering Design with Science. Honoken (USA): Wiley, 2012.
Neville A, Morina A, Haque T, Voong Q. Compatibility between tribological surfaces and lubricant additives— How friction and wear reduction can be controlled by surface/lube synergies. Tribol Int 40(10–12): 1680–1695 (2007)
Van Duin A C T, Dasgupta S, Lorant F, Goddard III W A. ReaxFF: A reactive force field for hydrocarbons. J Phys Chem A 105(41): 9396–9409 (2001)
Senftle T P, Hong S, Islam M M, Kylasa S B, Zheng Y X, Shin Y K, Junkermeier C, Engel-Herbert R, Janik M J, Aktulga H M, Verstraelen T, Grama A, Van Duin A C T. The ReaxFF reactive force-field: Development, applications and future directions. NPJ Comput Mater 2(1): 15011 (2016)
Liang T, Shin Y K, Cheng Y T, Yilmaz D E, Vishnu K G, Verners O, Zou C Y, Phillpot S R, Sinnott S B, Van Duin A C T. Reactive potentials for advanced atomistic simulations. Annu Rev Mater Res 43: 109–129 (2013)
Böhm O, Pfadenhauer S, Leitsmann R, Plänitz P, Schreiner E, Schreiber M. ReaxFF+—A new reactive force field method for the accurate description of ionic systems and its application to the hydrolyzation of aluminosilicates. J Phys Chem C 120(20): 10849–10856 (2016)
Chenoweth K, Van Duin A C T, Goddard III W A. ReaxFF reactive force field for molecular dynamics simulations of hydrocarbon oxidation. J Phys Chem A 112(5): 1040–1053 (2008)
Yeon J, He X, Martini A, Kim S H. Mechanochemistry at solid surfaces: Polymerization of adsorbed molecules by mechanical shear at tribological interfaces. ACS Appl Mater Interfaces 9(3): 3142–3148 (2017)
Yue D C, Ma T B, Hu Y Z, Yeon J, Van Duin A C T, Wang H, Luo J B. Tribochemistry of phosphoric acid sheared between quartz surfaces: A reactive molecular dynamics study. J Phys Chem C 117(48): 25604–25614 (2013)
Yeon J, Adams H L, Junkermeier C E, Van Duin A C T, Tysoe W T, Martini A. Development of a ReaxFF force field for Cu/S/C/H and reactive MD simulations of methyl thiolate decomposition on Cu(100). J Phys Chem B 122(2): 888–896 (2018)
Ostadhossein A, Rahnamoun A, Wang Y X, Zhao P, Zhang S L, Crespi V H, Van Duin A C T. ReaxFF reactive force-field study of molybdenum disulfide (MoS2). J Phys Chem Lett 8(7): 631–640 (2017)
Mosey N J, Woo T K. Finite temperature structure and dynamics of zinc dialkyldithiophosphate wear inhibitors: A density functional theory and ab initio molecular dynamics study. J Phys Chem A 107(25): 5058–5070 (2003)
Mosey N J, Müser M H, Woo T K. Molecular mechanisms for the functionality of lubricant additives. Science 307(5715): 1612–1615 (2005)
Mosey N J, Woo T K. Insights into the chemical behavior of zinc dialkyldithiophosphate anti-wear additives in their isomeric and decomposed forms through molecular simulation. Tribol Int 39(9): 979–993 (2006)
Mosey N J, Woo T K. An ab initio molecular dynamics and density functional theory study of the formation of phosphate chains from metathiophosphates. Inorg Chem 45(18): 7464–7479 (2006)
Levita G, Righi M C. Effects of water intercalation and tribochemistry on MoS2 lubricity: An ab initio molecular dynamics investigation. ChemPhysChem 18(11): 1475–1480 (2017)
Onodera T, Morita Y, Suzuki A, Koyama M, Tsuboi H, Hatakeyama N, Endou A, Takaba H, Kubo M, Dassenoy F, et al. A computational chemistry study on friction of h-MoS2. Part I. Mechanism of single sheet lubrication. J Phys Chem B 113(52): 16526–16536 (2009)
Onodera T, Morita Y, Suzuki A, Sahnoun R, Koyama M, Tsuboi H, Hatakeyama N, Endou A, Takaba H, Del Carpio C A, et al. Tribochemical reaction dynamics of molybdenum dithiocarbamate on nascent iron surface: A hybrid quantum chemical/classical molecular dynamics study. J Nanosci Nanotechnol 10(4): 2495–2502 (2010)
Onodera T, Morita Y, Nagumo R, Miura R, Suzuki A, Tsuboi H, Hatakeyama N, Endou A, Takaba H, Dassenoy F, et al. A computational chemistry study on friction of h-MoS2. Part II. Friction anisotropy. J Phys Chem B 114(48): 15832–15838 (2010)
Onodera T, Martin J M, Minfray C, Dassenoy F, Miyamoto A. Antiwear chemistry of ZDDP: Coupling classical MD and tight-binding quantum chemical MD methods (TB-QCMD). Tribol Lett 50(1): 31–39 (2013)
Loehle S, Matta C, Minfray C, Le Mogne T, Martin J M, Iovine R, Obara Y, Miura R, Miyamoto A. Mixed lubrication with C18 fatty acids: Effect of unsaturation. Tribol Lett 53(1): 319–328 (2014)
Loehlé S, Matta C, Minfray C, Le Mogne T, Iovine R, Obara Y, Miyamoto A, Martin J M. Mixed lubrication of steel by C18 fatty acids revisited. Part I: Toward the formation of carboxylate. Tribol Int 82: 218–227 (2015)
Loehlé S, Matta C, Minfray C, Le Mogne T, Iovine R, Obara Y, Miyamoto A, Martin J M. Mixed lubrication of steel by C18 fatty acids revisited. Part II: Influence of some key parameters. Tribol Int 94: 207–216 (2016)
Knight C, Maupin C M, Izvekov S, Voth G A. Defining condensed phase reactive force fields from ab initio molecular dynamics simulations: The case of the hydrated excess proton. J Chem Theory Comput 6(10): 3223–3232 (2010)
Ma C R, Martin-Samos L, Fabris S, Laio A, Piccinin S. QMMMW: A wrapper for QM/MM simulations with QUANTUM ESPRESSO and LAMMPS. Comput Phys Commun 195: 191–198 (2015)
Makov G, Gattinoni C, De Vita A. Ab initio based multiscale modelling for materials science. Modell Simul Mater Sci Eng 17(8): 084008 (2009)
Bernstein N, Kermode J R, Csányi G. Hybrid atomistic simulation methods for materials systems. Rep Prog Phys 72(2): 026501 (2009)
Lin H, Truhlar D G. QM/MM: What have we learned, where are we, and where do we go from here? Theor Chem Acc 117(2): 185–199 (2007)
Singh M K, Ilg P, Espinosa-Marzal R M, Kröger M, Spencer N D. Polymer brushes under shear: Molecular dynamics simulations compared to experiments. Langmuir 31(16): 4798–4805 (2015)
Singh M K, Ilg P, Espinosa-Marzal R M, Spencer N D, Kröger M. Influence of chain stiffness, grafting density and normal load on the tribological and structural behavior of polymer brushes: A nonequilibrium-molecular-dynamics study. Polymers 8(7): 254 (2016)
Farrow M R, Chremos A, Camp P J, Harris S G, Watts R F. Molecular simulations of kinetic-friction modification in nanoscale fluid layers. Tribol Lett 42(3): 325–337 (2011)
Everaers R, Sukumaran S K, Grest G S, Svaneborg C, Sivasubramanian A, Kremer K. Rheology and microscopic topology of entangled polymeric liquids. Science 303(5659): 823–826 (2004)
Holland D M, Lockerby D A, Borg M K, Nicholls W D, Reese J M. Molecular dynamics pre-simulations for nanoscale computational fluid dynamics. Microfluid Nanofluidics 18(3): 461–474 (2015)
Smith E R, Heyes D M, Dini D, Zaki T A. Controlvolume representation of molecular dynamics. Phys Rev E 85(5): 056705 (2012)
Smith E R, Heyes D M, Dini D, Zaki T A. A localized momentum constraint for non-equilibrium molecular dynamics simulations. J Chem Phys 142(7): 074110 (2015)
O’Connell S T, Thompson P A. Molecular dynamicscontinuum hybrid computations: A tool for studying complex fluid flows. Phys Rev E 52(6): R5792–R5795 (1995)
Flekkøy E G, Wagner G, Feder J. Hybrid model for combined particle and continuum dynamics. Europhys Lett 52(3): 271–276 (2000)
Nie S Y, Chen S Y, W N E, Robbins M O. A continuum and molecular dynamics hybrid method for micro- and nano-fluid flow. J Fluid Mech 500: 55–64 (2004)
Ren W Q. Analytical and numerical study of coupled atomistic-continuum methods for fluids. J Comput Phys 227(2): 1353–1371 (2007)
Mohamed K M, Mohamad A A. A review of the development of hybrid atomistic-continuum methods for dense fluids. Microfluid Nanofluidics 8(3): 283–302 (2010)
Smith E R, Trevelyan D, Ramos E. cpl-library. https://doi.org/10.5281/zenodo.46573, 2016.
Acknowledgements
J. P. E thanks the Engineering and Physical Sciences Research Council (EPSRC) for financial support through a Doctoral Prize Fellowship. D. D. acknowledges the EPSRC for an Established Career Fellowship EP/N025954/1. The authors also thank Hugh A. Spikes and B. D. Todd for helpful discussions.
Author information
Authors and Affiliations
Corresponding author
Additional information
James P. EWEN. He is an EPSRC Doctoral Prize Fellow in the Tribology Group at Imperial College London. He gained his master degree in chemistry from the University of Bath in 2014 and his PhD degree from Imperial College in 2017 with a Thesis entitled “Molecular dynamics simulations of lubricants and additives”. His research involves utilising molecular simulations to investigate the structure, flow, and friction behaviour of a range of tribological systems.
David. M HEYES. He is a Principal Research Fellow in the Tribology Group at Imperial College London. He received his PhD degree from the University of Manchester in 1977. He has held postdoctoral research positions in the Vitreous State Laboratory, Department of Physics, Catholic University of America, Washington DC, USA, Department of Physical Chemistry at the University of Amsterdam, and at the Department of Chemistry, Royal Holloway, University of London. He was a Royal Society (London) University Research Fellow between 1983 and 1992, first at Royal Holloway. His research interests are in the development of computer-based theoretical and modelling techniques applied to condensed matter liquid systems, principally in the areas of phase equilibria, and structural and transport properties of bulk and confined systems.
Daniele DINI. He is Head of the Tribology Group at Imperial College London. Prior to joining Imperial College in 2006, Professor Dini received the DPhil degree from the Department of Engineering Science at the University of Oxford in 2004. He now leads the advanced modelling research within the Tribology Group and collaborates closely with its experimentalists. His current individual research portfolio supports a large team of researchers focused on studies related to the modelling of tribological systems and materials. Most of these projects are multidisciplinary and range from atomic and molecular simulation of lubricant, additives and surfaces to the modelling of machine or biomechanical components. His group performs fundamental research, while successfully supporting the application of tribology in industry; the strong links with industrial partners have been recently recognised by the Imperial College President’s Award and Medal for Excellence in External Collaboration and Partnerships (2017). Professor Dini has also been the recipient of a number of other individual awards, he has also been elected a Fellow of the IMechE in 2014, and is the recipient of the prestigious EPSRC Established Career Fellowship, awarded in 2016.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made.
The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder.
To view a copy of this licence, visit https://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Ewen, J.P., Heyes, D.M. & Dini, D. Advances in nonequilibrium molecular dynamics simulations of lubricants and additives. Friction 6, 349–386 (2018). https://doi.org/10.1007/s40544-018-0207-9
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s40544-018-0207-9