A theory of managerial compensation and taxation with endogenous risk

Abstract

We study the impact of endogenous shocks driven by collective actions of managers. We analyze how such endogenous shocks impact social welfare by employing an overlapping-generations model. We first prove that the competitive equilibrium allocation is suboptimal because of the externalities in managers’ wages and in equity market. We establish that a socially optimal allocation can be achieved if the planner imposes wage taxes (or subsidies) on managers and equity taxes. Our results help provide an alternative explanation as to why managers are compensated and taxed differently than other workers. We then extend the model by incorporating unobservable actions for managers and show that a second-best allocation can be implemented if the planner imposes equity taxes.

1 Introduction

Economists have always been of two minds when it comes to modeling uncertainty. The earliest approach, which is called the “state-of-nature” (or Savage) approach, models the probabilities of possible outcomes as fixed and independent of agents’ actions. In the traditional models, it is reasonable to assume that output shocks are exogenous and independent of agents’ actions as agents take the states of the world given. However, the state-of-nature approach may be inadequate when a model considers settings in which agents’ actions intrinsically affect the state of the economy and hence the well-being or distress of firms and households in the economy.

A good recent example of how agents’ actions affects the state of the economy is how the seizing up of the mortgage-backed securities market ended up freezing the world financial markets in 2008. In principle, bundling mortgages from different areas and different income profiles made a perfect sense as a way of diversifying the risk of a borrower defaulting on the mortgage. Packaging these up as securities to sell to large numbers of investors also further diversified the default risk. One drawback of the process, however, was the decoupling of loan origination from risk bearing. In a number of un- or under-regulated real estate markets, far too many so-called “sub-prime loans” were made to borrowers who clearly could not afford to carry the mortgage. This also generated an increase in housing values, which led a number of homeowners to increase consumption. When the inevitable defaults started and the housing price bubble deflated, credit markets ended up in panic, because no one knew what the various mortgage-backed securities were actually worth. Securitization of loans indeed reduced an individual bank’s risk exposure, but the collective actions of all banks (or bank managers) engaging in securitization resulted in a spectacular failure.

Motivated by examples like the 2008 crisis, we study how endogenous shocks driven by collective actions of managers impact social welfare. In particular, we ask the following research questions: (Q1) do markets deliver a socially optimal allocation in the presence of endogenous shocks? (Q2) if not, how can the social planner implement a socially optimal allocation? Because managers’ actions may be unobservable, we examine how endogenous shocks impact social welfare under information asymmetry. Specifically, we ask: (Q3) how can the social planner implement a second-best allocation?

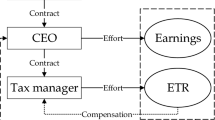
To address these questions, we construct an overlapping-generations model with two-period-lived agents in which young agents serve as line workers/lower level employees, whereas old agents serve as managers. This is because we observe a natural division of labor across the age spectrum. For most established companies, top-tier managers are middle aged or older. For example, 87% of S&P 100 companies have CEOs older than 50 years, and for S&P 500 companies, median age of CEOs is 56, and average age of CEOs is 55 (cf. Spencer Stuart). This is because management activities are qualitatively different from even the most technically demanding production activities that firm engage in. Managers fundamentally work to maximize the probability of favorable outcomes (e.g., innovating new products) and minimize the probability of adverse outcomes (improving quality and safety of current products) in their firms’ production activities. This task necessitates a degree of comprehension of the overall structure and function of the firm that even very well-educated line workers typically do not have. Obtaining this knowledge requires a combination of early on-the-job training at entry level activities, typically followed by the attainment of an advanced degree (generally an MBA), and then another stint on the managerial career ladder learning the idiosyncrasies of the firm’s overall performance. Because this all takes time to accomplish, we see a natural division of labor across the age spectrum: young agents serve as line workers/lower level employees, while old agents serve as risk managers. In return for their service, young and old agents earn wages. Moreover, agents hold ownership shares of firms, i.e., equities.

Before answering the research questions (Q1)–(Q3) listed above, we first prove the existence of a competitive equilibrium allocation (Proposition 1). We then prove that competitive equilibrium allocation is suboptimal in the presence of endogenous shocks driven by collective actions of managers (Proposition 2). The source of inefficiency is the externalities in managers’ wages and in equity market. These externalities must be internalized to restore efficiency. We establish that to attain a socially optimal allocation, the social planner must impose wage taxes (or subsidies) on managers and equity taxes (Theorem 1). Our results provide an alternative explanation as to why managers are compensated and taxed differently than other workers. We then extend the model by incorporating unobservable actions for managers and show that a second-best allocation can be implemented if the social planner imposes equity taxes (Proposition 3).

1.1 Related literature

This paper is broadly related to three streams of the literature: the overlapping generations, managerial compensation, and managerial taxation literature.

The overlapping-generations literature has long been concerned about the optimality of competitive equilibrium under different settings. For example, Cass (1972) and Gale (1973) provide ways to determine whether competitive equilibrium is Pareto optimal. Peled (1982) and Chattopadhyay and Gottardi (1999) prove the optimality of competitive equilibrium in a pure-exchange economy, where agents live two periods. Demange (2002) gives a comprehensive characterization of different optimality notions for competitive equilibrium. We contribute to this literature by incorporating endogenous shocks and characterizing the equilibrium under endogenous shocks.

Other streams of related literature are managerial compensation and taxation literature. Since Jensen and Meckling (1976), the managerial compensation literature focuses on the agency problem—in particular, managers do not necessarily act in the best interest of shareholders, so shareholders offer contracts to incentivize managers to maximize shareholder value (see Murphy 1999 for a comprehensive survey). The managerial taxation literature usually assumes that managers’ effort is private information, so the social planner pays informational rent to managers to extract that information (see Mankiw et al. 2009; Diamond and Saez 2011 for surveys).¹ Both managerial compensation and taxation literature derive impactful results and provide useful insights by considering information asymmetries. We contribute to these streams of the literature by identifying an alternative reason why managers are compensated and taxed differently than other workers using a model without information asymmetries.

The closest study to this paper is a recent paper by Magill et al. (2015). Magill et al. (2015) show that firms must act in the best interest of all stakeholders, i.e., firms’ consumers, workers, and shareholders instead of only shareholders. This is because the firm faces endogenous shocks influenced by its investments, and these shocks create externalities on consumers and workers of the firm. Because of these externalities, the competitive equilibrium is suboptimal. The competitive equilibrium becomes Pareto optimal, however, if the firm adopts the stakeholder criterion, which takes into account shareholders as well as consumers and workers. In the present paper, shareholders of firms are also consumers and workers of firms, and yet, the competitive equilibrium is suboptimal, because endogenous shocks driven by collective actions of managers create externalities in managers’ wages and in the equity market. While Magill et al. (2015) consider a simple economy with a single firm, we consider a general economy with many firms competing on the product and labor markets. While Magill et al. (2015) argue that taxes cannot be used to internalize the externalities, because the firm’s investments are unobservable, we show that if managers’ actions are unobservable, equity taxes can internalize the externalities in the equity market, and hence, imposition of equity taxes makes Pareto improvements.

The remainder of this paper is organized as follows. In Sect. 2, we elaborate on model ingredients and define competitive equilibrium. In Sect. 3, we analyze the existence and optimality of competitive equilibrium, managers’ wages, and taxes, and extend the model by incorporating information asymmetry. In Sect. 4, we conclude with a brief discussion. We present all proofs in Appendix.

2 Model

Consider an infinite-time horizon, stochastic overlapping-generations model in which agents become economically active at the age of 20 and live for two periods, each of which spans 30 years. Agents become young in the first periods of their lives and old in the second periods. At each period, new young agents are born, and young agents of the previous period become old agents. There is a continuum of identical agents and a continuum of identical firms, so the impact of each agent (he) or each firm (it) on prices is negligible.

Agents. To reflect the natural life-cycle division of labor, young agents provide unskilled labor $a_{y}$, whereas old agents provide skilled labor $a_{o}$. By the same reasoning, while young agents serve as line workers, old agents serve as risk managers. Throughout this paper, we shall use “old agents” and “managers” interchangeably. We focus on managers’ risk management activities, and normalize the management of other activities to zero to isolate the impact of risk management activities. Young agents earn unit wages $w_{y}$, and old agents earn unit wages $w_{o}$ in exchange for their labor inputs. Young agents supply their labor inelastically. Old agents choose their labor supply by taking into account disutility from labor, and their disutility function $\phi :{\mathbb {R}}_{+}\rightarrow {\mathbb {R}}_{+}$ is increasing and strictly convex in their labor inputs $a_{o}$. We shall use “labor inputs” and “actions” interchangeably throughout this paper.

Agents hold ownership shares of firms, i.e., equities. Equity e is a productive asset, and the supply of equity is fixed and normalized to one. Old agents initially possess equities,² but they sell these equities to young agents at a price p. At each period, equity holders earn a dividend $\delta$, which takes on high (H) or low (L) value, i.e., $\delta \in \{\delta ^{H},\delta ^{L}\}$, where $\delta ^{H}>\delta ^{L}$. Agents’ preferences are given by a utility function $U=u(c_y)+E[u(c_{o})-\phi (a_{o})]$, where $c_{y}$ and $c_{o}$ are consumptions when young and old, respectively; $u:{\mathbb {R}}_{+}\rightarrow {\mathbb {R}}$ is twice continuously differentiable, strictly increasing and strictly concave in c, and satisfy Inada conditions. Agents face budget constraint $c_{y}\le a_{y}w_{y}-pe$ when they are young and $c_{o}\le a_{o}w_{o}+(p+\delta )e$ when they are old wherein the consumption good is numeraire, so its price is normalized to one.

Firms. Firms produce the consumption good using agents’ labor inputs and in return, pay wages to agents. Firms engage in production processes determined by two factors: a deterministic and a stochastic component. The deterministic component is represented by a production function f $:{\mathbb {R}}_{+}\rightarrow {\mathbb {R}}_{+}$ which uses young agent’s labor input $a_{y}$, and is increasing and concave in $a_{y}$. The stochastic component is represented by an individual output shock z, which takes on high (H) or low (L) value, i.e., $z\in \{z^{H},z^{L}\}$, where $z^{H}>z^{L}$. The addition of the stochastic and the deterministic components is equal to the total output $\gamma$, i.e., $\gamma =z+f(a_{y})$. The total output $\gamma$ and production $f(a_{y})$ are observable, so the output shock z is also observable. Each firm maximizes its profit $P=E[\gamma ]-a_yw_y-a_ow_o$ in which the consumption good is numeraire, and hence, its price is normalized to one.

As we discuss in ”Introduction”, managers’ actions may affect aggregate shocks. The most precise way of modeling it would be that each manager’s actions drive his own firm’s idiosyncratic shocks, and these idiosyncratic shocks produce aggregate shocks. However, such a model would create infinitely many types of firms under infinite-time horizon and hence would be intractable. To keep the model tractable, we assume that individual output shocks are (perfectly) correlated across firms, which in turn generate aggregate shocks. These aggregate shocks are driven by the collective actions of managers—in particular, probabilities of possible outcomes are influenced by the actions $\widehat{a_o}$ of all managers in the economy. The probability function of high output shock $\pi :{\mathbb {R}}_{+}\rightarrow [0,1]$ is increasing and concave in $\widehat{a_o}$. Note that output shocks of different periods are independent but not necessarily identically distributed, because when $\widehat{a_o}$ differs across periods, $\pi (\widehat{a_o})$ differs, and hence, the probability distributions across periods are non-identical.

Although we assume perfectly correlated shocks for firms, we allow different beliefs for firms to mimic the impact of idiosyncratic shocks on firms’ decisions. Each firm believes that the risk it faces is a convex combination of the risk managed by the actions $a_o$ of its own managers and the risk managed by the actions $\widehat{a_o}$ of all managers in the economy, i.e., by the state of the economy. In particular, each firm believes that the probability of observing high output shock is $\rho \pi (a_o)+(1-\rho )\pi (\widehat{a_o})$, where $\rho$ $(\in [0,1])$ is an exogenously given weight. Each firm can control its own managers’ actions $a_o$ by adjusting their wages $w_o$, but cannot control all managers’ actions $\widehat{a_o}$. Thus, our model encompasses all possible beliefs for firms ranging from full control (i.e., $\rho =1$) to no control (i.e., $\rho =0$) over the probability distribution of firms’ shocks. In a rational-expectations competitive equilibrium, every manager of the same period will take the same action $a_o=\widehat{a_o}$, which will in turn yield the following probability for high output shock according to the beliefs of firms:

$$\begin{aligned} \left. \rho \pi (a_o)+(1-\rho )\pi (\widehat{a_o})\right| _{a_o=\widehat{a_o}}= \pi (\widehat{a_o}). \end{aligned}$$

Note that $\pi (\widehat{a_o})$ is the actual probability of high output shock in equilibrium, so firms’ beliefs are consistent with the actual probability in equilibrium. Thus, each $\rho$ yields a rational-expectations competitive equilibrium as we show later in our analysis.

Table 1 Timeline

Timeline. As shown in Table 1, the sequence of events is as follows. First, new young agents are born, and young agents of the previous period become old. Second, young and old agents’ labor inputs $a_{y}$ and $a_{o}$ and wages $w _{y}$ and $w _{o}$ are determined, and hence, labor markets clear. Third, output shock z is realized. Finally, depending on the output shock, young and old agents’ consumptions $c_{y}$ and $c_{o}$, equity e, its price p, and dividend $\delta$ are determined. Thus, the consumption good and equity markets clear.

Equilibrium. Before defining a rational-expectations competitive equilibrium, we discuss each agent’s and firm’s optimization problems and market-clearing conditions. Each agent solves the following problem:

$$\begin{aligned}&\underset{c_y^s,c_o^s,e^s,a_o}{\max }\quad u(c_{y}^s)+\pi (\widehat{a}_o) u(c_o^H)+(1-\pi (\widehat{a}_o))u(c_o^L)-\phi (a_o) \end{aligned}$$

(1)

$$\begin{aligned}&\qquad \; \text {s.t.}\quad \;\, c_{y}^{s}\le a_{y}w _{y}-p^{s}e^s \end{aligned}$$

(2)

$$\begin{aligned}&\qquad \qquad \quad \;\, c_{o}^s\le a_{o}w _{o}+(p^{s}+\delta ^{s})e^{s},\quad \text {where}\;\; s \in \{H,L\}. \end{aligned}$$

(3)

The objective of each agent given in (1) is to maximize his expected life-time utility by choosing his consumption $c_y^s$ when young and $c_o^s$ when old, equity demand $e^s$, and labor input $a_{o}$ when old. Note that the agent does not choose his labor input $a_y$ when young, because he supplies his labor inelastically when he is young. Moreover, each agent takes the probability of high shock (and of low shock), given that because this probability is influenced by the actions $\widehat{a}_o$ of all (old) agents in the economy, and a single agent has a negligible effect on this probability. Budget constraints (2) and (3) ensure that each agent’s consumption does not exceed his income when he is young and old, respectively. Similarly, each firm solves the following problem:

$$\begin{aligned}&\underset{a_{y},a_{o}}{\max }[\rho \pi (a_{o})+(1-\rho )\pi (\widehat{a_o})]z^{H}+[1-\rho \pi (a_{o})-(1-\rho )\pi (\widehat{a_o})]z^{L}\nonumber \\&\quad +f(a_{y})-a_{y}w _{y}-a_{o}w _{o}\text {.} \end{aligned}$$

(4)

The objective of each firm given in (4) is to maximize its expected profit by choosing its labor demand $a_y$ for young and $a_o$ for old agents.

In addition to conditions stemming from agents’ and firms’ optimization problems, a rational-expectations competitive equilibrium satisfies market-clearing conditions for the consumption good, equity, and labor markets as well. The market-clearing condition for the consumption good is $c_{y}^s+c_{o}^s\le \gamma ^s$, where $s \in \{H,L\}$. This condition ensures that the total consumption of young and old agents does not exceed the total output. Equity market clears when the demand for equity is equal to the supply of equity. Because the supply of equity is fixed and normalized to one, the equity market-clearing condition is $e^s=1$, where $s \in \{H,L\}$. Moreover, dividend is equal to the remaining output after wages are paid, so dividend $\delta ^s=\gamma ^s-a_{y}w_{y}-a_{o} w_{o}$, where $s \in \{H,L\}$. Labor market clears when the demand for labor is equal to the supply of labor. Because young agents supply their labor inelastically, the labor market-clearing condition for young is $a_y=\overline{a_y}$. We next define a rational-expectations competitive equilibrium as follows.

Definition 1

A rational-expectations competitive equilibrium $\{\widehat{c}_y^s, \widehat{c}_o^s, \widehat{p}^s, \widehat{\delta }^s, \widehat{e}^s, \widehat{w}_y,\widehat{w}_o, \widehat{a}_y, \widehat{a}_o\}$ is such that $\{\widehat{c}_y^s, \widehat{c}_o^s, \widehat{e}^s, \widehat{a}_o\}$ solves (1)–(3) and $\{\widehat{a}_y,\widehat{a}_o\}$ solves (4) given $\widehat{c}_y^s+\widehat{c}_o^s=\widehat{\gamma }^s$, $\widehat{\delta }^s=\widehat{\gamma }^s -a_yw_y-a_ow_o$, $\widehat{e}^s=1$, and $\widehat{a}_y=\overline{a_y}$, where $s \in \{H,L\}$.

3 Analysis

3.1 Existence of equilibrium

As is common in the literature (e.g., Peled 1982; Aiyagari and Peled 1991), we restrict attention to strongly stationary equilibrium, because such an equilibrium allows us to derive analytical results, and it facilitates the interpretation of these results. Under a strongly stationary rational-expectations competitive equilibrium, endogenous variables depend only on the current realization of output shocks, so they do not depend on past realizations or lagged endogenous variables. We prove the existence of a strongly stationary rational-expectations competitive equilibrium in Proposition 1. Note that proofs of all propositions are presented in Appendix.

Proposition 1

For all $\rho \in [0,1]$, there exists a strongly stationary rational-expectations competitive equilibrium allocation.

Given this existence result, we focus on strongly stationary equilibrium for the rest of this paper.

3.2 Optimality of equilibrium

Up to now, we have discussed rational-expectations competitive equilibrium in which each agent solves his utility maximization problem individually. We next shift our focus to the efficiency of rational-expectations competitive equilibrium. To this end, we will examine the planner’s problem, wherein the social planner takes actions on behalf of all agents in the economy. In particular, the planner maximizes the weighted average of young and old agents’ expected utilities by choosing consumption $c_y$ for young and $c_o$ for old agents and labor input $a_o$ for old agents. Note that the planner does not choose labor input $a_y$ for young agents, because young agents supply their labor inelastically, i.e., $a_y=\overline{a_y}$. The planner is subject to a resource constraint which ensures that the total consumption of young and old agents does not exceed the total output. Given a Pareto weight $\alpha \in [0,1]$, a Pareto optimal allocation $\{c_{y}^{H,*}$, $c_{y}^{L,*}$, $c_{o}^{H,*}$, $c_{o}^{L,*}$, $a_o^*\}$ is a solution to the following planner’s problem:

$$\begin{aligned} \max _{c_{y}^{s},c_{o}^{s},a_{o}}&(1-\alpha )E[u(c_y)]+\alpha E[u(c_o)-\phi (a_o)] \end{aligned}$$

(5)

$$\begin{aligned} \text { subject to }&c_{y}^{s}+c_{o}^{s}\le \gamma ^{s},\quad \text {where}\ s\in \{H,L\}. \end{aligned}$$

(6)

We prove the inefficiency of rational-expectations competitive equilibrium allocation in the proposition below.

Proposition 2

The rational-expectations competitive equilibrium allocation is not Pareto optimal.³

As shown in Proposition 2, old agents’ collective risk management activities render rational-expectations competitive equilibrium inefficient. To restore efficiency, we first need to understand the source of inefficiency. The source of inefficiency is the externalities created by old agents’ collective risk management activities. On the firm side, when $\rho <1$, firms believe that the impact of their managers’ actions through the state of the economy is external to them, so firms will not compensate their managers for this impact.⁴ These uncompensated labor inputs of managers create an externality on the firm side. On the agent side, collective actions of old agents determine the state of the economy, and the state of the economy in turn affects consumption of young (through equity prices) and old agents (through old agents’ wages, equity prices, and dividends). However, while choosing his action, an individual old agent does not consider that old agents’ collective actions affect their (and young agents’) consumption through the state of the economy. Thus, the impact of old agents’ collective actions through the state of the economy is external to an individual old agent, which results in an externality on the agent side as well.

To attain socially optimal allocations, the externalities created by old agents’ collective risk management activities must be internalized. To do so, the social planner needs old agents to take socially optimal actions $a_o^*$ that consider both internal and external effects of old agents’ actions. To implement socially optimal actions $a_o^*$, old agents must be paid socially optimal wages $w_o^*$. To ensure that old agents are paid socially optimal wages $w_o^*$, the social planner must impose wage taxes $t_w$, which are equal to old agents’ competitive wages minus optimal wages, given that old agents take socially optimal actions $a_o^*$. Similarly, the social planner must impose equity tax $t^s$ (for $s\in \{H,L\}$), so that the externalities in the equity market will be internalized.

Theorem 1

To attain a socially optimal rational-expectations competitive equilibrium, old agents must be paid socially optimal wages $w_{o}^{*}=\frac{(1-\alpha )\pi '(a_o^*)[u(c_{y}^{H,*})-u(c_y^{L,*})] +\alpha \pi '(a_o^*)[u(c_o^{H,*})-u(c_o^{L,*})] }{\alpha [\pi (a_o^*)u'(c_o^{H,*})+(1-\pi (a_o^*))u(c_o^{L,*})]}$. A Pareto optimal allocation $\{c_{y}^{H,*}$, $c_{y}^{L,*}$, $c_{o}^{H,*}$, $c_{o}^{L,*}$, $a_{o}^{*}\}$ can be implemented if the social planner imposes wage tax $t_{w}=\rho \pi '(a_{o}^{*})(z^{H}-z^{L})-w _{o}^*$ and equity tax $t^{s}=\frac{E[u'(c_{o}^{*})(p^{*}+\delta ^*)]-u'(c_{y}^{s,*})p^{s,*}}{u'(c_{o}^{s,*})}$ for $s \in \{H,L\}$.

3.3 Numerical analysis

We next numerically analyze old agents’ competitive and optimal wages.⁵ In particular, we examine how the ratio of high shock to low shock $z^{H}/z^{L}$ and exogenous weight $\rho$ in firms’ profit functions affect old agents’ competitive and optimal wages. For this numerical analysis, we use the following functional forms. Each agent’s preferences are specified by a logarithmic utility function, so $u(c)=\mathrm{log}(c)$, where c is consumption of a young or old agent. Each old agent’s disutility function is of the form $\phi (a_{o})=a_{o}^{g}$, where $g>1$. Each firm’s production function is of the form $f(\overline{a_{y}})=\overline{a_{y}}$. The probability function of high output shock is of the form $\pi (a_{o})=1-\frac{1}{(1+a_{o})^{b}}$. Note that all functions satisfy the standard assumptions stated in Sect. 2, e.g., $\pi (0)=0$, $\lim _{a_o\rightarrow \infty }\pi (a_{o})=1$.

Fig. 1

Old agents’ competitive and optimal wages as a function of the ratio of high shock to low shock $z^{H}/z^{L}$ and weight $\rho$ (which represents firms’ beliefs about how much of the risk they face is controlled by the actions of their own old agents), where young agent’s labor input $\overline{a_{y}}=10$, exponent of old agent’s disutility function $g=2$, probability function exponent $b=2$, and Pareto weight $\alpha =0.5$

Figure 1a illustrates that as the ratio of high shock to low shock $z^{H}/z^{L}$ increases, old agents’ both optimal and competitive wages increase, but optimal wages increase more. When high shock is equal to low shock (i.e., $z^{H}=z^{L}$), firms do not face any risk that old agents can manage, so old agents’ competitive wages $w_o=0$. As the gap between high shock and low shock enlarges (i.e., $z^H/z^L$ rises), however, marginal contributions of old agents’ actions to their firms increase, and hence, old agents’ competitive wages increase. Moreover, as the gap between high shock and low shock widens (i.e., $z^H/z^L$ rises), marginal contributions of old agents’ actions to the state of the economy increase as well. Because old agents’ optimal wages $w_o^*$ consider both internal and external effects of old agents’ actions, old agents’ optimal wages increase more than their competitive wages as the gap between high shock and low shock enlarges (i.e., $z^H/z^L$ rises).

Figure 1b depicts that as the exogenous weight $\rho$ (which represents firms’ beliefs about how much of the risk they face is controlled by the actions of their own old agents) increases, old agents’ optimal wages do not change while competitive wages increase. As $\rho$ increases, firms believe that a higher portion of the risk they face is controlled by the actions of their own old agents, and hence, firms pay higher wages to their old agents (i.e., competitive wages rise). On the other hand, optimal wages $w_o^*$ implement socially optimal actions $a_o^*$ for old agents, and these actions are determined by the social planner, so they do not depend on firms’ beliefs. Thus, as $\rho$ approaches one, old agents’ competitive wages get closer to their optimal wages.

3.4 Information asymmetry

So far, we have analyzed the model in which managers’ actions and unit wages are observable by the social planner. However, in the real world, managers’ actions $a_o$ and unit wages $w_o$ may not be observable to the social planner, so we extend the model by incorporating unobservable actions and unobservable unit wages for managers.

As we have discussed after Proposition 2, a rational-expectations competitive equilibrium is suboptimal because of the externalities in old agents’ wages and in equity market. The former can be internalized by imposing wage taxes (on old agents) which are equal to old agents’ competitive wages minus optimal wages. When old agents’ competitive wages $w_o$ (and labor inputs $a_o$) are unobservable, however, the social planner cannot impose wage taxes on old agents, so cannot implement a Pareto optimal allocation. However, the social planner can make Pareto improvements by implementing a second-best allocation. Because the labor input $a_o$ cannot be observed by the social planner, it will be determined in the market, where the demand for old agents’ labor [(24) in Appendix] is equal to the supply of old agents’ labor [(20) in Appendix]. Substituting the labor demand into the labor supply, we obtain the following condition:

$$\begin{aligned} \big [ \pi (a_{o})u'(c_{o}^{H})+(1-\pi (a_{o}))u'(c_{o}^{L})\big ] \rho \pi '(a_{o})(z^{H}-z^{L})-\phi '(a_{o})=0. \end{aligned}$$

(7)

To ensure that the labor market condition (7) is satisfied, we add it as a constraint to the planner’s problem (5), (6). Given a Pareto weight $\alpha \in [0,1]$, a second-best allocation $\{\tilde{c}_y^H,\tilde{c}_y^L,\tilde{c}_o^H,\tilde{c}_o^L,\tilde{a}_o \}$ is a solution to the following planner’s problem:

$$\begin{aligned} \max _{c_y^s,c_o^s,a_o}&(1-\alpha )E[ u(c_{y})] +\alpha (E[u(c_o)]-\phi (a_{o})) \end{aligned}$$

(8)

$$\begin{aligned} \text {subject to}&c_y^s+c_o^s\le \gamma ^s \end{aligned}$$

(9)

$$\begin{aligned}&\big [\pi (a_{o})u'(c_{o}^{H})+(1-\pi (a_{o}))u'(c_{o}^{L}) \big ] \rho \pi '(a_{o})(z^{H}-z^{L})-\phi '(a_{o})=0.\nonumber \\ \end{aligned}$$

(10)

When old agents’ unit wages $w_o$ and labor inputs $a_o$ are unobservable, the externalities in old agents’ wages cannot be internalized, so a Pareto optimal allocation cannot be implemented. However, a second-best allocation can be implemented if the externalities in the equity market are internalized by imposing an equity tax $t^s$, where $s\in \{H,L\}$.

Proposition 3

If the social planner imposes equity tax

$$\begin{aligned} t^{s}=\frac{E[u'(\tilde{c}_o)(\tilde{p}+\tilde{\delta })]-u'(\tilde{c}_y^s)\tilde{p}^s}{u'(\tilde{c}_o^s)}\quad \text { for}\ s\in \{H,L\}, \end{aligned}$$

a second-best allocation $\{\tilde{c}_y^H,\tilde{c}_y^L,\tilde{c}_o^H,\tilde{c}_o^L,\tilde{a}_o \}$ can be implemented.

Fig. 2

Ratio of social welfare under second best to social welfare under Pareto optimal $u^\mathrm{SB}/u^\mathrm{PO}$ as a function of the ratio of high shock to low shock $z^{H}/z^{L}$, where exponent of old agent’s disutility function $g=2$, probability function exponent $b=2$, and Pareto weight $\alpha =0.5$

We next numerically analyze social welfare under a second-best allocation compared to social welfare under a Pareto optimal allocation. Figure 2 demonstrates that as the ratio of high shock to low shock $z^{H}/z^{L}$ increases, social welfare under a second-best allocation diverges from social welfare under a Pareto optimal allocation. When high shock is equal to low shock (i.e., $z^{H}=z^{L}$), firms do not face any risk that old agents can manage, and hence old agents’ competitive wages $w_o=0$ (see Fig. 1a). Then, failing to observe $w_o$ (and labor input $a_o$) is not costly, so social welfare under second best reaches social welfare under Pareto optimal. As the gap between high shock and low shock widens (i.e., $z^H/z^L$ rises), however, old agents’ wages $w_o$ are positive (see Fig. 1a), so failing to observe $w_o$ (and $a_o$) becomes costly, and hence, social welfare under second best diverges from social welfare under Pareto optimal.

4 Conclusion

We have studied how endogenous output shocks driven by collective actions of managers impact social welfare. We prove the inefficiency of rational-expectations competitive equilibrium in the presence of such endogenous shocks. The source of inefficiency is the externalities in managers’ wages and in equity market. We establish that to attain a socially optimal allocation, these externalities must be internalized by imposing wage taxes (or subsidies) on managers and equity taxes. Our results provide an alternative explanation as to why managers are compensated and taxed differently than other workers. It may be optimal to give managers wage tax subsidies because of the (positive) externalities their collective actions create. We then extend the model by incorporating unobservable actions for managers and show that a second-best allocation can be implemented by imposing equity taxes.

There are several avenues for future research. First, although we consider identical agents and identical firms in this paper, it would be an interesting extension to incorporate heterogeneity. For example, the economy is populated with a few big firms, whose managers’ actions affect the state of the economy and with many small firms, whose managers’ actions do not affect the state of the economy. In this case, one can examine how the actions of big firms’ managers affect managers and low-skilled workers at small firms, and how such externalities can be internalized. Second, our model suggests imposing wage taxes (or subsidies) on managers to attain socially optimal allocations. On the other hand, it is well known that the drastic increase in managerial compensation over a couple of decades is an important determinant of the income distribution inequality. In this case, an interesting avenue would be to investigate how wage taxes (or subsidies) on managers influence the income distribution inequality.

Appendix

Proof of Proposition 1

We will prove the existence of a strongly stationary rational-expectations competitive equilibrium. Given twice continuously differentiable utility function u, the resulting consumption demands $c_{y}[ p,a_{y}]$ and $c_{o}[ p^{H},p^{L},\delta ^{H},\delta ^{L}]$, the optimal labor input $a_{o}[ p^{H},p^{L},\delta ^{H},\delta ^{L}]$ when old, and the demand for equity $e[p^{H}, p^{L},\delta ^{H},\delta ^{L}]$ are continuous functions of prices and dividends (and of shock parameters), so utility function (1) is continuous. The constraint set (2), (3) is convex and compact. Thus, there exists a solution to agent’s utility maximization problem (1)–(3) by Weierstrass Theorem. Similarly, profit function (4) is continuous and its domain is compact, so there exists a solution to firm’s profit maximization problem (4) by Weierstrass Theorem.

The utility function u is concave in consumptions $c_y^H$, $c_y^L$, $c_o^H$, and $c_o^L$, and the disutility function $\phi$ is convex in labor input $a_o$. Every term in utility function U given in (1) appears in additive form, so Hessian of U is a diagonal matrix. The diagonal entries of Hessian are $\frac{\partial ^2 U}{\partial (c_y^H)^2}=u''(c_y^H)<0$, $\frac{\partial ^2 U}{\partial (c_y^L)^2}=u''(c_y^L)<0$, $\frac{\partial ^2 U}{\partial (c_o^H)^2}=\pi (\widehat{a}_o)u''(c_o^H)<0$, and $\frac{\partial ^2 U}{\partial (c_o^L)^2}=(1-\pi (\widehat{a}_o))u''(c_o^L)<0$ and $\frac{\partial ^2 U}{\partial a_o^2}=-\phi ''(a_o)<0$. Thus, Hessian of U is negative definite, and hence, U is concave. Since agent’s optimization problem (1)–(3) is a concave programming, its first-order conditions are sufficient for optimality and yield unique solution. Similarly, the production function f is concave in young agent’s labor input $a_y$ and the probability function $\pi$ is concave in old agent’s labor input $a_o$. Every term in profit function P given in (4) appears in additive form, so Hessian of P is a diagonal matrix. The diagonal entries of Hessian are $\frac{\partial ^2 P}{\partial a_y^2}=f''(a_y)<0$ and $\frac{\partial ^2 P}{\partial a_o^2}=\rho \pi ''(a_o)(z^H-z^L)<0$. Note that because there is a continuum of agents, a single old (respectively, young) agent’s action $a_o$ (respectively, $a_y$) does not affect his unit wage $w_o$ (respectively, $w_y$). Hence, Hessian of P is negative definite, so P is concave. Because firm’s optimization problem (4) is a concave programming, its first-order conditions are sufficient for optimality and yield unique solution.

Via the solutions to the firm’s problem and the definition of dividends, we can substitute into the agent’s demand functions and eliminate the need to consider the supply side of the economy. Let $\zeta ^{s}_y$ be the excess demand of the young agent born in state $s\in \{H,L\}$ and $\zeta _o^s$ be the excess demand of the old agent when the current state is $s\in \{H,L\}$, such that

$$\begin{aligned}&\zeta _{y}^{s}=c_{y}^{s}[ p^{s}] -a_{y}w _{y} \end{aligned}$$

(11)

$$\begin{aligned}&\zeta _{o}^{s}=c_{o}^{s}[ p^{H},p^{L}] -a_{o}w _{o}. \end{aligned}$$

(12)

Note that equity $e^s=1$ in equilibrium, and the demand of the old agent cannot depend on the state he is born in. We then define aggregate excess demand:

$$\begin{aligned} \zeta ^{s}=\zeta _{y}^{s}+\zeta _{o}^{s},\quad \text {where}\; s\in \{H,L\}. \end{aligned}$$

(13)

Moreover, equilibrium requires that $\zeta ^{s}-\delta ^{s}=0$.

To prove the existence of an equilibrium, we first show that prices must be bounded above zero and below infinity. Using sequential budget constraints (11) and (12), we get

$$\begin{aligned} \zeta _{y}^{s}= & {} -p^{s}e^s \end{aligned}$$

(14)

$$\begin{aligned} \zeta _{o}^{s}= & {} ( p^{s}+\delta ^{s})e^{s}. \end{aligned}$$

(15)

Substituting equity $e^s$ in (15) into (14), we obtain the agent’s intertemporal budget constraint

$$\begin{aligned} \zeta _{y}^{s}+q^{ss'}\zeta _{o}^{s'}=0 \end{aligned}$$

(16)

the present-value price of consumption

$$\begin{aligned} q^{ss'}=\frac{p^{s}}{p^{s'}+\delta ^{s'}},\quad \text {where} \; s\in \{H,L\}\quad \text {and}\; s'\in \{H,L\}. \end{aligned}$$

(17)

If $p^{s}\rightarrow 0$ (regardless of how $p^{s'}$ changes), we have $q^{ss'}\rightarrow 0$ from (17), and the old agent can afford unlimited consumption, and hence we have $\zeta _{o}^{s}\rightarrow \infty$ from (16). As $p^{s}\rightarrow 0$, we have $\zeta _{y}^{s}\rightarrow 0$ from (14), so we have $\zeta ^{s}\rightarrow \infty$ from (13). Next, if $p^{s}\rightarrow \infty$ while $p^{s'}$ is bounded, we have $q^{ss'}\rightarrow \infty$ from (17), so $\zeta _{o}^{s}\rightarrow 0$ from (16). As $p^{s}\rightarrow \infty$, we have from (14) either $\zeta _{y}^{s}\rightarrow -\infty$ if we allow unlimited short-sales of consumption, or, more realistically, $\zeta _{y}^{s}<0$ if we restrict consumption to be non-negative. In either case, we have $\zeta ^{s}<0$ from (13). Finally, if both $p^{s}\rightarrow \infty$ and $p^{s'}\rightarrow \infty$ simultaneously, we have $q^{ss'}\rightarrow 1$ from (17), which then implies that $\zeta ^{s}\rightarrow 0$ from (13) and (16).

We restrict prices $p^{s}$ to lie in a square $B=[ p_{\min },p_{\max }] \times [ p_{\min },p_{\max }]$ with the minimum and maximum prices chosen, so that any equilibrium price must lie in the box, if it exists. We then define a mapping $\nu :B\rightarrow {\mathbb {R}}^{2}$, such that

$$\begin{aligned} \nu ( p^{H},p^{L}) =\left[ \begin{array}{c}p^{H} \\ p^{L} \end{array}\right] +\left[ \begin{array}{c}\zeta ^{H}-\delta ^{H} \\ \zeta ^{L}-\delta ^{L}\end{array}\right] =\tau +Z \end{aligned}$$

The mapping $\nu$ naturally defines a vector field on B via $Z=\nu ( p^{H},p^{L}) -\tau$. We now apply the Poincare–Hopf Theorem to this vector field. Note that from our limit calculations above, if any price gets small, the Z vector will be positive; if any price gets large, the Z vector will become negative; and if both prices become large (but finite) simultaneously, Z will also become negative. Hence, the vector field points into B on $\partial B$, so the Poincare–Hopf Theorem applies, and the number of zeros mod 2 of the vector field in B must be equal to the Euler characteristic of B. Since B is homeomorphic to a two-simplex, its Euler characteristic is 1. Thus, there must be at least one zero for Z in the interior of B,  and this is clearly an equilibrium for the model. Note that in the entire proof, endogenous variables do not depend on past realizations of output shocks or on lagged variables. Therefore, there exists a strongly stationary rational-expectations competitive equilibrium. $\square$

Proof of Proposition 2

We will prove that a rational-expectations competitive equilibrium is not Pareto optimal. To do so, we will first derive a rational-expectations competitive equilibrium equations and Pareto optimality equations.

A rational-expectations competitive equilibrium satisfies market-clearing conditions and first-order conditions stemming from agents’ and firms’ optimization problems. After substituting the market-clearing conditions $\widehat{e}^s=1$ and $\widehat{a}_y=\overline{a_y}$, first-order conditions of agents’ optimization problem (1)–(3) are

$$\begin{aligned} \frac{\partial U}{\partial e^H}= & {} -u'(\widehat{c}_{y}^{H})\widehat{p}^H+\pi (\widehat{a}_o)u'(\widehat{c}_o^H)(\widehat{p}^H+\widehat{\delta }^H)\nonumber \\&+\,(1-\pi (\widehat{a}_o))u'(\widehat{c}_o^L)(\widehat{p}^L+\widehat{\delta }^{L}) =0 \end{aligned}$$

(18)

$$\begin{aligned} \frac{\partial U}{\partial e^L}= & {} -u'(\widehat{c}_{y}^{L})\widehat{p}^{L}+\pi (\widehat{a}_o)u'(\widehat{c}_o^H)(\widehat{p}^{H}+\widehat{\delta }^{H})\nonumber \\&+\,(1-\pi (\widehat{a}_o))u'(\widehat{c}_o^L)(\widehat{p}^{L}+\widehat{\delta }^{L})=0 \end{aligned}$$

(19)

$$\begin{aligned} \frac{\partial U}{\partial a_o}= & {} \big [\pi (\widehat{a}_o)u'(\widehat{c}_o^H)+ (1-\pi (\widehat{a}_o))u'(\widehat{c}_o^L)\big ]\widehat{w}_o-\phi ' (\widehat{a}_o)=0 \end{aligned}$$

(20)

$$\begin{aligned} \widehat{c}_y^s= & {} \overline{a_y}\widehat{w}_y-\widehat{p}^s,\quad \text {where}\; s\in \{H,L\} \end{aligned}$$

(21)

$$\begin{aligned} \widehat{c}_o^{s'}= & {} \widehat{a}_o \widehat{w}_o+\widehat{p}^{s'}+\widehat{\delta }^{s'},\quad \text {where}\; s'\in \{H,L\}. \end{aligned}$$

(22)

After substituting $\widehat{a}_y=\overline{a_y}$, first-order conditions of firms’ optimization problem (4) are

$$\begin{aligned}&\frac{\partial P}{\partial a_y}=f'(\overline{a_y})-\widehat{w}_y=0 \end{aligned}$$

(23)

$$\begin{aligned}&\frac{\partial P}{\partial a_o}=\rho \pi '(\widehat{a}_o)( z^{H}-z^{L}) -\widehat{w}_o=0. \end{aligned}$$

(24)

The remaining market-clearing conditions are

$$\begin{aligned}&\widehat{\delta }^s=\widehat{\gamma }^s-\overline{a_y}\widehat{w}_y-\widehat{a}_o\widehat{w}_o \end{aligned}$$

(25)

$$\begin{aligned}&\widehat{c}_y^s+\widehat{c}_o^s=\widehat{\gamma }^s,\quad \text {where}\; s\in \{H,L\}. \end{aligned}$$

(26)

Because (21), (22), and (25) together imply (26), rational-expectations competitive equilibrium equations are (18)–(25). We then derive the equations that a Pareto optimal allocation satisfies. Letting $\lambda ^s \ge 0$ be the Lagrange multiplier of (6), the first-order conditions of the planner’s problem (5), (6) are

$$\begin{aligned}&(1-\alpha )\pi (a_o^*)u'(c_y^{H,*})-\lambda ^{H}=0 \end{aligned}$$

(27)

$$\begin{aligned}&(1-\alpha )(1-\pi (a_o^*))u'(c_y^{L,*})-\lambda ^L=0 \end{aligned}$$

(28)

$$\begin{aligned}&\alpha \pi (a_o^*)u'(c_o^{H,*})-\lambda ^{H}=0 \end{aligned}$$

(29)

$$\begin{aligned}&\alpha (1-\pi (a_o^*))u'(c_o^{L,*})-\lambda ^{L}=0 \end{aligned}$$

(30)

$$\begin{aligned}&(1-\alpha )\pi '(a_o^*)\big [u(c_y^{H,*})-u(c_y^{L,*})\big ]+\alpha \big [\pi '(a_o^*) \big [u(c_o^{H,*})-u(c_o^{L,*})\big ]-\phi '(a_o^*)\big ]=0 \end{aligned}$$

(31)

$$\begin{aligned}&c_y^{s,*}+c_o^{s,*}=\gamma ^{s,*},\quad \text {where}\; s\in \{H,L\}. \end{aligned}$$

(32)

Suppose to the contrary that rational-expectations competitive equilibrium allocation is Pareto optimal. We let $\{\widehat{c}_y^s, \widehat{c}_o^s, \widehat{p}^s, \widehat{\delta }^s, \widehat{e}^s, \widehat{w}_y, \widehat{w}_o, \widehat{a}_y, \widehat{a}_o\}$ be a rational-expectations competitive equilibrium. Then, $\{\widehat{c}_y^s, \widehat{c}_o^s, \widehat{p}^s, \widehat{\delta }^s, \widehat{e}^s, \widehat{w}_y, \widehat{w}_o, \widehat{a}_y, \widehat{a}_o\}$ satisfies the rational-expectations competitive equilibrium equations (18)–(25) by definition, and there is a Pareto weight $\alpha \in [0,1]$, such that $\{\widehat{c}_y^s, \widehat{c}_o^s, \widehat{p}^s, \widehat{\delta }^s, \widehat{e}^s, \widehat{w}_y, \widehat{w}_o, \widehat{a}_y, \widehat{a}_o\}$ satisfies Pareto optimality equations (27)–(32). Because (21), (22), and (25) together imply (32), it suffices to show that (27)–(31) are satisfied. Given the market-clearing conditions $\widehat{e}^s=1$ and $\widehat{a}_y=\overline{a_y}$, we have a system of 16 equations: (18)–(25) and (27)–(31) and 14 variables: $c_y^s$, $c_o^s$, $p^s$, $\delta ^s$, $\lambda ^s$, $w_y$, $w_o$, $a_o$, $\alpha$, where $s\in \{H,L\}$. If these equations were dependent, we could have a Pareto optimal rational-expectations competitive equilibrium. However, a routine application of the multi-jet transversality theorem will establish that generically, there are no solutions to over-determined system of equations. An even easier way to show this is outlined below.

We want to define a smooth perturbation of the young agent’s utility function on a small neighborhood of his consumption $c_{y}^{H}$ in the high state. We define the neighborhood as the interval $[c_{y}^{H}-b,c_{y}^{H}+b]$. Furthermore, within this interval, we define a sub-interval $[c_{y}^{H}-a,c_{y}^{H}+a]$ , where $b>a>0$. For the remainder of this calculation, we will re-center the coordinates so that $c_{y}^{H}=0.$ We now use a bump function $\eta (x)$ as defined on page 42 in Hirsch (1976) to construct a perturbation on the neighborhood of the utility function as

$$\begin{aligned} \tilde{u}( x)= & {} [ 1-\eta ( x) ] u( x) +\eta ( x) [ u( x) +\varepsilon ]\\= & {} u( x) +\eta ( x) \varepsilon . \end{aligned}$$

We need the perturbed function to be strictly concave, such that (in translated coordinates) $\tilde{u}'( x) =u'( x) .$ The condition on the derivative is guaranteed by that fact that $\eta ( x) =1$ for $x\in [ -a,a].$ To guarantee the concavity condition, we need to consider the second derivative of $\eta$. To define the bump function $\eta$ formally, we first define a function g (as in Hirsch 1976 on page 42), on the interval $a\le x\le b$, such that

$$\begin{aligned} g( x) =\frac{1}{B}\int _{x}^{b}e^{-\frac{( b-a) }{ ( y-a) ( b-y) }}\mathrm{d}y,\quad \text {where}\; B=\int _{a}^{b}e^{-\frac{( b-a) }{( y-a) (b-y) }}\mathrm{d}y. \end{aligned}$$

We now define the complete bump function:

$$\begin{aligned} \eta ( x) =\left\{ \begin{array}{ll} 0&{}\quad \text { if }x\le -b \\ g( -x)&{}\quad \text { if }-b\le x\le -a \\ 1&{}\quad \text { if }-a\le x\le a \\ g( x)&{}\quad \text { if }a\le x\le b \\ 0&{}\quad \text { if }x\ge b \end{array}\right\} . \end{aligned}$$

Given the symmetry in the definition of the bump function, we can restrict attention to the case $a\le x\le b$ for derivative calculations. In this case, we have $g'( x) =-\frac{1}{B}e^{-\frac{( b-a) }{(x-a) ( b-x) }}$, so that we get

$$\begin{aligned} g''( x) =\frac{1}{B}e^{-\frac{( b-a) }{(x-a) ( b-x) }}\cdot \frac{( b-a) }{(x-a) ( b-x) }\cdot \frac{( b-a) [ 2x-( a+b)] }{[ ( x-a) ( b-x) ] ^{2}}. \end{aligned}$$

Since the first two terms are non-negative, the sign of $g''$ depends on the third term, and $g''(x) <0$ when $a<x<\frac{a+b}{2}$, and $g''( x) >0$ for $\frac{a+b}{2}<x<b$. Since $g''( x) =0$ for $0\le x\le a$ and for $x\ge b$, $g''$ is bounded on the interval [0, b] . By symmetry, the same is true on the interval $[ -b,0] ,$ so $g''$ is bounded. Hence, by taking $\varepsilon$ sufficiently small, we can guarantee that $\tilde{u} ( x)$ is strictly concave. Therefore, this perturbation will keep the rational-expectations competitive equilibrium equations (18)–(25) the same, but change Pareto optimality equations (27)–(32). Hence, a rational-expectations competitive equilibrium is not Pareto optimal. $\square$

Proof of Theorem 1

We will first show that to attain a Pareto optimal rational-expectations competitive equilibrium, old agents must be paid optimal wages $w_o^*=\frac{(1-\alpha )\pi '(a_o^*)[u(c_{y}^{H,*})-u(c_y^{L,*})] +\alpha \pi '(a_o^*)[u(c_o^{H,*})-u(c_o^{L,*})] }{\alpha [\pi (a_o^*)u'(c_o^{H,*}) +(1-\pi (a_o^*))u(c_o^{L,*})]}$. We will then prove that a Pareto optimal allocation can be implemented by imposing wage tax $t_{w }=\rho \pi '(a_{o}^{*})(z^{H}-z^{L})-$ $\frac{\phi ^{\prime }(a_{o}^{*})}{E[u^{\prime }(c_{o}^{*})]}$ and equity tax $t^{s}= \frac{E[u^{\prime }(c_{o}^{*})(p^{*}+\delta ^{*})]-u^{\prime }(c_{y}^{s,*})p^{s,*}}{u^{\prime }(c_{o}^{s,*})}$ for $s\in \{H,L\}$.

As shown in Proposition 2, a rational-expectations competitive equilibrium is not Pareto optimal, because it fails to satisfy all rational-expectations competitive equilibrium (18)–(25) and Pareto optimality equations (27)–(32) simultaneously. Both rational-expectations competitive equilibrium and Pareto optimality equations involve first-order conditions with respect to $a_{o}$, and unless these two equations (i.e., (20) and (31)) are combined, a rational-expectations competitive equilibrium allocation cannot satisfy both sets of equations. Substituting (20) into (31), we obtain the old agent’s optimal wage

$$\begin{aligned} w_{o}^{*}=\frac{(1-\alpha )\pi '(a_o^*)[u(c_{y}^{H,*})-u(c_y^{L,*})] +\alpha \pi '(a_o^*)[u(c_o^{H,*})-u(c_o^{L,*})] }{\alpha [\pi (a_o^*)u'(c_o^{H,*})+(1-\pi (a_o^*))u(c_o^{L,*})]}. \end{aligned}$$

(33)

We will derive the updated rational-expectations competitive equilibrium equations after imposing wage tax $t_w$ and equity tax $t^s$. After imposing wage tax $t_w$, the old agent’s wage equation (24) changes as follows:

$$\begin{aligned} w_{o}= & {} \rho \pi ^{\prime }(a_{o})(z^{H}-z^{L})-t_{w }. \end{aligned}$$

(34)

After imposing equity tax $t^{s}$, the old agent’s budget constraint becomes

$$\begin{aligned} c_{o}^{s}= & {} a_{o}w _{o}+(p^{s}-t^{s}+\delta ^{s})e^s+t^{s}. \end{aligned}$$

(35)

Substituting the equity market-clearing condition $e^s=1$, (35) collapses to the old agent’s original budget constraint (22). After imposing equity tax $t^{s}$, the first-order conditions with respect to equity (18) and (19) change as follows

$$\begin{aligned}&\pi (a_{o})[u^{\prime }(c_{o}^{H})(p^{H}-t^{H}+\delta ^{H})]+(1-\pi (a_{o}))[u^{\prime }(c_{o}^{L})(p^{L}-t^{L}+\delta ^{L})]\nonumber \\&\quad =u^{\prime }(c_{y}^{H})p^{H} \end{aligned}$$

(36)

$$\begin{aligned}&\pi (a_{o})[u^{\prime }(c_{o}^{H})(p^{H}-t^{H}+\delta ^{H})]+(1-\pi (a_{o}))[u^{\prime }(c_{o}^{L})(p^{L}-t^{L}+\delta ^{L})]\nonumber \\&\quad =u^{\prime }(c_{y}^{L})p^{L}. \end{aligned}$$

(37)

Thus, the updated rational-expectations competitive equilibrium equations are (20)–(23), (25), (34), (36), and (37).

Let $\{c_{y}^{H,*}\,$, $c_{y}^{L,*}$, $c_{o}^{H,*}$, $c_{o}^{L,*}$, $a_{o}^{*}\}$ be a Pareto optimal allocation, then it satisfies the Pareto optimality equations (27)–(32) by definition. We will prove, by construction, that $\{c_{y}^{H,*}\,$, $c_{y}^{L,*}$, $c_{o}^{H,*}$, $c_{o}^{L,*}$, $a_{o}^{*}\}$ satisfies the updated rational-expectations competitive equilibrium equations (20)–(23), (25), (34), (36), and (37). Substituting $c_{o}^{H,*},c_{o}^{L,*}$, and $a_o^*$ into (20) gives the old agent’s optimal wage $w _{o}^{*}=\frac{\phi ^{\prime }(a_{o}^{*})}{E[u^{\prime }(c_{o}^{*})]}$. Substituting $a_y^{*}=\overline{a_y}$ into (23) yields the young agent’s optimal wage $w _{y}^{*}=f^{^{\prime }}(\overline{a_{y}})$. Plugging $w_{y}^{*},w_{o}^{*}$, and $a_o^*$ into (25), we get dividend $\delta ^{s,*}=\gamma ^{s,*}-\overline{a_{y}}w _{y}^{*}-a_{o}^{*}w _{o}^{*}$, where $s\in \{H,L\}$. Plugging $c_y^{s,*}$ and $w_{y}^{*}$ into (21), we obtain prices $p^{s,*}=\overline{a_{y}} w _{y}^{*}-c_{y}^{s,*}$, where $s \in \{H,L\}$. Because we now know the expressions for all variables (i.e., $c_{y}^{L,*}$, $c_{o}^{H,*}$,  $c_{o}^{L,*}$, $p^{H,*}$, $p^{L,*}$, $\delta ^{H,*}$, $\delta ^{L,*}$, $w _{y}^{*}$, $w _{o}^{*}$, $a_{o}^{*}$), we next verify the remaining equations. Plugging $c_o^{s,*}$, $a_o^*$, $w _{o}^{*}$, $p^{s,*}$, and $\delta ^{s,*}$ verifies (22) as follows:

$$\begin{aligned} c_{o}^{s,*}=a_{o}^{*}w _{o}^{*}+p^{s,*}+\delta ^{s,*}=-c_{y}^{s,*} +\gamma ^{s}=c_{o}^{s,*},\quad \text {where}\; s \in \{H,L\}. \end{aligned}$$

Substituting $w _{o}^{*}$, $a_o^*$, and $t_{w }$ into (34), we obtain

$$\begin{aligned} w _{o}^{*}=\rho \pi ^{\prime }(a_{o}^{*})(z^{H}-z^{L})-t_{w } = \frac{\phi ^{\prime }(a_{o}^{*})}{E[u^{\prime }(c_{o}^{*})]}. \end{aligned}$$

Plugging $c_o^{s,*}$, $c_y^{s,*}$, $a_o^*$, $p^{s,*}$, $\delta ^{s,*}$, and $t^{s}$ into (36), we get

$$\begin{aligned}&u^{\prime }(c_{y}^{H,*})p^{H,*} = \pi (a_{o}^{*})[u^{\prime }( c_{o}^{H,*})(p^{H,*}-t^{H}+\delta ^{H,*})]\nonumber \\&\qquad +\,(1-\pi (a_{o}^{*}))[u^{\prime }( c_{o}^{L,*})(p^{L,*}-t^{L}+\delta ^{L,*})] \nonumber \\&\quad =\pi (a_{o}^{*})\left[ u^{\prime }(c_{o}^{H,*})\left( p^{H,*}-\frac{ E[u^{\prime }(c_{o}^{*})(p^{*}+\delta ^{*})]-u^{\prime }(c_{y}^{H,*})p^{H,*}}{u^{\prime }(c_{o}^{H,*})}+\delta ^{H,*}\right) \right] \nonumber \\&\qquad +\, (1-\pi (a_{o}^{*}))\left[ u^{\prime }(c_{o}^{L,*})\left( p^{L,*}- \frac{E[u^{\prime }(c_{o}^{*})(p^{*}+\delta ^{*})]-u^{\prime }(c_{y} ^{L,*})p^{L,*}}{u'(c_{o}^{L,*})}+\delta ^{L,*}\right) \right] \nonumber \\&\quad =E[u^{\prime }(c_{o}^{*})(p^{*}+\delta ^{*})]+\pi (a_{o}^{*})u^{\prime }(c_{y}^{H,*})p^{H,*}\nonumber \\&\qquad + (1-\pi (a_{o}^{*}))u^{\prime }(c_{y}^{L,*} )p^{L,*}-E[u^{\prime }(c_{o}^*)(p^{*}+\delta ^*)] \nonumber \\&\quad =\pi (a_{o}^{*})u^{\prime }(c_{y}^{H,*})p^{H,*}+(1-\pi (a_{o}^{*}))u^{\prime }(c_{y}^{L,*})p^{L,*}. \end{aligned}$$

(38)

Moreover, (36) and (37) imply that $u^{\prime }(c_{y}^{H,*})p^{H,*}=u^{\prime }(c_{y}^{L,*})p^{L,*}$, and plugging this into (38) gives

$$\begin{aligned} u^{\prime }(c_{y}^{H,*})p^{H,*} =\pi (a_{o}^{*})u^{\prime }(c_{y}^{H,*})p^{H,*}+(1-\pi (a_{o}^{*}))u^{\prime }(c_{y}^{L,*})p^{L,*}=u^{\prime }(c_{y}^{H,*})p^{H,*}. \end{aligned}$$

Similarly, substituting $c_o^{s,*}$, $c_y^{s,*}$, $a_o^*$, $p^{s,*}$, $\delta ^{s,*}$, $t^{s}$, and $u^{\prime }(c_{y}^{H,*})p^{H,*}=u^{\prime }(c_{y}^{L,*})p^{L,*}$ into (37) yields

$$\begin{aligned} u^{\prime }(c_{y}^{L,*})p^{L,*} =\pi (a_{o}^{*})u^{\prime }(c_{y}^{H,*}) p^{H,*}+(1-\pi (a_{o}^{*}))u^{\prime }(c_{y}^{L,*})p^{L,*}=u'(c_{y}^{L,*}) p^{L,*}.\quad \quad \end{aligned}$$

$\square$

Proof of Proposition 3

We will show that a second-best allocation can be implemented if the social planner imposes equity tax $t^{s}$ $=\frac{E[u^{\prime }(\tilde{c}_o)(\tilde{p}+\tilde{\delta })]-u^{\prime }(\tilde{c}_y^s)\tilde{p}^s}{u^{\prime }(\tilde{c}_o^s)}$, where $s\in \{H,L\}$.

We first derive the equations that a second-best allocation satisfies. Letting $\mu \ge 0$ be the Lagrange multiplier of (10), the first-order conditions of (8)–(10) are

$$\begin{aligned}&\pi (a_{o})[(1-\alpha )u^{\prime }(c_{y}^{H})-\alpha u^{\prime }(\gamma ^{H}-c_{y}^{H})-\mu u^{\prime \prime }(\gamma ^{H}-c_{y}^{H})\rho \pi ^{\prime }(a_{o})(z^{H}-z^{L})]=0 \end{aligned}$$

(39)

$$\begin{aligned}&(1-\pi (a_{o}))[(1-\alpha )u^{\prime }(c_{y}^{L})-\alpha u^{\prime }(\gamma ^{L}-c_{y}^{L})-\mu u^{\prime \prime }(\gamma ^{L}-c_{y}^{L})\rho \pi ^{\prime }(a_{o})(z^{H}-z^{L})]\nonumber \\&\quad =0 \end{aligned}$$

(40)

$$\begin{aligned}&(1-\alpha )\pi ^{\prime }(a_o)[u(c_{y}^{H})-u(c_{y}^{L})]+\alpha \pi ^{\prime }(a_o) [u(\gamma ^{H}-c_{y}^{H})-u(\gamma ^{L}-c_{y}^{L})]\nonumber \\&\quad -\alpha \phi ^{\prime }(a_{o})-\mu \phi ^{\prime \prime }(a_{o}) \end{aligned}$$

(41)

$$\begin{aligned}&\quad +\mu \pi ^{\prime }(a_{o})[u'(\gamma ^{H}-c_{y}^{H})-u'(\gamma ^{L}-c_{y}^{L})] \rho \pi '(a_{o})(z^{H}-z^{L})\nonumber \\&\quad +\mu E[u^{\prime }(\gamma -c_{y})]\rho \pi ''(a_{o})(z^{H}-z^{L}) =0 \end{aligned}$$

(42)

$$\begin{aligned}&[\pi (a_o)u^{\prime }(c_o^H)+(1-\pi (a_o))u'(c_o^L)]\rho \pi ^{\prime }(a_{o})(z^{H}-z^{L})-\phi ^{\prime }(a_{o})=0 \end{aligned}$$

(43)

$$\begin{aligned}&c_{y}^{s}+c_{o}^{s}=\gamma ^{s},\quad \text {where}\; s\in \{H,L\}. \end{aligned}$$

(44)

After equity tax $t^s$ is imposed, first-order conditions with respect to equity (18) and (19) change to (36) and (37), so the updated rational-expectations competitive equilibrium equations are (20)–(25), (36), and (37).

Let $\{\tilde{c}_y^H,\tilde{c}_y^L,\tilde{c}_o^H,\tilde{c}_o^L,\tilde{a}_o \}$ be a second-best allocation, then it satisfies the second-best equations (39)–(44) by definition. We will prove, by construction, that $\{\tilde{c}_y^H,\tilde{c}_y^L,\tilde{c}_o^H,\tilde{c}_o^L,\tilde{a}_o\}$ satisfies the updated rational-expectations competitive equilibrium equations (20)–(25), (36), and (37). We obtain (43) by substituting (24) into (20), so $\{\tilde{c}_y^H,\tilde{c}_y^L,\tilde{c}_o^H,\tilde{c}_o^L,\tilde{a}_o\}$ satisfies (20) and (24), and hence, the old agent’s wage $\tilde{w}_o=\rho \pi ^{\prime }(\tilde{a}_o)(z^H-z^L)$. Substituting $\tilde{a}_y=\overline{a_y}$ into (23) yields the young agent’s wage $\tilde{w}_y=f^{\prime }(\overline{a_y})$. Plugging $\tilde{a}_o$, $\tilde{w}_y$, and $\tilde{w}_o$ into (25) gives dividend $\tilde{\delta }^s=\tilde{\gamma }^s-\overline{a_y}\tilde{w_y}-\tilde{a_o}\tilde{w_{o}}$ for $s \in \{H,L\}$. Substituting $\tilde{c}_y^s$ and $\tilde{w_{y}}$ into (21), we get prices $\tilde{p}^s=\overline{a_y}\tilde{w}_y-\tilde{c}_y^s$ for $s\in \{H,L\}$. Since we know the expressions for all variables (i.e., $\tilde{c_{y}^{H}},\tilde{c}_y^L,\tilde{c}_o^H,\tilde{c}_o^L,\tilde{p}^H,\tilde{p}^L,\tilde{\delta }^H,\tilde{\delta }^L,\tilde{w}_y,\tilde{w}_o,\tilde{a}_o$), we next verify the remaining equations. Substituting $\tilde{c}_o^s$, $\tilde{a}_o$, $\tilde{w}_o$, $\tilde{p}^s$, and $\tilde{\delta }^s$ verifies (22) as follows:

$$\begin{aligned} \tilde{c}_o^s=\tilde{a}_o\tilde{w}_o+\tilde{p}^s+\tilde{\delta }^s=-\tilde{c}_y^s +\gamma ^s=\tilde{c}_o^s\quad \text { for}\; s\in \{H,L\}. \end{aligned}$$

Plugging $\tilde{c}_y^s$, $\tilde{c}_o^s$, $\tilde{a}_o$, $\tilde{p}^s$, $\tilde{\delta }^s$, and $t^s$ into (36), we obtain

$$\begin{aligned}&u^{\prime }(\tilde{c}_y^H)\tilde{p}^H=\pi (\tilde{a}_o)[u^{\prime } (\tilde{c}_o^H)(\tilde{p}^H-t^H+\tilde{\delta }^H)]\nonumber \\&\qquad +\,(1-\pi (\tilde{a}_o)) [u^{\prime }(\tilde{c}_o^L)(\tilde{p}^L-t^L+\tilde{\delta }^L)] \nonumber \\&\quad =\pi (\tilde{a}_o)\left[ u^{\prime }(\tilde{c}_o^H)\left( \tilde{p}^H-\frac{E[u^ {\prime }(\tilde{c}_o)(\tilde{p}+\tilde{\delta })]-u^{\prime }(\tilde{c}_y^H)\tilde{p}^H}{u^{\prime }(\tilde{c}_o^H)}+\tilde{\delta }^H\right) \right] \nonumber \\&\qquad +\, (1-\pi (\tilde{a}_o))\left[ u^{\prime }(\tilde{c}_o^L)\left( \tilde{p}^L-\frac{E[u^{\prime }(\tilde{c}_o)(\tilde{p}+\tilde{\delta })]-u^{\prime }(\tilde{c}_y^L)\tilde{p}^L}{u^{\prime }(\tilde{c}_o^L)}+\tilde{\delta }^L\right) \right] \nonumber \\&\quad =E[u^{\prime }(\tilde{c}_o)(\tilde{p}+\tilde{\delta })]+\pi (\tilde{a}_o)u'(\tilde{c}_y^H)\tilde{p}^H\nonumber \\&\qquad +\,(1-\pi (\tilde{a}_o))u^{\prime }(\tilde{c}_y^L)\tilde{p}^L-E[u'(\tilde{c}_o)(\tilde{p}+\tilde{\delta })] \nonumber \\&\quad =\pi (\tilde{a}_o)u^{\prime }(\tilde{c}_y^H)\tilde{p}^H+(1-\pi (\tilde{a}_o))u^{\prime }(\tilde{c}_y^L)\tilde{p}^L . \end{aligned}$$

(45)

Moreover, (36) and (37) imply that $u'(\tilde{c}_{y}^{H})\tilde{p}^{H}=u'(\tilde{c}_{y}^{L})\tilde{p}^{L}$, and plugging this into (45) gives

$$\begin{aligned} u^{\prime }(\tilde{c}_{y}^{H})\tilde{p}^{H} =\pi (\tilde{a}_o)u'(\tilde{c}_{y}^{H}) \tilde{p}^{H}+(1-\pi (\tilde{a}_{o}))u'(\tilde{c}_{y}^{L})\tilde{p}^{L}=u'(\tilde{c}_{y}^{H})\tilde{p}^{H}. \end{aligned}$$

Similarly, substituting $\tilde{c}_y^s$, $\tilde{c}_o^s$, $\tilde{a}_o$, $\tilde{p}^s$, $\tilde{\delta }^s$, $t^s$, and $u'(\tilde{c}_{y}^{H})\tilde{p}^{H}= u'(\tilde{c}_{y}^{L})\tilde{p}^{L}$ into (37) yields

$$\begin{aligned} u^{\prime }(\tilde{c}_{y}^{L})\tilde{p}^{L} =\pi (\tilde{a}_o)u'(\tilde{c}_{y}^{H}) \tilde{p}^{H}+(1-\pi (\tilde{a}_{o}))u'(\tilde{c}_{y}^{L})\tilde{p}^{L}=u'(\tilde{c}_{y}^{L})\tilde{p}^{L}. \end{aligned}$$

$\square$