Thermo-mechanical behavior of a functionally graded hollow cylinder with an elliptic hole

Abstract

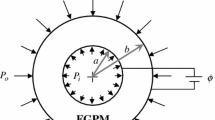
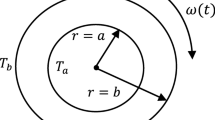
In this paper, the behavior of functionally graded material hollow cylinders with an elliptic hole under thermal and mechanical loads is investigated. The problem is considered as plane strain condition, and to obtain the governing equations and boundary condition for this complex geometry, an elliptic cylindrical coordinate is used. The material properties are considered to vary along the elliptic cylindrical direction with power-law function except for the Poisson’s ratio. To solve the two coupled differential equations, differential quadrature method is used. For solving the governing equations, two different boundary conditions are considered for thermal and mechanical loads. The results show that unconventional shape for a hole in the cylinder can affect the results expected such as stresses or displacements, and this information about thermo-mechanical loads can be used for designing the advanced sensors. Also with considering special material index, the stress and displacement along the cylinder can be controlled. The presented results in this paper are verified with those reported in the previous publication.

Appendices

Appendix A

The relations between elliptic cylindrical coordinate and Cartesian coordinate are presented as [38]:

$$\begin{aligned} x & = \cosh \psi \cos \eta \quad \psi \ge 0\quad h_{1} = \sqrt {\sinh^{2} \psi + \sin^{2} \eta } \\ y & = \sinh \psi \sin \eta \quad 0 \le \eta \le 2\pi \quad h_{2} = \sqrt {\sinh^{2} \psi + \sin^{2} \eta } \\ z & = z\quad - \infty < z < \infty \quad h_{3} = 1. \\ \end{aligned}$$

(38)

And the coordinate curves formed by the intersection of coordinate are as follows:

$$\begin{aligned} \frac{{x^{2} }}{{\cosh^{2} \psi }} + \frac{{y^{2} }}{{\sinh^{2} \psi }} & = 1\quad {\text{Elliptic}}\,{\text{cylinders}} \\ \frac{{x^{2} }}{{\cos^{2} \eta }} - \frac{{y^{2} }}{{\sin^{2} \eta }} & = 1 \quad {\text{Hyperbolic}}\,{\text{cylinders}} \\ z & = {\text{Constant}} .\\ \end{aligned}$$

(39)

Accordingly, the position vector for each point is:

$$\vec{r} = \left( {\cosh \psi \cdot \cos \eta } \right)\vec{i} + \left( {\sinh \psi \cdot \sin \eta } \right)\vec{j} + z\vec{k}.$$

(40)

And the unit vector for each direction is shown as:

$$\begin{aligned} \widehat{{e_{\psi } }} & = \frac{1}{{h_{1} }}e_{\psi } = \frac{\sinh \psi \cdot \cos \eta }{A}\vec{i} + \frac{\cosh \psi \cdot \sin \eta }{A}\vec{j} \\ \widehat{{e_{\eta } }} & = \frac{1}{{h_{2} }}e_{\eta } = \frac{ - \cosh \psi \cdot \sin \eta }{A}\vec{i} + \frac{\sinh \psi \cdot \cos \eta }{A}\vec{j} \\ \widehat{{e_{z} }} & = \frac{1}{{h_{3} }}e_{z} = \vec{k} \\ \end{aligned}$$

(41)

where “A” for simplicity is defined as:

$$A = \sqrt {\sinh^{2} \psi + \sin^{2} \eta }$$

(42)

Displacement vector in elliptic cylindrical coordinate is defined:

$$\vec{u} = u\widehat{{e_{\psi } }} + v\widehat{{e_{\eta } }} + w\widehat{{e_{z} }}$$

(43)

The strain tensor is defined as:

$$\varepsilon = \frac{1}{2}\left( {\nabla u + \nabla u^{T} } \right)$$

(44)

Using Eqs. (38)–(42) and substituting Eq. (43) into Eq. (44), the strains yield as:

$$\begin{aligned} \varepsilon_{\psi \psi } & = \frac{1}{A}\frac{\partial u}{\partial \psi } + \frac{\sin \eta \cdot \cos \eta }{{A^{3} }}v \\ \varepsilon_{\eta \eta } & = \frac{1}{A}\frac{\partial v}{\partial \eta } + \frac{\sinh \psi \cdot \cosh \psi }{{A^{3} }}u \\ \varepsilon_{\psi \eta } & = \frac{1}{2}\left( {\frac{1}{A}\frac{\partial u}{\partial \eta } + \frac{1}{A}\frac{\partial v}{\partial \psi } - \frac{\sinh \psi \cdot \cosh \psi }{{A^{3} }}v - \frac{\sin \eta \cdot \cos \eta }{{A^{3} }}u} \right). \\ \end{aligned}$$

(45)

Appendix B

The static equilibrium equation in a linear elastic material is expressed:

$$\sigma_{i, j}^{i} + \varrho b_{i} = 0\;i,j = 1, 2, 3$$

(46)

where $\sigma_{i, j}^{i}$, $\varrho$ and $b_{i}$ are stress tensor derivatives, density and the external body forces, respectively. Assuming an orthogonal coordinate system, the $\sigma_{i,j}^{i}$ is expressed [38]:

$$\sigma_{i,j}^{i} = \frac{1}{\sqrt g }\frac{\partial }{{\partial x^{j} }}\left( {\sqrt g \sigma_{i}^{j} } \right) - \left[ {ij,m} \right]\sigma^{mj}$$

(47)

where “g” is the metric components of the orthogonal system and expressed as:

$$g_{ij} = \left( {\begin{array}{*{20}c} {h_{1}^{2} } & 0 & 0 \\ 0 & {h_{2}^{2} } & 0 \\ 0 & 0 & {h_{3}^{2} } \\ \end{array} } \right).$$

(48)

And the equilibrium equations are presented as:

$$\mathop \sum \limits_{j = 1}^{3} \frac{1}{\sqrt g }\frac{\partial }{{\partial x^{j} }}\left( {\frac{{\sqrt g h_{i} \sigma_{ij} }}{{h_{j} }}} \right) - \frac{1}{2}\mathop \sum \limits_{j = 1}^{3} \frac{{\sigma_{jj} }}{{h_{j}^{2} }}\frac{{\partial \left( {h_{j}^{2} } \right)}}{{\partial x^{i} }} + h_{i} \varrho b_{i} = 0$$

(49)

where there is no summation on “i.”

Using Eqs. (46)–(49) and (38), the equilibrium equations along the “ψ” and “η” directions are presented, respectively, as:

$$\begin{aligned} &\frac{{\partial \sigma_{\psi \psi } }}{\partial \psi } + \frac{{\partial \sigma_{\psi \eta } }}{\partial \eta } + \frac{2\sin \eta \cos \eta }{{A^{2} }}\sigma_{\psi \eta }\\&\quad + \frac{\sinh \psi \cosh \psi }{{A^{2} }}\left( {\sigma_{\psi \psi } - \sigma_{\eta \eta } } \right) + \varrho b_{\psi } = 0 \end{aligned}$$

(50)

$$\begin{aligned}& \frac{{\partial \sigma_{\eta \eta } }}{\partial \eta } + \frac{{\partial \sigma_{\psi \eta } }}{\partial \psi } + \frac{2\sinh \psi \cosh \psi }{{A^{2} }}\sigma_{\psi \eta }\\&\quad + \frac{\sin \eta \cos \eta }{{A^{2} }}\left( {\sigma_{\eta \eta } - \sigma_{\psi \psi } } \right) + \varrho b_{\eta } = 0. \end{aligned}$$

(51)

Appendix C

The constants for material properties in Eqs. (18) and (19) are as follows:

$$\begin{aligned} d_{1} & = \frac{{N_{13}^{0} }}{{N_{11}^{0} }},\,d_{2} = \frac{{N_{12}^{0} }}{{N_{11}^{0} }},\,d_{3} = \frac{{2N_{12}^{0} }}{{N_{11}^{0} }},\,d_{4} = \frac{{ - 3N_{12}^{0} }}{{N_{11}^{0} }},\,d_{5} = \frac{{ - 2N_{13}^{0} }}{{N_{11}^{0} }},\,d_{6} = \frac{{N_{13}^{0} + N_{12}^{0} }}{{N_{11}^{0} }}, \\ d_{7} & = \frac{{N_{12}^{0} + N_{22}^{0} }}{{N_{11}^{0} }},\,d_{8} = \frac{{N_{11}^{0} + N_{13}^{0} }}{{N_{11}^{0} }},\,d_{9} = \frac{{ - N_{22}^{0} }}{{N_{11}^{0} }},\,d_{10} = \frac{{ - N_{13}^{0} }}{{N_{11}^{0} }},\,d_{11} = \frac{{3N_{13}^{0} - 2N_{11}^{0} - N_{12}^{0} }}{{N_{11}^{0} }}, \\ d_{12} & = \frac{{ - \kappa^{0} .\alpha^{0} .C_{1} }}{{N_{11}^{0} }},\,d_{13} = \frac{{ - 2\kappa^{0} .\alpha^{0} .C_{2} }}{{N_{11}^{0} }},\,d_{14} = \frac{{N_{13}^{0} }}{{N_{22}^{0} }},\,d_{15} = \frac{{N_{12}^{0} + N_{13}^{0} }}{{N_{22}^{0} }},\,d_{16} = \frac{{ - N_{13}^{0} - N_{11}^{0} }}{{N_{22}^{0} }}, \\ d_{17} & = \frac{{N_{22}^{0} + N_{13}^{0} }}{{N_{22}^{0} }},\,d_{18} = \frac{{2N_{12}^{0} }}{{N_{22}^{0} }},\,d_{19} = \frac{{ - 2N_{12}^{0} + N_{11}^{0} }}{{N_{22}^{0} }},\,d_{20} = \frac{{ - N_{13}^{0} }}{{N_{22}^{0} }},\,d_{21} = \frac{{ - N_{13}^{0} + N_{12}^{0} }}{{N_{22}^{0} }},\,d_{22} = \frac{{ - 2N_{13}^{0} }}{{N_{22}^{0} }} \\ d_{23} & = \frac{{ - N_{22}^{0} + N_{13}^{0} - N_{12}^{0} }}{{N_{22}^{0} }} \\ \end{aligned}$$

(52)