Skip to main content
SpringerLink
Log in

An approximate method for solution of nonlocal boundary value problems via Gaussian radial basis functions

  • Published:
SeMA Journal Aims and scope Submit manuscript

Abstract

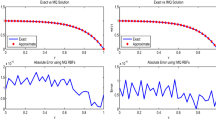
In this paper, we convert the parabolic and hyperbolic partial differential equations with initial and integral boundary conditions into classical Dirichlet initial-boundary value problems. We use a new scheme to solve the nonlocal initial-boundary value problems using collocation points and approximating the solution using radial basis functions (RBFs). We introduce radial basis functions and a new operational matrix of derivative for Gaussian (GA) radial basis functions is employed to reduce the problem to a set of algebraic equations. The results of numerical experiments are presented and compared with the results of other methods to confirm the validity of this method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  1. Ang, W.T.: A numerical method for the wave equation subject to a non-local conservation condition. Appl. Numer. Math. 56, 1054–1060 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  2. Atluri, S.N., Zhu, T.: New meshless local Petrov–Galerkin (MLPG) approach in computational mechanics. Comput. Mech. 22(2), 117–127 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  3. Avazzadeh, Z., Hosseini, V.R., Chen, W.: Radial basis functions and FDM for solving fractional diffusion-wave equation. Iran. J. Sci. Technol. 38, 205–212 (2014)

    MathSciNet  Google Scholar 

  4. Babuska, I., Melenk, J.: The partition of unity method. Int. J. Numer. Meth. Eng. 40, 727–758 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  5. Beilin, S.A.: Existence of solutions for one-dimensional wave equations with nonlocal conditions. Electron. J. Differ. Equ. 76, 1–8 (2001)

    MathSciNet  MATH  Google Scholar 

  6. Belytschko, T., Lu, Y.Y., Gu, L.: Element-free Galerkin methods. Int. J. Numer. Methods Eng. 37, 229–256 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  7. Biazar, J., Hosami, M.: Two efficient approaches based on radial basis functions to nonlinear time-dependent partial differential equations. J. Math. Comput. Sci. 9, 1–11 (2014)

    Article  Google Scholar 

  8. Bougoffa, L., Rach, R.C.: Solving nonlocal initial-boundary value problems for linear and nonlinear parabolic and hyperbolic partial differential equations by the Adomian decomposition method. Appl. Math. Comput. 225, 50–61 (2013)

    MathSciNet  MATH  Google Scholar 

  9. Bouziani, A.: On the solvability of parabolic and hyperbolic problems with a boundary integral condition. Int. J. Math. Math. Sci. 31, 201–213 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  10. Bouziani, A.: Problemes aux limites pour certaines équations de type non classique du troisieme ordre. Bull. Class. Sci. Acad. R. Belg. 6, 215–221 (1995)

    MathSciNet  MATH  Google Scholar 

  11. Caglar, H., Yilmaz, S.: A non-polynomial spline solution of the one-dimensional wave equation subject to an integral conservation condition. In: Proceedings of the 9th Wseas international conference on applied computer and applied computational science, Hangzhou (2010)

  12. Dehghan, M.: A meshless method for numerical solution of the one-dimensional wave equation with an integral condition using radial basis functions. Numer. Algor. 52, 461–477 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  13. Dehghan, M.: Numerical solution of a parabolic equation with non-local boundary specifications. Appl. Math. Comput. 145, 185–194 (2003)

    MathSciNet  MATH  Google Scholar 

  14. Dehghan, M.: On the solution of an inital-boundary value problem that combines Neumann and integral condition for the wave equation. Numer. Methods Partial Differ. Equ. 21, 24–40 (2005)

    Article  MATH  Google Scholar 

  15. Golbabai, A., Saeedi, A.: An investigation of radial basis function approximation methods with application in dynamic investment model. Iran. J. Sci. Technol. 39, 221–231 (2015)

    MathSciNet  Google Scholar 

  16. Golberg, M.A.: Some recent results and proposals for the use of radial basis functions in the BEM. Eng. Anal. Bound. Element 23(4), 285–296 (1999)

    Article  MATH  Google Scholar 

  17. Kadalbajoo, M.K., Kumar, A., Tripathi, L.: A radial basis functions based finite differences method for wave equation with an integral condition. Appl. Math. Comput. 253, 8–16 (2015)

    MathSciNet  MATH  Google Scholar 

  18. Kreyszig, E.: Introductory Functional Analysis with Applications. Wiley, New York (1978)

    MATH  Google Scholar 

  19. Lee, C.K., Liu, X., Fan, S.C.: Local multiquadric approximation for solving boundary value problems. Comput. Mech. 30, 396–409 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  20. Liszka, T.: An interpolation method for an irregular net of nodes. Int. J. Numer. Meth. Eng. 20, 1599–1612 (1984)

    Article  MATH  Google Scholar 

  21. Liu, W., Jun, S., Zhang, Y.: Reproducing kernel particle methods. Int. J. Numer. Methods Fluids 20, 1081–1106 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  22. Micchelli, C.: Interpolation of scattered data: distance matrices and conditionally positive definite functions. Constr. Approx. 2(1), 11–22 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  23. Nayroles, B., Touzot, G., Villon, P.: Generalizing the finite element method: diffuse approximation and diffuse elements. Comput. Mech. 10, 307–318 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  24. Onate, E., Idelsohn, S., Zienkiewicz, O.C., Taylor, R.L.: A finite point method in computational mechanics, application to convective transport and fluid flow. Int. J. Numer. Meth. Eng. 39, 3839–3866 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  25. Ramezani, M., Dehghan, M., Razzaghi, M.: Combined finite difference and spectral methods for the numerical solution of hyperbolic equation with an integral condition. Numer. Methods Partial Differ. Equ. 24, 1–8 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  26. Saadatmandi, A., Dehghan, M.: Numerical solution of the one-dimensional wave equation with an integral condition. Numer. Methods Partial Differ. Equ. 23, 282–292 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  27. Sarabadan, S., Shahrezaee, M., Rad, J.A., Parand, K.: Numerical solution of Maxwell equations using local weak form meshless techniques. J. Math. Comput. Sci. 13, 168–185 (2014)

    Article  Google Scholar 

  28. Shakeri, F., Dehghan, M.: The method of lines for solution of the one-dimensional wave equation subject to an integral conservation condition. Comput. Math. Appl. 56, 2175–2188 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  29. Shingmin, W., Yanping, L.: A numerical method for the diffusion equation with nonlocal boundary specifications. Int. J. Eng. Sci. 28, 543–546 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  30. Shu, C., Ding, H., Yeo, K.S.: Solution of partial differential equations by a global radial basis function-based differential quadrature method. Eng. Anal. Bound. Element 28, 1217–1226 (2004)

    Article  MATH  Google Scholar 

  31. Tatari, M., Dehghan, M.: On the solution of the non-local parabolic partial differential equations via radial basis functions. Appl. Math. Model. 33, 1729–1738 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  32. Wendland, H.: Piecewise polynomial, positive definite and compactly supported radial functions of minimal degree. Adv. Comput. Math. 4(4), 389–396 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  33. Wu, Z.M.: Radial basis function scattered data interpolation and the meshless method of numerical solution of PDEs. Chin. J. Eng. Math. 19(2), 1–12 (2002)

    MathSciNet  MATH  Google Scholar 

  34. Wu, Z.M., Schaback, R.: Local error estimates for radial basis function interpolation of scattered data. IMA J. Numer. Anal. 13(1), 13–27 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  35. Zerroukat, M., Power, H., Chen, C.S.: A numerical method for heat transfer problem using collocation and radial basis functions. Int. J. Numer. Methods Eng. 42, 1263–1278 (1992)

    Article  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Faculty of Mathematical Sciences, Alzahra University, Tehran, Iran

    M. Khaksarfard & Y. Ordokhani

  2. Faculty of Mathematical Sciences and Computer, Kharazmi University, Tehran, Iran

    E. Babolian

Authors
  1. M. Khaksarfard
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Y. Ordokhani
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. E. Babolian
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Y. Ordokhani.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Khaksarfard, M., Ordokhani, Y. & Babolian, E. An approximate method for solution of nonlocal boundary value problems via Gaussian radial basis functions. SeMA 76, 123–142 (2019). https://doi.org/10.1007/s40324-018-0165-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40324-018-0165-1

Keywords

Mathematics Subject Classification

Search

Navigation