Skip to main content
SpringerLink
Log in

A Müntz wavelets collocation method for solving fractional differential equations

  • Published:
Computational and Applied Mathematics Aims and scope Submit manuscript

Abstract

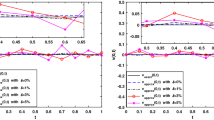
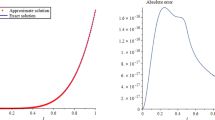
In this paper, we introduce a new set of wavelets, namely Müntz wavelets. These wavelets are defined based on Müntz Legendre polynomials which have the property of orthogonality. We stabilize the wavelet coefficients by utilizing Jacobi polynomials to construct the modified Müntz wavelets. Next, we present a collocation scheme based on Müntz wavelets for solving fractional differential equations. The fractional derivative is described in the Caputo sense. Some error estimates are given and numerical examples are included to demonstrate the applicability and accuracy of the proposed method. This includes the elastic-dissipative mathematical model of linear mechanical devices known as Boltzman operator.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  • Almira J (2007) Müntz type theorems I. Surv Approx Theory 3:152–194

    MathSciNet  MATH  Google Scholar 

  • Babaaghaie A, Maleknejad K (2017) Numerical solutions of nonlinear two-dimensional partial Volterra integro-differential equations by Haar wavelet. J Comput Appl Math 317:643–651

    Article  MathSciNet  MATH  Google Scholar 

  • Bahmanpour M, Fariborzi Araghi MA (2012) Numerical solution of Fredholm and Volterra integral equations of the first kind using wavelets bases. J Math Comput Sci 5(4):337–345

    Article  Google Scholar 

  • Bahmanpour M, Fariborzi Araghi MA (2013) A method for solving Fredholm integral equation of the first kind based on Chebyshev wavelets. Anal Theory Appl 29(3):197–207

    MathSciNet  MATH  Google Scholar 

  • Diethelm K (2010) The analysis of fractional differential equations. Springer, Berlin

    Book  MATH  Google Scholar 

  • Diethelm K, Walz G (1997) Numerical solution of fractional order differential equations by extrapolation. Numer. Algorithms 16:231–253

    Article  MathSciNet  MATH  Google Scholar 

  • Diethelm K, Ford NJ, Freed AD (2002) A Predictor–Corrector approach for the numerical solution of fractional differential equations. Nonlinear Dyn 16:3–22

    Article  MathSciNet  MATH  Google Scholar 

  • El-sayed A, Gaber M (2006) The Adomian decomposition method for solving partial differential equations of fractional order in finite domains. Phys Lett A 359:175–182

    Article  MathSciNet  MATH  Google Scholar 

  • Eslahchi MR, Kavoosi M (2017) The use of Jacobi wavelets for constrained approximation of rational Bézier curves. Comput Appl Math (accepted manuscript). https://doi.org/10.1007/s40314-017-0552-8

  • Esmaeili S, Shamsi M, Luchko Y (2011) Numerical solution of fractional differential equations with a collocation method based on Müntz polynomials. Comput Math Appl 62(3):918–929

    Article  MathSciNet  MATH  Google Scholar 

  • Friborzi Araghi MA, Bahmanpour M (2008) Numerical solution of Fredholm integral equation of the first kind using Legendre, Chebyshev and CAS wavelets. Int J Math Sci Eng Appl 2(4):1–9

    MATH  Google Scholar 

  • Galeone L, Garrappa R (2006) On multistep methods for differential equations of fractional order. Mediterr J Math 3:565–580

    Article  MathSciNet  MATH  Google Scholar 

  • Garrappa R, Popolizio M (2011) On accurate product integration rules for linear fractional differential equations. J Comput Appl Math 235:1085–1097

    Article  MathSciNet  MATH  Google Scholar 

  • Grzesikiewicz W, Wakulicz A, Zbiciak A (2013) Non-linear problems of fractional calculus in modeling of mechanical systems. Int J Mech Sci 70:90–98

    Article  Google Scholar 

  • Hilfer R (ed) (2000) Applications of fractional calculus in physics. World Scientific Publishing Company, Singapore

    MATH  Google Scholar 

  • Ishikawa S, Nussbaum RD (1991) Some remarks on differential equations of quadratic type. J Dyn Differ Equ 3(3):457–490

    Article  MathSciNet  MATH  Google Scholar 

  • Kilbas AA, Srivastava HM, Trujillo JJ (2006) Theory and applications of fractional differential equations, North-Holland Mathematics Studies. Elsevier Science B.V, Amsterdam, pp 7–10

    Google Scholar 

  • Lakestani M, Dehghan M, Irandoust-Pakchin S (2012) The construction of operational matrix of fractional derivatives using B-spline functions. Commun Nonlinear Sci Numer Simul 17:1149–1162

    Article  MathSciNet  MATH  Google Scholar 

  • Maleki M, Hashim I, Tavassoli Kajani M, Abbasbandy S (2012) An adaptive pseudospectral method for fractional order boundary value problems. Abstr Appl Anal 2012:1–19

    Article  MathSciNet  MATH  Google Scholar 

  • Massopust P (2016) Fractal Functions, Fractal Surfaces, and Wavelets, Second edn. Academic Press, New York, pp 261–327

    Book  MATH  Google Scholar 

  • Miller KS, Ross B (1993) An introduction to the fractional calculus and fractional differential equations. A Wiley-Interscience Publication. Wiley, New York

    Google Scholar 

  • Mohyud-Din ST, Khan H, Arif M, Rafiq M (2017) Chebyshev wavelet method to nonlinear fractional Volterra–Fredholm integro-differential equations with mixed boundary conditions. Adv Mech Eng 9(3):18

    Article  Google Scholar 

  • Podlubny I (1999a) Fractional differential equations. Academic Press, San Diego

  • Podlubny I (1999b) Fractional differential equations: an introduction to fractional derivatives, fractional differential equations, to methods of their solution and some of their applications, Mathematics in Science and Engineering. Academic Press, New York, p 198

  • Rasouli Gandomani M, Tavassoli Kajani M (2015) Application of shifted Müntz–Legendre polynomials for solving fractional differential equations. Int J Pure Appl Math 103(2):263–279

    Google Scholar 

  • Ross B (1977) The development of fractional calculus 1695–1900. Hist Math 4(1):75–89

    Article  MathSciNet  MATH  Google Scholar 

  • Rossikhin YA, Shitikova MV (2009) Application of fractional calculus for dynamic problems of solid mechanics: novel trends and recent result. Appl Mech Rev 63(1):52

    Google Scholar 

  • Xu X, Xu D (2018) A semi-discrete scheme for solving fourth-order partial integro-differential equation with a weakly singular kernel using Legendre wavelets method. Comput Appl Math (accepted manuscript). https://doi.org/10.1007/s40314-017-0566-2

  • Yang XJ (2018) New rheological problems involving general fractional derivatives with nonsingular power-law kernels. Proc Roman Acad Ser A 19(1):45–52

    MathSciNet  Google Scholar 

  • Yang XJ, Tenreiro Machado JA, Baleanu D, Gao F (2016) A new numerical technique for local fractional diffusion equation in fractal heat transfer. J Nonlinear Sci Appl 9(10):5621–5628

    Article  MathSciNet  MATH  Google Scholar 

  • Yang XJ, Tenreiro Machado JA, Baleanu D (2017a) Anomalous diffusion models with general fractional derivatives within the kernels of the extended Mittag–Leffler type functions. Roman Rep Phys 69(4):1–19

  • Yang XJ, Srivastava HM, Torres. Delfim FM, Zhang Y (2017b) Non-differentiable solutions for local fractional nonlinear Riccati differential equations. Fundam Inform 151(1–4):409–417

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Isfahan (Khorasgan) branch, Islamic Azad University, Isfahan, Iran

    M. Bahmanpour, Majid Tavassoli-Kajani & M. Maleki

Authors
  1. M. Bahmanpour
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Majid Tavassoli-Kajani
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. M. Maleki
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Majid Tavassoli-Kajani.

Additional information

Communicated by José Tenreiro Machado.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Bahmanpour, M., Tavassoli-Kajani, M. & Maleki, M. A Müntz wavelets collocation method for solving fractional differential equations. Comp. Appl. Math. 37, 5514–5526 (2018). https://doi.org/10.1007/s40314-018-0636-0

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40314-018-0636-0

Keywords

Mathematics Subject Classification

Search

Navigation