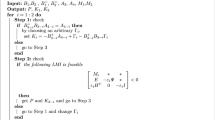Skip to main content
SpringerLink
Log in

Control of Discrete 2-D Takagi–Sugeno Systems via a Sum-of-Squares Approach

  • Published:
Journal of Control, Automation and Electrical Systems Aims and scope Submit manuscript

Abstract

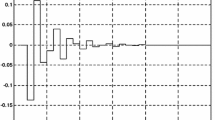
The stabilization of Takagi–Sugeno systems is solved here for the two-dimensional polynomial discrete case, by using the sum-of-squares approach. First, we provide a stabilization condition formulated in terms of polynomial multiple Lyapunov functions. Then, a non-quadratic stabilization condition is developed by applying relaxed stabilization technique. Both conditions can be used for design, by solving them using numerical tools such as SOSTOOLS. A numerical example illustrates the effectiveness of the results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  • Benzaouia, A., Hmamed, A., & Tadeo, F. (2016). Two-dimensional systems. Berlin: Springer.

    Book  MATH  Google Scholar 

  • Boukili, B., Hmamed, A., & Benzaouia, A. (2015). \(H_{\infty }\) state control for 2D fuzzy FM systems with stochastic perturbation. Circuits, Systems, and Signal Processing, 34(3), 779–796.

    Article  MATH  Google Scholar 

  • Boukili, B., Hmamed, A., Benzaouia, A., & El Hajjaji, A. (2014). \(H_{\infty }\) filtering of two-dimensional T–S fuzzy systems. Circuits, Systems, and Signal Processing, 33(6), 1737–1761.

    Article  MathSciNet  Google Scholar 

  • Boukili, B., Hmamed, A., & Tadeo, F. (2016). Robust \(H_{\infty }\) filtering for 2-D discrete Roesser systems. Journal of Control, Automation and Electrical Systems, 27(5), 497–505.

    Article  Google Scholar 

  • Chen, J., Xu, S., Zhang, B., Chu, Y., & Zou, Y. (2016). New relaxed stability and stabilization conditions for continuous-time T–S fuzzy models. Information Sciences, 329, 447–460.

    Article  MATH  Google Scholar 

  • Dang, Q. V., Vermeiren, L., Dequidt, A., & Dambrine, M. (2017). Robust stabilizing controller design for Takagi–Sugeno fuzzy descriptor systems under state constraints and actuator saturation. Fuzzy Sets and Systems, 329, 77–90.

    Article  MathSciNet  MATH  Google Scholar 

  • de Oliveira, M. C., Bernussou, J., & Geromel, J. C. (1999). A new discrete-time robust stability condition. Systems and Control Letters, 37(4), 261–265.

    Article  MathSciNet  MATH  Google Scholar 

  • Ding, D., Li, X., Xie, X., & Liu, D. (2011). Relaxed stabilization conditions for discrete-time 2-D TS fuzzy systems. In Chinese control and decision conference (CCDC) (pp. 3459–3464). IEEE.

  • Du, C., & Xie, L. (2002). \(H_{\infty }\) control and filtering of two-dimensional systems (Vol. 278). Berlin: Springer.

  • El-Kasri, C., Hmamed, A., Tissir, E. H., & Tadeo, F. (2013). Robust \(H_{\infty }\) filtering for uncertain two-dimensional continuous systems with time-varying delays. Multidimensional Systems and Signal Processing, 24(4), 685–706.

    Article  MathSciNet  MATH  Google Scholar 

  • Fang, C. H., Liu, Y. S., Kau, S. W., Hong, L., & Lee, C. H. (2006). A new LMI-based approach to relaxed quadratic stabilization of TS fuzzy control systems. IEEE Transactions on Fuzzy Systems, 14(3), 386–397.

    Article  Google Scholar 

  • Feng, G. (2004). Stability analysis of discrete-time fuzzy dynamic systems based on piecewise Lyapunov functions. IEEE Transactions on Fuzzy Systems, 12(1), 22–28.

    Article  MathSciNet  Google Scholar 

  • Gao, J., & Wang, W. (2014). \(H_{\infty }\) control For 2-D fuzzy FM II system with actuator saturation. In Proceedings of the 33rd Chinese control conference (CCC) (pp. 4435–4439).

  • Guelton, K., Manamanni, N., Koumba-Emianiwe, D. L., & Chinh, C. D. (2011). SOS stability conditions for nonlinear systems based on a polynomial fuzzy Lyapunov function. IFAC Proceedings Volumes, 44(1), 12777–12782.

    Article  Google Scholar 

  • Hmamed, A., Chaibi, R., & Tadeo, F. (2016). Stabilization of discrete-time 2-D TS Fuzzy system via a sum of squares (SOS) approach. In 5th IEEE international conference on systems and control (ICSC) (pp. 109–114).

  • Hmamed, A., Mesquine, F., Tadeo, F., Benhayoun, M., & Benzaouia, A. (2010). Stabilization of 2D saturated systems by state feedback control. Multidimensional Systems and Signal Processing, 21(3), 277–292.

    Article  MathSciNet  MATH  Google Scholar 

  • Johansson, M., Rantzer, A., & Arzen, K. E. (1999). Piecewise quadratic stability of fuzzy systems. IEEE Transactions on Fuzzy Systems, 7(6), 713–722.

    Article  Google Scholar 

  • Li, Z., Park, J. B., & Joo, Y. H. (2001). Chaotifying continuous-time TS fuzzy systems via discretization. IEEE Transactions on Circuits and Systems I: Fundamental Theory and Applications, 48(10), 1237–1243.

    Article  Google Scholar 

  • Marszalek, W. (1984). Two-dimensional state-space discrete models for hyperbolic partial differential equations. Applied Mathematical Modelling, 8, 11–14.

    Article  MathSciNet  MATH  Google Scholar 

  • Mozelli, L. A., Palhares, R. M., Souza, F. O., & Mendes, E. M. (2009). Reducing conservativeness in recent stability conditions of TS fuzzy systems. Automatica, 45(6), 1580–1583.

    Article  MathSciNet  MATH  Google Scholar 

  • Naamane, K., Chaibi, R., Tissir, E. H., & Hmamed, A. (2017). Stabilization of discrete-time TS fuzzy systems with saturating actuators. In International conference on advanced technologies for signal and image processing (ATSIP) (pp. 1–5). IEEE.

  • Owens, D. H., Amann, N., Rogers, E., & French, M. (2000). Analysis of linear iterative learning control schemes—A 2D systems/repetitive processes approach. Multidimensional Systems and Signal Processing, 11(1), 125–177.

    Article  MathSciNet  MATH  Google Scholar 

  • Papachristodoulou, A., Anderson, J., Valmorbida, G., Prajna, S., Seiler, P., & Parrilo, P. A. (2013). SOSTOOLS: Sum of squares optimization toolbox for MATLAB version 3.00. Pasadena: California Institute of Technology.

    Google Scholar 

  • Parrilo, P. A. (2000). Structured semidefinite programs and semialgebraic geometry methods in robustness and optimization (Doctoral dissertation, California Institute of Technology).

  • Prajna, S., Papachristodoulou, A., Seiler, P., & Parrilo, P. A. (2004). SOSTOOLS: Sum of squares optimization toolbox for MATLAB. Users guide, version 2.00. Pasadena: California Institute of Technology.

    Google Scholar 

  • Rogers, E., & Owens, D. H. (1992). Stability analysis for linear repetitive processes. Berlin: Springer.

    Book  MATH  Google Scholar 

  • Sala, A., & Arino, C. (2007). Relaxed stability and performance conditions for Takagi–Sugeno fuzzy systems with knowledge on membership function overlap. IEEE Transactions on Systems, Man, and Cybernetics, Part B (Cybernetics), 37(3), 727–732.

    Article  Google Scholar 

  • Shaker, H. R., & Shaker, F. (2014). Lyapunov stability for continuous-time multidimensional nonlinear systems. Nonlinear Dynamics, 75(4), 717–724.

    Article  MathSciNet  MATH  Google Scholar 

  • Siala, F., Gassara, H., Chaabane, M., & El Hajjaji, A. (2013). Stability analysis of polynomial fuzzy systems with time-delay via sum of squares (SOS) approach. In Proceedings of 14th international conference on sciences and techniques of automatic control and computer engineering (STA) (pp. 197–200).

  • Silva, L. F., Leite, V. J., Castelan, E. B., & Klug, M. (2014). Local stabilization of time-delay nonlinear discrete-time systems using Takagi-Sugeno models and convex optimization. Mathematical Problems in Engineering, 2014, 587510. https://doi.org/10.1155/2014/587510.

    Article  MathSciNet  Google Scholar 

  • Sulikowski, B., Galkowski, K., Rogers, E., & Owens, D. H. (2004). Output feedback control of discrete linear repetitive processes. Automatica, 40(12), 2167–2173.

    MathSciNet  MATH  Google Scholar 

  • Sulikowski, B., Galkowski, K., Rogers, E., & Owens, D. H. (2006). PI control of discrete linear repetitive processes. Automatica, 42(5), 877–880.

    Article  MathSciNet  MATH  Google Scholar 

  • Takagi, T., & Sugeno, M. (1985). Fuzzy identification of systems and its applications to modeling and control. IEEE Transactions on Systems, Man, and Cybernetics, 1, 116–132.

    Article  MATH  Google Scholar 

  • Tanaka, K., Ohtake, H., & Wang, H. O. (2008). An SOS-based stable control of polynomial discrete fuzzy systems. In IEEE American control conference (pp. 4875–4880).

  • Tanaka, K., Yoshida, H., Ohtake, H., & Wang, H. O. (2007). A sum of squares approach to stability analysis of polynomial fuzzy systems. In American control conference, ACC’07 (pp. 4071–4076). IEEE.

  • Wang, H. O., Tanaka, K., & Griffin, M. F. (1996). An approach to fuzzy control of nonlinear systems: Stability and design issues. IEEE Transactions on Fuzzy Systems, 4(1), 14–23.

    Article  Google Scholar 

  • Xiang-Peng, X., & Zhang, H. G. (2010a). Stabilization of discrete-time 2-D TS fuzzy systems based on new relaxed conditions. Acta Automatica Sinica, 36(2), 267–273.

    MathSciNet  Google Scholar 

  • Xiang-Peng, X. I. E., & Zhang, H. G. (2010b). Convergent stabilization conditions of discrete-time 2-D TS fuzzy systems via improved homogeneous polynomial techniques. Acta Automatica Sinica, 36(9), 1305–1311.

    MathSciNet  Google Scholar 

  • Xiaodong, L., & Qingling, Z. (2003). New approaches to \(H_{\infty }\) controller designs based on fuzzy observers for TS fuzzy systems via LMI. Automatica, 39(9), 1571–1582.

    Article  MathSciNet  MATH  Google Scholar 

  • Xie, X. P., Zhang, Z. W., & Hu, S. L. (2015). Control synthesis of roesser type discrete-time 2-D T–S fuzzy systems via a multi-instant fuzzy state-feedback control scheme. Neurocomputing, 151, 1384–1391.

    Article  Google Scholar 

  • Yang, H., Xia, Y., Qiu, J., & Zhang, J. (2010). Filtering for a class of discrete-time systems with time-delays via delta operator approach. International Journal of Systems Science, 41(4), 423–433.

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

Prof. Fernando Tadeo is funded by Conserjería de Educación, Junta de Castilla y Leon with European Regional Development Funds (Grant No. CLU 2017-09 and UIC 233), and by Secretaría de Estado de Investigación, Desarrollo e Innovación (Grant No. DPI2014-54530-R).

Author information

Authors and Affiliations

  1. LESSI, Department of Physics, Faculty of Sciences Dhar El Mehraz, B.P. 1796, Fes-Atlas, Morocco

    Redouane Chaibi, Abdelaziz Hmamed & El Houssaine Tissir

  2. Departamento de Ingenieria de Sistemas y Automatica, Institute of Sustainable Processes, Universidad de Valladolid, 47005, Valladolid, Spain

    Fernando Tadeo

Authors
  1. Redouane Chaibi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Abdelaziz Hmamed
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. El Houssaine Tissir
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Fernando Tadeo
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Fernando Tadeo.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chaibi, R., Hmamed, A., Tissir, E.H. et al. Control of Discrete 2-D Takagi–Sugeno Systems via a Sum-of-Squares Approach. J Control Autom Electr Syst 30, 137–147 (2019). https://doi.org/10.1007/s40313-018-00433-y

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40313-018-00433-y

Keywords

Search

Navigation