Abstract
Nanostructuring approach on TiNiSn-based half-Heusler (HH) thermoelectric materials (TE) has been well established as the most prominent paradigm for achieving high figure of merit (ZT). Herein, we have extended this approach on our previously reported bulk nanocomposite (BNC), containing HH and Full Heusler (FH) with little traces of Ti6Sn5 phase in a stoichiometric composition Ti9Ni7Sn8 for the optimization of high thermoelectric performance. A synergistic effect of nanostructuring of Ti9Ni7Sn8 bulk nanocomposite (BNC) on its thermoelectric properties was noticed, revealing an enhanced value of ZT ~ 0.83 at 773 K. This enhancement in ZT value is mainly ascribed to significant reduction in thermal conductivity (κ ~ 1.0 W/mK at 773 K), through modification in grain as well as phase boundary scattering. The marginal enhancement in Seebeck coefficient observed is attributed to charge carrier filtering effect at the interface of HH/FH phases.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
Introduction
Innovative developments in small-scale energy conversion technologies have gained enormous attention, especially in the utilization of low-potential heat energy. Thermoelectric (TE) energy converters, in particular, thermoelectric generators (TEG), occupy an important niche in modern technology and have a big potential for application in future energy-harvesting technologies, as well [1,2,3,4,5]. However, comparatively low efficiency of thermoelectric materials and devices makes a wide-scale expansion of this technology economically non-profitable. Therefore, the final goal of fundamental investigations in the field of thermoelectric is to search for cost-effective, non-toxic, and highly efficient materials for TEGs. The thermoelectric figure of merit (ZT) [6], given below, gauges the efficiency of thermoelectric materials:
where α, σ, and κ (\(\mathrm{w}\mathrm{h}\mathrm{e}\mathrm{r}\mathrm{e} {\kappa =\kappa }_{\mathrm{l}}+ {\kappa }_{\mathrm{e}}\)) is the thermopower, electrical conductivity, and thermal conductivity accounting both electronic (κe) and phonon (κl) contributions, respectively, and T is absolute temperature. High ZT can be achieved via enhancing α, σ, and reducing κ. However, the simultaneous optimization of these three transport properties is a highly challenging task due to their close interdependencies. As α increases, σ usually decreases which in turn affects \({k}_{\mathrm{e}}\) based on the Wiedemann–Franz law [6].
In the past few years, doping, band engineering, solid solution alloying, PGEC (phonon-glass electron-crystal), nanostructuring, and spin fluctuation driven effect on electronic transport have been the prime focus for tuning thermoelectric parameters simultaneously for optimizing large ZT in different class of materials [6,7,8,9,10,11,12]. However, unfortunately, a large number of high ZT materials are either toxic or expensive which makes them irrelevant for practical use in thermoelectric generators (TEG) [13, 14]. (The practical use of several high ZT thermoelectric materials in thermoelectric generators (TEG) is difficult due to either high cost or toxic nature.) Thus, study of cost-effective and non-toxic materials is relevant for thermoelectric device fabrication at a large scale.
Among several TE materials, the TE materials based on HH [15] have been shown to be potential thermoelectric materials as they include abundant non-toxic components, and also, they offer distinct structural advantages for designing bulk nanocomposites [16, 17]. In addition to this, they are site selective, such that if substituted on one site, there usually created lot of defects/disorder which further gets energetically minimized via phase separation of other intermetallic phases [18,19,20]. It is well documented that Heusler class of materials is very important from the waste-heat utilization perspective [21], provided that high ZT materials can be produced at low cost.
Although, (Zr,Hf)NiSn-based (n-type) [22,23,24,25,26] and (Zr,Hf)CoSb-based (p-type) [27,28,29] HH materials have shown decent ZT values with Hf being used as an ingredient element, it makes these materials highly expensive which resists their deployment in developing thermoelectric generators (TEG) for commercial application [13, 14]. Recently, a new family of NbFeSb-based p-type half-Heusler alloys has been discovered with high performance (ZT > 1.0) [30,31,32,33] which advances the applications of HH materials. An n-type counterpart of Hf-free p-type is essential for finding suitable applications with the existing materials. One may consider an alternative option with Hf-free TiNiSn-based n-type HH materials and these have been optimized with enhanced ZT [34, 35]. A high ZT near 1 has been achieved at 823 K in TiNiSn via modified synthesis route by Gurth et al. [34]. Two methods are commonly used to increase the thermoelectric efficiency, the alloying, and the nanostructuring. Alloying is a traditional method for modification of thermoelectrics, while aiming at the suppression of the thermal conductivity due to lattice part, and it also modified the band structure of the material, i.e., improved electronic properties. On the other hand, nanostructuring (nanocomposite) is the modern approach targeted on both phonon and electron transports to achieve high ZT. The bulk nanocomposite having multiphase nanocomposites with the high density of secondary-phase nanoinclusions can lead to enhancement in the ZT value; and this is via reducing thermal conductivity at boundaries and increasing power factor. This is caused either by modification of the band structure or by the effect of carrier filtering.
In many TE materials, nanostructuring has been observed to improve the ZT by reduction of thermal conductivity. Through the modification only in grain boundaries, there is an increase in phonon scattering from various interfaces between the grains [36, 37] or through an increase in Seebeck coefficient due to charge carrier filtering [38,39,40]. Nanostructuring on the bulk nanocomposite provides a constructive combination of modifying grain as well as phase boundaries for effective phonon scattering and, hence, to optimize the thermoelectric performance. In the present work, we have investigated the effect of nanostructuring of our previously reported Ti9Ni7Sn8 bulk nanocomposite (Nano-BNC) which is a composite having 97.1 ± 0.12% HH phase, 2.6 ± 0.2% in FH and traces of metallic Ti6Sn5 phase for the optimization of high ZT [41]. The phase separation and long-term stability of present composite material have been detailed in our earlier report [41]. Interestingly, a reasonably good ZT ~ 0.83 at 773 K has been demonstrated for nanostructured Ti9Ni7Sn8 bulk nanocomposite (Nano-BNC) which resulted from a synergistically combined effect of modifying grains and phase boundary scattering. Furthermore, the cost of this material is estimated to be almost 15% cheaper than the best ZT ~ 1.2 at 823 K in Hf-free n-type HH material [34]. The cost estimation has been performed using reports [13, 42, and 43]; though the ZT value of present material is lower than 1.0 compared to that of Hf containing n-type HH materials [19, 44, 45], but lower cost with such reasonably good ZT ~ 0.83 at 773 K justifies these materials to be used in large-scale applications.
Experimental details
The polycrystalline samples having stoichiometric compositions of TiNiSn, Ti9Ni7Sn8, and TiNi2Sn with high-purity elements were prepared by Arc-melting process. All the elemental powder for each stoichiometry was mixed using mortar and pestle, cold-pressed to make cylindrical pellets, and arc melted several times in the presence of high-purity argon atmosphere for making a homogeneous alloy. These homogenized alloys were crushed to make fine powders and subsequently densified using spark plasma sintering (SPS) technique up to 800 °C temperature and by applying 50 MPa pressure for 10 min. Here, TiNiSn; as HH (half-Heusler), TiNi2Sn; as FH (Full Heusler), Ti9Ni7Sn8; as BNC (Bulk Nanocomposite) are abbreviated. A part of the annealed sample of Ti9Ni7Sn8 (BNC) was further milled by planetary ball milling (HEBM, Fritsch, Pulverisette-4) to reduce the grain sizes of the bulk nanocomposite Ti9Ni7Sn8 for 30 h. The nanopowder of Ti9Ni7Sn8 was subjected to SPS to obtain a dense pellet of nanostructured bulk nanocomposite (Nano-BNC) of Ti9Ni7Sn8. The density (ρ) of these SPSed pellets/samples was measured using an Archimedes’ kit and was observed to be greater than 99% of their theoretical density. Microstructure investigation was performed by TEM (transmission electron microscopy, Technai G2T30) of all the samples. There are three steps involved in the preparation of samples for TEM investigation [41]. For computing the thermoelectric properties, σ (T) and α (T) were obtained using ULVAC, ZEM3 equipment. Moreover, thermal diffusivity (d) and specific heat (Cp) measurement were performed using laser flash system; Lineseis, LFA 1000 and differential scanning calorimetry (DSC) instrument; 822eMettler Toledo, respectively. Thermal conductivity was calculated using the formula, κ = d × Cp × ρ. To obtain carrier concentration, Hall measurement was carried out employing the Van der Pauw method at room temperature. Both sample preparation procedure and thermoelectric measurements of Nano-BNC were carried out three times to confirm their reproducibility. The values were realized to spread within 3% under the same experimental conditions.
Results and discussion
Microstructural characterization
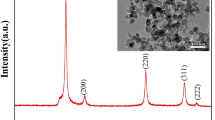
The detail TEM investigation is performed on a Nano-BNC sample of Ti9Ni7Sn8 to investigate the minor details of microstructure of nanostructuring of bulk nanocomposite in the present work. The TEM investigation of Ti9Ni7Sn8—BNC has already been presented in our previous report by Misra et al. [41] revealing the confirmation of three phases namely HH TiNiSn, FH TiNi2Sn, and Ti6Sn5. Figure 1 exhibits a bright-field TEM image obtained from the sample of nano-BNC. The micrograph (Fig. 1a) clearly shows the grain size of HH phase (marked as rectangle) varying from 25 to 100 nm, while the grain size of FH inclusion phase (marked as dotted circle) varies from 3 to 10 nm. The trace amount of Ti6Sn5 phase as white precipitate is also noted as another minor phase. This uniform distribution of inclusions at multiple length scales of grain size distribution integrates many exciting mechanisms such as electron injection, electron filtering jointly with an ample range of phonon dispersal to realize elevated ZT. Figure 1b presents SAED along [1 1 -1] zone axis parallel to the electron beam, obtained from the dotted white lines confirming it to be HH phase with a lattice parameter of 6.14 Å. Furthermore, the dotted circle area as shown in Fig. 1a has been magnified showing the lattice scale image (Fig. 1c) along (220) plane of FH phase. Fast Fourier Transform (FFT) was generated corresponding to the feature displayed (Inset Fig. 1c) revealing full-Heusler phase with [1-1-2] zone axis.
a Low-magnification TEM micrograph of the Ti9Ni7Sn8 (Nano-BNC) displaying two phase contrasts of HH phase (marked as dotted white lines) and FH inclusions (marked as yellow-colored circle) along with the trace amount of Ti6Sn5 phase (marked as blue-color rectangle); b selected area electron diffraction pattern (SAED) obtained from the dotted white lines showing the HH phase along the [1 1 – 1] zone axis; c HRTEM image obtained along (220) plane of FH phase revealing a lattice scale image. The fast Fourier transform (FFT) taken from this lattice scale image as shown in the inset confirms the black contrast to be FH phase
Electronic transport properties
To investigate the effect of nanostructuring on bulk nanocomposite for further optimization of the thermoelectric properties, the Nano-BNC sample of Ti9Ni7Sn8 have been subjected to measurements of electronic transport. For better visibility of improvement in electronic transport of nanostructured BNC sample, we have compared the present result with our reported data of TiNiSn HH and BNC sample of Ti9Ni7Sn8 [41]. The present result is also compared with TiNiSn synthesized via modified route by Gurth et al. [34]. Figure 2 represents the electronic transport characteristics of Ti9Ni7Sn8 Nano-BNC with temperature and compared with that of Ti9Ni7Sn8 BNC and TiNiSn HH materials [34, 41]. One can notice that the nanostructuring of BNC sample so-called Nano-BNC exhibits significantly reduced electrical conductivity (σ) at room temperature. Moreover, the σ (T) of Nano-BNC sample increases with rising temperature showing semiconducting behavior rather than to remain almost constant up to 150 °C as in case of BNC sample and which was further found to be increasing with rising temperature. We also noticed that increase in α is associated with decrease in the electrical conductivity. However, in case of Nano-BNC sample, surprisingly, the temperature variations of α (T) and σ (T) trends displayed are rather unusual, as it indicates a weak correlation between these parameters.
The carrier concentration (\(n=1/ {R}_{\mathrm{H}}\mathrm{e}\)) from Hall measurement and mobility at room temperature using relation \(\upsigma =\mathrm{n}\mathrm{e}\upmu\) was computed (Table 1). The carrier concentration of Nano-BNC sample was estimated to be ~ 1.2 × 1019/cm3, which was observed to be decreased with respect to Ti9Ni7Sn8 BNC (n ~ 2.8 × 1019/cm3) [41]. The reduced value of carrier concentration is abnormal in case of Nano-BNC sample which may be due to the low energy electron filtering at the interfaces of HH/FH phases as reported by Faleev et al. [46] and by others [47,48,49,50]. Figure 2b exhibits α (T) of Nano-BNC samples which was compared with that of BNC and HH sample [34, 41]. The α (T) value of Ti9Ni7Sn8 Nano-BNC sample at 323 K temperature is largest as compared to that of Ti9Ni7Sn8 BNC and TiNiSn HH. For instance, α (T) at room temperature for Ti9Ni7Sn8 Nano-BNC is – 61.2 µV/K which is ~ 12.5% larger than the value of – 54.4 µV/K obtained for Ti9Ni7Sn8 BNC [41] and ~ 40% larger than that of normal TiNiSn HH [41], as shown in Fig. 2b. This enhancement in α can be attributed to the electron filtering effect as suggested in many other reports [47,48,49,50].
A plausible explanation for this mechanism in which an increased value of α was observed for Ti9Ni7Sn8 Nano-BNC as compared to that of Ti9Ni7Sn8 BNC sample and TiNiSn HH can be given on the basis of the model proposed by Nolas et al. [51] and can be articulated as:
Here, r is the scattering factor, kB; the Boltzmann constant, and ξ; the reduced Fermi energy. In our present work, the reduced carrier concentration in Ti9Ni7Sn8 Nano-BNC sample decreases the Fermi energy which leads to increase in α (T) in comparison to its Ti9Ni7Sn8 BNC and TiNiSn HH. Moreover, increased α can be ascribed to an augmented r as well based on potential barrier scattering effect [52]. These results are consistent with a similar type of report on HH ZrNiSn/ZrO2-based bulk nanocomposites [53]. Exclusively, the behavior of α (T), from the room temperature, and strange observations of α (T) and \(\sigma (T)\) were found to be simultaneously increasing with rising temperature. To investigate the complex behavior in the transport properties of Nano-BNC sample, a Pisarenko plot which relates the α(T) and carrier density may be discussed as follows:
Assuming single parabolic bands up to 573 K, the Pisarenko plot of Ti9Ni7Sn8 Nano-BNC is constructed together with TiNiSn HH, Ti9Ni7Sn8 BNC for comparison (Fig. 3). In this graph, α corresponds to the particular effective mass (m*) and m* of materials was estimated from n by Eq. (3) and the slope of α vs T. The estimated m* at 323 K are noticed to be 0.305me for HH, 0.232me for BNC, and 0.149me for Nano-BNC, respectively. The m* was found to be varying for all the composites and does not satisfy the single parabolic band indicating a complexity in the electronic structure of these composites at Fermi level.
Power factor
Figure 2c shows the calculated value of power factor of Ti9Ni7Sn8 Nano-BNC samples as a function of temperature and is compared with that of reported TiNiSn HH and Ti9Ni7Sn8 BNC [34, 41]. The power factor of Ti9Ni7Sn8 Nano-BNC sample is slightly smaller than that of bare Ti9Ni7Sn8 BNC sample [41]. This is mainly due to the considerable decrease in \(\upsigma (\mathrm{T})\) associated with effective electron scattering.
Thermal transport properties
Figure 4a shows κ (T) of Ti9Ni7Sn8 Nano-BNC samples and is compared with that of TiNiSn HH and Ti9Ni7Sn8 BNC [34, 41]. Regardless of temperature, the κ (T) of Ti9Ni7Sn8 Nano-BNC samples have been observed to be decreased significantly when compared to that of TiNiSn HH and BNC samples. The reduced value of κ (T) may be ascribed to a synergy integrated for both phase and grain boundaries scattering in the present Ti9Ni7Sn8 Nano-BNC sample.
Temperature dependence behavior of a the total thermal conductivity, κ (T) of Ti9Ni7Sn8 Nano-BNC and its comparison with that of reported TiNiSn (HH), Ti9Ni7Sn8 (BNC) [41]; b calculated effective thermal conductivity for the composites Ti9Ni7Sn8 (BNC) using the effective medium theory and Maxwell–Eucken approximations; inset of b shows the total thermal conductivity of FH TiNi2Sn; c the lattice thermal conductivity, κL(T) of Ti9Ni7Sn8 Nano-BNC; d the electronic thermal conductivity, κe (T)
Interestingly, an ultra-low thermal conductivity (κ ~ 1.0 W/mK at 773 K) of Ti9Ni7Sn8 Nano-BNC sample was observed yielding to a high ZT ~ 0.83 at 773 K. It may be mentioned here that the phonons which have mean free paths ranging from short to mid can be scattered by modifying phase boundaries due to FH phase precipitation, Ti6Sn5 embedded in the HH matrix, while phonons having large mean free path can be scattered by numerous number of grain boundaries modified during the nanostructuring of bulk nanocomposite. The Wiedemann–Franz law is used to estimate κe = LσT, where L is the Lorentz number and \({k}_{l}\)=\({\upkappa -\upkappa }_{\mathrm{e}}\). Here, exact determination of Lorentz number with varying temperature is essential to estimate the correct value of κe and hence, in turn, \({\mathrm{k}}_{\mathrm{l}}\). This finding is very important to closely understand the boundary engineering effect on reduction of\({\mathrm{k}}_{\mathrm{l}}\). The Lorenz number varying with temperature is plotted in Fig. 5a for all the samples. Figure 5b also shows the relation between Lorenz number and Seebeck coefficient along with the estimated value of L based on single parabolic band model with acoustic phonon scattering (SPB-APS model) with a degenerated limit of Lorenz number [54].
a Temperature-dependent Lorenz number (L) of Ti¬9Ni7Sn8 Nano-BNC and TiNiSn (HH) and Ti9Ni7Sn8 (BNC) for comparison; b Relation between Lorenz number (L) and Seebeck coefficient, α (T) for all samples along with the estimated value of L based on single parabolic band model with acoustic phonon scattering (SPB-APS model) with degenerated limit of Lorenz number
Figure 4d shows κe vs T of Nano-BNC sample and the same is compared with that of the HH, BNC sample [41]. Consistent with the increasing \(\sigma\) with temperature, the κe was observed to enhance for all the samples with temperature. The κl of Nano-BNC sample as displayed in Fig. 4c shows that κl decreases with increasing temperature. As a result, the Nano-BNC sample displayed an ultra-low thermal conductivity (~ 1.0 W/mK) which is ~ 76% lower than that of TiNiSn HH [41] at 773 K temperature and ~ 62% lower than that of the BNC sample [41]. It is worth mentioning that such structural modification by incorporating the phase and grain boundary engineering leads to the reduced κl which is about more than six times reduction when compared to κl of TiNiSn for the best reported high ZT by Downie et al. [25].
The reduction in κ can be ascribed by synergistic effect of nanostructuring on bulk nanocomposite to modifying phase as well as grain boundaries or in other words known as boundary engineering approach as shown in the schematic diagram Fig. 6. This reduction is attributed to increased phonon scattering by various grain boundaries formation as well as the phase boundaries created due to the formation of nano inclusions of TiNi2Sn FH and Ti6Sn5 in Ti9Ni7Sn8 Nano-BNC sample, as presented in Fig. 6. The effective κ for Ti9Ni7Sn8 Nano-BNC and Ti9Ni7Sn8 BNC samples has been calculated theoretically via considering the minimal effect of Ti6Sn5 phase through Maxwell–Eucken approximations equation given below [55]:
\(\mathrm{H}\mathrm{e}\mathrm{r}\mathrm{e}, k\) is the effective thermal conductivity for the composite, where κ1,κ2 is the thermal conductivity due to continuous phase, i.e., HH matrix, and dispersed phase, i.e., FH, respectively, and \({v}_{ 1} and {v}_{2}\) stand for the volume fractions of HH and FH, respectively. Using above model, the thermal conductivity of Ti9Ni7Sn8 Nano-BNC samples was calculated and found to be much deviated as compared to the BNC sample, as shown in Fig. 4a, b [41], which could be due to the effect of nanostructuring; though the trends of the thermal conductivity graph were almost similar to this theoretically calculated model value as inclusion phases are same in Ti9Ni7Sn8 Nano-BNC sample.
Thermoelectric figure of merit
Estimated thermoelectric figure of merit (ZT) of Ti9Ni7Sn8 Nano-BNC is presented in Fig. 7a which have been compared with that of bare TiNiSn HH and Ti9Ni7Sn8 BNC sample [34, 41]. A maximum ZT ~ 0.83 at temperature of 773 K for Ti9Ni7Sn8 Nano-BNC was optimized, which was noticed to be significantly enhanced (> 300%) than ZT ~ 0.20 at 773 K for pure TiNiSn and ~ 170% enhanced than that of BNC sample (ZT ~ 0.32 at 773 K) [41]. This large enhancement in the ZT for Ti9Ni7Sn8 Nano-BNC was realized due to observed ultra-low κ (~ 74%) as compared to bare TiNiSn (HH) and BNC samples [41]. This favorable condition of drastic reduction in κ is attributed to the structurally modified grains as well as phase boundaries by means of precisely performing the engineering on the composition to form bulk nanocomposite and its nanostructuring via ball milling.
Figure 7b displays a comparison of ZT in the present research work with several other n-type HH based TE materials. ZT for various state-of-the-art HH materials compared (Fig. 7b) is from higher temperature side, i.e., above 773 K [56,57,58,59,60]. These ZTs if compared at 773 K with the ZT of materials carried out in the present work, ZT of all the state-of-the-art HH materials found to be smaller at 773 K. Moreover, the expensive elements like Hf or Zr and toxic element Pb involved in such HH materials limit their large-scale industrial application and making them economically non-profitable and also have a harsh impact on the environment. It is worth mentioning here that TiNiSn-based nanostructured bulk nanocomposite studied in the present work is the most cost-effective and non-toxic alternative option with such reasonably high ZT along with easy synthesis route can provide an emerging pathway for large-scale applications.
Compatibility factor
The thermoelectric compatibility factor (S) is an essential parameter to be calculated for designing the highly efficient TE devices [61]. S (T, α) is given by:
This factor gives an idea about the feasibility of the materials with another counterpart to be deployed in thermoelectric devices. Figure 8a displays the estimated value of compatibility factor of Ti9Ni7Sn8 Nano-BNC which has been compared with that of bare TiNiSn HH and Ti9Ni7Sn8 BNC with increasing temperature. The compatibility factor of Ti9Ni7Sn8 Nano-BNC was observed to be ~ – 3.9 V−1 at 773 K. This value of S is much higher than that of the bare TiNiSn HH sample and BNC sample, as shown in Fig. 8a, which is ascribed to simultaneous effect of high ZT and low α at high temperature.
The compatibility factors of the present materials are compared with reported materials, as shown in Fig. 8b. “The comparison reveals that Ti9Ni7Sn8 (Nano-BNC) exhibits reasonably good compatibility factor which is either slightly larger in comparison to few materials or even comparable to few other reported materials as depicted in Fig. 8. Thus, it can be noticed that the present material offers possibility of high probability of being segmented with even other class of compounds other than its own family of compounds. However, it is always suggestive to use materials with similar Compatibility Factor for p and n-type components for thermoelectric device fabrication.” This material has a broad range for segmentation with other TE materials (approximately 2 times) to yield high TE device efficiency, benefitted in TE power production [62,63,64,65].
Concluding remarks and future prospects
In the present study, effect of nanostructuring of our previously reported bulk nanocomposite Ti9Ni7Sn8 has been investigated for the optimization of high ZT. Interestingly, a significant reduction in thermal conductivity without deteriorating electronic transport properties is observed due the synergistic effect of nanostructuring of bulk nanocomposite (i.e., Ti9Ni7Sn8 BNC). Nanostructuring of BNC sample leads to modify grain and phase boundary scattering which result in enhanced value of ZT ~ 0.83 at 773 K in Nano-BNC sample. This enhanced value of ZT could be realized due to an ultra-low thermal conductivity (κ ~ 1.0 W/mK at 773 K) and due to marginal enhancement in Seebeck coefficient (~ 18% larger in comparison to TiNiSn HH). The severe reduction in k was resulted on account of scattering of all short-to-long-range phonons from the distinct microstructures obtain via several nanostructured grain boundaries and interface boundaries, while an increase in Seebeck coefficient is corroborated to carrier filtering effect. We strongly believe that rapid energy-efficient processed materials employing arc melting followed by mechanical milling provide an easy path for developing such strategically modified TE materials via synergizing two approaches of phase boundary and grain boundary scattering together for optimizing high ZT.
References
Bell, L.E., Francis, J.: DiSalvo: cooling, heating, generating heat with and recovering waste thermoelectric. Science (2008). https://doi.org/10.1126/science.1158899
Qiu, P., Shi, X., Chen, L., Sui, J., Li, J., Liu, Z., Ren, Z., Mori, T., Mao, J.: Other thermoelectric materials. In: Advanced Thermoelectrics: Materials, Contacts, Devices, and Systems (2017)
He, J., Tritt, T.M.: Advances in thermoelectric materials research: Looking back and moving forward, (2017)
Petsagkourakis, I., Tybrandt, K., Crispin, X., Ohkubo, I., Satoh, N., Mori, T.: Thermoelectric materials and applications for energy harvesting power generation. Sci. Technol. Adv. Mater. 19, 836–862 (2018)
Mori, T., Priya, S.: Materials for energy harvesting: At the forefront of a new wave. MRS Bull. 43, 176–180 (2018)
Snyder, G.J., Toberer, E.S.: Complex thermoelectric materials. Nat. Mater. 7, 105–114 (2008). https://doi.org/10.1038/nmat2090
Song, L., Zhang, J., Iversen, B.B.: Thermal stability of p-type Ag-doped Mg 3 Sb 2 thermoelectric materials investigated by powder X-ray diffraction. Phys. Chem. Chem. Phys. 21, 4295–4305 (2019)
Pei, Y., Wang, H., Snyder, G.J.: Band engineering of thermoelectric materials. Adv. Mater. 24, 6125–6135 (2012)
Bhardwaj, A., Chauhan, N.S., Misra, D.K.: Significantly enhanced thermoelectric figure of merit of p-type Mg 3 Sb 2-based Zintl phase compounds via nanostructuring and employing high energy mechanical milling coupled with spark plasma sintering. J. Mater. Chem. A. 3, 10777–10786 (2015)
Mori, T.: Novel principles and nanostructuring methods for enhanced thermoelectrics. Small. 13, 1702013 (2017)
Mao, J., Liu, Z., Zhou, J., Zhu, H., Zhang, Q., Chen, G., Ren, Z.: Advances in thermoelectrics. Adv. Phys. 67, 69–147 (2018)
Tsujii, N., Nishide, A., Hayakawa, J., Mori, T.: Observation of enhanced thermopower due to spin fluctuation in weak itinerant ferromagnet. Sci. Adv. 5, eaat5935 (2019)
LeBlanc, S., Yee, S.K., Scullin, M.L., Dames, C., Goodson, K.E.: Material and manufacturing cost considerations for thermoelectrics. Renew. Sustain. Energy Rev. 32, 313–327 (2014)
Yazawa, K., Shakouri, A.: Cost-efficiency trade-off and the design of thermoelectric power generators. Environ. Sci. Technol. 45, 7548–7553 (2011)
Müchler, L., Yan, B., Casper, F., Chadov, S., Felser, C.: Topological insulators. Spring Ser. Mater. Sci. 182, 123–139 (2013)
Yu, J., Xia, K., Zhao, X., Zhu, T.: High performance p-type half-Heusler thermoelectric materials. J. Phys. D. Appl. Phys. 51, 113001 (2018)
Huang, L., Zhang, Q., Yuan, B., Lai, X., Yan, X., Ren, Z.: Recent progress in half-Heusler thermoelectric materials. Mater. Res. Bull. 76, 107–112 (2016)
Gurth, M., Grytsiv, A., Vrestal, J., Romaka, V.V., Giester, G., Bauer, E., Rogl, P.: On the constitution and thermodynamic modelling of the system Ti-Ni-Sn. RSC Advances. 5, 92270–92291 (2015)
Kirievsky, K., Gelbstein, Y., Fuks, D.: Phase separation and antisite defects in the thermoelectric TiNiSn half-Heusler alloys. J. Solid State Chem. 203, 247–254 (2013)
Wang Chai, Y., Kimura, Y.: Nanosized precipitates in half-Heusler TiNiSn alloy. Appl. Phys. Lett. 100, 33114 (2012)
Hinterleitner, B., Knapp, I., Poneder, M., Shi, Y., Müller, H., Eguchi, G., Eisenmenger-Sittner, C., Stöger-Pollach, M., Kakefuda, Y., Kawamoto, N., Guo, Q., Baba, T., Mori, T., Ullah, S., Chen, X.-Q., Bauer, E.: Thermoelectric performance of a metastable thin-film Heusler alloy. Nature 576, 85–90 (2019)
Bhardwaj, A., Chauhan, N.S., Sancheti, B., Pandey, G.N., Senguttuvan, T.D., Misra, D.K.: Panoscopically optimized thermoelectric performance of a half-Heusler/full-Heusler based in situ bulk composite Zr 0.7 Hf 0.3 Ni 1+x Sn: An energy and time efficient way. Phys. Chem. Chem. Phys. 17, 30090–30101 (2015). https://doi.org/10.1039/c5cp05213k
Misra, D.K., Bhardwaj, A., Singh, S.: Enhanced thermoelectric performance of a new half-Heusler derivative Zr 9 Ni 7 Sn 8 bulk nanocomposite: enhanced electrical conductivity and low thermal conductivity. J. Mater. Chem. A. 2, 11913–11921 (2014)
Sakurada, S., Shutoh, N.: Effect of Ti substitution on the thermoelectric properties of (Zr, Hf) NiSn half-Heusler compounds. Appl. Phys. Lett. 86, 82105 (2005)
Downie, R.A., Barczak, S.A., Smith, R.I., Bos, J.-W.G.: Compositions and thermoelectric properties of XNiSn (X= Ti, Zr, Hf) half-Heusler alloys. J. Mater. Chem. C. 3, 10534–10542 (2015)
Gandi, A.N., Schwingenschlögl, U.: Electron dominated thermoelectric response in MNiSn (M: Ti, Zr, Hf) half-Heusler alloys. Phys. Chem. Chem. Phys. 18, 14017–14022 (2016). https://doi.org/10.1039/C6CP01786J
Chauhan, N.S., Bathula, S., Vishwakarma, A., Bhardwaj, R., Johari, K.K., Gahtori, B., Dhar, A.: Enhanced thermoelectric performance in p-type ZrCoSb based half-Heusler alloys employing nanostructuring and compositional modulation. J. Mater. 5, 94–102 (2019)
Yan, X., Liu, W., Chen, S., Wang, H., Zhang, Q., Chen, G., Ren, Z.: Thermoelectric Property Study of Nanostructured p‐Type Half‐Heuslers (Hf, Zr, Ti) CoSb0. 8Sn0. 2. Adv. Energy Mater. 3, 1195–1200 (2013)
Rausch, E., Balke, B., Ouardi, S., Felser, C.: Enhanced thermoelectric performance in the p-type half-Heusler (Ti/Zr/Hf) CoSb 0.8 Sn 0.2 system via phase separation. Phys. Chem. Chem. Phys. 16, 25258–25262 (2014)
Shen, J., Fu, C., Liu, Y., Zhao, X., Zhu, T.: Enhancing thermoelectric performance of FeNbSb half-Heusler compound by Hf-Ti dual-doping. Energy Storage Mater. 10, 69–74 (2018)
Silpawilawan, W., Kurosaki, K., Ohishi, Y., Muta, H., Yamanaka, S.: FeNbSb p-type half-Heusler compound: beneficial thermomechanical properties and high-temperature stability for thermoelectrics. J. Mater. Chem. C. 5, 6677–6681 (2017)
Fu, C., Zhu, T., Liu, Y., Xie, H., Zhao, X.: Band engineering of high performance p-type FeNbSb based half-Heusler thermoelectric materials for figure of merit zT> 1. Energy Environ. Sci. 8, 216–220 (2015)
Fu, C., Bai, S., Liu, Y., Tang, Y., Chen, L., Zhao, X., Zhu, T.: Realizing high figure of merit in heavy-band p-type half-Heusler thermoelectric materials. Nat. Commun. 6, 8144 (2015)
Gürth, M., Rogl, G., Romaka, V.V., Grytsiv, A., Bauer, E., Rogl, P.: Thermoelectric high ZT half-Heusler alloys Ti1-x-yZrxHfyNiSn (0≤x≤1; 0≤y≤1). Acta Mater. 104, 210–222 (2016)
Rogl, G., Sauerschnig, P., Rykavets, Z., Romaka, V.V., Heinrich, P., Hinterleitner, B., Grytsiv, A., Bauer, E., Rogl, P.: (V, Nb)-doped half Heusler alloys based on {Ti, Zr, Hf}NiSn with high ZT. Acta Mater. 131, 336–348 (2017)
Poudel, B., Hao, Q., Ma, Y., Lan, Y., Minnich, A., Yu, B., Yan, X., Wang, D., Muto, A., Vashaee, D., Chen, X., Liu, J., Dresselhaus, M.S., Chen, G., Ren, Z.: High-thermoelectric performance of nanostructured bismuth antimony telluride bulk alloys. Science (80). 320, 634–638 (2008). https://doi.org/10.1126/science.1156446
Zhang, X., Zhao, L.-D.: Thermoelectric materials: Energy conversion between heat and electricity. J. Mater. 1, 92–105 (2015)
Zide, J.M.O., Vashaee, D., Bian, Z.X., Zeng, G., Bowers, J.E., Shakouri, A., Gossard, A.C.: Demonstration of electron filtering to increase the Seebeck coefficient in In 0.53 Ga 0.47 As∕ In 0.53 Ga 0.28 Al 0.19 As superlattices. Phys. Rev. B. 74, 205335 (2006)
Vashaee, D., Shakouri, A.: Improved thermoelectric power factor in metal-based superlattices. Phys. Rev. Lett. 92, 106103 (2004)
Vashaee, D., Shakouri, A.: Thermionic power generation at high temperatures using Si Ge∕ Si superlattices. J. Appl. Phys. 101, 53719 (2007)
Misra, D.K., Rajput, A., Bhardwaj, A., Chauhan, N.S., Singh, S.: Enhanced power factor and reduced thermal conductivity of a half-Heusler derivative Ti9Ni7Sn8: a bulk nanocomposite thermoelectric material. Appl. Phys. Lett. (2015). https://doi.org/10.1063/1.4914504
Leblanc, S.: Thermoelectric generators: linking material properties and systems engineering for waste heat recovery applications. Sustain. Mater. Technol. 1–2, 26–35 (2014)
Amatya, R., Ram, R.J.: Trend for thermoelectric materials and their earth abundance. J. Electron. Mater. 41, 1011–1019 (2012)
Joshi, G., Yan, X., Wang, H., Liu, W., Chen, G., Ren, Z.: Enhancement in thermoelectric figure-of-merit of an N-type half-Heusler compound by the nanocomposite approach. Adv. Energy Mater. 1, 643–647 (2011). https://doi.org/10.1002/aenm.201100126
Visconti, A., Navone, C., Leforestier, J., Mingo, N., Bernard-Granger, G.: Influence of the addition of HfO2 particles on the thermoelectric properties of an N-type half-Heusler alloy sintered by spark plasma sintering. J. Alloys Compd. 709, 36–41 (2017)
Faleev, S.V., Léonard, F.: Theory of enhancement of thermoelectric properties of materials with nanoinclusions. Phys. Rev. B. 77, 214304 (2008). https://doi.org/10.1103/PhysRevB.77.214304
Sahoo, P., Liu, Y., Poudeu, P.F.P.: Nanometer-scale interface engineering boosts the thermoelectric performance of n-type Ti 0.4 Hf 0.6 Ni 1+ z Sn 0.975 Sb 0.025 alloys. J. Mater. Chem. A. 2, 9298–9305 (2014)
Sahoo, P., Liu, Y., Makongo, J.P.A., Su, X.-L., Kim, S.J., Takas, N., Chi, H., Uher, C., Pan, X., Poudeu, P.F.P.: Enhancing thermopower and hole mobility in bulk p-type half-Heuslers using full-Heusler nanostructures. Nanoscale. 5, 9419–9427 (2013)
Makongo, J.P.A., Misra, D.K., Zhou, X., Pant, A., Shabetai, M.R., Su, X., Uher, C., Stokes, K.L., Poudeu, P.F.P.: Simultaneous large enhancements in thermopower and electrical conductivity of bulk nanostructured half-Heusler alloys. J. Am. Chem. Soc. 133, 18843–18852 (2011)
Chauhan, N.S., Bhardwaj, A., Senguttuvan, T.D., Pant, R.P., Mallik, R.C., Misra, D.K.: A synergistic combination of atomic scale structural engineering and panoscopic approach in p-type ZrCoSb-based half-Heusler thermoelectric materials for achieving high ZT. J. Mater. Chem. C. 4, 5766–5778 (2016)
Nolas 1962-, G.S. (George S..: Thermoelectrics : basic principles and new materials developments / G.S. Nolas, J. Sharp, H.J. Goldsmid. Springer, Berlin (2001)
Bytenskii, L.I., Gudkin, T.S., Iordanishvili, E.K., Kazmin, S.A., Kaidanov, V.I., Nemov, S.A., Ravich, Y.I.: Influence of potential barriers on thermoelectric properties of lead chalcogenide films. Sov. Phys. Semicond. 11, 894–896 (1977)
Huang, X.Y., Xu, Z., Chen, L.D.: The thermoelectric performance of ZrNiSn/ZrO2 composites. Solid State Commun. 130, 181–185 (2004)
Kim, H.-S., Gibbs, Z.M., Tang, Y., Wang, H., Snyder, G.J.: Characterization of Lorenz number with Seebeck coefficient measurement. APL Mater. 3, 41506 (2015)
Wang, J., Carson, J.K., North, M.F., Cleland, D.J.: A new approach to modelling the effective thermal conductivity of heterogeneous materials. Int. J. Heat Mass Transf. 49, 3075–3083 (2006)
Liu, Y., Xie, H., Fu, C., Snyder, G.J., Zhao, X., Zhu, T.: Demonstration of a phonon-glass electron-crystal strategy in (Hf, Zr) NiSn half-Heusler thermoelectric materials by alloying. J. Mater. Chem. A. 3, 22716–22722 (2015)
He, R., Zhu, H., Sun, J., Mao, J., Reith, H., Chen, S., Schierning, G., Nielsch, K., Ren, Z.: Improved thermoelectric performance of n-type half-Heusler MCo1-xNixSb (M= Hf, Zr). Mater. Today Phys. 1, 24–30 (2017)
Mao, J., Zhou, J., Zhu, H., Liu, Z., Zhang, H., He, R., Chen, G., Ren, Z.: Thermoelectric properties of n-type ZrNiPb-based half-Heuslers. Chem. Mater. 29, 867–872 (2017)
He, R., Huang, L., Wang, Y., Samsonidze, G., Kozinsky, B., Zhang, Q., Ren, Z.: Enhanced thermoelectric properties of n-type NbCoSn half-Heusler by improving phase purity. Apl Mater. 4, 104804 (2016)
Yu, C., Zhu, T.-J., Shi, R.-Z., Zhang, Y., Zhao, X.-B., He, J.: High-performance half-Heusler thermoelectric materials Hf1− x ZrxNiSn1− ySby prepared by levitation melting and spark plasma sintering. Acta Mater. 57, 2757–2764 (2009)
Snyder, G.J., Ursell, T.S.: Thermoelectric efficiency and compatibility. Phys. Rev. Lett. 91, 148301 (2003)
Snyder, G.J.: Application of the compatibility factor to the design of segmented and cascaded thermoelectric generators. Appl. Phys. Lett. 84, 2436–2438 (2004)
Maciá, E.: Compatibility factor of segmented thermoelectric generators based on quasicrystalline alloys. Phys. Rev. B. 70, 100201 (2004)
Brown, S.R., Kauzlarich, S.M., Gascoin, F., Snyder, G.J.: Yb14MnSb11: New high efficiency thermoelectric material for power generation. Chem. Mater. 18, 1873–1877 (2006)
Ngan, P.H., Christensen, D.V., Snyder, G.J., Hung, L.T., Linderoth, S., Nong, N.V., Pryds, N.: Towards high efficiency segmented thermoelectric unicouples. Phys. Status Solid. 211, 9–17 (2014)
Acknowledgements
Authors highly acknowledge Director, NPL, New Delhi for encouragement and support. One of the authors, Ashish Kumar, acknowledges the financial support to JRF- DST-Inspire. Dr. Aman Bhardwaj especially acknowledges DST-SERB for NPDF award (PDF/2017/001250). AB acknowledges Ministry of Education and Science of the Russian Federation in the framework of increase Competitiveness Program of NUST “MISIS” (Grant No. К3-2017–061).
Author information
Authors and Affiliations
Corresponding authors
Ethics declarations
Conflict of interest
The authors declare that there have no conflicts of interest.
Code availability
Not applicable.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article's Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article's Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Kumar, A., Chaturvedi, K.M., Bhardwaj, A. et al. Implication of nanostructuring of bulk nanocomposite Ti9Ni7Sn8 on the optimization of high thermoelectric performance. Mater Renew Sustain Energy 9, 12 (2020). https://doi.org/10.1007/s40243-020-00172-8
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s40243-020-00172-8